The Atmos Clock: Perpetual Motion Machine

reply to post by Mary Rose



I know what he's saying, and it's incorrect. You don't use different units for different ways the same amount of heat is applied. Heat is heat. It doesn't matter what's being heated,or how the heat got there. He's absolutely dead incorrect. A Watt is a Watt. A calorie is a calorie. A gram of water is the same mass as a gram of lead. It should NOT be different. And it isn't different.

reply to post by Mary Rose

Mary, bedlam is right. There is no question about the value of the units, because we define them. So a watt is a watt is a watt, and can never be anything else because we define that's what it is. There are very esoteric discussions about some of our unit definitions that have no practical bearing on the topics we are discussing here...like one unit that we still need to convert from a physical standard to a definition standard is the unit of mass. But the discrepancies between mass standards are teeny tiny fractions of a percent, so those tiny fractions of a percent wouldn't explain the type of differences that are being claimed.

The efficiency of a machine is generally expressed in the same units, like the light bulb example I gave earlier could be expressed in watts of power in versus watts of light output. So look at efficiency of converting electrical power to light (and heat, but the heat is usually waste since the light is usually what we want, with a few exceptions). These are rough numbers, exact values of course will depend on the exact bulb:

Incandescent: power in: 60W Light out: 6W Heat out: 54W Light conversion efficiency: 10%
Fluorescent: power in: 60W Light out: 12W Heat out: 48W Light conversion efficiency: 20%
LED array: power in: 60W Light out: 57W Heat out: 3W Light conversion efficiency: 95%

Actually the LED array can go over 100% because it can actually absorb heat from the environment under certain very low current conditions, and convert that to light so you might get:
LED array: power in: 60W Light out: 61W Heat out: -1W Light conversion efficiency: 102%

Something along the lines of that last one has been done in a lab but it may or may not make it to mainstream use for various reasons.

But the main point is, it is the efficiency of conversion which varies from machine to machine, and this is watts out over watts in this example. However to total watts out is always the same as the total watts in, even in the machine that is over 100%, you still have net power 60W in and net power 60W out, as all the above examples do.

This is pretty much true of every machine device or system which converts energy from one form to another, since energy isn't created or destroyed, it's the conservation of energy principle. The efficiency measure is for the output of energy which we consider useful (in the above example, it's light), not the total output, which always equals the input. The only case where you don't get the same total watts out as you do watts in would be with nuclear related technology because then you have conversion of mass to energy. However even in that case, the energy of the mass "lost" (per E=mc^2) is exactly the same as the energy out.

So the claim about energy conversion rates varying from machine to machine is partially correct, as seen in the above example, where the power in is converted to light at rates of 10%, 20%, 95% and 102%.

However all four of those have 60 watts in and 60 watts out total, so in all four examples the total power out equals exactly 100% of the total power in.

Now about the claim of converting watts to BTUs in this slide:

Converting watt-seconds to BTUs has nothing to do with the machine, it's a completely mathematical conversion because we define both units. To use BTUs (energy instead of power) we need a time element so let's run the 60 watts of input for 17.46 seconds (which is 1 BTU) and re-do the table above:

Incandescent: Energy in: 1.0 BTU Light out: .1 BTU Heat out: .9 BTU Light conversion efficiency: 10%
Fluorescent: Energy in: 1.0 BTU Light out: .2 BTU Heat out: .8 BTU Light conversion efficiency: 20%
LED array: Energy in: 1.0 BTU Light out: .95 BTU Heat out: .05 BTU Light conversion efficiency: 95%

So converting watt-seconds to BTU does not depend on the machine. All of those are 60 watts for 17.46 seconds (=1BTU) The conversion efficiency to light is exactly the same as before for the three different machines, and this will hold true whether we express the units in watts, watt-seconds, BTUs, joules, kilojoules, kilowatt-hours, or any other related well defined unit. The claim otherwise on that slide is completely WRONG just as bedlam said.

Now if you don't get that, try this alternate explanation. To convert watt seconds to BTU, go to this calculator online:

extraconversion.com...

Type in any number for watt seconds and it will calculate the BTUs. Notice there is no input for the machine or efficiency, because that's irrelevant. The conversion is always the same. The machine has nothing to do with that particular calculation.

Get it now?

reply to post by Arbitrageur


I need a specific response to my post that addresses it the way I've put it before I can do justice to the time you've graciously put in to explaining this to me (as in I haven't even read it yet).

Please just talk about the modern equivalent of the experiment and the averaging to come up with a "constant" and specifically why Peter Lindemann does not offer a valid criticism.

Mary Rose
Please just talk about the modern equivalent of the experiment and the averaging to come up with a "constant" and specifically why Peter Lindemann does not offer a valid criticism.

Units are not determined by averaging. That's a lie.

Units are determined by definition.

Mary Rose
He said that water at different temperatures requires slightly different amounts of stirring to raise its temperature by more than one degree Fahrenheit.

Aren't the keywords "different amounts of stirring"?

Mary Rose
Aren't the keywords "different amounts of stirring"?

No. There is no stirring in the definition of a Joule, which is the basic unit of energy:

Joule

It is equal to the energy expended (or work done) in applying a force of one newton through a distance of one metre (1 newton metre or N·m), or in passing an electric current of one ampere through a resistance of one ohm for one second.

One joule can also be defined as:

The work required to move an electric charge of one coulomb through an electrical potential difference of one volt, or one '"coulomb volt" (C·V). This relationship can be used to define the volt.
The work required to produce one watt of power for one second, or one "watt second" (W·s) (compare kilowatt hour). This relationship can be used to define the watt.

Where is the stirring? There isn't any.

Two joules will raise the temperature of liquid water twice as much as one joule, but a joule is always the same amount regardless of how much you raise the temperature. You can add joules with a heater, by stirring or other means, but the amount of a joule is always the same, by definition.

reply to post by Arbitrageur


Let's go back to 1845, when Joule published based on a series of experiments whereby heat was produced in four different ways:



Lindemann said that while each experiment produced slightly different conversion rates, they were all so close that Joule believed the differences were due to measurement error.

This is not necessary for the discussion, but it's interesting to look at the apparatus that was used for the paddle wheel experiment:



Now, again, fast-forwarding to today's equivalent, and the point I'm trying to make: The amount of work required for water at different temperatures is not consistent. It varies. He said there's a rate for just above freezing, 10 degrees, 20 degrees, 30 degrees, etc. Yet we're told there is an absolute conversion rate. He said the rate is generally set at approximately 772 foot-pounds of mechanical work to raise the temperature of one pound of water one degree Fahrenheit.

Mary Rose
From Peter Lindemann's Perpetual Motion Reality, presented at the 2012 Bedini-Lindemann Science and Technology Conference:

Lindemann also talked about the wonders of nitinol:

An alloy of nickel and titanium that has the ability to return to a predetermined shape when heated.

He said that this engine is still running today, and the materials are just getting stronger and stronger:



He said nitinol was discovered in 1961 and during the Carter administration there was a convention to encourage development of it, but not much has happened since.

Peter Lindemann has his own idea for the use of nitinol:



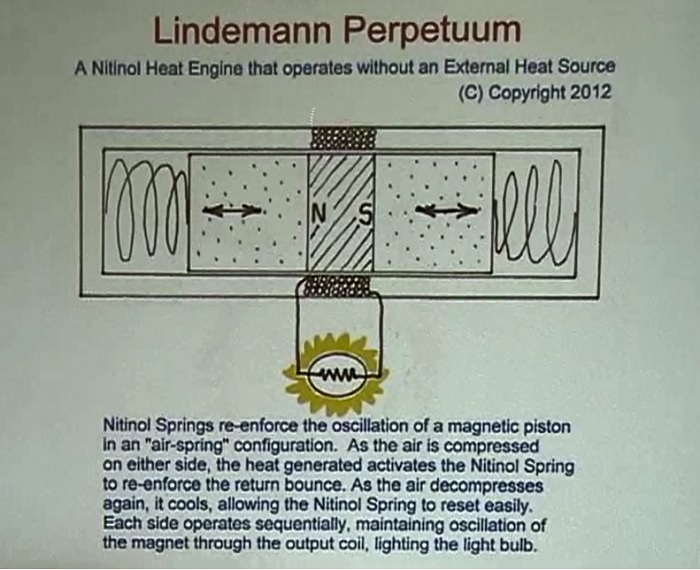
He also has a hypothesis that nitinol does not actually convert heat into mechanical energy. He believes that what actually happens is that it transitions its crystal structure when there is a temperature change. In other words, we're tapping molecular power instead of atomic power.

reply to post by Mary Rose

The amount of work required for water at different temperatures is not consistent. It varies. He said there's a rate for just above freezing, 10 degrees, 20 degrees, 30 degrees, etc.

What are the different rates? I don't even know if that's correct or not but I'm curious if there's evidence of that. Something beyond "Lindemann said".

DenyObfuscation
What are the different rates? I don't even know if that's correct or not but I'm curious if there's evidence of that. Something beyond "Lindemann said".

The standard heat curve for water in all the textbooks shows a straight line from 0 C to 100C. If the rates at 10C and 20C were different, the line wouldn't be straight. See leg "C":

www.chemtutor.com...

Leg 'A' is the warming of ice. Leg 'B' is the melting of ice. Leg 'C' is the warming of water. Leg 'D' is the boiling of water to steam. Leg 'E' is the warming of steam

So either all the texts are wrong, or the guy making stuff up is wrong. If he's got some really good evidence to prove the texts are wrong that would be interesting, but I'm betting he doesn't.

Arbitrageur

DenyObfuscationSo either all the texts are wrong, or the guy making stuff up is wrong. If he's got some really good evidence to prove the texts are wrong that would be interesting, but I'm betting he doesn't.

I was taught a while back that the average temperature of a pot filled with ice will warm up to 32F, and that temperature will remain constant until the ice has fully melted, then the temperature will rise to 212F, give or take depending on elevation, where it will remain until the water has completely transitioned to steam.

I must confess, this agrees with my observations concerning boiling water to make ramen, spaghetti and other pastas as well as hydrating rice and various 'bean' products.

abecedarian
I was taught a while back that the average temperature of a pot filled with ice will warm up to 32F, and that temperature will remain constant until the ice has fully melted, then the temperature will rise to 212F, give or take depending on elevation, where it will remain until the water has completely transitioned to steam.

Yes, those are shown in the graph I posted, but the claim is the rate at 10 degrees and 20 degrees is different. It's not.

reply to post by Arbitrageur


Is this reference related?

From The Engineering Toolbox: "Water - Specific Volume and Weight Density"

Mary Rose
reply to post by Arbitrageur

 

Is this reference related?

From The Engineering Toolbox: "Water - Specific Volume and Weight Density"

Start here:
Heat, Work and Energy

FYI:

He said nitinol was discovered in 1961

Nitinol was invented in 1959, with the effects that ultimately lead to that invention observed in 1932.

and during the Carter administration there was a convention to encourage development of it, but not much has happened since.

Nitinol production was technologically limited until the 1990's. No convention would have changed that. At current, nitinol is used as fast as it is made, with industry demand significantly higher then production capabilities.

Arbitrageur

abecedarian
I was taught a while back that the average temperature of a pot filled with ice will warm up to 32F, and that temperature will remain constant until the ice has fully melted, then the temperature will rise to 212F, give or take depending on elevation, where it will remain until the water has completely transitioned to steam.

Yes, those are shown in the graph I posted, but the claim is the rate at 10 degrees and 20 degrees is different. It's not.

Hi, guys, back on line now that it's dark. Thought I was on tonight but another group has the customer equipment to themselves, so I'm off to the big city of Bakersfield soon to find something to eat that I didn't cook.

In this case, it's true! Water DOES heat up at different rates depending on the temperature of the water you're heating. That's a very subtle effect, and in most cases (steam engines, turbines etc) it's so small an effect that it doesn't have any effect at all on the outcome.

In calorimetry, you DO have to worry about it. The specific heat for water is listed at STP, like a lot of other constants in chemistry.

But WHY is that true? It's not because of some plot by evil big science, nor is it because of the types of heat that are mixing in the water. It's because water calorimetry is actually pretty complex, and it's related to the same thing you mentioned about the temperature plateaus during phase changes.

As you heat water, the specific heat (that's how much the temperature changes with the addition of a stated unit of heat, Mary) varies with the temperature of the water. For solid water, it's about 2.05 J/(g-k) on average, and for gaseous water it's about 2.08. For liquid water, though, it's a lot higher, more than double, and that varies a little in a non-obvious way as the temperature goes from the triple point to boiling.

So, why does that happen, you ask. Because the water is going through different sorts of changes as you heat it, and it's the same sort of thing that's happening when it's melting or vaporizing. When it's in ice, you have a different sort of molecule-molecule interaction than when it's liquid. In fact, water is one of the few things that's less dense as a solid than as liquid water. That expansion comes because the water molecules are structured a lot differently when they're cold. The heat breaks down that structure, and now you've got liquid water which has a lot more intermolecular action going on. So it has a higher specific heat. As it warms to 30 degrees C, you start pumping less energy into internal excitation of the water and more into the group average velocity (temperature). So at first, you're heating the water but also getting those hydrogen bonds banging around which doesn't come in as a temperature change. As you reach 30C, it becomes easier to heat because more of the incoming heat is going into molecular motion and less into internal molecular states. But above 30C, you start losing input heat to yet another internal excitation mode, and the water becomes "harder to heat" until you hit the phase transition into steam.

Once the water is steam, the specific heat drops by half because all the hydrogen bond interaction is gone - the water is spread out too much, so you're not losing input heat to exciting hydrogen bond modes.

It gets even more complicated - different ice phases have different specific heats for example but it's all the same effect. Input heat doesn't just linearly increase temperature because it's going to be split among all the 'sinks' in the material. Some will cause molecular motion which we measure as temperature. But some will be diverted to whatever intra and inter molecular interactions are going to happen at that temperature, and then you see the specific heat change, sometimes radically. The obvious one is a phase change. But there are not so obvious ones like hitting temperatures that cause peak reactions to some sort of q-m motion, such as scissoring, rocking, rotation and so on, and they'll sop up some of the energy as well.

Mary Rose
Peter Lindemann's Perpetual Motion Reality, presented at the 2012 Bedini-Lindemann Science and Technology Conference

Lindemann said that Helmholtz’s 1847 “Treatise on the Conservation of Energy” begins with the hypothesis that, since no one had ever built a perpetual motion machine that worked, then it must be impossible. If it was impossible, there had to be a reason: There was some natural law preventing their construction. He said the only thing it could be is the conservation of energy.

Lindemann said that initially the premise was considered so speculative, that it was denied publication, but that shortly thereafter, it was considered brilliant, and brought in a new scientific paradigm.

Obviously, to say something hasn’t been done so it can’t be done isn’t scientific.

Also, again:



Lindemann said data derived from a machine does not apply to a different machine. If you made a measurement on a machine, that's what that machine will do, but that doesn't mean that's the only thing that can happen.

Bedlam
In this case, it's true! Water DOES heat up at different rates depending on the temperature of the water you're heating. That's a very subtle effect, and in most cases (steam engines, turbines etc) it's so small an effect that it doesn't have any effect at all on the outcome.

OK I guess I should have said it doesn't change significantly, but you are right about a subtle change.

It looks to me like even if you try to plot this subtle change on the graph I posted, it will still look like a straight line because the effect is so small. Or if you have a more accurate graph on the same scale, that would be interesting to see

I could claim all the mass standards are different too, but aside from a few scientists who are concerned with ultra-precise measurements, and working on new and better mass standards, for most purposes this difference is not significant.

reply to post by Mary Rose

So are you going to read my post where I give examples of the different energy conversion rates? I make a couple important points in it, but the most important one is that these different conversion rates have no effect on unit conversions as Lindemann claims, and I show examples of why this is true.

Arbitrageur
It looks to me like even if you try to plot this subtle change on the graph I posted, it will still look like a straight line because the effect is so small.

Oh, yeah, it's out at the second and third decimal places, and it's pretty hard to measure. But to be fair, it is there. It just doesn't mean anything in terms of Lindemann's argument, at least not in the way he says it does.

Bedlam
When it's in ice, you have a different sort of molecule-molecule interaction than when it's liquid.

That rings a bell because of Lindemann's hypothesis about nitinol:

Mary Rose
He also has a hypothesis that nitinol does not actually convert heat into mechanical energy. He believes that what actually happens is that it transitions its crystal structure when there is a temperature change. In other words, we're tapping molecular power instead of atomic power.

I'm not making any claims. I'm just trying to fit puzzle pieces together.