It looks like you're using an Ad Blocker.
Please white-list or disable AboveTopSecret.com in your ad-blocking tool.
Thank you.
Some features of ATS will be disabled while you continue to use an ad-blocker.
share:
reply to post by tgidkp
Told you I was a nutter. My c3p0 keeps saying does not compute does not compute.No, but on a serious not, I keep getting error messages when I try the ways I was thinking. I guess I should leave the math up to you cranius titaniouses. I know I some what know what I am doing though because I can duplicate your images using the polester whata whats. lol Sleep deprivation should be left up to those tough badasses in the military. Im gonna quit while Im behind
Good night all. .
Told you I was a nutter. My c3p0 keeps saying does not compute does not compute.No, but on a serious not, I keep getting error messages when I try the ways I was thinking. I guess I should leave the math up to you cranius titaniouses. I know I some what know what I am doing though because I can duplicate your images using the polester whata whats. lol Sleep deprivation should be left up to those tough badasses in the military. Im gonna quit while Im behind
Good night all. .
reply to post by tgidkp
Yes my brain was also screaming biology. if you kept going you would back track your self back to the seed of life.
Yes my brain was also screaming biology. if you kept going you would back track your self back to the seed of life.
Originally posted by ringlejames
reply to post by tgidkp
Yes my brain was also screaming biology. if you kept going you would back track your self back to the seed of life.
Which is where we were going with the "divinity" thing with the images we posted.




The interesting part is that the 'art' is a by-product of what the 'real' goal is.
Peace Love Light
tfw
[align=center][color=magenta]Liberty & Equality or Revolution[/align]
Originally posted by tgidkp
Originally posted by ringlejames
reply to post by tgidkp
What would happen if you reversed all the tag values for all the numbers. Meaning what would happen if you made all the negative numbers positive and all the positives negative.
that is an extremely astute observation....especially for someone that doesnt quite know what he is talking about!
Thanks I constantly work with tag values in my line of fun/work. That being blackjack. If you really like math like you seem too, you would be really good at blackjack. I have the Holy Grail of Gambling books, which cost $2000-$3500, on my dropbox account. If you are interested, I'll give you the link in a PM. I work with standard deviations, Expected value formulas along with a list of others all the time in blackjack. Extremely easy math. I think you could have quite a bit of fun learning the game and make a "few" dollars will your at it. lol
Cheers man.
edit on 25-3-2012 by ringlejames because: (no reason given)
well, i have spent all day (again) on this thing.
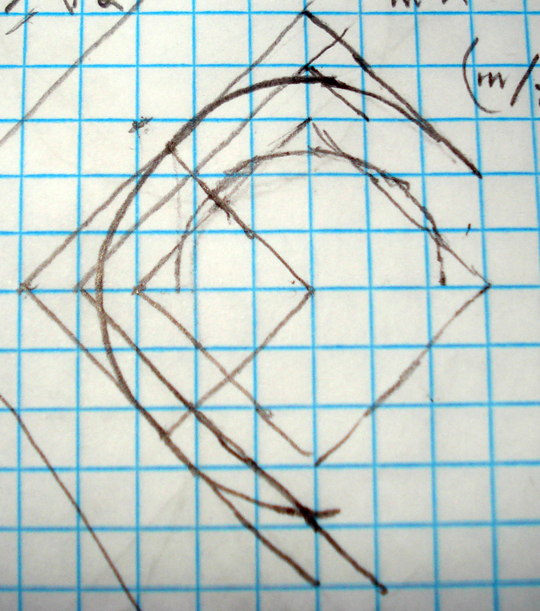
i went back to the old drawing board. below are images from my notebook in which i spent a good deal of time learning how to "square the circle". that is what got me started down this path in the first place.
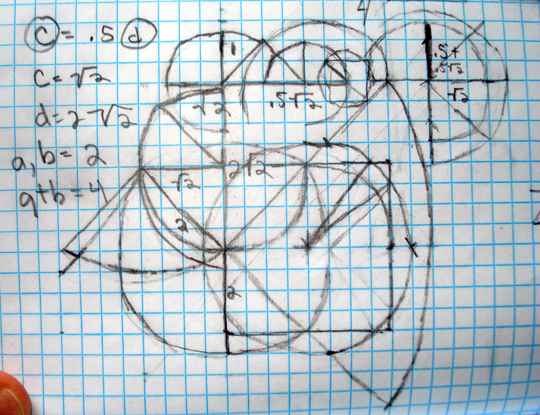
i made a 3D model of the unit lengths on the graphs (below), which also appear in my chaotic function.
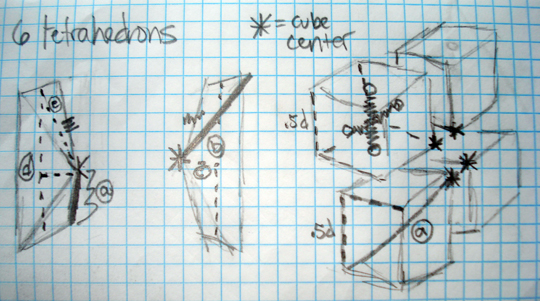
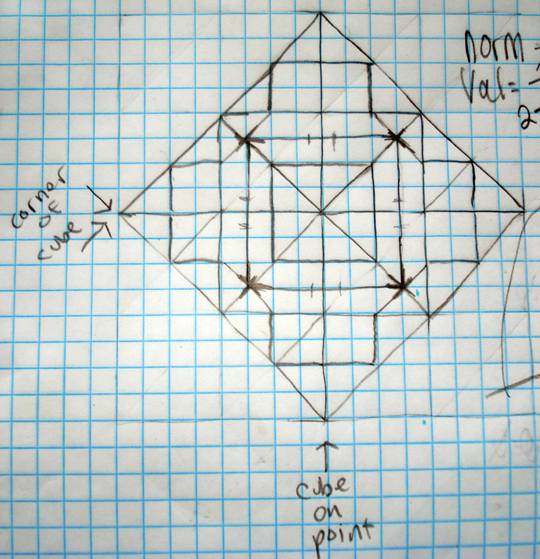
CUBIC SECTIONS
and then, finally, i worked-up a summary of the process by which i used all of the above geometrical units to "bootstrap" the chaotic function series. the summary is contained as a web-page with a java application embedded in it. there is a page showing the functions from the ground-up, and another page (scroll to the right) with a plot of the function.
i still am really hoping that i can draw the attention of someone who can help me pull all of this together. a long shot, i am sure.

attractor-series_java_webpage
....circlemaker, where are you?!
i went back to the old drawing board. below are images from my notebook in which i spent a good deal of time learning how to "square the circle". that is what got me started down this path in the first place.
i made a 3D model of the unit lengths on the graphs (below), which also appear in my chaotic function.
CUBIC SECTIONS
and then, finally, i worked-up a summary of the process by which i used all of the above geometrical units to "bootstrap" the chaotic function series. the summary is contained as a web-page with a java application embedded in it. there is a page showing the functions from the ground-up, and another page (scroll to the right) with a plot of the function.
i still am really hoping that i can draw the attention of someone who can help me pull all of this together. a long shot, i am sure.





attractor-series_java_webpage
....circlemaker, where are you?!
reply to post by tgidkp
I checked out your root3 interactive example and I see the code/math area but got an error at the bottom of the page. Is that where a render is supposed to show up?
Anyway your last post has familiar geometries. It looks to me like you're analyzing the ratios between the cube and octahedron which combine in this way (by connecting all the points) to form a tesseract. I'm a little lost in how this relates to the other (much more complicated) imagery.
Since you've expressed an interest in sacred geometry you may find this interesting:

The above are xyz perspective views.
The root circle geometry (3D sine wave) wraps around the tesseract (oct/cube combo). It's like a curved solution to metatron's cube and as you can see can be easily extrapolated to include the seed of life and flower of life geometries via duplication/division. But here it's all in 3D which makes it even cooler.
Here's a xy view of the first image for reference:
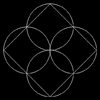
It looks exactly the same from the top and side, but when rotated so that the cube/oct looks like a hexagon the FOL geometries become apparent.
I checked out your root3 interactive example and I see the code/math area but got an error at the bottom of the page. Is that where a render is supposed to show up?
Anyway your last post has familiar geometries. It looks to me like you're analyzing the ratios between the cube and octahedron which combine in this way (by connecting all the points) to form a tesseract. I'm a little lost in how this relates to the other (much more complicated) imagery.
Since you've expressed an interest in sacred geometry you may find this interesting:

The above are xyz perspective views.
The root circle geometry (3D sine wave) wraps around the tesseract (oct/cube combo). It's like a curved solution to metatron's cube and as you can see can be easily extrapolated to include the seed of life and flower of life geometries via duplication/division. But here it's all in 3D which makes it even cooler.
Here's a xy view of the first image for reference:

It looks exactly the same from the top and side, but when rotated so that the cube/oct looks like a hexagon the FOL geometries become apparent.
reply to post by circlemaker
....from the wiki Rhombic_dodecahedron
this is sooooo close to the answer! but the problem with this statement is that the appropriate sphere (circle) that is to be drawn using these ratios is neither nor circumscribed.
rather, as i have shown (without sufficient explanation) in the first image taken from my notebook, the squared-circle is a mean value between two neighboring diagonal lengths. such that, the circle straddles (wraps around) the square. similarly, the "cubed sphere" must also straddle the cube.
so, i have taken the cubic diagonals and computing trigonometric functions for them.
to effectively "cube the sphere", i had to find the mean value between the sqrt(2) and sqrt(3). this mean value looks very much like the overlapping sections of the circles in the pictures you posted. (btw, i had seen these pics before but never realized that they are x,y,z. it took me several minutes of focus for them to "pop-out" into three dimensions. so awesome!)
i started this before i started trig this semester, and so it took me a REALLY long time to figure out that the mean value is equivalent to ((sqrt(3)/2))+((sqrt(2)/2))=1.573132184971. it is important to note that i am finding the nonlinear (irrational) mean of the diagonal length, NOT the equivalent mean using the integers. so the radius of the sphere tangent to the length of ((sqrt(3)/2))+((sqrt(2)/2))=1.573132184971 using the "straddling" mean-value method is:
please compare this with the above values. all of this is shown in the java app example. also, notice that this number and multiples of this number have been found in the shape of the great pyramid....but that is beyond the point.
what i unexpectedly found is that the radius of a sphere at sqrt(3), when plotted on an x-y axis, produces what APPEARS to be an asymptote at integer 3. but IN FACT, as can be seen from the function plot in the java app, it is not an asymptote but rather an infinitely receding curve to infinity.
i have taken this to mean that, while the ratio of pi describes the CURVE (en.wikipedia.org...) of the sphere (circle), it does not describe the BOUNDARY of the sphere (circle).
the boundary is defined as an infinitely receding recursion toward the attractor at the unit length of the sphere (circle).
so, for the unit circle (sqrt(1)), the boundary is defined as the attractor at the unit length (sqrt(1)).
for the unit sphere (sqrt(3)), the boundary is defined as the attractor at the unit length (sqrt(3)).
so, how does this produce the fractals? in simplest terms.....
....i had noticed that the solutions for the recursive function (in the java app) were showing a very striking pattern. the first twenty solutions of the recursion using '0' as the starting value of 'x' gives the following values:
so, in order to discover what this pattern contained, i used the z axis to plot the rational values of (x/y). so, in other words, the x,y,z plot would give a solution on the z axis when the ratio of (x/y) is a whole number ratio.
it just so happens that the whole number solutions (on the z axis) of the attractor series (on the x,y axis) produces a solution set which has some fascinating properties.
i have drawn the fractal in order to visualize these properties.
BTW, i know that my answers are always longwinded. sorry about that. in many ways, i am explaining this to myself as much as to you.
thanks, man.
....from the wiki Rhombic_dodecahedron
If the edge length of a rhombic dodecahedron is a, the radius of a sphere (tangent to each of the rhombic dodecahedron's faces) is....
inscribed = 0.8164965809
circumscribed = 1.154700538
this is sooooo close to the answer! but the problem with this statement is that the appropriate sphere (circle) that is to be drawn using these ratios is neither nor circumscribed.
rather, as i have shown (without sufficient explanation) in the first image taken from my notebook, the squared-circle is a mean value between two neighboring diagonal lengths. such that, the circle straddles (wraps around) the square. similarly, the "cubed sphere" must also straddle the cube.
so, i have taken the cubic diagonals and computing trigonometric functions for them.
to effectively "cube the sphere", i had to find the mean value between the sqrt(2) and sqrt(3). this mean value looks very much like the overlapping sections of the circles in the pictures you posted. (btw, i had seen these pics before but never realized that they are x,y,z. it took me several minutes of focus for them to "pop-out" into three dimensions. so awesome!)
i started this before i started trig this semester, and so it took me a REALLY long time to figure out that the mean value is equivalent to ((sqrt(3)/2))+((sqrt(2)/2))=1.573132184971. it is important to note that i am finding the nonlinear (irrational) mean of the diagonal length, NOT the equivalent mean using the integers. so the radius of the sphere tangent to the length of ((sqrt(3)/2))+((sqrt(2)/2))=1.573132184971 using the "straddling" mean-value method is:
(((√(6))/(4))+((1)/(2))) = 1.1123724356958
please compare this with the above values. all of this is shown in the java app example. also, notice that this number and multiples of this number have been found in the shape of the great pyramid....but that is beyond the point.
what i unexpectedly found is that the radius of a sphere at sqrt(3), when plotted on an x-y axis, produces what APPEARS to be an asymptote at integer 3. but IN FACT, as can be seen from the function plot in the java app, it is not an asymptote but rather an infinitely receding curve to infinity.
i have taken this to mean that, while the ratio of pi describes the CURVE (en.wikipedia.org...) of the sphere (circle), it does not describe the BOUNDARY of the sphere (circle).
the boundary is defined as an infinitely receding recursion toward the attractor at the unit length of the sphere (circle).
so, for the unit circle (sqrt(1)), the boundary is defined as the attractor at the unit length (sqrt(1)).
for the unit sphere (sqrt(3)), the boundary is defined as the attractor at the unit length (sqrt(3)).
so, how does this produce the fractals? in simplest terms.....
....i had noticed that the solutions for the recursive function (in the java app) were showing a very striking pattern. the first twenty solutions of the recursion using '0' as the starting value of 'x' gives the following values:
[1.,0.50000000000003,0.40000000000002,0.33333333333336,0.28571428571429,0.25000000000001,
0.22222222222223,0.20000000000001,0.18181818181819,0.16666666666667,0.15384615384616,
0.14285714285715,0.13333333333334,0.12500000000001,0.11764705882354,0.11111111111112,
0.10526315789474,0.10000000000001,0.095238095238101,0.090909090909095,0.086956521739134]
so, in order to discover what this pattern contained, i used the z axis to plot the rational values of (x/y). so, in other words, the x,y,z plot would give a solution on the z axis when the ratio of (x/y) is a whole number ratio.
it just so happens that the whole number solutions (on the z axis) of the attractor series (on the x,y axis) produces a solution set which has some fascinating properties.
i have drawn the fractal in order to visualize these properties.
BTW, i know that my answers are always longwinded. sorry about that. in many ways, i am explaining this to myself as much as to you.
thanks, man.
edit on 26-3-2012 by tgidkp because: (no reason given)
reply to post by tgidkp
could you send me the software for these patterns..i want it on my windows pc...
how long does it take to generate them?
could you send me the software for these patterns..i want it on my windows pc...
how long does it take to generate them?
reply to post by tgidkp
i am vey happy to see your progress,
the answer is within your grasp,
sorry to be criptic,
but
if you look to a true space time manifold, it will unravel for you
exellent advances
keep up the good work
your friend,
xploder
i am vey happy to see your progress,
the answer is within your grasp,
sorry to be criptic,
but
if you look to a true space time manifold, it will unravel for you
exellent advances
keep up the good work
your friend,
xploder
edit on 26-3-2012 by XPLodER because: (no reason given)
Originally posted by tgidkp
....from the wiki Rhombic_dodecahedron
If the edge length of a rhombic dodecahedron is a, the radius of a sphere (tangent to each of the rhombic dodecahedron's faces) is....
inscribed = 0.8164965809
circumscribed = 1.154700538
Just pointing this out in case you missed it...
1.155 = the square root of 3/2
0.816 = the square root of 2/3 (it's reciprocal)
it just so happens that the whole number solutions (on the z axis) of the attractor series (on the x,y axis) produces a solution set which has some fascinating properties.
Indeed! While counting in sequential square roots on the x & y axis while simultaneously counting in whole numbers on the z axis, some interesting connections show up which can relate to sine, cosine, and tangent as they relate in 3D. Typically these values are only understood in 2D.

Some of the language is over my head but I'm doing my best to follow and point out the connections I know of.
reply to post by circlemaker
yes. this is a very similar depiction of what the initial solution set of the attractor series looks like in 3D. this makes sense because it represents a function sine and cosine.
now i will speak to the image you have just posted as though it were my solution set.
if you take the values of the 3D sine and subtract the values of 3D cosine, you should get unity. also, if you take a ratio of those values, you will get unity.
so, i have a similar solution set that wraps around its inverse in 3D. this looks very much like if you interlock your thumb and forefinger on one hand with the other. (i can upload this image if you like.)
but performing the above difference and ratio operations to these solutions does not yield a null value. rather, there is a tiny, tiny sliver of values (see the number pairs image below) which oscillate around each other as they progress to infinity in both directions (above 1 and between 1 and 0).

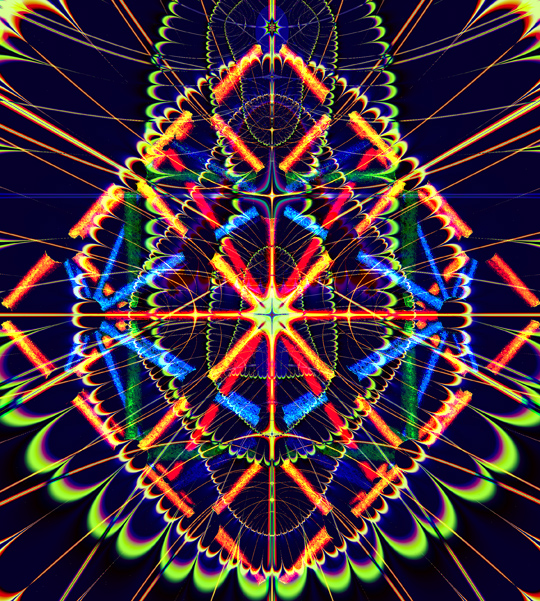
when you look at the fractal images, especially the most recent one (download this), you are seeing the convergence and divergence of this residual value as the functions relate themselves to the whole numbers (plotted on the x axis). you should also notice the difference of one number pair to its neighboring pairs in the series. so weird.
i have thoroughly verified that all of the most interesting artifacts in the fractal image occur at the positions of the whole numbers along the x axis. if i recall correctly, the two largest starburst formations occur at -3 and -7....
i am interested to analyze these whole number solutions against the formations that they produce, as i think there may be useful information there. but i have been waaaay too busy focusing on other aspects.
for example. i discovered this morning that i can form a line segment of the "mean value" length using my geometry set which i used to produce the images in the thread from last night. when i get home tonight, i am going to plug those in and find out how these new lines interact with the rhombic dodecahedron.
should be pretty interesting.
yes. this is a very similar depiction of what the initial solution set of the attractor series looks like in 3D. this makes sense because it represents a function sine and cosine.
now i will speak to the image you have just posted as though it were my solution set.
if you take the values of the 3D sine and subtract the values of 3D cosine, you should get unity. also, if you take a ratio of those values, you will get unity.
so, i have a similar solution set that wraps around its inverse in 3D. this looks very much like if you interlock your thumb and forefinger on one hand with the other. (i can upload this image if you like.)
but performing the above difference and ratio operations to these solutions does not yield a null value. rather, there is a tiny, tiny sliver of values (see the number pairs image below) which oscillate around each other as they progress to infinity in both directions (above 1 and between 1 and 0).

when you look at the fractal images, especially the most recent one (download this), you are seeing the convergence and divergence of this residual value as the functions relate themselves to the whole numbers (plotted on the x axis). you should also notice the difference of one number pair to its neighboring pairs in the series. so weird.
i have thoroughly verified that all of the most interesting artifacts in the fractal image occur at the positions of the whole numbers along the x axis. if i recall correctly, the two largest starburst formations occur at -3 and -7....
i am interested to analyze these whole number solutions against the formations that they produce, as i think there may be useful information there. but i have been waaaay too busy focusing on other aspects.
for example. i discovered this morning that i can form a line segment of the "mean value" length using my geometry set which i used to produce the images in the thread from last night. when i get home tonight, i am going to plug those in and find out how these new lines interact with the rhombic dodecahedron.
should be pretty interesting.
Originally posted by tgidkp
reply to post by SevenThunders
I think that I understand your hangup. I am sure its my fault, not yours.
after you have written the function, you must run operate it as z,y) NOT x,y)
this is a "lost in the translation" between my calculator and the rendering program.
likewise, if you are attempting to iterate it directly in the rendering program, replace all variables 'x' with 'z'.
so then, 'ultra fractal', the program I am using, uses a loop/endloop format...just like I have shown.
literally, if you paste the functions as I have shown (replacing z for x) between loop and endloop tags you should get the same results.
I get the feeling this doesn't help much.
I will check out the python. I may have some time once my semester has ended.
thank you very much for your help.
Sorry that hasn't helped me understand. Your z10(x,y) and z11(x,y) functions are just the negation of each other. That is z10(x,y) = -z11(x,y) . Thus all iterations lie on the line x + y = 0, and can not produce the fractals you are showing. You must be using a nonstandard way of generating your fractals. I don't understand how renaming variables is going to help. I notice also you are declaring your variables as complex, but do not have any complex constants in your function. Does your iterator generate complex inputs?
reply to post by SevenThunders
there is a fairly hefty formula hiding behind both z10 and z11. these formulas and their calculations were formulated without complex numbers. the z plane and its resulting 3D form has been used only to derive a very specific set of solutions to formulas which came from a previous part of my process. (the details of this process are given in the second post of the OP.)
so, z10 and z11 as i have given them are approximations of two formulas which, in their exact form are both really really huge and do not negate each other (the approximations read as...999999999 and .333333333, etc)
in the final step in this image....

....you must take the results of z11(x,y) and plug them as coordinates of z10(x,y)
this will yield a solution set which must satisfy the domain requirements of both formulas. being that they are almost (but not quite) negations of one another, their shared domains yield the complex solutions that are rendered in the images.
you then take the results from the last step (z10(z11,z11)) and plug them back into z11 (z11(z10(z11,z11),z10(z11,z11))) etc.
i am sorry that this is so difficult to understand. now you see why i have asked for help. we are both using language that the other does not understand.
the software i am using (ultra fractal) is responsible for drawing the pixels. i have no idea how to program that. i made a screen grab of the 'LOOP' section, which appears in a previous post.
thanks for coming back! i hope that this is a little more clear.
there is a fairly hefty formula hiding behind both z10 and z11. these formulas and their calculations were formulated without complex numbers. the z plane and its resulting 3D form has been used only to derive a very specific set of solutions to formulas which came from a previous part of my process. (the details of this process are given in the second post of the OP.)
so, z10 and z11 as i have given them are approximations of two formulas which, in their exact form are both really really huge and do not negate each other (the approximations read as...999999999 and .333333333, etc)
in the final step in this image....

....you must take the results of z11(x,y) and plug them as coordinates of z10(x,y)
this will yield a solution set which must satisfy the domain requirements of both formulas. being that they are almost (but not quite) negations of one another, their shared domains yield the complex solutions that are rendered in the images.
you then take the results from the last step (z10(z11,z11)) and plug them back into z11 (z11(z10(z11,z11),z10(z11,z11))) etc.
i am sorry that this is so difficult to understand. now you see why i have asked for help. we are both using language that the other does not understand.
the software i am using (ultra fractal) is responsible for drawing the pixels. i have no idea how to program that. i made a screen grab of the 'LOOP' section, which appears in a previous post.
thanks for coming back! i hope that this is a little more clear.
reply to post by tgidkp
Very cool pics!
I wonder if you put an 8 in the place of all those 9's?
As wishy-washy as this sounds, isn't 8 a symbol of infinity?
Other than that, this is way over my head!
Very cool pics!
I wonder if you put an 8 in the place of all those 9's?
As wishy-washy as this sounds, isn't 8 a symbol of infinity?
Other than that, this is way over my head!
Hmmm... Fractals and forming biological structures?... Emergent patterns... Reminds me of some other ideas.
I thought it would be cool if somebody took that biological soup experiment which forms amino acids and basic lipids, and modified it in two ways. In some of the mixing steps, agitate the mix at various frequencies to create cymatic patterns. Also throw in exposure to magnetic fields during some steps. Both of these could be considered naturally occuring events in a (presumably) random envionment. (Things like waterfalls, and naturally occuring magnetite, etc.) However, I think those would have some profound effects on organizing molecular structures. It would also be interesting to see what magnetic fields do to form complex structures in polar molecules. (Magnetic fields form very complex structures in simple ferro-fluids, so it would be neat to see what effect it has on chemicals that can chain up and lock together in a more persistent way.)
Those things would be considered chaotic systems and possibly fractaline in nature. I wonder how far off the math would be compared to your simulation experiments.
As a layman, I'm surprised there hasn't been much publicly done to modify that "organic soup" experiment in this way. I'm curious if the results would yield more complex compounds.
Unfortunately I'm not up to analyzing your stuff (it's cool though, the fractals look nifty), but figured such an idea might be along your lines of study and worth suggesting.
I thought it would be cool if somebody took that biological soup experiment which forms amino acids and basic lipids, and modified it in two ways. In some of the mixing steps, agitate the mix at various frequencies to create cymatic patterns. Also throw in exposure to magnetic fields during some steps. Both of these could be considered naturally occuring events in a (presumably) random envionment. (Things like waterfalls, and naturally occuring magnetite, etc.) However, I think those would have some profound effects on organizing molecular structures. It would also be interesting to see what magnetic fields do to form complex structures in polar molecules. (Magnetic fields form very complex structures in simple ferro-fluids, so it would be neat to see what effect it has on chemicals that can chain up and lock together in a more persistent way.)
Those things would be considered chaotic systems and possibly fractaline in nature. I wonder how far off the math would be compared to your simulation experiments.
As a layman, I'm surprised there hasn't been much publicly done to modify that "organic soup" experiment in this way. I'm curious if the results would yield more complex compounds.
Unfortunately I'm not up to analyzing your stuff (it's cool though, the fractals look nifty), but figured such an idea might be along your lines of study and worth suggesting.
hello to anyone still following this thread!
i realize that the "freshness date" has passed on this thread, but i am still working on this project every single day and i will continue to use this thread as a repository of my findings.
once again, i have come across a bizarre coincidence. i have produced a rhombic dodecahedron (see the other thread of mine) this time with the "red lines" in place. i was able to identify that the red lines, representing the "mean value" method discussed earlier, were critical to the correct interpretation of the line lengths in the polygon.
so, i made this structure earlier in the week, and just today got around to doing some editing. and i am pleasantly surprised at what happened when i put an overlay of the polygon on top of the fractal render i made last week.
i do not have time to go into further details right now. but if anyone is interested, i will probably post more images and explanations tomorrow.....i am making some very interesting connections ATM.
i realize that the "freshness date" has passed on this thread, but i am still working on this project every single day and i will continue to use this thread as a repository of my findings.
once again, i have come across a bizarre coincidence. i have produced a rhombic dodecahedron (see the other thread of mine) this time with the "red lines" in place. i was able to identify that the red lines, representing the "mean value" method discussed earlier, were critical to the correct interpretation of the line lengths in the polygon.
so, i made this structure earlier in the week, and just today got around to doing some editing. and i am pleasantly surprised at what happened when i put an overlay of the polygon on top of the fractal render i made last week.
i do not have time to go into further details right now. but if anyone is interested, i will probably post more images and explanations tomorrow.....i am making some very interesting connections ATM.

reply to post by XPLodER
That's cool. I just did the measurements myself to check the precision and it's not exact, but still close enough to be significant. For example if the length of the square is 8, the length of the circle is 7.992... That's by using phi in my measurements. If the square and circle are exactly the same length, then phi loses precision instead.
That's cool. I just did the measurements myself to check the precision and it's not exact, but still close enough to be significant. For example if the length of the square is 8, the length of the circle is 7.992... That's by using phi in my measurements. If the square and circle are exactly the same length, then phi loses precision instead.
Originally posted by pauljs75
Hmmm... Fractals and forming biological structures?... Emergent patterns... Reminds me of some other ideas.
I thought it would be cool if somebody took that biological soup experiment which forms amino acids and basic lipids, and modified it in two ways. In some of the mixing steps, agitate the mix at various frequencies to create cymatic patterns. Also throw in exposure to magnetic fields during some steps. Both of these could be considered naturally occuring events in a (presumably) random envionment. (Things like waterfalls, and naturally occuring magnetite, etc.) However, I think those would have some profound effects on organizing molecular structures. It would also be interesting to see what magnetic fields do to form complex structures in polar molecules. (Magnetic fields form very complex structures in simple ferro-fluids, so it would be neat to see what effect it has on chemicals that can chain up and lock together in a more persistent way.)
Those things would be considered chaotic systems and possibly fractaline in nature. I wonder how far off the math would be compared to your simulation experiments.
This has been done before, not quite on the biological soup, but in other contexts.
In fact, it was such a good idea that it was done by Alan Turing, in particular reaction-diffusion equations as a basis for morphogenesis. Started back in the 1950's but probably not really appreciated until the 80's or 90's.
The idea of complex nonlinear, possibly chaotic dynamics in active biological systems as a mechanism for pattern formation is now well established. In particular, the various patterns you may see when you close your eyes in a really darkened room, and especially when on mind-altering hallucinogens, have been quite well correlated with the waves of partial differential equations
www-news.uchicago.edu...
In sum, you're on the right track, but lots of people have been there before. If you want to learn more, get a PhD in mathematical biology with the right dissertation advisor.
By the way, don't get too hung up on 'fractal'---nonlinear dynamics goes beyond 'fractals'.
reply to post by tgidkp
Just found this thread and briefly scanned the responses. Obviously you have been getting some good answers.
Circlemaker quickly picked up on your square root of 3 seeding. As has already been explaned this is very old, Archimedes (chose) 265/153 for his approximation of the square root of 3. We've done 153 as a Christian rune number in the NT book of John to death already. As you hinted there are 23 chromosomes in human DNA And the female X chromosome spans 153 million base pairs so here it shows up again in biochemistry.
Ringlejames interest in blackjack reminds me of some entropy experiments I did years ago involving runs theory.
If you find a casino that doesn't have a huge house advantage your odds of winning or losing at a single hand of blackjack approaches that of a simple coin toss. Many gamblers extend their play by pyramiding bets.
After playing a trillion (consecutive) hands of blackjack you could expect to lose 28 times in a row at some point so pyramiding has its limits. The interesting thing that keeps blackjack on topic for this thread is that an amazing fractal emerges in the run counts of supposedly random coin toss events. All of a sudden some of the randomness disappears and is replaced by destiny.
These days the casinos are retiring 6 deck shoes long before they start dealing out of the last deck so maybe there is something that has NOT been said about making money at blackjack.
My intuition leads me to believe the keys to a better understanding of entropy may lie in the difference between the quantum nature of matter and what may be just a perceived illusion of random chaos. Superfluid plasmas have very low entropy, close to that of their stable chrystalline structures. Perhaps the lack of chaos in a superfluid plasma makes it an ideal media to find fractal patterns of seemingly random noise. This has been done to death in the cryptography world. Digital silicon chip computers using serial operations take to the heat death of the universe to solve problems like the factoring of large composites Quantum computers running massive parallel operations appear to solve the same problem instantly out of time.
The artistic quality of your fractals is excellent, several of us immediately saw the seemingly unrelated meditating figure! For some reason I started thinking about Homers Odyssey where Odysseus spends 17 days on a raft in calm weather before Poseidon sends a powerful storm on the 18th. Perhaps meditation can help free the human soul from the chaotic storms of the mind like that. Oh well probably a good time to stop before I get washed up on the beach with a piano like a true nutter!
Just found this thread and briefly scanned the responses. Obviously you have been getting some good answers.
Circlemaker quickly picked up on your square root of 3 seeding. As has already been explaned this is very old, Archimedes (chose) 265/153 for his approximation of the square root of 3. We've done 153 as a Christian rune number in the NT book of John to death already. As you hinted there are 23 chromosomes in human DNA And the female X chromosome spans 153 million base pairs so here it shows up again in biochemistry.
Ringlejames interest in blackjack reminds me of some entropy experiments I did years ago involving runs theory.
If you find a casino that doesn't have a huge house advantage your odds of winning or losing at a single hand of blackjack approaches that of a simple coin toss. Many gamblers extend their play by pyramiding bets.
After playing a trillion (consecutive) hands of blackjack you could expect to lose 28 times in a row at some point so pyramiding has its limits. The interesting thing that keeps blackjack on topic for this thread is that an amazing fractal emerges in the run counts of supposedly random coin toss events. All of a sudden some of the randomness disappears and is replaced by destiny.
These days the casinos are retiring 6 deck shoes long before they start dealing out of the last deck so maybe there is something that has NOT been said about making money at blackjack.
My intuition leads me to believe the keys to a better understanding of entropy may lie in the difference between the quantum nature of matter and what may be just a perceived illusion of random chaos. Superfluid plasmas have very low entropy, close to that of their stable chrystalline structures. Perhaps the lack of chaos in a superfluid plasma makes it an ideal media to find fractal patterns of seemingly random noise. This has been done to death in the cryptography world. Digital silicon chip computers using serial operations take to the heat death of the universe to solve problems like the factoring of large composites Quantum computers running massive parallel operations appear to solve the same problem instantly out of time.
The artistic quality of your fractals is excellent, several of us immediately saw the seemingly unrelated meditating figure! For some reason I started thinking about Homers Odyssey where Odysseus spends 17 days on a raft in calm weather before Poseidon sends a powerful storm on the 18th. Perhaps meditation can help free the human soul from the chaotic storms of the mind like that. Oh well probably a good time to stop before I get washed up on the beach with a piano like a true nutter!
edit on 2-4-2012 by Cauliflower because: (no reason given)
new topics
-
SCOTUS Chief Justice JOHN ROBERTS Ends 2024 Describing His Fears for Safety of U.S. Judges.
Above Politics: 23 minutes ago -
How we've changed in 100 years
Ancient & Lost Civilizations: 6 hours ago -
US disburses $3.4 billion in budget aid for Ukraine, Yellen says
US Political Madness: 7 hours ago -
Nigel Farage's New Year Message.
Politicians & People: 9 hours ago -
I dont understand what i just witnessed
Social Issues and Civil Unrest: 10 hours ago
top topics
-
I dont understand what i just witnessed
Social Issues and Civil Unrest: 10 hours ago, 11 flags -
US disburses $3.4 billion in budget aid for Ukraine, Yellen says
US Political Madness: 7 hours ago, 11 flags -
Happy New Year from Aussie
General Chit Chat: 13 hours ago, 8 flags -
How we've changed in 100 years
Ancient & Lost Civilizations: 6 hours ago, 7 flags -
Nigel Farage's New Year Message.
Politicians & People: 9 hours ago, 6 flags -
House republicans prove to be drama queens
US Political Madness: 14 hours ago, 5 flags -
The Silent Motion…
Short Stories: 13 hours ago, 5 flags -
SCOTUS Chief Justice JOHN ROBERTS Ends 2024 Describing His Fears for Safety of U.S. Judges.
Above Politics: 23 minutes ago, 1 flags
active topics
-
Happy New Year from Aussie
General Chit Chat • 17 • : bally001 -
SCOTUS Chief Justice JOHN ROBERTS Ends 2024 Describing His Fears for Safety of U.S. Judges.
Above Politics • 1 • : BernnieJGato -
How we've changed in 100 years
Ancient & Lost Civilizations • 11 • : JJproductions -
Simulation theory and have we reset before like a game?
Conspiracies in Religions • 35 • : FullHeathen -
The C.D.C. Says There Was NO INFLUENZA Worth Reporting for the 2020-2021 Flu Season.
Diseases and Pandemics • 54 • : doctordark -
Strange fog all over the northern hemisphere
General Conspiracies • 36 • : bscotti -
Nigel Farage's New Year Message.
Politicians & People • 16 • : LuciusDriftwood -
Outgoing Lame Duck BIDEN Officials and Democrats Voice Their Regrets.
2024 Elections • 29 • : Dalamax -
US disburses $3.4 billion in budget aid for Ukraine, Yellen says
US Political Madness • 17 • : bluesman023 -
2 Guys die hunting Bigfoot
Cryptozoology • 26 • : 2025yuga
