It looks like you're using an Ad Blocker.
Please white-list or disable AboveTopSecret.com in your ad-blocking tool.
Thank you.
Some features of ATS will be disabled while you continue to use an ad-blocker.
share:
before i talk, please allow me to show off the results of my research into nonlinear chaos dynamics.....
01-
02-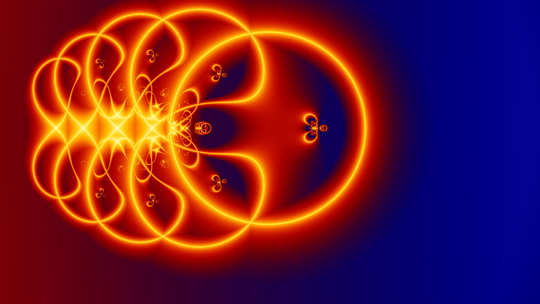
03-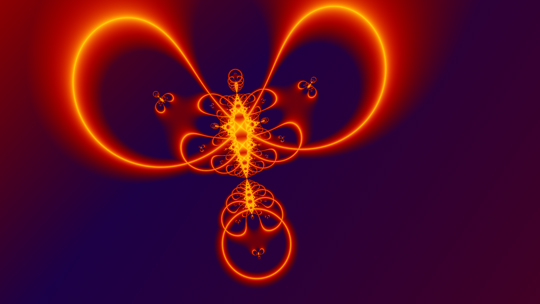
04-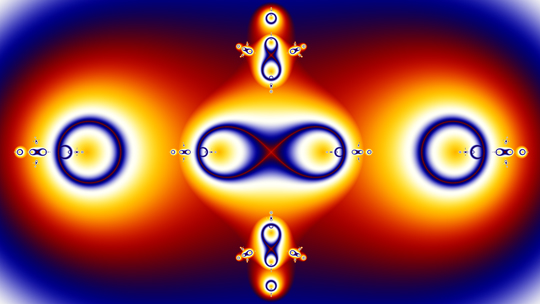
05-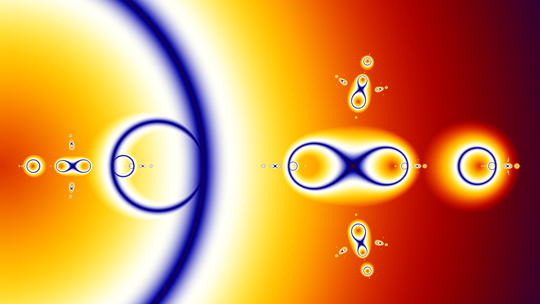
06-
07-
08-
09-
10-
11-
12-
13-
14-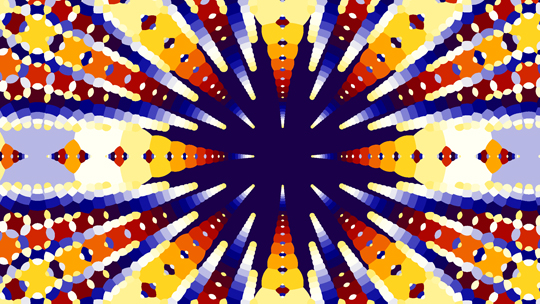
15-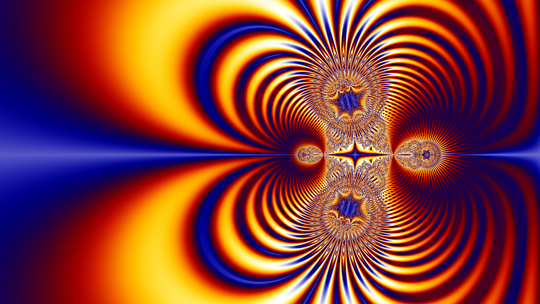
16-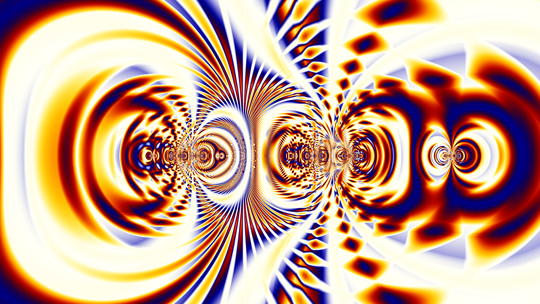
download uncompressed images dl.dropbox.com...
all of these fractal images are based on a chaotic attractor that i have been cooking up for the past four weeks. this is part of the work that i am doing for my bioinformatics thesis in which i am attempting to establish a basis for nonlinear chaos dynamics as the driving force of self-organizing biomolecular systems.
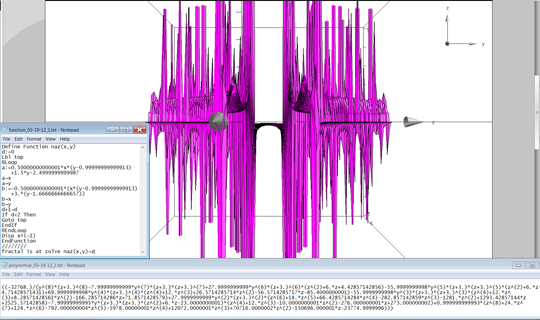
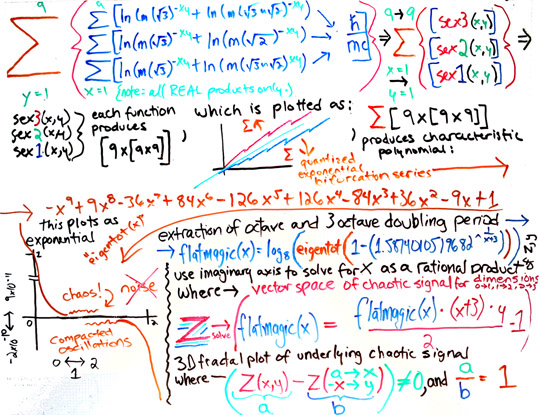
this is the iterating function which is used to generate all of the fractals:
01-

02-

03-

04-

05-

06-

07-

08-

09-

10-

11-

12-

13-

14-

15-

16-

download uncompressed images dl.dropbox.com...
all of these fractal images are based on a chaotic attractor that i have been cooking up for the past four weeks. this is part of the work that i am doing for my bioinformatics thesis in which i am attempting to establish a basis for nonlinear chaos dynamics as the driving force of self-organizing biomolecular systems.
this is the iterating function which is used to generate all of the fractals:
Define Function naz(x,y)
d:=0
Label top
©Loop
a:=0.50000000000001*x*(y-0.99999999999913)+1.5*y-2.4999999999987
a=:x
a=:y
b:=-0.50000000000001*(x*(y-0.99999999999913)+3.*(y-1.6666666666657))
b=:x
b=:y
d+1=:d
If d
so, the reason that i am writing this thread is that i am a bit stuck at the moment.
i am not a mathematician, nor a physicist (just a lowly biologist, ha!).....the math that i have been slogging away at for weeks and weeks has been a process of 1-part inspiration and 2-parts "letting the numbers do the talking".
now that i have gotten this far, i have satisfied myself that i have accomplished my inital intentions for the project which was simply to learn how to represent and design a chaotic system.
but i have NOT satisfied myself that i may be able to use the number set which i have thus generated in order to make inferences about the specific nature of biological chaotic systems.....
....that is where YOU come in!
i have given several of the intermediate graphic plots below. ALL of my graphic plots and ALL solutions for my math process (from inital design through iterative function) are contained in the two zip files attatched below.
please, if you are interested and capable, look through everything and if you see anything that you recognize as having a specific application in any scientific field, do let me know. it is a bit of a mess, tho. so....sorry.
thanks!!!
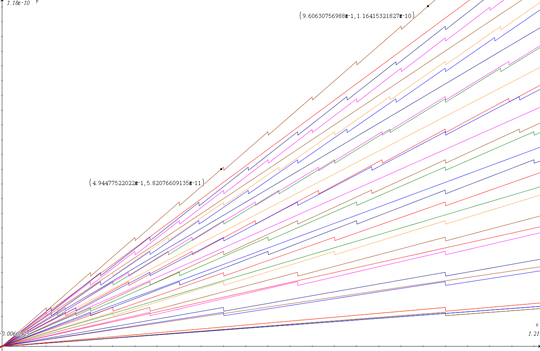
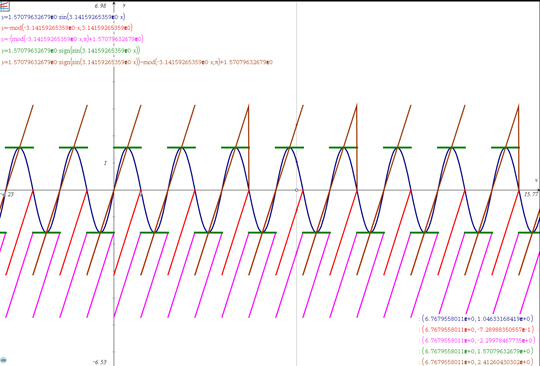
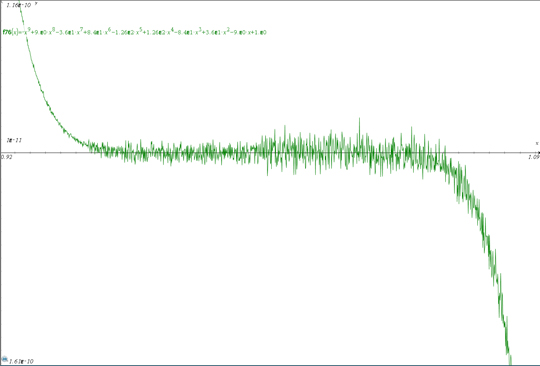
download function plots dl.dropbox.com...
download solutions dl.dropbox.com...
i am not a mathematician, nor a physicist (just a lowly biologist, ha!).....the math that i have been slogging away at for weeks and weeks has been a process of 1-part inspiration and 2-parts "letting the numbers do the talking".
now that i have gotten this far, i have satisfied myself that i have accomplished my inital intentions for the project which was simply to learn how to represent and design a chaotic system.
but i have NOT satisfied myself that i may be able to use the number set which i have thus generated in order to make inferences about the specific nature of biological chaotic systems.....
....that is where YOU come in!
i have given several of the intermediate graphic plots below. ALL of my graphic plots and ALL solutions for my math process (from inital design through iterative function) are contained in the two zip files attatched below.
please, if you are interested and capable, look through everything and if you see anything that you recognize as having a specific application in any scientific field, do let me know. it is a bit of a mess, tho. so....sorry.
thanks!!!





download function plots dl.dropbox.com...
download solutions dl.dropbox.com...
Wow...
Math and I have never really gotten along, so I can't help ya there....but those are some really cool looking images!
Math and I have never really gotten along, so I can't help ya there....but those are some really cool looking images!
to get us started on the right track, here is a quick summary of the iterating functions (same as given in the OP):

function 'a' and function 'b' are inverse sets, as shown by their ratio.
however, the difference between the two functions does NOT equal zero.
this goes against everything i have been taught about math! how can this be reconciled?!
the fractal is iterated by nesting one function as coordinates of the other.
thanks for reading and FEEDBACK!

function 'a' and function 'b' are inverse sets, as shown by their ratio.
however, the difference between the two functions does NOT equal zero.
this goes against everything i have been taught about math! how can this be reconciled?!
the fractal is iterated by nesting one function as coordinates of the other.
thanks for reading and FEEDBACK!
I know nothing about this kinda stuff but all your fractal images sure are pretty
S&F
S&F
reply to post by sugarcookie1
hey thanks! its always nice to receive praise. I think they are awesome as well.
if I can figure out how to get the function to iterate to infinity (without hanging my computer), I am sure that the product will be outstanding.
!!!HEY--> all of you non-math heads...if you get any "impressions" from the images...or maybe it reminds you of something.....that is a valid analytical tool, IMO.
hey thanks! its always nice to receive praise. I think they are awesome as well.
if I can figure out how to get the function to iterate to infinity (without hanging my computer), I am sure that the product will be outstanding.
!!!HEY--> all of you non-math heads...if you get any "impressions" from the images...or maybe it reminds you of something.....that is a valid analytical tool, IMO.
reply to post by boncho
there is an obvious "interactivity" between the elements within each fractal, but I had not really considered it as it is depicted in the video.
that is exactly the type of feedback I need. thank you!
there is an obvious "interactivity" between the elements within each fractal, but I had not really considered it as it is depicted in the video.
that is exactly the type of feedback I need. thank you!
reply to post by tgidkp
I am probably the worst math-head on ATS. but as for what I see in the images:
Unfortunately, I have been up way too long so maybe I'm reading too much into the pictures and they are nothing more than very pretty fractals.
!!!HEY--> all of you non-math heads...if you get any "impressions" from the images...or maybe it reminds you of something.....that is a valid analytical tool, IMO.
I am probably the worst math-head on ATS. but as for what I see in the images:
- Picture 3 looks like a womb
- Picture 5 looks like an solar system
- Picture 6 reminds me of the "Big Bang"
- Picture 8 looks like a super solar flare and in the middle X marks the spot
Unfortunately, I have been up way too long so maybe I'm reading too much into the pictures and they are nothing more than very pretty fractals.
Math, we meet again...
(we never got on well) Here's some 'interpretation of the renders, initial thoughts:
1. -
2. Trilobite
3. Flying insect, eg gnat, moth, butterfly, etc.
4 and 5. cellular bioogy.
6, 7, 8 and 9. ?magnetism?
10 and 11. ?The Sun? notice in eleven, the large straight bands, and closer 'around' the centre the crescent shapes kinda remind me of the arcs and loops on the suns surface.
12 and 13. kinda make me think of molecular computing (or whatever the term is; bio-computing?)
14. -
15 and 16. ? I dunno, also kinda reminds me of magnetism or vibrations/energetic emanations.
Are you using Fractal Explorer? It looks familiar. That programs a lot of fun, even though I suck at math. It makes pretty pictures.. good backrounds.
All the best with your thesis.
Bring out the Math Guru's!!
ps. why not Number the pics for reference through the thread?
(we never got on well) Here's some 'interpretation of the renders, initial thoughts:
1. -
2. Trilobite
3. Flying insect, eg gnat, moth, butterfly, etc.
4 and 5. cellular bioogy.
6, 7, 8 and 9. ?magnetism?
10 and 11. ?The Sun? notice in eleven, the large straight bands, and closer 'around' the centre the crescent shapes kinda remind me of the arcs and loops on the suns surface.
12 and 13. kinda make me think of molecular computing (or whatever the term is; bio-computing?)
14. -
15 and 16. ? I dunno, also kinda reminds me of magnetism or vibrations/energetic emanations.
Are you using Fractal Explorer? It looks familiar. That programs a lot of fun, even though I suck at math. It makes pretty pictures.. good backrounds.
All the best with your thesis.
Bring out the Math Guru's!!
ps. why not Number the pics for reference through the thread?
edit on 2012/3/20 by Jimjolnir because: ^^
reply to post by Jimjolnir
thanks to the both of you.
the images were rendered using 'ultra fractal'. all of the calcs were done on my TInspire.
I am not really all that great at math, myself.
thanks to the both of you.
the images were rendered using 'ultra fractal'. all of the calcs were done on my TInspire.
I am not really all that great at math, myself.
I wonder if the chaotic system of wave interaction in the distribution of oil film on the surface of water has anythign to do with this.
really pretty though.... and infinity is really big... so i would assume your computer would crash.
really pretty though.... and infinity is really big... so i would assume your computer would crash.
Originally posted by tgidkp
reply to post by sugarcookie1
if I can figure out how to get the function to iterate to infinity (without hanging my computer), I am sure that the product will be outstanding.
I've already mentioned my math skills aren't really up there with anything along the lines of what you're working on...but I do know a bit about code.
And I don't know how you would possibly get a representation of infinity in a calculation, since the only ways I could think of would cause an endless loop, thus hanging your computer. Then again....my skills are in cardiology and psychology...lol. Not even top of the class in coding so...lol.
Since I understand what you're trying to do now...before it was all Mandarin Chinese to me...not even greek...but now it gives me something to think about at least.
The first two remind me of the Elvish script that Tolkien created for The Lord of the Rings. The rest give me a headache, but then again, I am prone
to migraines and have been suffering from vertigo lately.
OK first why are you using such a crappy language to express your fractals? What is this some kind of calculator language? It almost looks like
assembly code. One thing I don't understand about your code is what is the meaning of the :x operator? When you do a=:x it looks like you are
overwriting a, assigned previously to a hyperbolic function of x and y to x. But apparently there are two assignment operators in your language of
choice, := and =: . Perhaps the second form means that x is assigned to a? Wow how horrific. What is this language? intercal?
Try matlab (or octave) or python or even C++/C for performance. I'd actually try this in python/numpy, actually even better would be to make use of a good symbolic processing language like maple or mathematica, though they have a horrific underlying programming language as well.
At any rate each iteration will double the degree of the two variable polynomial in x and y, each pass is a quartic polynomial in x and y. As such you can probably completely characterize the fixed points of the 4'th order function and probably get some bounds on the regions of convergence/divergence. But then that's what 'chaos' is all about.
As for an explanation of life, I find it lacking in complexity actually. Might be better as an explanation for randomness in physics or something.
Try matlab (or octave) or python or even C++/C for performance. I'd actually try this in python/numpy, actually even better would be to make use of a good symbolic processing language like maple or mathematica, though they have a horrific underlying programming language as well.
At any rate each iteration will double the degree of the two variable polynomial in x and y, each pass is a quartic polynomial in x and y. As such you can probably completely characterize the fixed points of the 4'th order function and probably get some bounds on the regions of convergence/divergence. But then that's what 'chaos' is all about.
As for an explanation of life, I find it lacking in complexity actually. Might be better as an explanation for randomness in physics or something.
Define Function naz(x,y)
d:=0
Label top
©Loop
a:=0.50000000000001*x*(y-0.99999999999913)+1.5*y-2.4999999999987
a=:x
a=:y
b:=-0.50000000000001*(x*(y-0.99999999999913)+3.*(y-1.6666666666657))
b=:x
b=:y
d+1=:d
If d
reply to post by webpirate
well, the software does give a "bailout" factor, after which the function is clipped short. so it will never actually go to infinity.
the problem is that i cannot get the iterating function, itself, to go beyond exactly 5 iterations (where one iteration is the first function into the second function; the second iteration is the results of the first plugged back into the first function......etc, etc.).
so the apparent infinite regression of all of these fractals is simply the effect of a single polynomial built from a single iteration of the original function.
youre right, though. it would probably require a quantum computer to properly render a nonlinear function into infinity without crashing itself.
well, the software does give a "bailout" factor, after which the function is clipped short. so it will never actually go to infinity.
the problem is that i cannot get the iterating function, itself, to go beyond exactly 5 iterations (where one iteration is the first function into the second function; the second iteration is the results of the first plugged back into the first function......etc, etc.).
so the apparent infinite regression of all of these fractals is simply the effect of a single polynomial built from a single iteration of the original function.
youre right, though. it would probably require a quantum computer to properly render a nonlinear function into infinity without crashing itself.
reply to post by XL5
for the largest polynomial that i can generate, at the fifth iteration, the number in the numerator is as follows:
-9.22337203684E18
it is my assumption that the number in the series which comes next is much larger than the system can handle.
but, you are on the right track, thats for sure.
for the largest polynomial that i can generate, at the fifth iteration, the number in the numerator is as follows:
-9.22337203684E18
it is my assumption that the number in the series which comes next is much larger than the system can handle.
but, you are on the right track, thats for sure.
YES! this.
let us add this to the list of things i am NOT! -----> programmer.
i am using the TInspire CAS calculator. whatever is the language for this is what i am using. i have enough things to worry about without teaching myself one of the languages you have suggested.
more about it here:
tibasicdev.wikidot.com...
good eye. the first is used to assign a value to a variable or function name. the second is used to update the value of a variable. so what you are seeing can be translated as:
1) parse function 1(x,y)
2) assign the result of this function to variable 'a'
3) update variables 'x' and 'y' with the value of 'a'
4) parse function 2(x,y) /this step uses the updtated x and y values.
5) assign the result of this function to variable 'b'
6) update variables 'x' and 'y' with the value of 'b'
7) parse function 1 (x,y) /with updated x and y values)
loop......
display the value of variable (x)^-1
yes, this is one of the options i have considered. i have also thought about calculating the average change from one iteration to the next and finding out if there is a constant in there somewhere which i can use to generalize the whole system.
thanks for your post!
Originally posted by SevenThunders
OK first why are you using such a crappy language to express your fractals?
let us add this to the list of things i am NOT! -----> programmer.
i am using the TInspire CAS calculator. whatever is the language for this is what i am using. i have enough things to worry about without teaching myself one of the languages you have suggested.
more about it here:
tibasicdev.wikidot.com...
there are two assignment operators in your language of choice, := and =:
good eye. the first is used to assign a value to a variable or function name. the second is used to update the value of a variable. so what you are seeing can be translated as:
1) parse function 1(x,y)
2) assign the result of this function to variable 'a'
3) update variables 'x' and 'y' with the value of 'a'
4) parse function 2(x,y) /this step uses the updtated x and y values.
5) assign the result of this function to variable 'b'
6) update variables 'x' and 'y' with the value of 'b'
7) parse function 1 (x,y) /with updated x and y values)
loop......
display the value of variable (x)^-1
you can probably completely characterize the fixed points of the 4'th order function and probably get some bounds on the regions of convergence/divergence.
yes, this is one of the options i have considered. i have also thought about calculating the average change from one iteration to the next and finding out if there is a constant in there somewhere which i can use to generalize the whole system.
thanks for your post!
Originally posted by SevenThunders
As for an explanation of life, I find it lacking in complexity actually. Might be better as an explanation for randomness in physics or something.
this is my suspicion also. but it leaves me wondering what, exactly, would be the characteristic features of a biological attractor? i dont suppose you have seen anything that fits that discription?
new topics
-
SCOTUS Chief Justice JOHN ROBERTS Ends 2024 Describing His Fears for Safety of U.S. Judges.
Above Politics: 53 minutes ago -
How we've changed in 100 years
Ancient & Lost Civilizations: 7 hours ago -
US disburses $3.4 billion in budget aid for Ukraine, Yellen says
US Political Madness: 8 hours ago -
Nigel Farage's New Year Message.
Politicians & People: 10 hours ago -
I dont understand what i just witnessed
Social Issues and Civil Unrest: 11 hours ago
top topics
-
I dont understand what i just witnessed
Social Issues and Civil Unrest: 11 hours ago, 11 flags -
US disburses $3.4 billion in budget aid for Ukraine, Yellen says
US Political Madness: 8 hours ago, 11 flags -
Happy New Year from Aussie
General Chit Chat: 14 hours ago, 8 flags -
How we've changed in 100 years
Ancient & Lost Civilizations: 7 hours ago, 8 flags -
Nigel Farage's New Year Message.
Politicians & People: 10 hours ago, 6 flags -
House republicans prove to be drama queens
US Political Madness: 15 hours ago, 5 flags -
The Silent Motion…
Short Stories: 14 hours ago, 5 flags -
SCOTUS Chief Justice JOHN ROBERTS Ends 2024 Describing His Fears for Safety of U.S. Judges.
Above Politics: 53 minutes ago, 2 flags
active topics
-
The C.D.C. Says There Was NO INFLUENZA Worth Reporting for the 2020-2021 Flu Season.
Diseases and Pandemics • 55 • : KrustyKrab -
Happy New Year from Aussie
General Chit Chat • 20 • : bally001 -
SCOTUS Chief Justice JOHN ROBERTS Ends 2024 Describing His Fears for Safety of U.S. Judges.
Above Politics • 1 • : BernnieJGato -
How we've changed in 100 years
Ancient & Lost Civilizations • 11 • : JJproductions -
Simulation theory and have we reset before like a game?
Conspiracies in Religions • 35 • : FullHeathen -
Strange fog all over the northern hemisphere
General Conspiracies • 36 • : bscotti -
Nigel Farage's New Year Message.
Politicians & People • 16 • : LuciusDriftwood -
Outgoing Lame Duck BIDEN Officials and Democrats Voice Their Regrets.
2024 Elections • 29 • : Dalamax -
US disburses $3.4 billion in budget aid for Ukraine, Yellen says
US Political Madness • 17 • : bluesman023 -
2 Guys die hunting Bigfoot
Cryptozoology • 26 • : 2025yuga
