A Question if You are Bored

So I love math. Sadly to say, I have probably forgotten more than I ever knew...leave that for the metaphysics forum. But I am starting to read and study it again.

So I am going to spell out a problem I don't know how to solve. If you are bored, please show how you would express it and solve it.

For the record, I feel music is my soul, and math/music make up a universal language that is the basis of our existence...again, not for this forum.

So here goes:

There is a 4 lane road, it stretches indefinitely. Two lanes run north, 2 south. Each lane is 8 feet wide. Walking North is a pedestrian. He is on the west side of the road. He is traveling at a speed of 4 miles per hour, and wants to cross the road. However, there are packs of cars traveling in either direction. Each car is traveling 35 mph. Each pack of cars consists of 7 cars. The first car is in the lane by the center line, the next is in the outer lane, the next by the center lane, etc. Each car is separated by the space of 1 car length, which we assume to be 8 feet. That pack of cars is separated by the back of the last car to the front of first car in the next pack by 47 seconds. The opposite lane has the same packs of cars separated by 45 seconds traveling in the opposite direction. Where the pedestrian is, the first car of the southbound cars is next to him. The first car of the northbound packs will pass the pedestrian as the 6th southbound car passes the pedestrian.

1. How long will it be before the pedestrian can safely cross the street?

2. How far will he walk until he can cross?

3. If he were to keep crossing back and forth, would a consistent pattern emerge?


Yup. This is what goes on in my head, scary?

a reply to: theatreboy
Not certain, but my feeling is the 4mph gait of the pedestrian has a window to cross safely, but he'll have to run faster than 4 mph.

That, or accidentally throw the bag of groceries down causing the cars to stop to allow him to repack grocery bag, then cross as they all blare their horns at him.
🙏❤

a reply to: theatreboy

if the pedestrian pulls his glock and points it sideways at the cars, eventually, one will screech to a halt, and the driver will get out and run, leaving his car for the pedestrian. Carjacking 101 brought to you by GTA. You are welcome.

a reply to: theatreboy

I hate to ask this, but it might make a difference....
What race is the pedestrian?


Are they self-driving cars or are there people behind the wheel and in control?

a reply to: theatreboy

My plebian attempt:
1 mile = 5280 feet
35mph = 184800
4mph = 21120

The cars are Travelling 51 feet a second with a gap of 2309 feet between the groups.
The person has to travel 32 feet total for all four lanes. the person travels 5.86 feet a second so roughly five to six seconds to cross the highway.
Given the large time gap, I would guess the that the person has plenty of time to wait for both lanes to clear before crossing.

a reply to: theatreboy

I am so bad at math I get itchy even thinking about it! lol For example: your first two sentences caused me to see an image and have a question in my mind..."There is a 4 lane road, it stretches indefinitely. Two lanes run north, 2 south."...they have to meet at one point don't they? there would have to be an intersection right where one begins to go north and the other begins to go south, right?

and next..."The first car is in the lane by the center line, the next is in the outer lane, the next by the center lane, etc."...
is this occurring in both north and south lanes?

and next: "However, there are packs of cars traveling in either direction."...
how is this possible when 4 only go south and the other 4 only go north?


you get the idea! I think my brain is scarier than yours when it comes to math. I keep getting stuck on "why?" when it comes to solving math problems. who said it is this way? who made that up? how do they know? etc....







*addition: I thought of one more thing...maybe he could just build a small bridge and go OVER the roads so he can cross any time he wants!

I really like your post, but with all the crap going on in the world, I can’t expand my mathematical Limitations at this time.
But, I don’t think there would be a pattern developing because they are two different systems on the same infinite path.

Any chickens?
Yes I know a stupid response but really, in my opinion, reflects the question
No matter how bored I get, never would I even consider attempting an answer, not maths minded, sadly
What goes on in my head is far more simpler, think my posts attest to that

I tried to calculate t=? when the man (northbound @ 5.86 ft/s) and the 6th car (southbound @ 51.3 ft/s) intersect.

And I am reminded that I am rusty with vectors...

a reply to: theatreboy

Sorry, I can't figure it out. I can't even count to ten without getting confused... I think it's called dyscalculia. Even though I'm ok with numbers in mass, stats, graphs, etc... go figure!

Anyway, your problem reminded me of a meme...



Sorry for the detour.

a reply to: theatreboy

Easy-peasy : if he wants to go and get Encia22's chicken on the other side of the road : he must cross when there are no cars.
Unless he can jump.

Maybe he could build a bridge over the highway ?

Maybe there was already a bridge ?

Has anybody seen the bridge ?

Where's that confounded bridge ?

a reply to: theatreboy

Oh, this should be fun.

The trick is to get the units consistent. You have feet combined with miles per hour, and that simply will not do. To convert from miles per hour to feet per second, we need to multiply by 5280 (feet in a mile), then divide by 3600 (seconds in an hour). So we get the following (I am rounding to two digits):

4 mph = 5.87 fps
35 mph = 51.33 fps

Now we have everything in consistent units, let's look at the problem again:

At the start point (t0), we do not know where the northbound pack of cars is. We do know where the southbound pack of cars is: the first car is beside our pedestrian. That means the last car will be past him at some future time. Now, how long is this pack of cars? There are seven cars, each separated by a car length, so that's 7+6=13 car lengths. Each car length is 8 feet, so that's 104 feet long.

(I'm not going to mention that these are extremely short cars spaced way, way, way too close for safety!)

Now, at some future time, we do know where the northbound cars are. That happens when the sixth southbound car is directly beside the pedestrian; the first northbound car is exactly beside the pedestrian as well. When does this happen? Well, between the first and sixth southbound cars (center to center) is 10 car lengths (five cars and five spaces), so that's 80 feet. So our second time point happens when the cars have traveled 80 feet, right?

Wrong! Relativity matters here. The southbound cars are traveling at 51.33 fps, while the pedestrian is traveling at 5.87 fps in the opposite direction. We need the speed of the cars relative to the pedestrian. Opposite directions, so the speeds add. The second point in time occurs when the cars have traveled 80 feet at the relative speed to the pedestrian, which is 51.33 + 5.87 = 57.2 fps. 80 ÷ 57.2 = 1.4 s (seconds). So this second point in time is at t1 = 1.4 s. Since we will later want to know how far our pedestrian has traveled at this point, we can go ahead and calculate that he has traveled 8.22 feet (5.87 fps x 1.4 s) at t1 = 1.4 s.

Now we use a little logic: the southbound cars are almost past him, but the northbound cars are just getting to him. So he will probably be able to cross as soon as the northbound cars pass him. When will that happen?

We know the total length of the northbound cars is 104 feet. We also know that the first northbound car is beside him, so we deduct half a car length, or 4 feet, for a total of 100 feet. How long will it take the northbound cars to move 100 feet relative to the pedestrian? In this case, since both the cars and the pedestrian are traveling in the same direction, their speeds subtract. 51.33 - 5.87 = 45.46 fps. 100 ft ÷ 45.46 fps = 2.2 s.

So we now know the road will be clear at 2.2 s past our t1 point of 1.4 s, which is a total of t2 = 3.6 s.

But we're not done yet! The southbound lanes next to the pedestrian will be clear before the northbound lanes will clear. Our pedestrian can start crossing before that point, as long as he is only halfway across when the northbound lanes clear. So how long will it take him to cross two lanes of traffic? 16 ft ÷ 5.87 fps = 2.73 s. According to that, he can start crossing at 3.6 - 2.73 = 0.87 s.

The problem is that at 1.4 seconds, the first two lanes are not yet clear! So he can't start crossing at 0.87 s; he will have to wait until the first two lanes are clear before he begins to cross. Since we know now that the northbound lanes will be clear by the time he gets to them, we don't have to worry about them. When will the southbound lanes be clear?

We know that from the start point, the southbound cars will clear after they have traveled 100 ft relative to the pedestrian, which is at a relative speed of 57.2 fps. So 100 ft ÷ 57.2 fps = 1.75 s. The first lane will clear sooner, though, when the cars have moved 100 - 16 = 84 feet relative to the pedestrian. That is 84 ft ÷ 57.2 fps = 1.47 s.

To double check, the time our pedestrian will take to cross one lane is 8 ft ÷ 5.87 fps = 1.36 s. The last car will have covered the needed 16 feet to clear the inner lane in 16 ft ÷ 51.33 fps (the pedestrian is moving sideways so relative and actual speeds are the same) = 0.31 s. So the last car will have easily cleared the inner lane long before the pedestrian gets there.

So, to sum all this up:

• The pedestrian can start to cross as soon as the outer lane southbound is clear, at 1.47 s.
  
• He will reach the inner southbound lane at 1.47 + 1.36 = 2.83 seconds. That lane will have cleared at 1.75 s.
  
• He will reach the middle of the road at 1.47 + 1.36 + 1.36 = 4.19 s. The last car northbound will have easily cleared both northbound lanes by that time.

So the answer to number 1, at what point can the pedestrian safely cross, is beginning at 1.47 s.

Question 2: How far will he have walked before he can start to cross? 5.87 fps x 1.47 s = 8.63 ft.

Question 3: The answer is yes, there will be a pattern, and requires no actual calculation. The pedestrian's speed is cyclical; he walks, crosses, walks, crosses back, and repeats. The traffic is cyclical; the cars come in evenly spaced increments of identical length and speed. Therefore the result will be a cyclical pattern. The crossing back may be at a different relative time than the first crossing, as the traffic is mow moving in the opposite direction to the first crossing, but the timing will be identical for each direction of crossing.



I was right; that was fun!

TheRedneck

a reply to: TheRedneck

According to my elementary level math, would it be possible to just wait until the fifth pass to go across easily?
The fifth wave of both side would occur at 235 seconds on both side. 7x5 because 47 and 45?

a reply to: theatreboy

42

originally posted by: theatreboy
1. How long will it be before the pedestrian can safely cross the street?

2. How far will he walk until he can cross?

3. If he were to keep crossing back and forth, would a consistent pattern emerge?


Yup. This is what goes on in my head, scary?

1. 0 seconds. He can turn right away onto the lane. Until the 7th. southbound car passes, the traveler has not crossed the lane.

The each lane is 8 feet wide. The traveler walks 5.86feet per seconds.
For the two southbound lanes, he needs 2.73 seconds. It takes 2.28 seconds for the northbound pack to pass. So he is safe.

2. 0 feet. He can turn before his next foot reaches the ground. See above

3.
It takes him 5.46 seconds to cross the lanes. We add a second to turn around. 6.46 seconds to cross one side and turn.
6.46s passed from second zero.
Now, I do not have excel on this computer, I would give you the pattern and when it splashes the first time.

I still have tons of other parameters calculated, I love math too.

Here is the starting situation. I understood it that it's a country where the lanes follow western driving sides and that the spacing sentence was meant that each lane has 1 car distance, not spread over two.




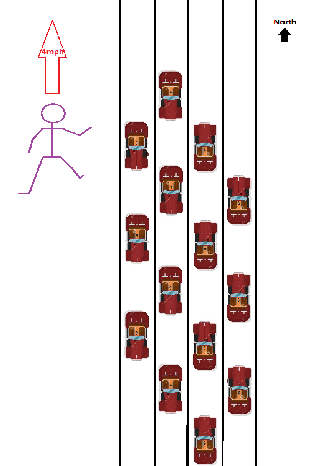
I am not bored but I need to keep it low, jabbed today.

a reply to: TheRedneck

Thank you. Makes total sense. I am looking forward to learning all this...again!

a reply to: ThatDamnDuckAgain

Oooh, bonus marks for the diagram (although it isn't quite accurate in terms of distance between cars and starting location of the NB pack).

By the way, you should know about google sheets! It's basically a free version of excel. You can program macros and everything.

sheets.new

originally posted by: theatreboy
So I love math.

For the record, I feel music is my soul, and math/music make up a universal language that is the basis of our existence...again, not for this forum.

I couldn't agree more with this.

Cars are unsafe heavy metals driven mainly by idiots. There is no safe place for the pedestrian.
Even on the sidewalk aka pavement.

BTW a van driver failed to stop at a pedestrian crossing on red light today. I could have been a woman pushing a pram. The baby could now be dead. Almost I got his licence. later.

originally posted by: Nothin
Has anybody seen the bridge ?

Where's that confounded bridge ?

I ain't seen the bridge!