Pi Equals Four When Motion Is Involved

originally posted by: Op3nM1nd3d
a reply to: ErosA433

Why? Well the ball is always applying a downward force on the tube, this point of contact will give it constant loss.

So you are saying that the ball on the straight tube does not apply downward force? Did you even watch the video? The reasoning in this case is just plain simple. Besides, in a perfect circle-ball fit tube, there won`t be any more friction than on a straight tube if both are traveling on a two dimesional space(x,y), are of the same size and weight and have the same velocity.

I can`t help you if you can`t see it.

Incorrect, and likewise i cannot help you, if you cannot look at the experiment and look at the forces and fully what is happening... your answer is not adequate and also incorrect. You seem to be ignorant of the concept of a banked turn and the forces and energy transfers involved.

a reply to: ConnectDots

Hi Connect,

Actually pi can't be determined.

If you take one of the most intriguing and beautiful formulas, Euler’s Identity,

e^(pi*i) = -1

and you substitute -1 by i^2

e^(pi*i) = i^2

then take the natural logarithm of each side

ln[e^(pi*i)] = ln[i^2]

take out the power

pi*i*ln(e) = 2*ln(i)

and ln(e) = 1

pi = 2*ln(i)/i

Now, if you consider the function y = 2*ln(x)/x (you can plot this in google if you want)

You can see that that function reaches a maximum in (x;y) = (e; 0.73575888234)

So there is no value for x that returns a value higher than 0.73575888234 for y, meaning there is no x that returns a y = pi (=3.14…); and so pi can't be determined.

Cheers!

which by the way the romans invented, with the help of some syracuse clock makers.

a reply to: Agnost

Look up complex logarithm.

ln(i) = i * pi/2

pi = 2*ln(i)/i == pi

originally posted by: Op3nM1nd3d
a reply to: Arbitrageur
No it`s not, unwind the curved tube and you`ll get the same distance. Every quarter is marked. Seems like you have fallen for the optical illusion yourself.

I don't have the tube to unwind, but I do have the photograph I posted and I measured the right half of the circle using a bent flexible plastic ruler to follow the contour of the circle, then I let the ruler snap back to its straight shape and measured the corresponding straight section, which confirmed I'm not suffering from any illusion and that you are wrong. The straight tube is definitely longer. Try using a measurement method similar to mine yourself, bend a sheet of paper around the curve if you don't have a flexible plastic ruler and mark the length of the right half of the circle, then compare that piece of paper to the straight section of tubing. The top of the circle does not match up with the second marking on the straight tubing when compared in this way.

originally posted by: Agnost
a reply to: ConnectDots

Hi Connect,

Actually pi can't be determined.

If you take one of the most intriguing and beautiful formulas, Euler’s Identity,

e^(pi*i) = -1

I don't know which is worse, your logic or your math but they are both dismal.

Euler told us how to calculate Pi to arbitrary precision using either of these formulae:

Infinite Expressions for Pi



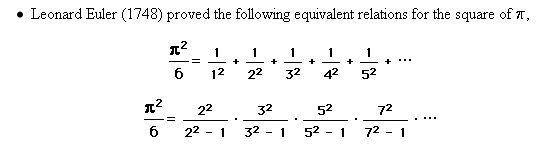
Of course expressing the exact value of Pi with an infinite number of digits isn't possible in a finite amount of time, but here's Pi determined to 5 trillion digits, far more than anybody really needs:

5 Trillion Digits of Pi - New World Record

There are also far more ways of determining Pi to arbitrary precision (such as 5 trillion digits if you want that many) than the ones provided by Euler:

Pi Algorithms

Another relevant Mathis paper is "Proof from NASA that π is 4":

Those who have found my paper on π to be shocking will find this one even more shocking. Here I will show that NASA’s own rockets have provided simple proof of my assertion concerning π, and have been providing it since 1958, the year of the first successful orbit. . . .

milesmathis.com...

originally posted by: ConnectDots
Another relevant Mathis paper is "Proof from NASA that π is 4":

Those who have found my paper on π to be shocking will find this one even more shocking. Here I will show that NASA’s own rockets have provided simple proof of my assertion concerning π, and have been providing it since 1958, the year of the first successful orbit. . . .

milesmathis.com...

Mary, do you even understand what pi is? Don't quote this guy. You tell me.

originally posted by: ConnectDots
Another relevant Mathis paper is "Proof from NASA that π is 4":

Those who have found my paper on π to be shocking will find this one even more shocking. Here I will show that NASA’s own rockets have provided simple proof of my assertion concerning π, and have been providing it since 1958, the year of the first successful orbit. . . .

milesmathis.com...

So he proves that pi=4 by ignoring what pi is. That takes a special kind of moron.

It's like saying "hey guys! I've just discovered that an apple is now an orange because I said so!"

The moron hasn't proven a thing and, yet again, you fall for the same BS as always. I kind of feel sorry for you, but then remember that you're promoting this rubbish.

originally posted by: TerryDon79

The moron hasn't proven a thing and, yet again, you fall for the same BS as always. I kind of feel sorry for you...

It's what you get when your science education stops at grade school, and you weren't really paying attention then, either.

You get people spraying vinegar in the backyard, or asking why their sprinklers are making rainbows, or the camcorder sees "ripply waves" in fine details like a window screen. It all seems reasonable, if you've got no starting point at all, I'd suppose.

a reply to: Bedlam

And why a guy thinks he's debunked gravity by jumping up....and down...

www.abovetopsecret.com...

Oh and I've sprayed vinegar in the backyard before, it's a great, mild cleaner.

originally posted by: Bedlam

originally posted by: TerryDon79

The moron hasn't proven a thing and, yet again, you fall for the same BS as always. I kind of feel sorry for you...

It's what you get when your science education stops at grade school, and you weren't really paying attention then, either.

It makes me wonder if some of these people ever went to school in the first place.

If Miles knew what pi was, he wouldn't be trying to change the meaning of it, regardless of its value.

As for the OP? They just fall for everything "edgy" because YouTube and a website tells them to. It's kind of sad.

originally posted by: TerryDon79

As for the OP? They just fall for everything "edgy" because YouTube and a website tells them to. It's kind of sad.

I think some of that can be phrased as "I don't understand it, therefore it has to be dodgy, and thus I shall prove that by presenting these posts that prove the boffins are all wrong. Thus I will not feel like a fool"

originally posted by: Bedlam

originally posted by: TerryDon79

As for the OP? They just fall for everything "edgy" because YouTube and a website tells them to. It's kind of sad.

I think some of that can be phrased as "I don't understand it, therefore it has to be dodgy, and thus I shall prove that by presenting these posts that prove the boffins are all wrong. Thus I will not feel like a fool"

Most definitely.

It's painfully obvious as the OP hasn't even explained it in their own words. They've avoided everything on this thread and just posted more from the morons website. That's normally a good indicator of "I don't know, but it looks good to my layman eyes".

a reply to: TerryDon79

It's only appealing because it's anti established science.

In the OP's mind, this is all that is needed to give something credibility.

Some one build a circular track for cars. The length of the track is a certain length because of PI. As the cars drive faster on the track, PI gets larger due to this theory. So the track must expand to account for the value of PI getting larger.

Explains NASCAR and possibly the crumbling infrastructure of the country.

a reply to: Chadwickus

There's anti established science and then there's pure stupidity.

Trying to redefine what pi is and what it's used for is pure stupidity.

a reply to: roadgravel

Are you talking about the same thing as Mathis is talking about starting here, and including a link to a 43 page PDF:

. . . No doubt many will answer me, “The surveyor's wheel doesn't fail, since what a surveyor is interested in is a simple length, not some mystical kinematic distance like you are inventing here.” But that is false as well. Let us say a surveyor is measuring a running track for the Olympics. Well, he has to measure both the straight legs of the track and the curves. And what he wants to know is how far the runners have run, right? Well, running is kinematic. It is like a little orbit. It requires real bodies to move through the curves. It is not just curves sitting on the ground, it is curves being run in real time. Therefore, to calculate the correct distances through the curves, the surveyor must integrate all the motions involved. Treating the curves as equivalent to the straights will fail to do that. And yes, I am telling you the inside lane of the standard track is longer than 400 meters. Or, the runners are running considerably farther than 400 meters. This should be easy to prove by timing groups of top athletes through straights and curves. I predict it will be found that the athletes appear to move through the curves much slower than can be accounted for by stress on the inside leg, etc. But if you use pi=4 to measure the length of the curve, this discrepancy will vanish.

In fact, I find that some tests have been run, confirming this. At this link to Brigham Young University [p. 25], we find that “Depending upon the track, athletes may spend up to 60% of the race on the turn (P. R. Greene & Monheit, 1990).” Holy Cow! 60%? And no one ever thought that was strange? Using pi=4, we would predict 56% of the time to be spent in the curves [4/7.14]. The other 4% would then be given to tighter curves requiring more leg adjustments. Using running dynamics, you would never predict a slowdown that great [60/40] in the turns. That's a slowdown of 33%. But if we give most of that slowdown to a mismeasurement of the curve using pi [a “slowdown” of 21%], it makes more sense.]

milesmathis.com...

a reply to: ConnectDots

Pwahahahahahahahahahahaha

I've never read so much rubbish in such a short quote.

"They're running longer than X because I changed the value of something to make it longer."

Do you seriously believe this? I mean, really. Anyone with a pair of eyes can see it's completely bull and hilariously wrong.

If the faster runner is running a longer distance because Pi changes then the timers should shave time off their elapsed time. Only fair.

So if a circle was made out of a rail and there is an object that rides upon it. Monorail. When the object moves and PI increase then the object must travel more distance as it navigates the circle. How does it do it since it can't move lateral to add extra distance to it path. The circumference at rest and in motion are different.

I need to learn this new math...

a reply to: roadgravel

In Mathis' biography there is an anecdote about him bringing to the attention of authorities for the PSAT test that there was an error he discovered that needed correcting:

. . . He should be remembered by the takers of the PSAT, 1980, for questioning one of the answers on the math portion. The PSAT admitted its error and was forced to change all scores nationally. Miles' score: 68/78:214.

mileswmathis.com...

What he has done is to start from scratch and corrected errors where he has found them. He has numerous papers posted on the page "The Greatest Standing Errors in Physics and Mathematics."