Pi Equals Four When Motion Is Involved

a reply to: ConnectDots

Are you going to address the many issues with this or are you going to pretend they don't exist?

Just like your other threads, you try pushing something yet don't even understand what is being said.

So, show us how pi magically changes into 4. In your own words. I won't hold my breathe because I know you can't.

originally posted by: TerryDon79
a reply to: ConnectDots

Are you going to address the many issues with this or are you going to pretend they don't exist?

Just like your other threads, you try pushing something yet don't even understand what is being said.

So, show us how pi magically changes into 4. In your own words. I won't hold my breathe because I know you can't.

Wow - LMAO!

Don't hold back my friend. Tell us what you really think.

And BTW I agree with you 100%.

#ing nonsense from top to bottom....

originally posted by: TerryDon79
I won't hold my breathe because I know you can't.

I'd be willing to bet she doesn't actually know what pi is, in terms of a circle.

a reply to: Riffrafter

I'm sorry, but I'm fed up with idiots on the internet trying to pass of some junk as science. All they do is make it seem "sciency" by using a whole mess of words, but when it comes down to it it means nothing.

I'm all for expanding on what we know, but to try and rewrite pi by ignoring what pi is like calling a square a triangle to be "edgy".

a reply to: Bedlam

this is because of trump.

i heard a trump conversation where he said he was going to grab the pi.

clearly trump has no respect for pi.

originally posted by: interupt42
...he was going to grab the pi.

Carpe crustulorem!

originally posted by: TerryDon79
a reply to: Riffrafter

I'm sorry, but I'm fed up with idiots on the internet trying to pass of some junk as science. All they do is make it seem "sciency" by using a whole mess of words, but when it comes down to it it means nothing.

I'm all for expanding on what we know, but to try and rewrite pi by ignoring what pi is like calling a square a triangle to be "edgy".

No apologies necessary my friend.

You spoke the truth.

Very rare commodity around these parts.

But I always honor it when I see it.

originally posted by: interupt42
a reply to: Bedlam

this is because of trump.

i heard a trump conversation where he said he was going to grab the pi.

clearly trump has no respect for pi.

LMAO!

Please STFU - you're killing me.

originally posted by: Bedlam

originally posted by: interupt42
...he was going to grab the pi.

Carpe crustulorem!

LOL!

We're definitely going to seize something.

But what that may be - I am in the dark as much as you folks.

Thoughts?

And in the interim...

Hail Eris! Hail Discordia!

Robert Anton Wilson rules....

a reply to: Soylent Green Is People

I'm sure that through experimentation, he can find a ratio between the paths the two balls take -- the straight one and the one that rides up on the walls of the curved tubed). HOWEVER, that ratio should be called something other than "Pi", since it is practically has no association to Pi.

Perhaps this will help.

Objects moving in circles have a speed which is equal to the distance traveled per time of travel. The distance around a circle is equivalent to a circumference and calculated as 2•pi•R where R is the radius. The time for one revolution around the circle is referred to as the period and denoted by the symbol T. Thus the average speed of an object in circular motion is given by the expression 2•pi•R / T.

I agree that the logic is wrong. Pi itself is not the actual distance as proposed in the OP. It is 2πr, every kid knows that, lol.

originally posted by: Op3nM1nd3d
a reply to: Soylent Green Is People

I'm sure that through experimentation, he can find a ratio between the paths the two balls take -- the straight one and the one that rides up on the walls of the curved tubed). HOWEVER, that ratio should be called something other than "Pi", since it is practically has no association to Pi.

Perhaps this will help.


"Objects moving in circles have a speed which is equal to the distance traveled per time of travel. The distance around a circle is equivalent to a circumference and calculated as 2•pi•R where R is the radius. The time for one revolution around the circle is referred to as the period and denoted by the symbol T. Thus the average speed of an object in circular motion is given by the expression 2•pi•R / T."


I agree that the logic is wrong. Pi itself is not the actual distance as proposed in the OP. It is 2πr, every kid knows that, lol.

That's why I said "practically no association to Pi".

I realize that Pi could be used to help describe the curved path, so that's a tangential association between Pi and this experiment's set-up.

However, the point I was making was that the affect of the ball taking longer through the curved path was not due to the geometric description of the curve (a curve that could be described/defined with the help of Pi), but actually due to the momentum lost by the ball pushing on the outside walls of the curved tube, thus imparting a force to the wall of the curved tube -- a force that needed to taken from somewhere, and that somewhere from which that force was taken was the ball's momentum, thus reducing that momentum.

So while there is a measurable effect that could be calculated due to that loss of momentum (a loss of momentum that would be greater than in the case of the ball rolling along the straight tube), and there maybe even be a "mathematical constant" that could be created to help calculate this loss of momentum, that mathematical constant should not be called "Pi", since it has little to do with what Pi actually is.

a reply to: Soylent Green Is People

I said I agree

The problem with your proposition is that if the curved tube is of the right proportions to just fit the ball and there is no space in between, there can be no fraction or loss of momentum under the right circumstances (if there is no z). So you would have to have more than one constant, depends on the variation of the tube compared to the ball. That`s why T, the revolution period, is the most practical solution. You just measure the time and that`s it.

The problem with the OP is that he just assumes that Pi is the distance even when you flat out the circle to fit the straight tube. Both are of the same length. It doesn`t have anything to do with friction. It`s the concept that is wrong in the first place.

π ≠ 2πr. It`s that simple to explain this case

So yeah, it does not have any association with Pi other than that the ball moves around a circle.

a reply to: Op3nM1nd3d
It does have quite a bit to do with friction

Why? Well the ball is always applying a downward force on the tube, this point of contact will give it constant loss. Always gravity is trying to make the ball accelerate in the downward direction, this point of contact generates some energy loss through friction.

Now when the ball travels around the tube, its point of contact is not the same, it has a frictional force that is causing it to change direction along with gravity. The energy loss between the ball and the wall is higher when going around in a circle than it is on a straight line.

You do not need a tube to prove this, there are more forces acting.

a reply to: ErosA433

Why? Well the ball is always applying a downward force on the tube, this point of contact will give it constant loss.

So you are saying that the ball on the straight tube does not apply downward force? Did you even watch the video? The reasoning in this case is just plain simple. Besides, in a perfect circle-ball fit tube, there won`t be any more friction than on a straight tube if both are traveling on a two dimesional space(x,y), are of the same size and weight and have the same velocity.

I can`t help you if you can`t see it.

Regarding friction:

I predict the main response to the video will be that the ball in the curve is feeling more friction. However, it is clear at a glance this is not the case. To start with, the ball in the curve would have to be feeling over 20% more friction than the straight ball. Again, the difference between 3.14 and 4 is not marginal. It is huge. There is no way to account for a difference that large with a difference in friction. Plus, if friction were the cause, the ball in the curve should be slowing down as it progresses around the curve. Friction is of course cumulative, so we would expect a ball feeling an excess 21% of friction to be going slower in the fourth quadrant of the circle than in the first. But we see with our own eyes that isn't true. Steven marks all four quarter points in the circle, and the ball hits them all perfectly in sync with the straight ball. If the ball in the curve were feeling more friction, we would expect it to hit the ¾ mark and final mark noticeably late compared to the ¼ mark. It doesn't. This indicates very strongly that neither friction nor any other cumulative effect in the curve is causing the difference. The ball in the curve is NOT slowing relative to the straight ball. This should look as curious to you as pi being 4. Given current theory, it is just as mysterious.

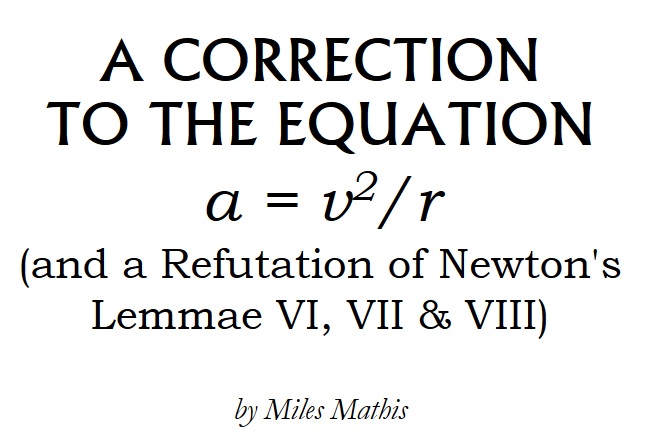
milesmathis.com...

a reply to: ConnectDots

That's nice.

Question.

How does that have anything to do with the ratio of a circles circumference to its diameter?

I think the guy is getting confused about friction, velocity and distance travelled and pi itself.

ETA: I've got this strange feeling he's using an oval or ellipses to try and prove pi wrong.

Here is how Mathis got started working on this issue:

I was working on Newton's orbital equations , which I saw as having some disclarities. To say it another way, they didn't make sense to me. I thought his proofs contained some big holes, and the more recent updates of these proofs by famous guys like Richard Feynman seemed to me no better. In fact, I showed they were worse.

Since an orbit is just motion in a circle, I think you can see the link. So I started over from the beginning, rerunning Newton's equations with a few corrections. These corrections solved many Since an orbit is just motion in a circle, I think you can see the link. So I started over from the beginning, rerunning Newton's equations with a few corrections. These corrections solved many problems, but not all of them. It wasn't until a couple of years later that I thought to look at pi itself. . .

milesmathis.com...

The paper on Newton's orbital equations:

milesmathis.com...

a reply to: ConnectDots

See my post above.

I see where his rediculous claim comes from. He's trying to change pi from being about a circle to being about ovals or ellipses.

He's literally trying to change what pi is used for lol.

originally posted by: Op3nM1nd3d
a reply to: Soylent Green Is People

I said I agree

The problem with your proposition is that if the curved tube is of the right proportions to just fit the ball and there is no space in between, there can be no fraction or loss of momentum under the right circumstances (if there is no z). So you would have to have more than one constant, depends on the variation of the tube compared to the ball. That`s why T, the revolution period, is the most practical solution. You just measure the time and that`s it.

The problem with the OP is that he just assumes that Pi is the distance even when you flat out the circle to fit the straight tube. Both are of the same length. It doesn`t have anything to do with friction. It`s the concept that is wrong in the first place.

π ≠ 2πr. It`s that simple to explain this case

So yeah, it does not have any association with Pi other than that the ball moves around a circle.

Under perfect hypothetical conditions where momentum cannot be lost, a ball rolling through a tube that is 3.14159... units long will take the same amount of time "T" to travel that distance, no matter if the tube is straight, curved, wavy, or a perfect circle.

The problem with this experiment was the set up did NOT have perfect conditions, and in fact momentum was lost in the curved tube.

That momentum was lost because the ball's inertia through the curve would push with a force outward on the tube which would be greater than the force of gravity acting downward on the ball in the straight tube. That's because inertia would be wanting the ball in the curved tube to go straight, but instead the ball is being forced into a curved path. Just like a driver of a car going around a curve in the road feels a force pushing outward from the direction of the curved road, the ball pushes outward from the direction of the curved tube (and transferred into the sidewall of the tube). This would still be true even if the tube was just the right size to fit the ball. There would still be an additional force pushing outwards in the curved tube that, along with the gravity of the ball pushing downward on the tube, creates a net greater force than just the gravity alone of the ball in the straight tube pushing downward on the tube.

This additional force acting on, and being transferred into, the side of the curved tube would need to be taken away from the overall energy of the rolling ball, thus slowing that ball down.

The fact that the experimenter is using the empirical results of this poorly set-up rig in an attempt to prove his hypothesis is the problem. If he could magically take away all friction and the transfer of the balls' energy to the tubes, then the experiment would show that the two balls would take the same amount of time to travel 3.14159(and so on) units -- or any distance, for that matter.

a reply to: Soylent Green Is People

No, I think that he did a pretty good job showing that the ball doesn`t lose much velocity while traveling in a circle. Not perfect conditions, I agree, but his observations are completely wrong. The balls did travel the same amount of let`s say decimeters. Both tubes are 40 dm long, one straight, one forming a circle. So he proved that both balls, despire traveling in a different pattern, had reached the end distance which is at 40 dm in the same amount of time. But that`s about it. What he proposes is just stupid, out of proportions kinda stupid.

If both tubes were 31.4 dm long (for example), under the same conditions, it would show that they both passed the finish line at the PI ratio so to speak (which of course doesn`t have anything to do with Pi, it only shows the numbers that resemble Pi), but he was clearly trying to reinvent math, or deceive people. I mean this is basic math and logic. I don`t know how else to explain

I thought it was obvious enough.