It looks like you're using an Ad Blocker.
Please white-list or disable AboveTopSecret.com in your ad-blocking tool.
Thank you.
Some features of ATS will be disabled while you continue to use an ad-blocker.
share:
a reply to: Hyperboles
From here en.wikipedia.org...
Prograde satellites of Uranus orbit in the direction Uranus rotates, which is retrograde to the Sun.
All eight planets in the Solar System orbit the Sun in the direction of the Sun's rotation, which is counterclockwise when viewed from above the Sun's north pole. Six of the planets also rotate about their axis in this same direction. The exceptions – the planets with retrograde rotation – are Venus and Uranus. Venus's axial tilt is 177°, which means it is rotating almost exactly in the opposite direction to its orbit. Uranus has an axial tilt of 97.77°, so its axis of rotation is approximately parallel with the plane of the Solar System. The reason for Uranus's unusual axial tilt is not known with certainty, but the usual speculation is that during the formation of the Solar System, an Earth-sized protoplanet collided with Uranus, causing the skewed orientation.
From here en.wikipedia.org...
edit on 24-3-2019 by blackcrowe because: (no reason given)
why should there be a predominance of prograde over retrograde
That is only if the moon has originated from the parent planet, I would imagine and gas giants having rocky moons is a non sequitur
originally posted by: ErosA433
a reply to: Hyperboles
Conservation of momentum.
The angular momentum does indeed reference moons which originated along with the planet with prograde orbits. The moons with retrograde and eccentric orbits are probably captured and didn't form with the planet.
originally posted by: Hyperboles
That is only if the moon has originated from the parent planet, I would imagine and gas giants having rocky moons is a non sequitur
originally posted by: ErosA433
a reply to: Hyperboles
Conservation of momentum.
I do not agree that rocky moons couldn't have formed around gas giants, since astronomers suspect that the gas giants themselves may have initially formed as rocky planets, before they gained enough mass to pull in hydrogen.
Gas Giants: Facts About the Outer Planets
Astronomers think the giants first formed as rocky and icy planets similar to terrestrial planets. However, the size of the cores allowed these planets (particularly Jupiter and Saturn) to grab hydrogen and helium out of the gas cloud from which the sun was condensing, before the sun formed and blew most of the gas away.
The moons having less gravity than the planet would not be able to pull in as much hydrogen and thus would remain rocky unlike the gas giant around which they formed, which did pull in hydrogen and and other gases.
a reply to: Hyperboles
As Arbitrageur said, it is quite the opposite. If planets form by the process of material accretion it is very logical that local densities coalesce due to the effect of gravity. One thing for certain about the universe is that objects within it tend to rotate. This is true of the gas cloud which the sun was formed from.
Rotation around a mass tends to prevent the objects from joining together, this goes for something like the Sun and the planets. The disk of material that orbited the proto sun is kept stable in that disk because the disk spins or orbits. Material within that disk however is free to clump slowly.
It logically follows that if a planet is orbiting the sun for sake of argument, in an anti-clock wise direction, that when the disk clumps and starts to form planets, that the angular momentum of the disk is conserved. The location of the gas that forms the planet has what is a defuse angular momentum or potential, concentrated as the material forms a planet in a similar way that a ice skater that spins and pulls their body into a tight ball begins to spin faster. It is a similar effect.
The same logical path is that of moons around planets. As a planetary body becomes a certain size, it will form accretion disk like structures around them as a method to pull material onto them from the local cloud and conserve angular momentum. It is then a miniature effect of the proto-planetary disk described above which forms the small rocky or icy moons.
These also conserve angular momentum in the same manner.
The system however is not perfect, it is also entirely possible that planets such as Jupiter and Saturn, with their large gravitational fields, could produce moons that are ejected from orbit due to close passes with other moons etc.
Orbits as we see them now are largely very stable configurations in which you observe distance patterns and resonances between orbital period and rotational period... why? because these states are represent the minimum energy and momentum transfer between the objects in the system. In the early solar system, this stability wasn't fully established and you can easily have a moon ejected from a planetary system only to be recaptured either by a different planet or recaptured in retrograde depending if its captured on the inside or outside of the primary objects orbit.
You then get catastrophic impacts which could cause weird things, like the axial tilt of Uranus
As Arbitrageur said, it is quite the opposite. If planets form by the process of material accretion it is very logical that local densities coalesce due to the effect of gravity. One thing for certain about the universe is that objects within it tend to rotate. This is true of the gas cloud which the sun was formed from.
Rotation around a mass tends to prevent the objects from joining together, this goes for something like the Sun and the planets. The disk of material that orbited the proto sun is kept stable in that disk because the disk spins or orbits. Material within that disk however is free to clump slowly.
It logically follows that if a planet is orbiting the sun for sake of argument, in an anti-clock wise direction, that when the disk clumps and starts to form planets, that the angular momentum of the disk is conserved. The location of the gas that forms the planet has what is a defuse angular momentum or potential, concentrated as the material forms a planet in a similar way that a ice skater that spins and pulls their body into a tight ball begins to spin faster. It is a similar effect.
The same logical path is that of moons around planets. As a planetary body becomes a certain size, it will form accretion disk like structures around them as a method to pull material onto them from the local cloud and conserve angular momentum. It is then a miniature effect of the proto-planetary disk described above which forms the small rocky or icy moons.
These also conserve angular momentum in the same manner.
The system however is not perfect, it is also entirely possible that planets such as Jupiter and Saturn, with their large gravitational fields, could produce moons that are ejected from orbit due to close passes with other moons etc.
Orbits as we see them now are largely very stable configurations in which you observe distance patterns and resonances between orbital period and rotational period... why? because these states are represent the minimum energy and momentum transfer between the objects in the system. In the early solar system, this stability wasn't fully established and you can easily have a moon ejected from a planetary system only to be recaptured either by a different planet or recaptured in retrograde depending if its captured on the inside or outside of the primary objects orbit.
You then get catastrophic impacts which could cause weird things, like the axial tilt of Uranus
Is this a physics question?
So you have two materials in a landfill. One material is much lighter and less dense than the material on top of it (a 15% clay mixture they are calling a "cap"). This landfill is in a floodplain. Is it possible, over time, that the lighter material under the 15% clay "cap" can migrate upwards to the surface because it's lighter, especially if the ground is often saturated with water because of flooding? Thanks in advance-
So you have two materials in a landfill. One material is much lighter and less dense than the material on top of it (a 15% clay mixture they are calling a "cap"). This landfill is in a floodplain. Is it possible, over time, that the lighter material under the 15% clay "cap" can migrate upwards to the surface because it's lighter, especially if the ground is often saturated with water because of flooding? Thanks in advance-
a reply to: CharlieAtTheGap
The details aren't specific enough to answer as a physics question, there are too many unspecified parameters. Solids, liquids and gases all have different properties, and some solids are much more soluble than others. You talk about a lighter material but don't specify what it is.
Landfills which conform to US EPA regulations are supposed to meet the following specifications:
Landfill Design
Here's a schematic diagram:
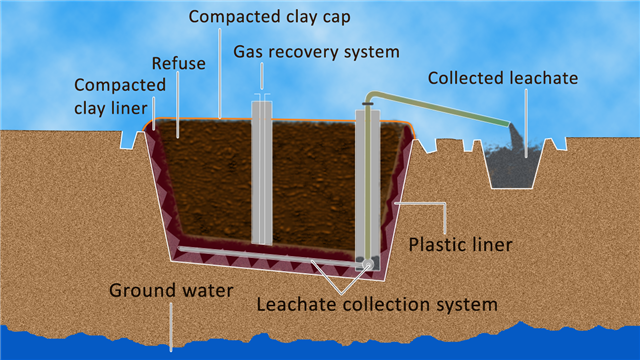
If you mix liquids of different density which are insoluble, like oil and water, the liquid with the lower density or "lighter" liquid in your terminology will rise above the denser liquid. But if the landfill meets the specifications above the liquids should be less than 30cm or one foot high, so this should not allow liquids to reach the top. If the landfill doesn't meet those specifications then again performance depends on specifics.
Solids in the landfill are less mobile than liquids and even though the plastic liner at the bottom of the landfill is less dense than the metallic motor inside the discarded vacuum cleaner above it, the less dense plastic is not going to rise above the more dense metal inside the vacuum cleaner for reasons which hopefully are intuitive and obvious.
The details aren't specific enough to answer as a physics question, there are too many unspecified parameters. Solids, liquids and gases all have different properties, and some solids are much more soluble than others. You talk about a lighter material but don't specify what it is.
Landfills which conform to US EPA regulations are supposed to meet the following specifications:
Landfill Design
According to regulations set by the U.S. Environmental Protection Agency (EPA), the composite liner must have two components: a layer of compacted soil at least two feet thick with a “hydraulic conductivity of no more than 1 X 10-7 centimeters per second. Atop the layer of soil is a flexible membrane liner (FML) at least 30-mil thick.”
“The regulations also require you to manage leachate so that it doesn’t build up on the liner,” says Pat Sullivan, senior vice president and solid waste practice leader for SCS Engineers.
Specifically, EPA regulations require operators to limit leachate buildup on the liner to less than 30 centimeters (just under one foot). To meet the requirement, the leachate management system must be extensive enough to capture the volume of leachate produced by the landfill, which can vary widely.
Here's a schematic diagram:

If you mix liquids of different density which are insoluble, like oil and water, the liquid with the lower density or "lighter" liquid in your terminology will rise above the denser liquid. But if the landfill meets the specifications above the liquids should be less than 30cm or one foot high, so this should not allow liquids to reach the top. If the landfill doesn't meet those specifications then again performance depends on specifics.
Solids in the landfill are less mobile than liquids and even though the plastic liner at the bottom of the landfill is less dense than the metallic motor inside the discarded vacuum cleaner above it, the less dense plastic is not going to rise above the more dense metal inside the vacuum cleaner for reasons which hopefully are intuitive and obvious.
a reply to: Arbitrageur
After watching the video you linked for me in my thread yesterday.
I don't like the coral analogy. It is similar to the Many Worlds theory. Which i thought was just more magic added into science.
Having thought about it. I can see how it could work without the magic.
If every person that ever was, is and ever will be.
Why would you need each individual living infinite infinities of universes etc?
If you now consider that every individual person is the universe in the Many Worlds theory. Then all eventualities can be fulfilled in our reality.
After watching the video you linked for me in my thread yesterday.
I don't like the coral analogy. It is similar to the Many Worlds theory. Which i thought was just more magic added into science.
Having thought about it. I can see how it could work without the magic.
If every person that ever was, is and ever will be.
Why would you need each individual living infinite infinities of universes etc?
If you now consider that every individual person is the universe in the Many Worlds theory. Then all eventualities can be fulfilled in our reality.
a reply to: blackcrowe
I didn't care for what Garrett Lisi said about Coral either, but even though his theory is apparently wrong, I still enjoyed the rest of his presentation, partly because he even admitted it's probably wrong like most high risk ideas in theoretical physics usually are.
However I've been doing some reading about what even I have referred to the "Everett Many-Worlds" interpretation of quantum mechanics, which I now believe to be a misnomer. That idea and phrase was not coined by Everett, but by DeWitt in "The Many Worlds Interpretation of Quantum Mechanics". I don't think Everett's idea was really one of many worlds, but one of a universal wave function, which is in a sense "one world" since that wave function covers everything.
Here's an interesting paper which points out these issues:
Making Sense of the Many Worlds Interpretation
So isn't it interesting that "Many Worlds" is often attributed to Everett, yet he not only didn't use the "Many worlds" terminology but I can't find anything he wrote that was ever his intent. So you may want to read the paper in that link or better yet, read Everett's papers to see what he actually said. I think there are some misunderstandings about what Everett's idea actually was and I was guilty of previously having such a misunderstanding myself. I think Everett was brilliant and when I read his paper it doesn't sound so crazy at all like you make out the "infinite universes" idea to be.
I think the real problem may result from our need to think of a classical-like "branch" because we still want to think classically and DeWitt introduced "Many Worlds"terminology toward this end, but if we can give that up and think more "quantumly" like Everett who didn't use that term and didn't have that idea, then that problem of "multiple universes" goes away, but that's hard to do because quantum mechanics doesn't come to us as naturally as classical physics does.
Maybe quantum mechanics is really the way the universe works, and maybe it's wrong to think of "classical-like" branches in the sense that DeWitt introduced with the "Many Worlds" term, which was not Everett's idea.
I didn't care for what Garrett Lisi said about Coral either, but even though his theory is apparently wrong, I still enjoyed the rest of his presentation, partly because he even admitted it's probably wrong like most high risk ideas in theoretical physics usually are.
However I've been doing some reading about what even I have referred to the "Everett Many-Worlds" interpretation of quantum mechanics, which I now believe to be a misnomer. That idea and phrase was not coined by Everett, but by DeWitt in "The Many Worlds Interpretation of Quantum Mechanics". I don't think Everett's idea was really one of many worlds, but one of a universal wave function, which is in a sense "one world" since that wave function covers everything.
Here's an interesting paper which points out these issues:
Making Sense of the Many Worlds Interpretation
It seems clear that DeWitt and Graham consider that the multitude of branching worlds are “real” in the ordinary sense of the word. In this sense, their Many Worlds perspective certainly departs from Everett’s intent.
In a 1976 philosophy paper on the interpretation of quantum mechanics, Levy-Leblond offers critical comments on the many worlds interpretation and compared it to his understanding of Everett’s theory.
Now, my criticism here is exactly symmetrical of the one I directed against the orthodox position: the “many worlds” idea again is a left-over of classical conceptions. The coexisting branches here, as the unique surviving one in the Copenhagen point of view, can only be related to “worlds” described by classical physics. The difference is that, instead of interpreting the quantum “plus” as a classical “or”, De Witt et al. interpret it as a classical “and”. To me, the deep meaning of Everett's ideas is not the coexistence of many worlds, but on the contrary, the existence of a single quantum one.The main drawback of the “many-worlds” terminology is that it leads one to ask the question of “what branch we are on”, since it certainly looks as if our consciousness definitely belonged to only one world at a time: But this question only makes sense from a classical point of view, once more. It becomes entirely irrelevant as soon as one commits oneself to a consistent quantum view.
In a letter to Levy-Leblond (Barrett 2011), Everett indicated that he quite agreed with Levy-Leblond’s argument and emphasized that the many worlds terminology was not his. I’m sympathetic with this view.
So isn't it interesting that "Many Worlds" is often attributed to Everett, yet he not only didn't use the "Many worlds" terminology but I can't find anything he wrote that was ever his intent. So you may want to read the paper in that link or better yet, read Everett's papers to see what he actually said. I think there are some misunderstandings about what Everett's idea actually was and I was guilty of previously having such a misunderstanding myself. I think Everett was brilliant and when I read his paper it doesn't sound so crazy at all like you make out the "infinite universes" idea to be.
I think the real problem may result from our need to think of a classical-like "branch" because we still want to think classically and DeWitt introduced "Many Worlds"terminology toward this end, but if we can give that up and think more "quantumly" like Everett who didn't use that term and didn't have that idea, then that problem of "multiple universes" goes away, but that's hard to do because quantum mechanics doesn't come to us as naturally as classical physics does.
Maybe quantum mechanics is really the way the universe works, and maybe it's wrong to think of "classical-like" branches in the sense that DeWitt introduced with the "Many Worlds" term, which was not Everett's idea.
edit on 2019422 by Arbitrageur because: clarification
a reply to: Arbitrageur
Thanks for the reply.
I think you make some very good points.
I will read the link. Thanks.
I had read about Everett. It was more of a biography style article.
And. Yes. It wasn't himself promoting the idea. But, others like DeWitt.
Although i disagreed with the Many Worlds Interpretation.
I do like how different people think and try to justify their existence.
Maybe i'll never know the answer in my lifetime.
If the answers are found however. I would prefer a person thought it rather than a future computer.
It's always interesting.
Thanks for the reply.
I think you make some very good points.
I will read the link. Thanks.
I had read about Everett. It was more of a biography style article.
And. Yes. It wasn't himself promoting the idea. But, others like DeWitt.
Although i disagreed with the Many Worlds Interpretation.
I do like how different people think and try to justify their existence.
Maybe i'll never know the answer in my lifetime.
If the answers are found however. I would prefer a person thought it rather than a future computer.
So isn't it interesting
It's always interesting.
Lol Ques:
What role does time play in nuclear and thermonuclear weapons?
What role does time play in nuclear and thermonuclear weapons?
See the time stamp and distance scale of this photo of the first atomic test?
originally posted by: Hyperboles
Lol Ques:
What role does time play in nuclear and thermonuclear weapons?

Estimate of the energy released in the first Atomic Bomb explosion.
Using that information, a physicist was able to look at that photo published in a magazine and estimate the yield which was still a secret. Without the time notation he wouldn't have been able to do that. The related maths explaining the relationship of time and other factors he used for the estimate are in the attached pdf. I thought this was an interesting problem and solution, which seems related to your rather vague question.
a reply to: Arbitrageurthanks, i will chk the link. tho actually I meant what role does time play in setting off the fission and
fusion processes themselves, in both the devices
a reply to: Hyperboles
nuclearweaponarchive.org...
Time is an independent variable. The reaction time is dependent on the neutron (I think). If we were observing the same reaction in a distant galaxy, it would appear to be slower due to time dilation.
If time played a part in the reaction (faster/slower), then you would have to be able to control time which, to my knowledge, is not possible.
2.1.3 Time Scale of the Fission Reaction
The amount of time taken by each link in the chain reaction is determined by the speed of the neutrons and the distance they travel before being captured. The average distance is called the mean free path. In fissile materials at maximum normal densities the mean free path for fission is roughly 13 cm for 1 MeV neutrons (a typical energy for fission neutrons). These neutrons travel at 1.4x10^9 cm/sec, yielding an average time between fission generations of about 10^-8 sec (10 nanoseconds), a unit of time sometimes called a "shake". The mean free path for scattering is only 2.5 cm, so on average a neutron will be scattered 5 times before causing fission. Actual 1 MeV mean free path values are: Density M.F.P. (cm) U-233 18.9 10.9 U-235 18.9 16.5 Pu-239 19.4 12.7 This shows that fission proceeds faster in some isotopes than others. The rate of multiplication can be calculated from the multiplication coefficient k given by: k = f - (lc + le) where f = avg. neutrons generated per fission lc = avg. neutrons lost to capture le = avg. neutrons lost by escaping assembly When k = 1 an assembly is exactly critical and a chain reaction will be self supporting, although it will not increase in rate. When k 1 then it is super-critical and the reaction will continually increase. To make an efficient bomb k must be as high as possible, usually somewhere near 2, when the chain reaction starts.
Many discussions of fission describe the chain reaction as proceeding by discrete generations. Generation zero has 1 neutron, generation one has 2 neutrons, generation two has 4 neutrons, etc. until, say, 2x10^24 atoms have been split - which produces 20 kilotons of energy. The formula for this is: Number of atoms split = 2^(n-1), where n is the generation number. Thus 2x10^24 = 2^(n-1) implies n = (log2 (2x10^24)) + 1 = 81.7 generations. That is, it takes about 82 generations to complete the fission process for a 20 kiloton bomb, if the reaction starts from one neutron. This calculation is a useful simplification, but the fission process does not really proceed by separate steps, each completing before the next begins. It is really a continuous process, the current oldest generation of neutrons starts creating the next generation even while it is still being formed by neutrons from still older generations. An accurate calculation thus requires the use of formulas derived from calculus. We find that both the number of neutrons present in the assembly (and thus the instantaneous rate of the fission reaction), and the number of fissions that have occurred since the reaction began, increase at a rate proportional to e^((k-1)*(t/g)), where e is the natural log base (2.712...), g is the average generation time (time from neutron emission to fission capture), and t is the elapsed time. If k=2, then a single neutron will multiply to 2x10^24 neutrons (and splitting the same number of atoms) in roughly 56 shakes (560 nanoseconds), yielding 20 kilotons of energy. This is one-third less time than the previous approximate calculation. Due to the exponential rate of increase, at any point in the chain reaction 99% of the energy will have been released in the last 4.6 generations. It is a reasonable approximation to think of the first 53 generations as a latency period leading up to the actual explosion, which only takes 3-4 generations. The extremely rapid buildup in the fission rate as the reaction proceeds has some important consequences that should be pointed out.
The longer a neutron takes to cause fission, the less significant it is in contributing to the chain reaction. This is because it becomes quickly outnumbered by the descendants of neutrons that undergo fission capture sooner.Thus faster, more energetic, neutrons contribute disproportionately compared to slower neutrons. This is called "time absorption" since it has the same effect as a neutron absorber with a cross-section inversely proportional to velocity. Similarly, if a neutron leaves the critical mass and is scattered back in, then its contribution is also considerably reduced. In fact since the path of a neutron that leaves the critical assembly, then re-enters is much longer than the average path of neutron that remains within the mass the time absorption in a reflector is very large.
nuclearweaponarchive.org...
Time is an independent variable. The reaction time is dependent on the neutron (I think). If we were observing the same reaction in a distant galaxy, it would appear to be slower due to time dilation.
If time played a part in the reaction (faster/slower), then you would have to be able to control time which, to my knowledge, is not possible.
new topics
-
Petition Calling for General Election at 564,016 and rising Fast
Social Issues and Civil Unrest: 2 hours ago -
Rep. Alexandria O. Cortez Says Forcing People to Use The Correct Bathroom is Dangerous.
US Political Madness: 9 hours ago -
Cooperation zones
World War Three: 10 hours ago
top topics
-
France gives Ukraine license to fire long-range missiles at Russia
World War Three: 14 hours ago, 9 flags -
Rep. Alexandria O. Cortez Says Forcing People to Use The Correct Bathroom is Dangerous.
US Political Madness: 9 hours ago, 5 flags -
Petition Calling for General Election at 564,016 and rising Fast
Social Issues and Civil Unrest: 2 hours ago, 5 flags -
Ok this is some BS now WTH
Rant: 12 hours ago, 4 flags -
Cooperation zones
World War Three: 10 hours ago, 3 flags -
Stalker 2 - Review from a Veteran
Video Games: 17 hours ago, 2 flags
active topics
-
Petition Calling for General Election at 564,016 and rising Fast
Social Issues and Civil Unrest • 14 • : gortex -
Well, here we go red lines crossed Biden gives the go ahead to use long range missiles
World War Three • 338 • : Xtrozero -
Jaguar Rebrand Video Causes "WTF?" Moment - Seriously Weird
Automotive Discussion • 32 • : gortex -
Biden's "Reckless" Decision To Escalate Russia-Ukraine War
World War Three • 124 • : PrivateAngel -
Well we know Putins ICBMs won't fail in their silos
World War Three • 213 • : Xtrozero -
Rep. Alexandria O. Cortez Says Forcing People to Use The Correct Bathroom is Dangerous.
US Political Madness • 21 • : UpIsNowDown2 -
Congress 2024 - We do not want me in our ladies rooms
US Political Madness • 21 • : TheSingleBillie -
Results of the use of the Oreshnik missile system in Dnepropetrovsk
World War Three • 166 • : Oldcarpy2 -
Cooperation zones
World War Three • 14 • : nugget1 -
France gives Ukraine license to fire long-range missiles at Russia
World War Three • 20 • : 38181
