It looks like you're using an Ad Blocker.
Please white-list or disable AboveTopSecret.com in your ad-blocking tool.
Thank you.
Some features of ATS will be disabled while you continue to use an ad-blocker.
share:
reply to post by Americanist
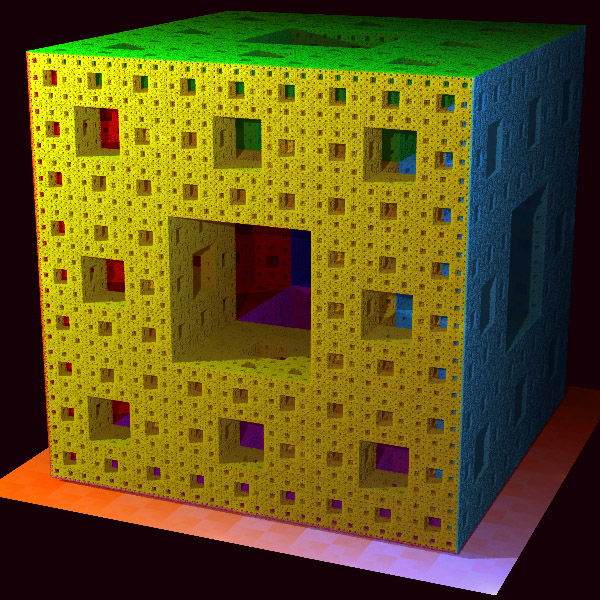
Might this be the pyramid do you think?

en.wikipedia.org...:Sierpinski_pyramid.jpg
It is the sierpenski triangle fractal and is one of quantum physics leading contenders for the shape of the universe. Those that study esoteric info would know it as the same thing by a different name -
The flower of life.
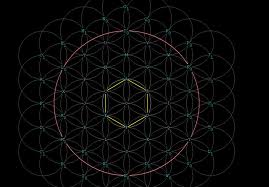
Are these two compressed into something like this?
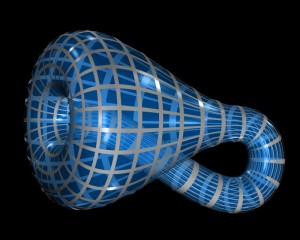
hans.wyrdweb.eu...
Add my avatar maybe - the golden mean.
Originally posted by Americanist
If you were to step back, and take a look at the whole of Creation...
You would see a pyramid with the cube for a cap stone.
Now imagine living inside this structure being collapsed all around you.
Source
Vortex radio waves could boost wireless capacity “infinitely”
Source
edit on 15-10-2012 by Americanist because: (no reason given)
Might this be the pyramid do you think?

en.wikipedia.org...:Sierpinski_pyramid.jpg
It is the sierpenski triangle fractal and is one of quantum physics leading contenders for the shape of the universe. Those that study esoteric info would know it as the same thing by a different name -
The flower of life.

Are these two compressed into something like this?

hans.wyrdweb.eu...
Add my avatar maybe - the golden mean.
edit on 25-10-2012 by primalfractal because: (no reason given)
edit on 25-10-2012 by
primalfractal because: (no reason given)
reply to post by primalfractal
How does the flower of life figure into the pyramid? The flower of life only hints at a pyramidal shape if one circle is taken and lines drawn through, ORRRR....if it is followed through with the fruit of life. The fruit of life is the closest you'll come to comparing the flower of life with the pyramid.
How does the flower of life figure into the pyramid? The flower of life only hints at a pyramidal shape if one circle is taken and lines drawn through, ORRRR....if it is followed through with the fruit of life. The fruit of life is the closest you'll come to comparing the flower of life with the pyramid.
reply to post by AfterInfinity
Yes the fruit matches the pattern. The flower also maps perfectly onto the triangle. Not that easy to do though by the account on the link and I dont know if anyone else has.
Exploring Sierpinski’s Triangle - Link

www.phidelity.com...
Yes the fruit matches the pattern. The flower also maps perfectly onto the triangle. Not that easy to do though by the account on the link and I dont know if anyone else has.
Exploring Sierpinski’s Triangle - Link

www.phidelity.com...
Originally posted by AfterInfinity
reply to post by primalfractal
How does the flower of life figure into the pyramid? The flower of life only hints at a pyramidal shape if one circle is taken and lines drawn through, ORRRR....if it is followed through with the fruit of life. The fruit of life is the closest you'll come to comparing the flower of life with the pyramid.
The flower of life is a 2-dimensional representation of the tube torus.
reply to post by primalfractal
Use the cantor set - The menger sponge is a 3D representation. The stacked (step) pyramid is your visual aid along with bubbles inside bubbles... "Everything in its Right Place" driven through the various scales/ occasions of our Universe.
Use the cantor set - The menger sponge is a 3D representation. The stacked (step) pyramid is your visual aid along with bubbles inside bubbles... "Everything in its Right Place" driven through the various scales/ occasions of our Universe.
Quick question for the resident quantum buffs who may be lurking this thread:
Has complimentarity been disproven? What are the arguments against it?
Has complimentarity been disproven? What are the arguments against it?
reply to post by AfterInfinity
john Von neumann, later in his life, claimed that complimentarity is a pseudo-problem which arises from the way quantum mechanics is represented mathematically. I rather like his interpretation, but his "new method" (rejection of the Hilbert space) was ignored and is now lost.
db.tt...
john Von neumann, later in his life, claimed that complimentarity is a pseudo-problem which arises from the way quantum mechanics is represented mathematically. I rather like his interpretation, but his "new method" (rejection of the Hilbert space) was ignored and is now lost.
db.tt...
pg868...it might be possible to describe intellectual processes (therefore ones essentially connected to life) in a non-geometrical manner (without locating them spatially). (von Neumann, 1939)
The observer is thought of as a non-geometrical concept, whose existence in space is quite irrelevant to quantum mechanics. To avoid pseudo-problems arising, the intellectual processes ascribed to the observer should not be described in spatial terms. This does not imply that they must be described in psychological terms though. Some other description is in fact available. von Neumann did not elaborate on this point further, except for suggesting that transformation theory in quantum mechanics would provide the framework for such a non-spatial description. Recall that quantum probabilities are defined by invariance under the transformations of the logical structure onto itself. Arguably, information theory offers a quantitative, non-psychological account of certain intellectual processes of the observer, such as computation, that integrates logic and probability.
reply to post by tgidkp
I failed as soon as the terms 'phase space' and 'spin matrix' came into view.
But perhaps not all is lost.
I clicked on this link, from the sandy/haarp one (OMG) because you said something about explaining how quantum mechanics is used in radio signals, and I really want to understand how 'quantum' relates to real-world engineering; but it doesn't seem to have been mentioned?
I haven't read the rest of the thread yet, so maybe it's in there.
Anyway, assuming you are talking to a primary school-level educated child, can you explain to me how quantum mechanics is used in computing programs, and if and how it is also used in cpu hardware, because I've heard the phrase 'quantum computers' somewhere and I've wondered wwhat the hell that means.
Also, and this is a seperate field but you seem to know stuff, is it true, and if so how and why, that einstin-ien relativity is proved by current computers and general technology? I don't know where I heard this (probably here somewhere) but it sounds intriguing. Again, remember you are explaining to a primary-school level mind.
Thanks.
Originally posted by tgidkp
....
see. that wasnt so hard, now, was it?
thanks for reading!
I failed as soon as the terms 'phase space' and 'spin matrix' came into view.
But perhaps not all is lost.
I clicked on this link, from the sandy/haarp one (OMG) because you said something about explaining how quantum mechanics is used in radio signals, and I really want to understand how 'quantum' relates to real-world engineering; but it doesn't seem to have been mentioned?
I haven't read the rest of the thread yet, so maybe it's in there.
Anyway, assuming you are talking to a primary school-level educated child, can you explain to me how quantum mechanics is used in computing programs, and if and how it is also used in cpu hardware, because I've heard the phrase 'quantum computers' somewhere and I've wondered wwhat the hell that means.
Also, and this is a seperate field but you seem to know stuff, is it true, and if so how and why, that einstin-ien relativity is proved by current computers and general technology? I don't know where I heard this (probably here somewhere) but it sounds intriguing. Again, remember you are explaining to a primary-school level mind.
Thanks.
reply to post by delusion
i have received some critisism of the apparent lack of sufficient explanation in this presentation. this is strange to me because i remember very clearly what it is like to be utterly confused by the actual application of quantum mechanics, and this post was written from the perspective of what i might like to have known at that time. i am still considering making a more explicit example, but honestly, i think it will be met with the same critisism. my main purpose was to show with visual aids (i always think math is too short on the graphic visualizations), how the various jargon of the science correlates to the evolution of an actual quantum system.
so, about the phase space. this is a great place to start your learning. think for a moment about the difference in characteristics of a laser light versus an incandescent bulb. the primary difference between the two can be described in terms of PHASE. whereas the laser has a coherent phase, the bulb has a mixed phase. conceptually, the phase of a quantum system is represented as a space called the 'Hilbert Space', also known as the phase space.
i am sure you have heard talk about "superposition" in quantum science? the superposition can be thought of as the full spectrum of angles between 0 and 90 degrees. usually, say in a classical computer, a bit is either ON or OFF. in the hilbert space, we could say that the classical bit is at 0degrees (off) or 90degrees (on)....but only one or the other. but in a quantum system, the phase angle can be represented at any position between 0 and 90. the phase of a qubit is represented as a superposition of both 0 and 90 (a little bit of both). like the sine and cosine of trionometry.
because of superposition, a single quantum system can interfere with itself. if you imagine a wave in the ocean, and another wave opposite in phase (one wave is at the top while the other wave is at the bottom), you can easily understand that the two waves will cancel each other out. so the "wave-state" of the entire wave-like system depends on the exact phase of all of the components in the system acting upon each other, cancelling and amplifying and producing all kinds of sometimes very striking dynamics (re: cymatics).
and this is the biggest difference between a classical and quantum computer. a classical computer requires a single bit to represent every single transition state in the system....and the system is dynamically changing from moment to moment....that is a LOT OF BITS! and very difficult for a classical computer to handle.
but a quantum computer, because of superposition, requires only one qubit to represent every single transition state in the system. this is because the qubit IS THE TRANSITION STATE, itself. this is why, during the OP i stressed so heavily the nature of the STATE FUNCTION. if you can understand this, you have understood quantum mechanics.
so, going back to the OP: you are looking at a single state function = a single qubit. the information content of the qubit is contained as the state of the entire system. but representation in a classical computer would represent all of the positions of the matrix as a single bit, as a sequence of one after the other, and the classical computer will never "comprehend" the state of the system, or that the system has a coherent state at all.
quantum mechanics is a description of the state of the wavefunction in the phase space according to a given unit of measurement.
.....for anyone wanting to learn the math specifically, you may view the sequence of spin values down the left column as the eigenstates of the KET and the sequence of values along the top row as the BRA, using the standard bra-ket notaion. the bra and ket represent the 0 and 90 degrees 'basis states' of the phase space, and the superposition of the wave function is expressed in the pattern of intermediate spin values over the full matrix of possible quantization values between the bra and ket.
and at the very heart of it all is the (totally anti-intuitive) mathematical definition known as the canonical commutator relation:
pq - qp = ih
where p is the bra, q is the ket , i is the imaginary number, and h is ??????
in standard math the following is usually true:
pq - qp = 0
but, you see, the wavefunction on the hilbert space always leaves you with some spare change. this should strike you as very, very strange. back when QM was developed, h had not been standardized. h was just a bunch of weird numbers that kept falling out of the math when it should have gone to 0 but didnt. the value of h was a major source of anxiety among the developers. but h has been standardized as the plank's constant.
it is the plank's constant, h, which is the quantization of the quantum math function.
the phase space NEVER goes to 0 (fully nilpotent
i have received some critisism of the apparent lack of sufficient explanation in this presentation. this is strange to me because i remember very clearly what it is like to be utterly confused by the actual application of quantum mechanics, and this post was written from the perspective of what i might like to have known at that time. i am still considering making a more explicit example, but honestly, i think it will be met with the same critisism. my main purpose was to show with visual aids (i always think math is too short on the graphic visualizations), how the various jargon of the science correlates to the evolution of an actual quantum system.
so, about the phase space. this is a great place to start your learning. think for a moment about the difference in characteristics of a laser light versus an incandescent bulb. the primary difference between the two can be described in terms of PHASE. whereas the laser has a coherent phase, the bulb has a mixed phase. conceptually, the phase of a quantum system is represented as a space called the 'Hilbert Space', also known as the phase space.
i am sure you have heard talk about "superposition" in quantum science? the superposition can be thought of as the full spectrum of angles between 0 and 90 degrees. usually, say in a classical computer, a bit is either ON or OFF. in the hilbert space, we could say that the classical bit is at 0degrees (off) or 90degrees (on)....but only one or the other. but in a quantum system, the phase angle can be represented at any position between 0 and 90. the phase of a qubit is represented as a superposition of both 0 and 90 (a little bit of both). like the sine and cosine of trionometry.
because of superposition, a single quantum system can interfere with itself. if you imagine a wave in the ocean, and another wave opposite in phase (one wave is at the top while the other wave is at the bottom), you can easily understand that the two waves will cancel each other out. so the "wave-state" of the entire wave-like system depends on the exact phase of all of the components in the system acting upon each other, cancelling and amplifying and producing all kinds of sometimes very striking dynamics (re: cymatics).
and this is the biggest difference between a classical and quantum computer. a classical computer requires a single bit to represent every single transition state in the system....and the system is dynamically changing from moment to moment....that is a LOT OF BITS! and very difficult for a classical computer to handle.
but a quantum computer, because of superposition, requires only one qubit to represent every single transition state in the system. this is because the qubit IS THE TRANSITION STATE, itself. this is why, during the OP i stressed so heavily the nature of the STATE FUNCTION. if you can understand this, you have understood quantum mechanics.
so, going back to the OP: you are looking at a single state function = a single qubit. the information content of the qubit is contained as the state of the entire system. but representation in a classical computer would represent all of the positions of the matrix as a single bit, as a sequence of one after the other, and the classical computer will never "comprehend" the state of the system, or that the system has a coherent state at all.
quantum mechanics is a description of the state of the wavefunction in the phase space according to a given unit of measurement.
.....for anyone wanting to learn the math specifically, you may view the sequence of spin values down the left column as the eigenstates of the KET and the sequence of values along the top row as the BRA, using the standard bra-ket notaion. the bra and ket represent the 0 and 90 degrees 'basis states' of the phase space, and the superposition of the wave function is expressed in the pattern of intermediate spin values over the full matrix of possible quantization values between the bra and ket.
and at the very heart of it all is the (totally anti-intuitive) mathematical definition known as the canonical commutator relation:
pq - qp = ih
where p is the bra, q is the ket , i is the imaginary number, and h is ??????
in standard math the following is usually true:
pq - qp = 0
but, you see, the wavefunction on the hilbert space always leaves you with some spare change. this should strike you as very, very strange. back when QM was developed, h had not been standardized. h was just a bunch of weird numbers that kept falling out of the math when it should have gone to 0 but didnt. the value of h was a major source of anxiety among the developers. but h has been standardized as the plank's constant.
it is the plank's constant, h, which is the quantization of the quantum math function.
the phase space NEVER goes to 0 (fully nilpotent
Can you explain the mathematics in the images in non-mathematical layman's terms? I have dyscalculia and poor "working memory" when it comes to
abstract numbers, and never got very far beyond basic fractions (with which I still struggle.) I understand the "gist" from your words and the
pictures of the emerging symmetry, but would love to have a slightly more comprehensive understanding of what the math itself is saying.
I know that may be a tall order as some things can only be described via math. Thanks for trying if you can, though. Peace.
I know that may be a tall order as some things can only be described via math. Thanks for trying if you can, though. Peace.
You failed to mention alot. Your just reiterating my original point, even you who seems to have a solid understanding of quantum mechanics are blowing
past almost all of the fundamentals. You failed to give an explaination for why energy is quantized, and a perfect way is to go threw the history of
what planck discovered. Quantization is the entire basis for QM, and without it the entire idea of the wave function is useless(the s wave equation
has h right in it!)
You also failed to explain non-locality when talking about superposition. This is a fail of epic proportions! Especially for a newb style guide!
As for complementarity not being significant. A lot of neutron interferometry experiments show the subtlety of the notions of duality and complementarity, but they are still present.
You also failed to explain non-locality when talking about superposition. This is a fail of epic proportions! Especially for a newb style guide!
As for complementarity not being significant. A lot of neutron interferometry experiments show the subtlety of the notions of duality and complementarity, but they are still present.
edit on 29-10-2012 by ubeenhad because: (no reason given)
reply to post by ubeenhad
he asked about the phase space and quantum effects in computation. as I said, I think that is a great place to start.
but I do have a (hopefully not lame) explanation of WHY energy is quantized...all due respect to Planck.
I drive a CNG (compressed natural gas) vehicle. the fueling routine is quite different than for petrolium as the pump outlet has a locking mechanism onto the car's fuel input. so the phenomenon I descr8be here is occurring in a very tightly coupled system where the gas pressure on the one end is much much higher than the other.
as fueling begins, the coupling lets out a bit of a whine at a very particular frequency. after about a minute, the decreasing pressure difference enables a more stable vibration mode and the whine starts to bifurcate between the initial tone and the new one....slowly at first...jumping back and forth somewhat randomly...and finally ending up emmitting the new tone only.
I really like this example of energetic quantization because 1) it is non standard and shows that quantum effects are more common and show up more places than "they" would have you believe. and more importantly 2) it shows that quantization is not a property of the energy itself, but rather, quantization occurrs because of the INTERACTION (re:state function) of the energy with the specific conditions of the physical system through which it is manifest.
and, as it turns out my car's physical coupling is only stable at very specific frequencies. someone else's car will have a totally different response at an equivalent energy (pressure).
he asked about the phase space and quantum effects in computation. as I said, I think that is a great place to start.
but I do have a (hopefully not lame) explanation of WHY energy is quantized...all due respect to Planck.
I drive a CNG (compressed natural gas) vehicle. the fueling routine is quite different than for petrolium as the pump outlet has a locking mechanism onto the car's fuel input. so the phenomenon I descr8be here is occurring in a very tightly coupled system where the gas pressure on the one end is much much higher than the other.
as fueling begins, the coupling lets out a bit of a whine at a very particular frequency. after about a minute, the decreasing pressure difference enables a more stable vibration mode and the whine starts to bifurcate between the initial tone and the new one....slowly at first...jumping back and forth somewhat randomly...and finally ending up emmitting the new tone only.
I really like this example of energetic quantization because 1) it is non standard and shows that quantum effects are more common and show up more places than "they" would have you believe. and more importantly 2) it shows that quantization is not a property of the energy itself, but rather, quantization occurrs because of the INTERACTION (re:state function) of the energy with the specific conditions of the physical system through which it is manifest.
and, as it turns out my car's physical coupling is only stable at very specific frequencies. someone else's car will have a totally different response at an equivalent energy (pressure).
We must asume behind this
force the existence of a
conscious inteligent mind
This mind is the matrix of
all matter.
Max Plank
Any thoughts?
reply to post by tgidkp
Thanks, that's a slightly better hand-holding for me. You've given my brain something to chew. As long a there's not a test afterwards.
Thanks, that's a slightly better hand-holding for me. You've given my brain something to chew. As long a there's not a test afterwards.
reply to post by tgidkp
With my theory I was wondering how a "probability" or mathematical wave could cause spin. Also the way I expect the wave to hold onto the barrel seems to show the wave is more than mathematical. I can't see why a mathematical wave, already part extruded, would have any reason to move with the barrel.
With my theory I was wondering how a "probability" or mathematical wave could cause spin. Also the way I expect the wave to hold onto the barrel seems to show the wave is more than mathematical. I can't see why a mathematical wave, already part extruded, would have any reason to move with the barrel.
reply to post by primalfractal
the wavefunction statistics do not conform to a linear time/space frame. I have said many times now that the state function is NONlinear. you appear to still be thinking about this in a sequential context.
non-linear = non-sequential.
the wavefunction exists ALL AT ONCE, in as many dimensions (degrees of freedom) as is necessary to describe it. this is the great paradox.
the mathematical model does not "cause" anything. QM is purely phenomenological.
the wavefunction statistics do not conform to a linear time/space frame. I have said many times now that the state function is NONlinear. you appear to still be thinking about this in a sequential context.
non-linear = non-sequential.
the wavefunction exists ALL AT ONCE, in as many dimensions (degrees of freedom) as is necessary to describe it. this is the great paradox.
the mathematical model does not "cause" anything. QM is purely phenomenological.
reply to post by tgidkp
Thanks, I agree. Others said otherwise.
Would your wavefunction theory above be classed as an "ether" theory or is it standard QM?
the wavefunction exists ALL AT ONCE, in as many dimensions (degrees of freedom) as is necessary to describe it. this is the great paradox.
Thanks, I agree. Others said otherwise.
Would your wavefunction theory above be classed as an "ether" theory or is it standard QM?
new topics
-
Gen Flynn's Sister and her cohort blow the whistle on DHS/CBP involvement in child trafficking.
Whistle Blowers and Leaked Documents: 1 hours ago -
Anybody else using Pomodoro time management technique?
General Chit Chat: 3 hours ago -
Bucks County commissioners vote to count illegal ballots in Pennsylvania recount
2024 Elections: 6 hours ago -
Trump sues media outlets -- 10 Billion Dollar lawsuit
US Political Madness: 6 hours ago -
Fired fema employee speaks.
US Political Madness: 7 hours ago -
How long till it starts
US Political Madness: 8 hours ago -
USSS Agent Fired for Having Sex In Michelle Obama's Bathroom
Politicians & People: 10 hours ago
top topics
-
Trump sues media outlets -- 10 Billion Dollar lawsuit
US Political Madness: 6 hours ago, 21 flags -
Bucks County commissioners vote to count illegal ballots in Pennsylvania recount
2024 Elections: 6 hours ago, 17 flags -
How long till it starts
US Political Madness: 8 hours ago, 15 flags -
USSS Agent Fired for Having Sex In Michelle Obama's Bathroom
Politicians & People: 10 hours ago, 9 flags -
Fired fema employee speaks.
US Political Madness: 7 hours ago, 9 flags -
Watching TV
Jokes, Puns, & Pranks: 13 hours ago, 8 flags -
Gen Flynn's Sister and her cohort blow the whistle on DHS/CBP involvement in child trafficking.
Whistle Blowers and Leaked Documents: 1 hours ago, 3 flags -
Anybody else using Pomodoro time management technique?
General Chit Chat: 3 hours ago, 2 flags
active topics
-
President-Elect DONALD TRUMP's 2nd-Term Administration Takes Shape.
Political Ideology • 199 • : xuenchen -
The Trump effect 6 days after 2024 election
2024 Elections • 138 • : Xtrozero -
The Acronym Game .. Pt.4
General Chit Chat • 959 • : JJproductions -
Trump sues media outlets -- 10 Billion Dollar lawsuit
US Political Madness • 34 • : WeMustCare -
Bucks County commissioners vote to count illegal ballots in Pennsylvania recount
2024 Elections • 17 • : xuenchen -
How can you defend yourself when the police will not tell you what you did?
Posse Comitatus • 81 • : SCUBAPAUL -
Gen Flynn's Sister and her cohort blow the whistle on DHS/CBP involvement in child trafficking.
Whistle Blowers and Leaked Documents • 5 • : xuenchen -
Anybody else using Pomodoro time management technique?
General Chit Chat • 6 • : Cloudbuster1 -
How long till it starts
US Political Madness • 11 • : awhispersecho -
WATCH LIVE: US Congress hearing on UFOs, unidentified anomalous phenomena
Aliens and UFOs • 83 • : DaydreamerX