Limits at Infinity, the number 1 is bigger than you may think

I post this due to recent contemplations I have been considering. This is a long thread that I hope you read, but if you really don’t want to then skip to the bottom, the last line, before you decide not to give it a chance. Honestly, I really think there needs to be a new "math-topics" forum, but anyway...

We shall examine a few simple (real) numbers and what they really represent in this thread.
There are 3 main points in this thread (which I will try very hard to relate at the end, despite my inability to communicate my ideas fluidly):

1. Nothing is exact, it is impossible to determine the actual quantity of a real object, as a quantity is only expressed as a unit which is a generalization.

2. I ask you to broaden your mind, and think about how the related rates of the world and the interaction between all the numbers you can think of what they really mean. Consider the fact that between each integer lies an infinite possible amount of numbers, and that each decimal has some decimal slightly larger than it as well as some decimal slightly less than it.

3. Infinity itself is a quantization. It is an assumption that we make, and the definition can be treated similarly to the rest of the numerical symbols that have been created, and thusly makes some infinities larger than others.

FIRST

Examine the hand you are typing with and think of what your hand would be like with just one more skin cell or perhaps just one less... does it appear to be any different when you think about it? That hand you see, you have always called... your hand. It has never lost a skin cell and then you say: well here is most of my hand. You wash your hand, you rub them together, you give someone a high five, yet you never realize that you are losing skin cells in the process. Yet, you always refer to your hand as being the same thing, day after day. You never realize all the skin that is lost, because your hand will replace the skin cells at a rate equal to (or close to) the rate at which you lose them... this hand that you have always referred to is considered a unit.

Ok so that is not hard to imagine at all, and perhaps you even see where I am going with this, but just in case let me explain with another example before I continue with my point.

Let us pretend you have three apples--go ahead, picture it in your mind. These apples may be oddly shaped, but more importantly we are going to consider them to be normal apples. What does normal mean? Well, statistically it means that all three of your apples fall within 3 standard deviations (or 99.7%) of all the apple data you can think of. Their shape, weight, nutritional value, ripeness, age, color, etc. all fall into the category of normal. Normal from what? In statistics we can relate everything to a, more or less, perfect apple. This apple we relate data to is the average-in-every-way apple. It has the exact mean, or average, of every apple that ever existed, and in every category assumed with apples. Now, this specific apple we relate things to may have a mean shape, weight, nutritional value, ripeness, age, color, etc, but to say that an apple with "perfect" everything (or average everything) at one point existed may not necessarily be true. There is a very likely chance that that apple never existed, and may never exist in time (all speculative of course). There may have been apples very close to being perfect, but there may have always been one conflicting variable in which it was slightly above or below average. And of course, as a mathematician, I can say that there may have even been a perfect apple at one point in time, but likely not many more than one, if any.

So what does this have to do with numbers? Well, in theory, all a number is is a symbol. It represents a way to quantitatively categorize some unit of matter/energy. Ex. 2 hands, 3 apples, 975 Watts, 321 MB... etc. But when we say a number, we are only ever really approximating. Those 3 apples we mentioned, are not perfect apples, and thusly are not exactly 3 apples. They may be 3.00000145 apples, or if they are very normal apples, then maybe they are 3.0000000000000047 apples, but not exactly 3. apples. So in theory, the chances of any number you can think of as existing may not actually exist in the real world. The probability of those apples existing as 3., or 3.00000145, or 3.0000000000000047 are as equally unlikely...

SECOND

So now we look at the conceptuality of the numerical system, and the units that may encumber all numbers, regardless of practical application...

What is, just 1? 1 is a number and aside from the units and imprecision in the "real-world", 1 can be dealt with graphically. Graphs, mathematically, are perfect. If you graph "1" in a Cartesian plane without defining parameters, it exists as nothing but a concept. If you define it, say by saying that y=1, then you have a horizontal line. For every x point that you can discover then has a set value of y=1. For that matter, regardless of x, you know that y=1 (for the only y that exists here is 1).

1 can also exist as, say, a radius. If you have a generic unit circle, and we can determine that its radius equals one, then for every point on the graph that exists a line, it has exactly 1 unit of distance away from the origin (the origin is also called the pole if you are graphing in polar mode, something you should look up if you need clarification here).

Now, before we continue on graph-theory, I do wish you to keep some things in mind. We are made up of atoms. Each atom is made up of subatomic particles, and perhaps each subatomic particle is made up of other things. Now, atoms make up molecules, molecules make up substances, substances make up objects, objects make up systems of objects, systems of objects make up the world, worlds make up a solar system, a solar system makes up a universe, and blah blah blah. It goes on forever as far as we know—in both directions. This means, that for everything you can think of small scale, there could be some macro version of it somewhere, sometime. So to say this does not apply graphically, would be inaccurate.

Still have that circle in mind, with radius 1? That circle has a radius of 1 and therefore a hypothetical area value of π. So an area of π perhaps, yet there seems to be one interesting aspect to this circle. Inside the circle lie an infinite number of points! Each data point has a value of zero, because as you zoom in further and further on a graph you can never actually see a data point, and you never will. There are an infinite number of points, each with an area of zero… yet the addition of all the points brings the circle to an area of π? So 0*∞= π? No, that does not seem to be right…

So now where does that leave us? Go ahead, get up and get a drink of water and maybe call a friend. You need time for this to soak in.

Back? Ok, so now where did we leave off… ah yes, an infinite amount of zero can actually equal some number. Yet, different infinite amounts of zero will lead us to different numbers each time.

THIRD

What is infinity? Infinity is... huge. Is it not? Well, you may argue that infinity is the largest number you know of plus 1, but in reality, infinity is perhaps much large than that and isn't really a number at all. Infinity describes inexact yet predictable amount of something. If I told you to walk for an infinite amount of time, then you would end up traveling an infinite distance, eventually. If I told you to count forever, then you would never reach infinity, and if I divided a cookie in half forever, I would never actually be able to stop dividing. However, I still think some infinities are bigger than others. Ex, If I told someone to run the same path as you walked forever, who would end up a farther distance? If I told you to count forever and a computer to keep adding 9 to the screen, who would count higher? If I divided a cookie an infinite times or if you blew up a cookie infinite times, who would have the most amount of cookie pieces? To say that all answers equal infinity, and are thusly equal would be debunkable by common sense.

Graphically, if you have lim x->∞ (read, the limit as x approaches infinity, or basically I am asking if you plug in ∞ for x numerically) of ln(x), you will reach infinity, but just barely. The amount x is increasing by gets so small after a long period of time, that it basically becomes zero after a long period of time. However, if you solve for x in 0=ln(x), you will find that because e^x=∞, then x must equal infinity. So the natural log of x is infinity, but it takes a very long time to get there. On the other hand, if you have the graph 10^x, it would appear graphically that you actually end up reaching infinity extremely fast. Go ahead, pull out your calculator and test it! Both graphs will reach infinity over time, yet we all know which one gets there faster.

This means, in theory, that some infinities can actually be bigger than others! Pretend the variable of time as a plane on a graph. We can assume that t, time, will eventually end up reaching infinity. Once t=∞, we have reached the plane of infinity. Not too hard to conceptualize, but we, for sake of debate, will assume that this plane both exists and is reachable. Ok, so now we apply that frame of time, the plane of infinity, to each graph we have discussed. When y= 1, at the plane of infinity you will still have data saying that y=1. Now, if we plug in infinity at y=ln(x), as well as y=10^x, we notice that both reach the plane of infinity. However by the time that ln(x) has reached this plane, 10^x is so far ahead of ln(x) (both have entered the plane of infinity mind you) that it has likely actually extended so far into the plane of infinity, that it may even be nearing the end of that plane as ln(x) has just broken the surface.

This is where (hopefully) it all ties in. If there really are infinite points in a unit of anything, and some infinities are bigger than others, then what does a generic unit mean? If I say unit circle has a radius of 1, then how much infinity does it contain? Easy. It contains 1 unit of ∞. Once that radius has been traveled an infinite amount of points to get to the edge of the circle, and you walk through the infinitely long barrier between 1 and 1.00000000000000000000000000000000000000000000000000000000000000000000000001, you eventually reach the realm of 2, in which there are another infinite points awaiting you.

By proxy, all mass is infinite, and each person has infinite mass. You may have n amount of liters of total material, but each liter can differ by 1/an infinite amount that your liters of material is only a generalization of what you actually are.

I hope that this has meant something to somebody out there… infinity and the math behind it is hard to think about—let alone explain. I am horrible with words anyway, so if you even made it to the end without either crying or giving up at least once, then I give you my thanks anyway. I hope what I am saying holds some meaning and that it brings people a good deal of thought about the universe and our numerical process.

Infinity is not impossible to reach, it just matters how fast you reach it.

Eh, while I am thinking about it... might as well go ahead and throw this in here...

Limits at Infinity.mp3

reply to post by PhysicsAdept

Let us pretend you have three apples--go ahead, picture it in your mind. These apples may be oddly shaped, but more importantly we are going to consider them to be normal apples. What does normal mean? Well, statistically it means that all three of your apples fall within 3 standard deviations (or 99.7%) of all the apple data you can think of. Their shape, weight, nutritional value, ripeness, age, color, etc. all fall into the category of normal. Normal from what? In statistics we can relate everything to a, more or less, perfect apple. This apple we relate data to is the average-in-every-way apple. It has the exact mean, or average, of every apple that ever existed, and in every category assumed with apples. Now, this specific apple we relate things to may have a mean shape, weight, nutritional value, ripeness, age, color, etc, but to say that an apple with "perfect" everything (or average everything) at one point existed may not necessarily be true. There is a very likely chance that that apple never existed, and may never exist in time (all speculative of course). There may have been apples very close to being perfect, but there may have always been one conflicting variable in which it was slightly above or below average. And of course, as a mathematician, I can say that there may have even been a perfect apple at one point in time, but likely not many more than one, if any.

It's getting late so I'm only going to reply to this part of your post.

1. The normal distribution is not a distribution of apples, it is a distribution of some measurable property of apples, such as their weight or volume.

2. Of course when we speak of the population of apples, we don't really know if any of the measured properties of apples is actually normal. For example, if we are measuring weight, we may have a skewed distribution with all those Macintosh apples pulling our average weight down counterbalancing he larger but fewer delicious apples.

3. However, there is a normal distribution in there somewhere. If we take samples of apples and the plot the mean of each sample, the distributions of sample means will be normal.

4. The last part of the quote tends now to deal more with how people organize categories in the mind. As Eleanor Rosch, the brilliant cognitive psychologist pointed out, when we think of the category "Apple," we represent in terms of a "best example of" or "prototype" or "exemplar" which we use as a measuring stick to determine how apple-ish other things in our environment are. A quick Wikipedia link which has some links to some good work (I particularly recommend George Lakoff's book) can be found here en.wikipedia.org...

What is, just 1? 1 is a number and aside from the units and imprecision in the "real-world", 1 can be dealt with graphically. Graphs, mathematically, are perfect. If you graph "1" in a Cartesian plane without defining parameters, it exists as nothing but a concept. If you define it, say by saying that y=1, then you have a horizontal line. For every x point that you can discover then has a set value of y=1. For that matter, regardless of x, you know that y=1 (for the only y that exists here is 1).

Can't sleep so... This is just wrong. There are two approaches that are interesting in considering numbers. The first, which is a very traditional meta-mathematical approach is that of the Peano Postulates or axioms that define the natural numbers in terms of starting with "0" and having a defined successor function.

But then of course what is 0? There is a set theoretic approach that starts with the idea that a number can be defined in terms of sets. We start with the empty set "e", then take the set consisting of just the empty set [e], then the set of consisting of the two sets we have just defined [e,[e]]. Now we define a unique property to each set so constructed called its cardinality. Then 0 is defined as card(e), 1 is defined as card([e]) and 2 is defined as card([e,[e]]).

No need to resort to graphing or to make any reference to any real world properties or ideas about what a number "is, and you don't need to resort to any geometrical intuition (graphs are perfect? huh?)

reply to post by metamagic

1. The normal distribution is not a distribution of apples, it is a distribution of some measurable property of apples, such as their weight or volume.

Correct, no dispute there. A theoretical graph plotting a multi-variate chart of the apples. You take one property, compare it to the rest of the sample (in the case every apple ever, or to be statistically more professional about 10% of the population id fine) and you receive a z-score for each property. Then you would do some other test comparing all the collected variance away from zero, or residuals, of each trait and give that a z-score of sorts. By doing this, would you not then conceive some notion of how "perfectly normal" some apple may score?

2. Of course when we speak of the population of apples, we don't really know if any of the measured properties of apples is actually normal. For example, if we are measuring weight, we may have a skewed distribution with all those Macintosh apples pulling our average weight down counterbalancing he larger but fewer delicious apples.

Well, ok fine we take a more specific example to explain it, that is beside the point...

Points three and four I see no contradiction, and agree with so I see no need to comment upon them.

reply to post by metamagic

But then of course what is 0? There is a set theoretic approach that starts with the idea that a number can be defined in terms of sets. We start with the empty set "e", then take the set consisting of just the empty set [e], then the set of consisting of the two sets we have just defined [e,[e]]. Now we define a unique property to each set so constructed called its cardinality. Then 0 is defined as card(e), 1 is defined as card([e]) and 2 is defined as card([e,[e]]).

Exactly, what is zero? Zero is some example of nothingness, or emptyness. However, zero is somewhat scalar. I examined s piece of vacuum in space, is that zero? It would be hard to detect, but who is to say that there aren't neutrinos bumbling about in there? Or, what about some mass of energy that very finitely makes up space? Zero does not exist, but in concept.

No need to resort to graphing or to make any reference to any real world properties or ideas about what a number "is, and you don't need to resort to any geometrical intuition (graphs are perfect? huh?)

How are graphs not perfect? They show the exact conceptual idea, and mold it to a scale that you can perceive. The closest you will ever get to a circle that equals pi, is through graphing. Otherwise, it is approximate, but this is aside from the point I was making in the thread. It is not about the numerical premise of a graph, or a unit, of of objects, but the conceptual impossibility that something can be as exact as a number can tell us.

Now, before we continue on graph-theory, I do wish you to keep some things in mind. We are made up of atoms. Each atom is made up of subatomic particles, and perhaps each subatomic particle is made up of other things. Now, atoms make up molecules, molecules make up substances, substances make up objects, objects make up systems of objects, systems of objects make up the world, worlds make up a solar system, a solar system makes up a universe, and blah blah blah. It goes on forever as far as we know—in both directions. This means, that for everything you can think of small scale, there could be some macro version of it somewhere, sometime. So to say this does not apply graphically, would be inaccurate. Still have that circle in mind, with radius 1? That circle has a radius of 1 and therefore a hypothetical area value of π. So an area of π perhaps, yet there seems to be one interesting aspect to this circle. Inside the circle lie an infinite number of points! Each data point has a value of zero, because as you zoom in further and further on a graph you can never actually see a data point, and you never will. There are an infinite number of points, each with an area of zero… yet the addition of all the points brings the circle to an area of π? So 0*∞= π? No, that does not seem to be right…

It is not right at all. You are really confused about the difference between rational and real numbers. The rational numbers are all numbers of the form p/q where p and q are integers and q is not zero. It's a basic exercise for first year students to show that while there are infinitely many rational numbers, they are "countable" which means we can list them -- or in other words, there are as many rational numbers as there are natural numbers (i.e. the natural numbers are the numbers [1,2,3...]. This infinity is represented in math by the Hebrew letter Aleph with a zero suscript (pronounced aleph null).

If we add in the limits of all the sequences of rational numbers to produce what we call the real numbers, we have what we call the real numbers which has an uncountable cardinality (represented by "c" the cardinal of the continuum). The real numbers are much much bigger than the rational numbers and include the transcendental numbers e (natural logarithm base) and pi.

Now in terms of area, your argument only applies if we consider ONLY some countable set of numbers since limits only work over countable sets, we have to use different sorts of things (called filters and nets) to take limits over uncountable sets.

But once we get into infinities, our intuitions about area and length don't work. A fascinating example is the Cantor set, which is constructed by removing the middle third of the line segment [0,1], then removing the middle thirds of the remainder and repeating this process an infinite number of times. Eventually we wind up with an uncountable set that has a total length of 0.

So I'll amend your last statement to read correctly "That just isn't right."

reply to post by metamagic

The real numbers are much much bigger than the rational numbers and include the transcendental numbers e (natural logarithm base) and pi.

Riiiight, again failing to see where this argument leads to describing how I am incorrect... you referencing Euler's formula has nothing, at least that I see, to do with anything as of now...

Now in terms of area, your argument only applies if we consider ONLY some countable set of numbers since limits only work over countable sets, we have to use different sorts of things (called filters and nets) to take limits over uncountable sets.

The number itself is a limit, which is pretty much what I am pointing out throughout the post...

"That just isn't right."

Originally posted by PhysicsAdept
reply to post by metamagic

 

1. The normal distribution is not a distribution of apples, it is a distribution of some measurable property of apples, such as their weight or volume.

Correct, no dispute there. A theoretical graph plotting a multi-variate chart of the apples. You take one property, compare it to the rest of the sample (in the case every apple ever, or to be statistically more professional about 10% of the population id fine) and you receive a z-score for each property. Then you would do some other test comparing all the collected variance away from zero, or residuals, of each trait and give that a z-score of sorts. By doing this, would you not then conceive some notion of how "perfectly normal" some apple may score

A "theoretical graph?" If we are talking statistics here, then a distribution is a distribution of measurements or values so I'm not sure what a "theoretical graph" would be. Nor would it make any difference how many variable we plot. However I'll calling the rest of this nonsense for the following reasons.

1. Sample sizes cannot be spoken of in terms of percentages of a population, only in terms of their size. And we assume we are measuring the actual population parameters -- instead we infer from out samples by making statistical hypothesis with measurable confidence intervals. The larger the sample sizes, the better our confidence that the sample statistic is close to a postulated population parameter, but then this of course leads us to problems with trying to define exactly what the underlying population is. The definition "every apple ever" is NOT a good population definition. Apples produced in Maine in the 2011 growing season.. that would be better.

2. The stuff about z-scores and variance measures is gibberish. A z score is used to transform an arbitrary NORMAL distribution to a standard normal distribution mean mean=1 and s.d. = 1.

2. Of course when we speak of the population of apples, we don't really know if any of the measured properties of apples is actually normal. For example, if we are measuring weight, we may have a skewed distribution with all those Macintosh apples pulling our average weight down counterbalancing he larger but fewer delicious apples.

Well, ok fine we take a more specific example to explain it, that is beside the point...

It's not beside the point, it IS your point. You are assuming a normal distribution.

reply to post by PhysicsAdept


I disagree with all three of your points

1. Nothing is exact, it is impossible to determine the actual quantity of a real object, as a quantity is only expressed as a unit which is a generalization.

A unit is not a generalization...it is a unit.

You apple example is just flat out wrong...because no one says I have 3 perfect apples...they just have 3 apples. The "normalcy" of the apple doesn't matter...all that matters is that it fits the minimum definition of an "apple"...which is not mathematical at all.

Same thing if you are counting cars, or clouds, or basketballs.

A better example for you to have used is units of measurment...because there is no exact "inch" or "centimeter". So you could argue that something is never exactly an "inch" long...but you can't say you can never have exactly 3 apples. You could say you can never have 3 "perfect apples" as defined by size, color, smoothness, luster, taste, nutritional value, etc. But just using the generic unit of "apple" has not other mathematical criteria rather than the fruit you are counting to be defined as an "apple".

2. I ask you to broaden your mind, and think about how the related rates of the world and the interaction between all the numbers you can think of what they really mean. Consider the fact that between each integer lies an infinite possible amount of numbers, and that each decimal has some decimal slightly larger than it as well as some decimal slightly less than it.
...
Still have that circle in mind, with radius 1? That circle has a radius of 1 and therefore a hypothetical area value of π. So an area of π perhaps, yet there seems to be one interesting aspect to this circle. Inside the circle lie an infinite number of points! Each data point has a value of zero, because as you zoom in further and further on a graph you can never actually see a data point, and you never will. There are an infinite number of points, each with an area of zero… yet the addition of all the points brings the circle to an area of π? So 0*∞= π? No, that does not seem to be right…
...
Ok, so now where did we leave off… ah yes, an infinite amount of zero can actually equal some number. Yet, different infinite amounts of zero will lead us to different numbers each time.

Each data point does not have an area of zero. It has an area that approaches zero...but it is never zero. It can have an area of 1 if you use some imaginary unit of measure that signifies a very very small area. Or it can have an area of 10^-1000000000000000000000 mm^2. But it will never ever have an area of zero.

If you divide into infinite points, than the area of each one could be described as the inverse of infinity...or you can say it is infinitely small...but not zero.

3. Infinity itself is a quantization. It is an assumption that we make, and the definition can be treated similarly to the rest of the numerical symbols that have been created, and thusly makes some infinities larger than others.

Infinity is a concept.

The "infinity" between 1 and 2 is the same as the "infinity" between 1 and infinity. That is why infinity is a concept and not a number.

When you start saying one is larger than the other, you are changing the definition.




Infinity is a concept that is fun to fantasize about...it is also very easy to confuse yourself about...but in reality it is just a concept. Once you start thinking about it as a number like you have...it is even easier to confuse yourself.

Originally posted by PhysicsAdept
reply to post by metamagic

 

But then of course what is 0? There is a set theoretic approach that starts with the idea that a number can be defined in terms of sets. We start with the empty set "e", then take the set consisting of just the empty set [e], then the set of consisting of the two sets we have just defined [e,[e]]. Now we define a unique property to each set so constructed called its cardinality. Then 0 is defined as card(e), 1 is defined as card([e]) and 2 is defined as card([e,[e]]).

Exactly, what is zero? Zero is some example of nothingness, or emptyness. However, zero is somewhat scalar. I examined s piece of vacuum in space, is that zero? It would be hard to detect, but who is to say that there aren't neutrinos bumbling about in there? Or, what about some mass of energy that very finitely makes up space? Zero does not exist, but in concept.

You are confusing uses of zero to describe or measure something with the definition of zero. The way zero is defined in mathematics is independent of any "meaning" of zero. If you measure something you are quite free to assign it the value 0, or the value pink. Zero is somewhat scalar? Jumping around again, scalars and vectors have nothing to do with zero, although there we can define zero elements in what we call scalar fields, but that also implies zero vectors too.

No need to resort to graphing or to make any reference to any real world properties or ideas about what a number "is, and you don't need to resort to any geometrical intuition (graphs are perfect? huh?)

How are graphs not perfect? They show the exact conceptual idea, and mold it to a scale that you can perceive. The closest you will ever get to a circle that equals pi, is through graphing. Otherwise, it is approximate, but this is aside from the point I was making in the thread. It is not about the numerical premise of a graph, or a unit, of of objects, but the conceptual impossibility that something can be as exact as a number can tell us.

What do you mean by perfect? In fact you seem to be saying that graphs are close approximations ("the closest you will ever get to a circle that equals pi" -- although it makes no sense to say that a circle equals pi). I would argue that graphs are horrible imperfect. A line has no width yet any depiction of a line has width.. imperfect by my definition.

Originally posted by PhysicsAdept
reply to post by metamagic

 

The real numbers are much much bigger than the rational numbers and include the transcendental numbers e (natural logarithm base) and pi.

Riiiight, again failing to see where this argument leads to describing how I am incorrect... you referencing Euler's formula has nothing, at least that I see, to do with anything as of now...

No reference to Euler's formula at all. But my argument is about the flaw in your reasoning, you clearly do not understand the mathematical definitions of infinity, area, number and limit, not to mention basic statistics, so that your arguments are invalid, not wrong but invalid. Sort of like asking a marine biologist to make a superbowl prediction for the Miami Dolphins.

Now in terms of area, your argument only applies if we consider ONLY some countable set of numbers since limits only work over countable sets, we have to use different sorts of things (called filters and nets) to take limits over uncountable sets.

The number itself is a limit, which is pretty much what I am pointing out throughout the post...

Which is pretty much a total misunderstanding of what a limit and number are. My suggestion is that if you want to publicly use mathematical terms and concepts, take the time to learn what they are. It's actually very interesting.

reply to post by PhysicsAdept

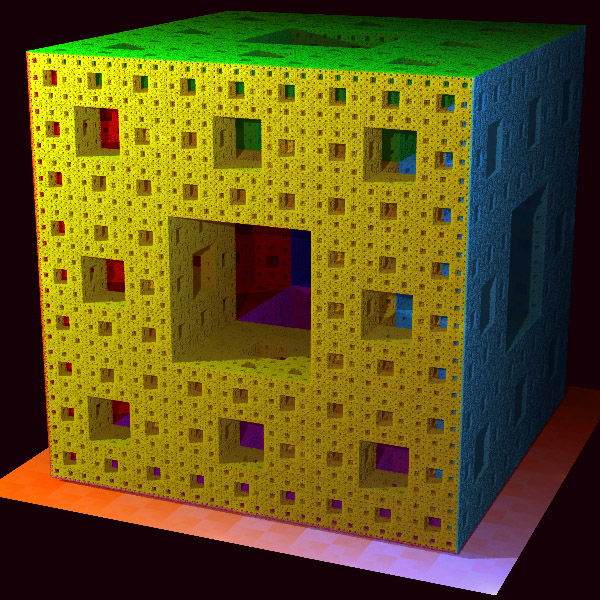
reply to post by Americanist


Lovely, it looks like a model of a three dimensional cantor set. Brilliant.

Originally posted by PhysicsAdept
I post this due to recent contemplations I have been considering. This is a long thread that I hope you read, but if you really don’t want to then skip to the bottom, the last line, before you decide not to give it a chance. Honestly, I really think there needs to be a new "math-topics" forum, but anyway...

We shall examine a few simple (real) numbers and what they really represent in this thread.
There are 3 main points in this thread (which I will try very hard to relate at the end, despite my inability to communicate my ideas fluidly):

1. Nothing is exact, it is impossible to determine the actual quantity of a real object, as a quantity is only expressed as a unit which is a generalization.

2. I ask you to broaden your mind, and think about how the related rates of the world and the interaction between all the numbers you can think of what they really mean. Consider the fact that between each integer lies an infinite possible amount of numbers, and that each decimal has some decimal slightly larger than it as well as some decimal slightly less than it.

3. Infinity itself is a quantization. It is an assumption that we make, and the definition can be treated similarly to the rest of the numerical symbols that have been created, and thusly makes some infinities larger than others.

edit on 16-3-2012 by PhysicsAdept because: (no reason given)

Good job ive always found it interesting that if you plot on a one dimensional x line graph with 3 points
The first and center one being x point = ( 0 ) the left of it being x point ( -1 ) and the right of center ( +1 ) its infinite in both directions away from 0 and the two 1s
but it is just as infinite as 0 or the space between -1 and +1 tell me

its like if you ask yourself how something can be infinitely close yet infinitely far?
theirs a good answer for that since it is infinite way that it can,

saying it cant means only that it can not and that if true means nothing at all to that which can

Ask yourselves this simple question which way can you travel that is not up
because from where im sitting every dircetion forward backward left right and down is even up if you think about it so

what is not up and that is what is within vs. what is without
-Ex nihilo nihil fit-

i like to think of it in terms of me myself and I cause its the only thing not beyond me or I
+1 is a true statement
-1 is a false statement
0 is a meaningless statement

Every person is their own world that just happen to exist on this world with many other worlds

We are all the definition of 3 point
since to triangulate something you need 3 point
beyond i hold one believe and it is that
"All statements are true in some sense, false in some sense, and meaningless in some sense." all you need is between -1 and +1 everything else is beyond 1 and ultimately meaningless