"Vortex Based Mathematics by Marko Rodin"

Originally posted by Mary Rose
reply to post by Arbitrageur

 

Do you have a resource recommendation?

And do you have a comment about regularities in the decimal number system? Rodin said his math has no anomalies. Is that a reference to regularities in the decimal number system?

You might enjoy reading Richard Buckminster "Bucky" Fuller's writing on Numerology. (You may have heard of "buckyballs" named after him). He liked looking for patterns. Here is page 1 of his writing:

www.rwgrayprojects.com...

Numerologists do not pretend to be scientific.
They are just fascinated
With correspondence of their key digits
With various happenstances of existence.
They have great fun
Identifying events and things
And assuming significant insights
Which from time to time
Seem well justified,
But what games numerologists
Chose to play with these tools
May or may not have been significant.
Possibly by coincidence, however,
And possibly because of number integrity itself
Some of the integer intergrating results
Are found to correspond elegantly
With experimentally proven, physical laws
And have subsequently proven to be
Infinitely reliable.
Half a century ago I became interested in seeing
How numerologists played their games.
I found myself increasingly intrigued
And continually integrating digits.

So he says it's not scientific, and in fact some of the things he writes about seem kind of wacky to me, but you'll probably like it for that reason? But I find a lot of the patterns he wrote about interesting. You have to click the link at the bottom of each page to get to the next one, and there's no link to the previous page so I use the back arrow on my browser to go back.

He covers modulo math, casting out 9s, which we've talked about here, and lots of other patterns.

I have no idea what Rodin meant by "no anomalies", but since he seemed to not know what he was talking about with so many other things where we can prove he's flat wrong, I wouldn't apply any special significance to his statement about "no anomalies". It may be reasonable to presume he has no idea what he's talking about with regard to that as well.

reply to post by Arbitrageur


Perhaps you misunderstood my question.

In your response to my request for resources to read up on numerical patterns, you recommended that I study patterns in other numbering systems in addition to the base 10 numbering system.

Do you have a recommendation for numbering systems in general, which will give me the perspective that you suggested?

Secondly, do you understand what Blake said here:

Mr. Rodin has discovered a series of regularities in the decimal number system heretofore undocumented in mathematics.

Specifically, the expression "regularities" in the decimal number system?

Originally posted by Mary Rose

Mr. Rodin has discovered a series of regularities in the decimal number system heretofore undocumented in mathematics.

Specifically, the expression "regularities" in the decimal number system?

Mary, the digitized sound of my flatulence contains a sequence of numbers heretofore undocumented in mathematics. With that sequence, I can travel to any corner of the Galaxy if I push hard. I just hope nobody is around. It's the fingerprint of God.

You can try to google "casting out 7 in octal", or in base 8, and try a few variations of the query. If you write down a column of numbers in octal notation and cast out 7, you'll come up with a sequence as well, except it will be different from Rodin. But why stop here, we can use other base notation. There is nothing special about 9. And Rodin is perfectly aware of that, so he's compelled to perpetrate a myth on you, which is that the number 10 was given to us by divine powers. What's really amazing here is that some people are willing to make an effort to be dumb enough to even believe this sort of crap. Some fool comes around and tells you that number 21 was given to us by ancient gods. Duh.

Originally posted by buddhasystem
Mary, the digitized sound of my flatulence contains a sequence of numbers heretofore undocumented in mathematics . . .

Wow. You are a resident math expert on ATS responding to my query regarding Russell Blake's statement?

God help us.

Originally posted by Mary Rose
Perhaps you misunderstood my question.

In your response to my request for resources to read up on numerical patterns, you recommended that I study patterns in other numbering systems in addition to the base 10 numbering system.

Do you have a recommendation for numbering systems in general, which will give me the perspective that you suggested?

I started looking for something appropriate for you, but haven't found anything yet. The sources I used weren't purely mathematical, but involved computer hardware and software design which encompassed binary, octal, and hexadecimal. Since computers work or worked in those bases, I had to learn them to learn computers but if you used my sources you'd probably get way too much computer stuff you don't want.

Secondly, do you understand what Blake said here:

Mr. Rodin has discovered a series of regularities in the decimal number system heretofore undocumented in mathematics.

Specifically, the expression "regularities" in the decimal number system?

My understanding is very much like milkyway12's post so I'll quote that here:

Originally posted by milkyway12
116256479883743529, i am still trying to figure out how he got this sequence ....... it makes no sense, but here is his explanation.

And the 9 demonstrates the omni dimension which is the higher dimensional flux emanation called Spirit that always occurs within the center of the magnetic field lines. The last number left to be explained from The MATHEMATICAL FINGERPRINT OF GOD is the number 9. The number nine is Energy being manifested in a single moment event of occurrence in our physical world of creation. It is unique because it is the focal center by being the only number identifying with the vertical upright axis. It is the singularity or the Primal Point of Unity. The number nine never changes and is linear. For example all multiples of 9 equal 9. 9x1=9, 9x2=18, but 1+8=9, 9x3=27, but 2+7=9. This is because it is emanating in a straight line from the center of mass out of the nucleus of every atom, and from out of the singularity of a black hole. It is complete, revealing perfection, and has no parity because it always equals itself. The number nine is the missing particle in the universe known as Dark Matter.

Wow! He really surprised with just how much .... he um, well, what was he saying again, and how did this explain sequence / pattern he came up with? I dont see it.

That's pretty much how I see it too, and to avoid re-quoting the entire long post, note milkyway12 points out that the supposed discoveries by Rodin, actually were not first discovered by him, unless you count opening a book and reading what someone else wrote and then proclaiming "look what I discovered written here!" because yeah, it was pretty much that kind of a discovery, which as you can see involved his distortion of modulo math and casting out nines, though I still haven't figured out how he used that to arrive at 116256479883743529.

However you may want to consider following the lead of Aloysius the Gaul and e-mail Blake to ask him what he meant since I'm sure nobody could explain what he meant better than he can.

reply to post by Arbitrageur

1. Russell Blake is an expert and he said what he said in 2001. What I am doing is taking his statements at face value and investigating them.
   
2. Milkyway12's post and your comment have nothing to do with Mr. Blake's use of the term "regularities" in the decimal number system heretofore undocumented in mathematics.
   

Normally I would have thought of emailing Mr. Blake myself, but since it's clear he's bad-mouthing Rodin at this point in time, it would be stupid to expect a straight answer out of him. Be reasonable.

~~~~~~~~~
Damn. As I recall 547000 described himself as a "math nerd," but I see he hasn't posted since March.

Originally posted by Mary Rose

1. Russell Blake is an expert

Expert in what?

Just because somebody says there's a pot of gold at the end of the rainbow, doesn't mean there is one, and the pursuit of an explanation of Blakes comment I suspect will be as fruitless as a search for the pot of gold.

Originally posted by Mary Rose
Damn. As I recall 547000 described himself as a "math nerd," but I see he hasn't posted since March.

But we just had a new poster in this thread, the MilkyWay, who has the right kind of knowledge to form a judgement. I'm sorry that this judgement was utterly unfavorable with regards to Rodin and his "math". Unless the phrase "grade A moron" is some new form of compliment.

reply to post by Arbitrageur


He has the resume of an expert in math-related technologies.

Are you playing dumb??

Originally posted by Mary Rose
He has the resume of an expert in math-related technologies.

Are you playing dumb??

I don't read his resume that way. He may also claim to be a theoretical physicist now that he's created a "theory of everything", but I wouldn't call him that, at least not until his hypothesis is proven (which probably won't happen).

His expertise was computer software, not math. Sure there's some overlap, but I wouldn't call him an expert in math. And no I'm not playing dumb, I've known programmers and mathematicians and personally know something of both fields. Though I don't claim to be an "expert" in either field, I do know enough to know they are different fields.

reply to post by Arbitrageur


You're splitting hairs.

You considered yourself an expert when you answered my post.

This is getting tiresome.



I'm researching general math knowledge as related to the statements in question.

No matter. I'll find the answers.

Thanks anyway.

reply to post by Mary Rose

I would probably consider myself an expert in math if I had a PhD in math, but I don't, nor were you likely to get a response in this thread from anybody with such qualifications, so I went ahead and answered. Like milkyway12, I've studied lots of math, but maybe not as much as buddhasystem.

My math studies have been focused on applications on physics and engineering, but not on the more pure types of math like number theory, which a math expert would know more about than me.

However for the purposes of taking Rodin's (or Blake's) claims and trying to engineer them into anything useful, I suppose I might be considered an expert for that limited scope of math, which is relevant to the topic of this thread.

reply to post by Arbitrageur


What I meant, when I asked the question on the thread, is someone knowledgeable who has an aptitude for math, who could answer the question I asked, in relation to the Blake quotes that I've posted.

This discussion has gotten way out of hand.

Nevermind.

Originally posted by Arbitrageur
Naudin's graph doesn't show zero input power, does it? You don't need to be an EE to see that.

I think the reference to zero is not input power. It sounds like it's a reference to Bishop's equation, where Bishop used an incorrect math function.

Originally posted by Mary Rose

This is from a 40 page .pdf file entitled "Analysis of the Rodin Coil and Its Applications (2001) by Russ Blake (PDF)" which can be clicked on at the Vortex Mathematics Gateway Portal:

ENDORSEMENTS and PAPERS

1. RUSSELL P. BLAKE

Wed, 14 Nov 2001 22:16:11
Subject: The Rodin Coil

To Whom It May Concern:

. . . It became clear to me that Mr. Rodin's work was a synthesis of numerical patterns which had previously been overlooked by conventional science and mathematics. . . .

The following must be what Blake was talking about.

This is from Page 10 of 132, "Notes on Vortex Based Mathematics":

. . . It is important to keep in mind that when we refer to the toroid, the term " Doubling Circuit" includes both of the positive (1-2-4-8-7-5) and negative (5-7-8-4-2-1) sequences that we sandwiched around the 6-9-3-3-9-6 (also called The Gap Space or Equipotential Major Groove) to form the grid that makes up the surface of it.

These three lines of numbers wrap around the top of the torus, go in through the middle, curve around the side at a slight inclinaton [sic] to make an s-curve, and then come up around the other end from the bottom to meet themselves head-on, making a yin-yang-like shape. . .

Originally posted by Mary Rose

. . . It is important to keep in mind that when we refer to the toroid, the term " Doubling Circuit" includes both of the positive (1-2-4-8-7-5) and negative (5-7-8-4-2-1) sequences that we sandwiched around the 6-9-3-3-9-6 (also called The Gap Space or Equipotential Major Groove) to form the grid that makes up the surface of it.

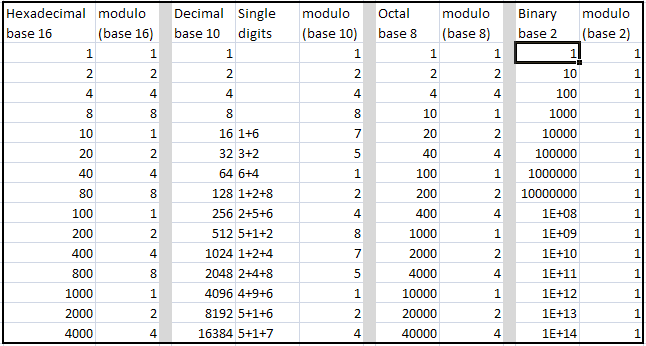
I didn't find anything suitable for you to read on other base numbering systems. But I made this table for you using Rodin's perverted modulo arithmetic, to show how much more elegant the doubling looks in the bases that engineers have used to make computers work (this is no accident; there are reasons engineers selected these particular bases, which is partly because of the elegant way they deal with doubling). Note I didn't even need the digits column in the other bases, because all the other digits are zero, so the "Rodin-style modulo computation" is trivial. Compare and contrast the patterns:


The decimal, base 10 is the most chaotic, and computers don't use that internally for that reason because the function of computers has some basis in doubling. So from an engineer's perspective, these other bases are more likely to be mathematical gifts from God than decimal, which probably just happened because we have 2 thumbs in addition to our 8 fingers, though at least one tribe did use base 8 for their numbering system.

Here's a little reading snippet on how all the colors you can see on your screen are represented by six hexadecimal digits:

Hexadecimal Numbers Guide

Hexadecimal numbers are a compact way of representing large numbers. They are useful in computer programming because computers use bytes as their main unit of information. A byte can represent an alphanumeric character or one of 256 decimal numbers. It normally requires 8 binary digits to represent a byte but in hexadecimal only two are required.

In the RGB Color System used in computers there are 256 possible levels of brightness for each component in an RGB (Red, Green, Blue) color. So the red component, blue component and the green component can each be represented by a byte, or two hexadecimal digits. An entire RGB color can therefore be represented by six hexadecimal digits.

So not only is it more mathematically elegant on doubling, but hexadecimal has real-world uses, which is more than I can say for Rodin's 1-2-4-8-7-5 pattern in decimal. I don't agree with everything Blake says, but I agree that scientists and mathematicians have not placed any special significance on this 1-2-4-8-7-5 pattern. Is there any reason they should? If so, I haven't seen any reason yet, other than a nonsensical proclamation by Rodin, which is not really a reason.

Originally posted by Arbitrageur

Would it be too much trouble for you to re-size your image to 575 pixels wide and re-upload so that there will be no scroll bar? (It will help me if I can print the image and make notes on it.)

Originally posted by Mary Rose
Would it be too much trouble for you to re-size your image to 575 pixels wide and re-upload so that there will be no scroll bar? (It will help me if I can print the image and make notes on it.)

I found a typo in the previous table anyway, in the last two entries of the decimal digits column which were apparently auto-filled by excel that I didn't overwrite, so I re-uploaded the table with that correction and clarified headings, and resized it using bbcode. How's that?

reply to post by Arbitrageur


Thanks.

reply to post by Arbitrageur


Compression via yanking 0's... Quite a comparison!

First question, what/ where/ and how does mapping vectors work in your view? Second, would the other systems display a lattice structure of any kind? Last but not least... Where is Zero-Point found?