Adventures In PRIME NUMBER Land !!! ... (A Dummies Guide to Prime Numbers)

Originally posted by mathfreak
Finding all the squares of primes (except 4 and 9) on line 1 is really cool, something I didn't know, and I salute you for it, but there's a reason for this, too. Modular arithmetic says we only need to check the squares of the first circle. If they all lie on line 1, then all possible prime squares will lie on line 1.

It's pretty easy to see why. Consider "p^2 - 1", where "p" is prime. The value will always be an even even multiple of 24. This is because "p^2 - 1" can be factored as "(p-1) * (p+1)".

Consider any sequence of three contiguous integers: "(x-1), x, (x+1)".

Two of them must be divisible by two (even numbers). One of them must be divisible by three, and one of them must be divisible by four. In the case of a prime number "p", which of course is not divisible by anything, then we can say: one of (p-1) or (p+1) is divisible by four, the other by two. Additionally, one of them is divisible by three.

Thus, "p^2 - 1" always has the factors 2, 4, and 3, and is divisible by 2*4*3 = 24. That's why it's always on the same radial line.

Originally posted by stander
If the elimination of non-prime numbers the way it was presented in its essence cannot find primes, would you be able to demonstrate the method which does the job in its numerical, not abstract/generalized form?

The OP mentioned all the arguments that you presented and concluded with prime factorization, the applicable part of primes. He just used a long lemma to get there as an intro for those who are not overly familiar with the subject.

[edit on 9/22/2009 by stander]

@stander, the problem is that this method doesn't eliminate all of the composite numbers, only some of them. And if I knew how to do this, I wouldn't post it here I'd publish it or use it to break public key crypto systems and become rich.

However, with that said, I have done some interesting work with prime number patterns. First hint: note that the digits of prime numbers are not uniformly distributed, that is, they do not occur with equal probability.

OP; Very interesting stuff, kept me out of the Pub this evening.

This thread caught my attention, so I delved in without much in the way of research I'm afraid.

Using my MS based PC and Excel I wrote some simple 'macros' and graphed the results:

For 1120001- 1130000 I find 718 primes
For 2120001- 2130000 I find 697 primes

For 12120001-12130000 I find 604 primes
For 22120001-22130000 I find 596 primes
For 32120001-32130000 I find 565 primes
For 42120001-42130000 I find 560 primes

For 142120001-142130000 I find 539 primes
For 242120001-242130000 I find 523 primes

For 1242120001-1242130000 I find 488 primes
...if I go above the 'Long' positive in Excel I get an error, so my probing ends there.

Looking at these numbers would imply that rather than 'infinate', that there is an ultimate prime number. The number of primes, -for my 10,000 selections-, decreases as the value increases. That would imply to me that there was an eventual destination?

Does the number of primes decrease as the value grows?

Is there a log or log-log graph available for view that records this as it runs beyond my capability?

The chance of a random integer n being prime is about 1/ln(n).
primes.utm.edu...

No progress then.

mmm... I'm sure I saw something that rang a bell in another post.

It was someting about 'A heartbroken father shows us how to protest' I think it was father of Lance Corporal Shaun Brierley, murdered for MONEY.

Originally posted by tauristercus
DIAGRAM1:
[atsimg]http://files.abovetopsecret.com/images/member/09a76a65da3c.jpg[/atsimg]

During my studies of different aspects of the universe and math, I came across this image:

I don't remember where I found this image, but I did save it on my hard drive.

I believe people are already aware of the prime pattern.

reply to post by ALLis0NE


These charts are great because they demonstrate clearly that center of everything is nothing.

"Nothing" is the true balance, the center, the total coherence, of the entire universe. The center of a vortex or even a spiral, as you can approach it infinitely, is a void you can never absolutely reach.

reply to post by bsbray11


Well from what I have read from other very knowledgeable people, and from what I concluded on my own, in the center of everything is GOD, not "nothing".

[edit on 30-10-2009 by ALLis0NE]

Originally posted by ALLis0NE
reply to post by bsbray11

 

Well from what I have read from other very knowledgeable people, and from what I concluded on my own, in the center of everything is GOD, not "nothing"

In my experience there is not much difference, if any.

The un-manifest is the ruler of the manifest.

Whether your idea is new or not, or can be explained by modular arithmetic or not, I find it a fascinating topic and more fascinating you found this out by yourself! So S&F from me for this great thread and your good explanations!

I'm making an Excel sheet now, putting it in a 24-column table instead of a circle, which basically works the same. Let's see if we can come up with a method to calculate the difference in row or circle position. Useful or not, it's a very interesting challenge that might shake the modern cryptographic cradle on it's foundation.

Keep up the great work!

reply to post by tauristercus


Dear Tauristercus,
I've been following your adventures in prime number land and have been quite fascinated by your work. You may have seen this on the front page of ATS recently:
www.abovetopsecret.com...
Perhaps when the numbers are arranged in the "24" array you've discovered have some relevance to the Fibonacci Sequence. I'm not a trained mathematician and certainly not as qualified as you, so I could just be a fool. Anyways, good luck in your research.
Best

reply to post by ALLis0NE


The center of your image is part of Euler's Formula. It's a famous formula and I have see no purpose to putting it inside of that shape. I believe it is a misuse.

Euler's Formula @ wikipedia

The base 24 only "seems" appealing because the numbers are small. The reason you don't have primes on several axes is because of the definition of a prime. Of course nothing, divisible by 2, or 3, or 5, etc, will have a prime number, which covers several of the axes of the base 24 graph. That isn't special.

The number spiral is more intriguing and was posted on the first page...

The Number Spiral (again)

If you want to see some crazy geometry, look at this:
Garrett Lisi on his theory of everything (TED)

[edit on 24-5-2010 by Professor Chaos]

DIAGRAM1:
[atsimg]http://files.abovetopsecret.com/images/member/09a76a65da3c.jpg[/atsimg]




Not sure how to quote properly


I have one question regarding the above image and the others like it in your thread further down.

Why have you disregarded 2 and 3 as prime numbers?

At the top of the page you had them included in the list but not number 1, however when you arrived at the diagram above you included 1 but not 2 and 3.



[edit on 24-5-2010 by Professor Chaos]

I am sorry to disappoint you but you idea is not really new. It's basically the same principle Gauß used in the 19th century for his clock-calculator. So while it is still useful it is just something very basic.
The current way mathematicians think we will understand prime numbers some day is Riemann's Conjure. It's implications are really mind boggling. Some arrive at numbers so big that they would have more digits than there are atoms in the known universe.

You can read all about it in the Book "Music of the Primes" from Marcus du Sautoy.
(The title is a bit misleading it's really mainly about Riehmann's work.)


I personally belive that the secret of primes is not just about the prime numbers themselves but also about all other natural numbers. Euler has done some pretty amazing stuff in inventing a formular which can derive somthing like a 'prime equivalent' for every number. While this formula can be used to detemine the sonic quality of rational musical intervals its result yield the number itself if it is prime and a lower number if it is not. Here are the results for numbers 1 to 24
1 1
2 2
3 3
4 3
5 5
6 4
7 7
8 4
9 5
10 6
11 11
12 5
13 13
14 8
15 7
16 5
17 17
18 6
19 19
20 7
21 9
22 12
23 23
24 6

You can lookup the "gradus suavitatis" funtion if you are interested. The really interesting thing would be calculating it the other way around, alias calculating the all the numbers which would result in a particular gradus value. The problem here is that there is no programming language I know of which can handle multidimensional arrays with variable magnitude. Without it the memory requirement would be much too high for practical applications.

originally posted by: tauristercus
Continued from previous post ...


PAGE 2


Ok, the 1st thing I need to state and absolutely crucial to everything that subsequently follows in this thread, is that
I believe the ENTIRE prime number system is BASED ON and COMPLETELY revolves around the number 24.

As they say, a picture is worth a thousand words.

To illustrate my statement above, lets create a number of concentric circles and divide each of them up into 24 segments. You can picture the 1st circle as a clock with 24 hours ... starting from 1 and going around to 24.
The next outer circle can be pictured as a clock starting at 25 and going around for another 24 segments to finish at 48.
The third outer circle can be pictured as a clock starting at 49 and going around for another 24 segments to finish at 72.

So what we end up with is an infinite number of concentric circles, each divided up into 24 segments:
Circle 1 ---> 24 segments labeled 1 to 24
Circle 2 ---> 24 segments labeled 25 to 48
Circle 3 ---> 24 segments labeled 49 to 72
Circle 4 ---> 24 segments labeled 73 to 96
and so on for infinity.

Notice that starting position 25 (on circle 2), is directly above starting position 1 (on circle 1) ... and that starting position 49 (on circle 3) is directly above starting position 25 (on circle 2), etc

Much easier to show then to explain !

DIAGRAM1:
[atsimg]http://files.abovetopsecret.com/images/member/09a76a65da3c.jpg[/atsimg]

So, in the above diagram, what I've essentially done is started counting numbers starting from 1 all the way to number 24, and put these numbers on the 1st circle ... I've then continued counting for another 24 numbers (i.e. numbers 25 to 48) and put them on circle 2 ... I've then continued counting for another 24 numbers (i.e. numbers 49 to 72) and put them on circle 3 ... and so on.

Right here in your diagram is all you need to see what you did!

Ray 1 = Ray 7 = Ray 13 = Ray 19 = Ray 25 (full circle)
Ray 5 = Ray 11 = Ray 17 = Ray 23 = Ray 29 (full circle)

Notice how they are all perpendicular to each other? That is because they are separated by six only you did this four times.

Another way of stating this is take any positive number n and divide by "6"

n / 6 is going to have remainders r = [o,1,2,3,4,5].
Any even number will have a remainder of [o, 2, 4]
Any odd number will have a remainder of [1, 3, 5]
When r = 3 you have an odd multiple of 3 meaning it is not prime.

That puts us back to two rays needed to describe all odd numbers: Ray 1, Ray 5.

The formulas are: 6x + 1, 6x + 5 for x ∈ [o,1,2,3,…]
or
6x +/- 1 (x > 0)
Which is well known mathematical reality. Although nobody is sure why all numbers can be divided by six (Goldbach conjecture basically).

Although I really do like the "iron cross of prime numbers"! And the slightly mystical approach to the topic. There does seem to be an underlying structure to the primes. Mathematicians are working on the boundaries of where primes reside (especially super large primes) and I hope somebody fooling around with Iron Crosses shows them that diff eq. is not needed!

This is one of my all time favorite threads on ATS!

The hidden symmetry in the primes is shown by the Riemann Zeta Function. (1/n^s) Add together values where n = 1, 2, 3... to infinity, and "s" is complex values.

This produces something like Fourier waves (but more complex) that when added together produce a series of wave peaks and troughs. When lined up with the real number line all of the spikes show where primes are located. The more individual values you add together the more accurate the function becomes.

It's a complicated subject with lot's of angles to explore but that is the basic idea.

The function also produces zeros called "non-trivial zeroes" that all line up on real number 1/2.

The Riemann hypothesis is that all the non-trivial zeroes are on that line. We only know that the first billion or so are on the line.
If a proof can be made it will enable us to do all kinds of cool things with the equations.

a reply to: tauristercus


I did notice that all the rays with the primes on them were uneven numbered rays. That being said the primes on each of the rays, might each have a repeating series peculiar to that ray.

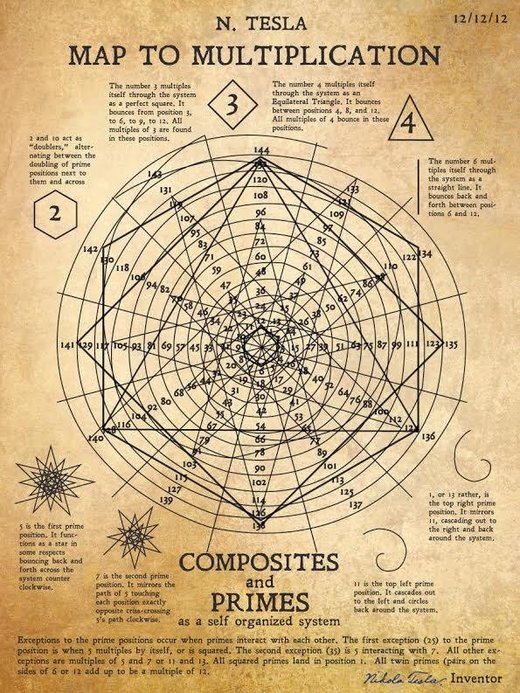
The diagram itself is very intuitive, allowing students to see how numbers all work together based on a spiral with 12 positions. 12, or 12x (multiples of 12) is the most highly composite system, which is why we have 12 months in a year, 12 inches in a foot, 24 hours in a day, etc. 12 can be divided by 2, 3, 4, and 6. So can all multiples of 12. For every 12 numbers there is a chance of 4 numbers being prime. They happen to fall in positions (think clock positions) 5, 7, 11, and 1.

...It turns out that when the device is examined, the digital roots of the numbers in positions 3, 6, 9, and 12 constantly repeat the same sequence 3, 6, 9!

Macedoniaonline.eu - Long-lost Nikola Tesla drawings reveal Map to Multiplication.

I believe that OP is onto something!

A little explanation. Think of an analog watch face. You place the digit "1" at the one o'clock position, digit "2" at the two o'clock position, all the way around to "12" at the "noon" position. Next comes "13" on the next ring out at the one o'clock position, etc. Each ring increases by 12 radiating outward.

At 1, 5, 7, and 11 o'clock on each ensuing ring (addition of 12) is the only place you will find prime numbers (not all are prime, but you will not find any prime at say, 8 o'clock position). Tesla only went to 12 before the over lap while OP went twice around.

At 1 o'clock, you will encounter the squares of all the primes! 25 (5x5), 49 (7x7), 121 (11x11), 169 (13x13), etc.

The notes around spiral explain a bit about how numbers double, twin primes, where certain composite numbers lie (a number that is a multiple of two smaller numbers.

I am not sure what all the lines mean! The triangles, the pentagon, the pentacle, etc. but they seem to be growing in size as you move outward.

Awesome!

a reply to: tauristercus

What an awesome thread to read, fascinating topic, this is the stuff that makes me want to come back to ATS! It's easy to read and understand, yet in-depth. Incredibly interesting and thought provoking stuff, thanks. There's something magical and mysterious about numbers and the role they play within the universe. IMO numbers and mathmatics are key in unlocking and further understanding our existence here in the universe.

Multiple stars & a flag, my hat's off to you!

By the way, not sure if the OP is still active here, but would love to know if there have been any updates in the meantime.