It looks like you're using an Ad Blocker.
Please white-list or disable AboveTopSecret.com in your ad-blocking tool.
Thank you.
Some features of ATS will be disabled while you continue to use an ad-blocker.
share:
Let me try one of these threads. I'm kinda a noob, but we'll see how it goes.
An article titled, "Scientists Discover 'Pure Math' Is Written Into Evolutionary Genetics" came through my Newsfeed.
www.sciencealert.com...
It seems black and white were all we saw in our infancy and the ratio may be golden, after all. They found a Fibonacci sequence in our genome.
The meaning of life the universe and everything may be a number thing afterall.
I swear I was just in thread on something almost similar...
They then looked into protein folding and RNA to see how genotype maps to a specific trait.
Now I can make commentary. Their words are better anyway.
This implications are that perhaps everything in the universe really is unified under a ratio in which to exist.
The Fibonacci sequence isn't just some solipsistic mathematical precept that only exists in observation, it existed before our planet had pawn scum and went into our very construction.
Thanks science alert, your pushed me a little further to the agnostic today... sorta
And I can't title it that without the song...
An article titled, "Scientists Discover 'Pure Math' Is Written Into Evolutionary Genetics" came through my Newsfeed.
www.sciencealert.com...
It seems black and white were all we saw in our infancy and the ratio may be golden, after all. They found a Fibonacci sequence in our genome.
The meaning of life the universe and everything may be a number thing afterall.
Luckily for us, a motley team of researchers has just uncovered another striking connection between math and nature; between one of the purest forms of mathematics, number theory, and the mechanisms governing the evolution of life on molecular scales, genetics.
Abstract as it may be, number theory might also be one of the more familiar forms of math to many of us. It encompasses the multiplication, subtraction, division, and addition (arithmetic functions) of integers, or whole numbers and their negative counterparts.
The famed Fibonacci sequence is but one example, where each number in the sequence is the sum of the previous two. Its patterns can be found all through nature, in pinecones, pineapples, and sunflower seeds.
"The beauty of number theory lies not only in the abstract relationships it uncovers between integers, but also in the deep mathematical structures it illuminates in our natural world," explains Oxford University mathematician Ard Louis, senior author of the new study.
Of interest to Louis and his colleagues were mutations, the genetic errors that slip into an organism's genome over time and drive evolution.
I swear I was just in thread on something almost similar...
This so-called mutational robustness generates genetic diversity, yet it varies between species, and can even be observed in the proteins inside cells.
Studied proteins can tolerate around two-thirds of random errors in their coding sequences, meaning 66 percent of mutations are neutral and have no effect on their final shape.
We have known for some time that many biological systems exhibit remarkably high phenotype robustness, without which evolution would not be possible," explains Louis.
"But we didn't know what the absolute maximal robustness possible would be, or if there even was a maximum.
They then looked into protein folding and RNA to see how genotype maps to a specific trait.
Louis and colleagues wondered how close nature could get to the upper bounds of mutational robustness, so ran numerical simulations to compute the possibilities.
They studied the abstract mathematical features of how many genetic variations map to a specific phenotype without changing it, and showed mutational robustness could indeed be maximized in naturally-occurring proteins and RNA structures.
What's more, the maximum robustness followed a self-repeating fractal pattern called a Blancmange curve, and was proportional to a basic concept of number theory, called the sum-of-digits fraction.
"We found clear evidence in the mapping from sequences to RNA secondary structures that nature in some cases achieves the exact maximum robustness bound," says Vaibhav Mohanty, of Harvard Medical School.
"It's as if biology knows about the fractal sums-of-digits function."
Now I can make commentary. Their words are better anyway.
This implications are that perhaps everything in the universe really is unified under a ratio in which to exist.
The Fibonacci sequence isn't just some solipsistic mathematical precept that only exists in observation, it existed before our planet had pawn scum and went into our very construction.
Thanks science alert, your pushed me a little further to the agnostic today... sorta
And I can't title it that without the song...
edit on 8-9-2023 by Degradation33 because: (no reason given)
Even If they don't want to marry her, it is good to see scientists at least flirting with the idea of intelligent design.
There's no shame in it, she's a Reasonable Lady
There's no shame in it, she's a Reasonable Lady
a reply to: Degradation33
You did great! Sacred Geometry taught me about the Fibonacci sequence and all I see are examples everywhere. Love the subject!
S&F
Let me try one of these threads.
You did great! Sacred Geometry taught me about the Fibonacci sequence and all I see are examples everywhere. Love the subject!
S&F
a reply to: Degradation33
There are many of wonderful discussions if we discover maths in the universe because it is what/how the universe is, or if it is because we use maths to describe the universe.
Max Tegmark (love that guy so smart!) said in a book or a talk I don't quite remember the universe is a mathmatical object.
That is possible, but really depending on your definition of mathmatical object. Does it have a geometric shape? Yes. Can it be described in maths. Well we're doing pretty great in doing exactly that.
But that doesn't mean it is meaningful to think that is what it is, because ultimately whenever you reduce something to one property like fe 'being a mathmatical object' you lose all the other information it also contains.
And for what exactly?
I mean it would maybe be helpful if you would try to compare it with another universe that is not a mathmatical object, alas you'd first have to find one and figure out the way that universe expresses itself.
So I think the reason why we find maths in everything is more or less just proof that maths works. It is the right logical approach to analyse patterns and structures etc. I doubt it has any significance beyond that.
but wtf do I know, right? lol
There are many of wonderful discussions if we discover maths in the universe because it is what/how the universe is, or if it is because we use maths to describe the universe.
Max Tegmark (love that guy so smart!) said in a book or a talk I don't quite remember the universe is a mathmatical object.
That is possible, but really depending on your definition of mathmatical object. Does it have a geometric shape? Yes. Can it be described in maths. Well we're doing pretty great in doing exactly that.
But that doesn't mean it is meaningful to think that is what it is, because ultimately whenever you reduce something to one property like fe 'being a mathmatical object' you lose all the other information it also contains.
And for what exactly?
I mean it would maybe be helpful if you would try to compare it with another universe that is not a mathmatical object, alas you'd first have to find one and figure out the way that universe expresses itself.
So I think the reason why we find maths in everything is more or less just proof that maths works. It is the right logical approach to analyse patterns and structures etc. I doubt it has any significance beyond that.
but wtf do I know, right? lol
a reply to: Degradation33
I'm maybe pressed for time here. Granddaughter just got home from school; probably will want me to watch youtubes, so haven't read the linked article yet, or looked up the lyrics for the song. I'm one of those people who has to read lyrics else I mishear certain words.
Ratio is a number derived by dividing an integer with an integer. That's where the word rational comes from.
So my Calculus teacher back in the day was this cool hippy chic math nerd. One day she just up and tells us a story about when she was in college and went to a masquerade party where people are supposed to guess each other's costume.
She was dressed in a toga all wet and covered in sea weed. She was the guy tossed in the ocean to drown by the Pythagoreans for revealing the closely held secret of the existence of irrational numbers.
So OK pi is irrational number when looked at numerically, and yet it is rational as ratio of physical circumference over diameter. So irrational can be rational when looked at a bit differently.
Really, I just wanted to tell the story of the hippy math nerd.
I'll listen to the music now.
perhaps everything in the universe really is unified under a ratio in which to exist.
I'm maybe pressed for time here. Granddaughter just got home from school; probably will want me to watch youtubes, so haven't read the linked article yet, or looked up the lyrics for the song. I'm one of those people who has to read lyrics else I mishear certain words.
Ratio is a number derived by dividing an integer with an integer. That's where the word rational comes from.
So my Calculus teacher back in the day was this cool hippy chic math nerd. One day she just up and tells us a story about when she was in college and went to a masquerade party where people are supposed to guess each other's costume.
She was dressed in a toga all wet and covered in sea weed. She was the guy tossed in the ocean to drown by the Pythagoreans for revealing the closely held secret of the existence of irrational numbers.
So OK pi is irrational number when looked at numerically, and yet it is rational as ratio of physical circumference over diameter. So irrational can be rational when looked at a bit differently.
Really, I just wanted to tell the story of the hippy math nerd.
I'll listen to the music now.
a reply to: Peeple
More than many that pretend.
It shows up everywhere.
The rotation of planets too.
medium.com...
Everyone knows trees do this:
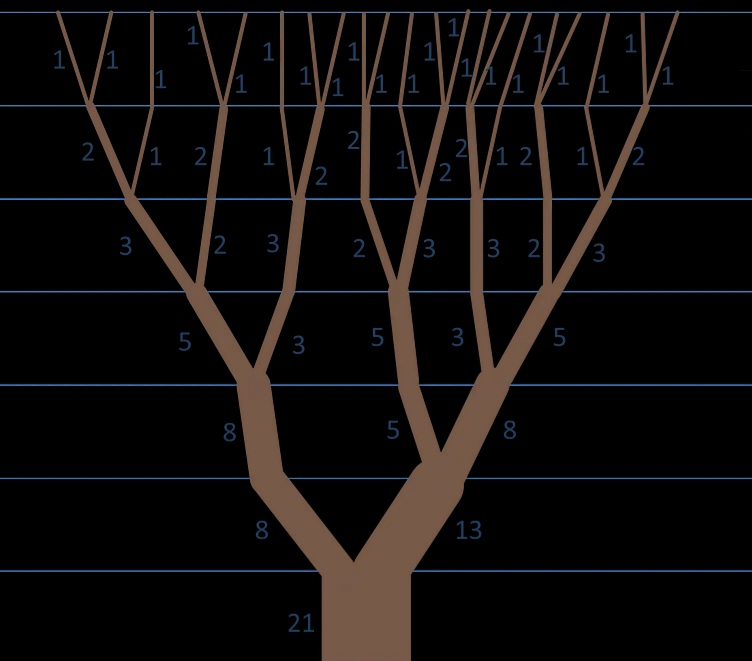
I had a, "Well you never know, this geometry is continuously sacred. You might want to rethink what goes into the randomness of it all"
May still be an aberration how it built itself up from smallest components.
And wouldn't you know it, The Golden ratio is there in pieces, but not across the board.
osjournal.org... (PDF WARNING)
It comes up a lot, but it's not always there to show correlation. It was surprising evolution was included in that.
"Evolution may follow sacred geometry" seems like a peace-mediating statement.
More than many that pretend.
It shows up everywhere.
The rotation of planets too.
medium.com...
The rotation of the sun: The rotation of the sun has been found to follow a pattern known as the Fibonacci sequence. This pattern is created by the way that the sun rotates on its axis, with each successive rotation forming a larger angle than the one before it
Everyone knows trees do this:

I had a, "Well you never know, this geometry is continuously sacred. You might want to rethink what goes into the randomness of it all"
May still be an aberration how it built itself up from smallest components.
And wouldn't you know it, The Golden ratio is there in pieces, but not across the board.
osjournal.org... (PDF WARNING)
Each column corresponding to mass number (A), neutron number (N) and proton number (Z) follows the Fibonacci sequence. For each nuclide in this group of Fibonacci nuclides the A, N and Z numbers are all sequential numbers in a Fibonacci sequence; a Fibonacci triad.
It comes up a lot, but it's not always there to show correlation. It was surprising evolution was included in that.
"Evolution may follow sacred geometry" seems like a peace-mediating statement.
edit on 8-9-2023 by Degradation33 because: (no reason
given)
a reply to: Degradation33
If I'm reading this correctly, it means that there is enough time for evolution to have made crows, cats, and us other people as we see them today. Thru maximizing. Less random.
"We found clear evidence in the mapping from sequences to RNA secondary structures that nature in some cases achieves the exact maximum robustness bound," says Vaibhav Mohanty, of Harvard Medical School.
"It's as if biology knows about the fractal sums-of-digits function."
If I'm reading this correctly, it means that there is enough time for evolution to have made crows, cats, and us other people as we see them today. Thru maximizing. Less random.
edit on 8-9-2023 by pthena because: (no reason given)
I came across a pattern relating to Fibonacci sequence and a DNA style pattern
Start by adding 1 to each number in the 1-9 sequence. Then just keep adding the sum of that number to the next. How the Fibonacci sequence works
The big numbers at reduced to digital sums. So 12 = 1+2=3 (Digit sums)
See the pattern in the vertical columns, not the left to right reading of the numbers.
111111111
123456789+
234567891
357924681
582582582
849516273
432198765
372615948
714714714
186429753
891234567
987654321
888888888
876543219
765432198
642975318
417417417
159483726
567891234
627384951
285285285
813579246
198765432
912345678
111111111
123456789 ( the sequence would repeat for infinity)
Notice the Fibonacci sequence moves down vertically on the outside columns.
Imagine this wrapped around a wheel. Connecting at the 111111111
Also imagine this sequence twisting to form like a DNA sequence
Start by adding 1 to each number in the 1-9 sequence. Then just keep adding the sum of that number to the next. How the Fibonacci sequence works
The big numbers at reduced to digital sums. So 12 = 1+2=3 (Digit sums)
See the pattern in the vertical columns, not the left to right reading of the numbers.
111111111
123456789+
234567891
357924681
582582582
849516273
432198765
372615948
714714714
186429753
891234567
987654321
888888888
876543219
765432198
642975318
417417417
159483726
567891234
627384951
285285285
813579246
198765432
912345678
111111111
123456789 ( the sequence would repeat for infinity)
Notice the Fibonacci sequence moves down vertically on the outside columns.
Imagine this wrapped around a wheel. Connecting at the 111111111
Also imagine this sequence twisting to form like a DNA sequence
edit on 8-9-2023 by Observationalist because: (no reason given)
a reply to: Degradation33
I've always said that interdisciplinary collaboration is what humanity needs to progress forward in the understanding of life's mysteries.
Here is a video which shows what your opening post describes for those who are visual learners.
I've always said that interdisciplinary collaboration is what humanity needs to progress forward in the understanding of life's mysteries.
Here is a video which shows what your opening post describes for those who are visual learners.
a reply to: nugget1
I am not going to jump and say it means anything just yet.
But all plants, coral, seashells, and on and on. And I thought number 23 was trippy.
a reply to: quintessentone
Thank you for video post..
And it seems the closer we get to the TOE the more things start to look like architecture.
a reply to: pthena
I think that's what it implies. I would assume. Maximizing it's neutral mutation tolerance per number theory.
You know this is sorta above me. All I saw was golden ratio and was transfixed, how it applies I need to read on.
royalsocietypublishing.org...
That happens in golden ratio.
I am not going to jump and say it means anything just yet.
But all plants, coral, seashells, and on and on. And I thought number 23 was trippy.
a reply to: quintessentone
Thank you for video post..
And it seems the closer we get to the TOE the more things start to look like architecture.
a reply to: pthena
I think that's what it implies. I would assume. Maximizing it's neutral mutation tolerance per number theory.
You know this is sorta above me. All I saw was golden ratio and was transfixed, how it applies I need to read on.
royalsocietypublishing.org...
The property that we will focus on in this paper is the mutational robustness ρp of a phenotype p, defined as the average probability that a single character mutation of a genotype mapping to phenotype p does not change the phenotype p. Typically larger neutral sets have higher robustness. For the (3-non-crossing [35]) RNA sequence-to-secondary structure GP map, it has been shown that the distribution of of robustness found upon random sampling of sequences accurately predicts the distribution of robustnesses for functional or non-coding RNAs found in nature [36], although for very short strands, naturally occurring RNA are marginally more robust [26]. In other words, for this system, the structure of GP map appears to largely determine the mutational robustness found in nature. Thus studying these more abstract mathematical features of the GP map may directly lead to predictions about naturally occurring phenotypes.
That happens in golden ratio.
edit on 8-9-2023 by Degradation33 because: (no reason given)
a reply to: quintessentone
It does look like a promo for inter-dip.
The age of polymath is over
The age of inter-dip super genius specialists has arrived.
Doesn't answer the question "Does the system operate just fine without human observation, or must humans observe so as to change reality in an order out of chaos sort of way?"
I don't know about any one else but that seems to be my question. I've already come to my own biased conclusion. It would be nice to get verifiable scientific proof.
I've always said that interdisciplinary collaboration is what humanity needs to progress forward in the understanding of life's mysteries.
It does look like a promo for inter-dip.
The age of polymath is over
The age of inter-dip super genius specialists has arrived.
Doesn't answer the question "Does the system operate just fine without human observation, or must humans observe so as to change reality in an order out of chaos sort of way?"
I don't know about any one else but that seems to be my question. I've already come to my own biased conclusion. It would be nice to get verifiable scientific proof.
originally posted by: pthena
a reply to: quintessentone
I've always said that interdisciplinary collaboration is what humanity needs to progress forward in the understanding of life's mysteries.
It does look like a promo for inter-dip.
The age of polymath is over
The age of inter-dip super genius specialists has arrived.
Doesn't answer the question "Does the system operate just fine without human observation, or must humans observe so as to change reality in an order out of chaos sort of way?"
I don't know about any one else but that seems to be my question. I've already come to my own biased conclusion. It would be nice to get verifiable scientific proof.
Wouldn't that type of inter-dip study involve one person who might need to be a remote viewer or astral projectionist and theoretical physicist (bio- astro- etc.) into the different systems of life? Maybe the act of observing can be done differently as in using remote viewing or by psychic means, so then would this be considered observing?
a reply to: quintessentone
I probably shouldn't have brought my questions into this. ********Thread drift warning*****
I think we should just stick with the immediate question. From royalsociety link:
Does this mean that natural mathematical functions increase the rate of evolution?
I haven't read the whole article yet, but it looks like it?
I better keep my side tangents to myself and just read the article to its conclusion.
Maybe the act of observing can be done differently as in using remote viewing or by psychic means, so then would this be considered observing?
I probably shouldn't have brought my questions into this. ********Thread drift warning*****
I think we should just stick with the immediate question. From royalsociety link:
Another important shared trait is that neutral sets are typically highly connected by point mutations due to a high average mutational robustness, meaning that they are likely to be fully connected, or percolate. This property hugely enhances the probability that a neutral set can be traversed by single mutational steps, allowing a much larger set of alternative phenotypes to be accessible than one could reach from a single genotype. In this way, enhanced robustness can lead to enhanced evolvability, which is the ability to discover new phenotypes
Does this mean that natural mathematical functions increase the rate of evolution?
I haven't read the whole article yet, but it looks like it?
I better keep my side tangents to myself and just read the article to its conclusion.
edit on 8-9-2023 by pthena because: (no reason
given)
a reply to: pthena
I was reading a science study not on this subject but rather on how will humans evolve in 50,000 years and the science theorizes that we will change very little. So, I surmise in answer to your question re: increase in rate of evolution, I would say 'no'.
I was reading a science study not on this subject but rather on how will humans evolve in 50,000 years and the science theorizes that we will change very little. So, I surmise in answer to your question re: increase in rate of evolution, I would say 'no'.
a reply to: pthena
After reading this, It looks that way. All the way to the upper bounds. That went way above my head at times.
It's like it's saying the allowance of single mutations to not change the phenotype leads to both more stability and useful natural selection. And that process follows a whatever curve that incorporates sum of digits fractions.
I may have read it wrong though.
Does this mean that natural mathematical functions increase the rate of evolution?
I haven't read the whole article yet, but it looks like it?
After reading this, It looks that way. All the way to the upper bounds. That went way above my head at times.
It's like it's saying the allowance of single mutations to not change the phenotype leads to both more stability and useful natural selection. And that process follows a whatever curve that incorporates sum of digits fractions.
I may have read it wrong though.
edit on 8-9-2023 by Degradation33 because: (no reason given)
a reply to: quintessentone
I had to skim a bit because my eyes were glazing over. Down by the end:
So this particular study wasn't designed to give the answer, hints for possible future study. The link 42 goes to a 1999 study.
I'm hesitant to offer hypotheses on subjects I'm not proficient at.
But what if once a large population of alpha predators exists there isn't much potential for evolution. Not much in the way of selecting for improvement.
Just a random thought.
I had to skim a bit because my eyes were glazing over. Down by the end:
Another interesting direction of future work would be to better understand the spectral properties of bricklayer’s graphs. These may provide insight into population distributions and average robustness on long evolutionary time scales [40,42],
So this particular study wasn't designed to give the answer, hints for possible future study. The link 42 goes to a 1999 study.
I'm hesitant to offer hypotheses on subjects I'm not proficient at.
But what if once a large population of alpha predators exists there isn't much potential for evolution. Not much in the way of selecting for improvement.
Just a random thought.
originally posted by: pthena
a reply to: quintessentone
I had to skim a bit because my eyes were glazing over. Down by the end:
Another interesting direction of future work would be to better understand the spectral properties of bricklayer’s graphs. These may provide insight into population distributions and average robustness on long evolutionary time scales [40,42],
So this particular study wasn't designed to give the answer, hints for possible future study. The link 42 goes to a 1999 study.
I'm hesitant to offer hypotheses on subjects I'm not proficient at.
But what if once a large population of alpha predators exists there isn't much potential for evolution. Not much in the way of selecting for improvement.
Just a random thought.
The study I read also mentioned that now that humanity is global and as such global mixing/procreation small changes in humanity won't be noticed for a very long time.
a reply to: Degradation33
Somewhere in all that a build up of not changing allows a quick one-step change.
but they leave it to future study for long term.
----------
If I'm guessing correctly, you are thinking about an underlying organizing principle that sort of runs on its own by preexisting formulae, per your creation story. Sums of digit fractions.
Maybe.
It's like it's saying the allowance of single mutations to not change the phenotype leads to both more stability and useful natural selection.
Somewhere in all that a build up of not changing allows a quick one-step change.
but they leave it to future study for long term.
----------
If I'm guessing correctly, you are thinking about an underlying organizing principle that sort of runs on its own by preexisting formulae, per your creation story. Sums of digit fractions.
Maybe.
a reply to: pthena
Yup.
I came across the idea on another site... and totally rejected it at the time, but it wouldn't extricate itself from my mind. It got more plausible.
The idea was "there is an organizing principle, that may be yet undiscovered, that drives biological life and the universe itself to build itself up in complexity."
Like if the universe had a Strong, Weak, Electromagnetic, Gravitational, and increased complexity force. Not dissimilar from increased disorder. Maybe the sequences we apply to what it does naturally caught this underlying principle in action.
I initially scoffed at the notion, but it's remained.
Yup.
I came across the idea on another site... and totally rejected it at the time, but it wouldn't extricate itself from my mind. It got more plausible.
The idea was "there is an organizing principle, that may be yet undiscovered, that drives biological life and the universe itself to build itself up in complexity."
Like if the universe had a Strong, Weak, Electromagnetic, Gravitational, and increased complexity force. Not dissimilar from increased disorder. Maybe the sequences we apply to what it does naturally caught this underlying principle in action.
I initially scoffed at the notion, but it's remained.
edit on 8-9-2023 by Degradation33 because: (no reason given)
a reply to: Degradation33
A Countervailing force to entropy
Half jesting I ask "Is it alive then?"
Like if the universe had a Strong, Weak, Electromagnetic, Gravitational, and increased complexity force. Not dissimilar from increased disorder.
A Countervailing force to entropy
Thus, if entropy is associated with disorder and if the entropy of the universe is headed towards maximal entropy, then many are often puzzled as to the nature of the "ordering" process and operation of evolution
...
suffices that living systems are open systems in which both heat, mass, and or work may transfer into or out of the system. Unlike temperature, the putative entropy of a living system would drastically change if the organism were thermodynamically isolated. If an organism was in this type of “isolated” situation, its entropy would increase markedly as the once-living components of the organism decayed to an unrecognizable mass.
Entropy_(order_and_disorder)
Half jesting I ask "Is it alive then?"
new topics
-
The daily fail trying to imply “it’s aliens”
The Gray Area: 2 hours ago -
Swarms of tiny 'ant-like' robots lift heavy objects and navigate obstacles
Science & Technology: 3 hours ago -
NYPD Chief Jeffrey Maddrey Resigns - Forced Officers to Give Sex for Overtime Pay and Favors.
Posse Comitatus: 4 hours ago -
The Carpet Coating that Attacked the Environment
Medical Issues & Conspiracies: 8 hours ago -
Microplastics in your drinks
Medical Issues & Conspiracies: 9 hours ago
top topics
-
The Carpet Coating that Attacked the Environment
Medical Issues & Conspiracies: 8 hours ago, 11 flags -
China Working on 'Drone Mothership' Plane
Military Projects: 15 hours ago, 10 flags -
Microplastics in your drinks
Medical Issues & Conspiracies: 9 hours ago, 5 flags -
Happy Rush Day 2024 - 2112
Music: 14 hours ago, 4 flags -
NYPD Chief Jeffrey Maddrey Resigns - Forced Officers to Give Sex for Overtime Pay and Favors.
Posse Comitatus: 4 hours ago, 2 flags -
12-21-24 Usyk-Fury II
World Sports: 17 hours ago, 1 flags -
Swarms of tiny 'ant-like' robots lift heavy objects and navigate obstacles
Science & Technology: 3 hours ago, 1 flags -
The daily fail trying to imply “it’s aliens”
The Gray Area: 2 hours ago, 1 flags
active topics
-
Why isn't Psychiatry involved?
Social Issues and Civil Unrest • 29 • : GENERAL EYES -
An Interesting Conversation with ChatGPT
Science & Technology • 33 • : GENERAL EYES -
The daily fail trying to imply “it’s aliens”
The Gray Area • 1 • : BeyondKnowledge3 -
Microplastics in your drinks
Medical Issues & Conspiracies • 16 • : BeyondKnowledge3 -
Just spotted an unusual aircraft Melbourne Australia
Aliens and UFOs • 7 • : Cavemannick -
Swarms of tiny 'ant-like' robots lift heavy objects and navigate obstacles
Science & Technology • 2 • : BeyondKnowledge3 -
Post A Funny (T&C Friendly) Pic Part IV: The LOL awakens!
General Chit Chat • 7942 • : imitator -
Drones everywhere in New Jersey ---and Elsewhere Master Thread
Aliens and UFOs • 221 • : imitator -
Donald Trump Should Offer AMNESTY to the BIDEN FAMILY For Indicting Blackmailers-Controllers.
US Political Madness • 78 • : WeMustCare -
JOSEPH BIDEN Says Democrat Coup Leaders Forced Him to Resign the Presidency - Eff 1.20.2025.
2024 Elections • 55 • : WeMustCare
