Does the Failure to Find Dark Matter Prove the Existence of Dark Matter?

a reply to: glend
Earlier in the thread I re-posted a graphic of some dark matter candidates, and indeed axions are shown as candidates, see the "Axion DM" reference near the lower left:



We don't know if the hypothesized axions exist but I know of only one experiment searching for axions, the The ADMX G2 Experiment, shown here:


I think the experiment has already ruled out some parameter space (from from 1.9 μeV to 3.53 μeV) but they have more parameter space to search which will take 10 years and maybe some equipment upgrades.

I'll take What Is Extra Dimensions for $500, Alex

a reply to: Arbitrageur

Firstly, I much appreciate your effort in writing this stuff. Your patience is admirable

The galaxy rotation curves and gravitational lensing should not be too hard to understand though.

Luckily, these terms and their meaning is within my level of understanding space.

One more question concerning this:

We have not ruled out the possibility of dark matter in our solar system but even if it's at the expected density it's too small to detect because on a galactic scale, the 93 million mines from Earth to the sun is a tiny distance and cosmically speaking it contains a tiny amount of dark matter. On a human scale the 93 million mile radius around the sun could be expected to contain a "large" amount of dark matter, something like 2.3 billion tons! That sounds like a lot, so how could we not detect that? The reason is because the mass of the sun is roughly a billion times a billion times larger than that and we don't have any measurement methods precise enough to detect something that's a billionth of a billionth of the sun's mass

So on solar system level, on a trajectory Earth-Sun the mass of dark matter "should" be minuscule...to small to measure. I find it surprising as..is it not theorized that the mass of dark matter (or gravity that it produces) holds galaxies together, yet on this trajectory of 93 mil miles radius, this mass is so minute, you said..million times a million smaller than that of a Sun. The mass of the Sun is 332,946 times mass of the Earth. Stands to reason that all celestial objects in the solar system have way more mass than respective theorized Dark matter in said region of space, and therefore way more gravitational pull towards each other than any 3rd party source of gravity.

On a layman level, one might be tempted to conclude that all those celestial objects when combined together would greatly overcome any such minuscule mass of dark matter for a given region.

Is it fair to say that this Dark matter mass is rather small to be holding galaxies together ?

If it's milion times a milion (that's a huuuuge number) less in mass then the sun for said radius (which is a rather small star when compared universally). Even Earth which is only a few hundred thousands less in mass.

Did I misunderstand or is it a part of the theory that Dark matter influences local planetary orbits ? If it holds galaxies together on such a large scale, surely...?

Again, thanks for your time

a reply to: Arbitrageur

I do not know anything about physics or what you are talking about...the only thing I have to say...or can say about it is that my underbelly says it has somwething to do with gravity. They say they have found out how gravity works but I just do not believe it. Because the experts are holding on to a false theory they are shooting themselves in the foot concerning making progress when it comes to dark matter and dark energy.

originally posted by: Arbitrageur
The two alternative ideas being debated to discuss observations have been these. Either:
A. There some kind of matter we can't see causing galaxies that are rotating so fast they would fly apart without it, or
B. Our model of gravity which seems to work at the scale of our solar system is completely broken at larger scales like galaxies.

Can anybody think of a third option?

It is also possible that our universe is not a closed system, and that the effects labeled "dark matter" and "dark energy" are actually signs of matter/energy continuously entering our universe from somewhere else.

The exact means by which that might be occurring could take many possible forms, most of which would probably be very hard to confirm within the constraints of current knowledge and technology.

With that in mind, my personal favorite theory -- which, like any theory, could be utterly wrong -- is that proposed by Nikodem Popławski, who hypothesizes that what happens within black holes may well be quite different from what is commonly assumed.

From the Wikipedia article:

Black holes as doorways

Popławski's approach is based on the Einstein–Cartan theory of gravity which extends general relativity to matter with intrinsic angular momentum (spin). Spin in curved spacetime requires that the affine connection cannot be constrained to zero and its antisymmetric part, the torsion tensor, must be a variable in Hamilton's principle of stationary action which gives the field equations. Torsion gives the correct generalization of the conservation law for the total (orbital plus intrinsic) angular momentum to the presence of the gravitational field, but also modifies the Dirac equation for fermions.

Gravitational effects of torsion on fermionic matter are significant at extremely high densities which exist inside black holes and at the beginning of the Universe. Popławski theorizes that torsion manifests itself as a repulsive force which causes fermions to be spatially extended and prevents the formation of a gravitational singularity within the black hole's event horizon.[12] Because of torsion, the collapsing matter on the other side of the horizon reaches an enormous but finite density, explodes and rebounds, forming an Einstein-Rosen bridge (wormhole) to a new, closed, expanding universe.[13][14] Analogously, the Big Bang is replaced by the Big Bounce before which the Universe was the interior of a black hole.[15] This scenario generates cosmic inflation, which explains why the present Universe at largest scales appears spatially flat, homogeneous and isotropic.[16][17] It may explain the arrow of time, solve the black hole information paradox, and explain the nature of dark matter.[18] Torsion may also be responsible for the observed asymmetry between matter and antimatter in the Universe.[19] The rotation of a black hole could influence the spacetime on the other side of its event horizon and result in a preferred direction in the new universe. Popławski suggests that the observed fluctuations in the cosmic microwave background might provide evidence for his hypothesis.[20]

Though by no means verified at this point, Doctor Popławski's hypothesis would explain a great deal that is not explained, and conforms to what is already known to a remarkable degree.

Of course, that may not be how things are at all, but at the very least, the assumption that our universe is all that exists remains nothing more nor less than an assumption -- an assumption increasingly challenged by observation.

originally posted by: MarioOnTheFly
Is it fair to say that this Dark matter mass is rather small to be holding galaxies together ?

Did you watch the video in the OP yet?

It mentions the "Fritz" galaxy where the dark matter mass is approximately zero. And another similar galaxy was also found. But those are atypical.

More typically the ratio is something like 20% luminous matter to 80% dark matter though that is not fixed, the amount seems to very from galaxy to galaxy, but this is the first time i heard of that galaxies were found which appeared to have no dark matter.

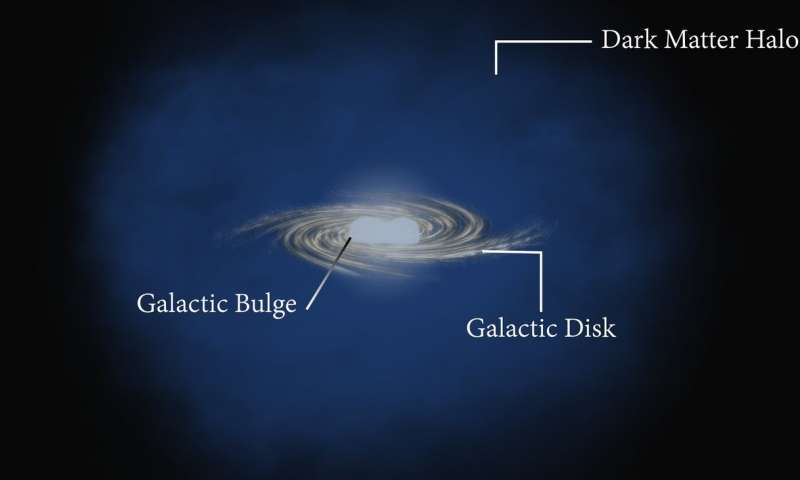
Also a point you may be missing which perhaps I didn't explain that well. For galaxies that do have dark matter, most if it may not even be inside the galactic disc, which for the Milky way might be roughly about 1000 light years thick and 100,000 light years across. It's hard to map exactly where the dark matter is for one galaxy but it's thought to be something like shown in this drawing of a dark matter halo, where most of it is not inside the galactic disk where we are:

phys.org...


That's sort of a guess though. I think the dark matter maps made using gravitational lensing are more reliable to show where the dark matter really is, and those are made with galaxy clusters.

Unprecedentedly wide and sharp dark matter map

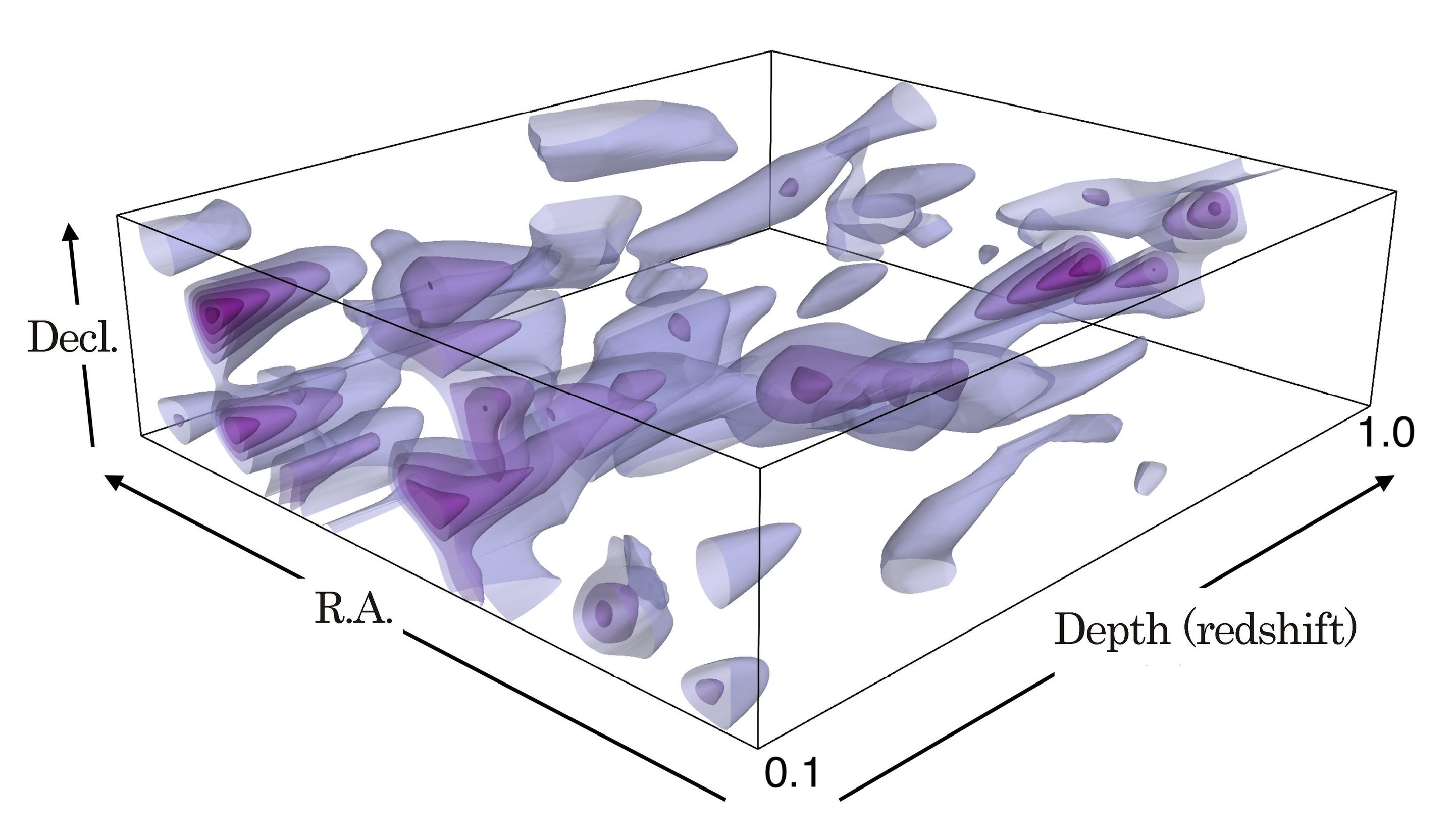
Figure 4: An example of 3D distribution of dark matter reconstructed via tomographic methods using the weak lensing technique combined with the redshift estimates of the background galaxies.

originally posted by: zatara
They say they have found out how gravity works but I just do not believe it.

Who said they know how gravity works? You are mistaken, we are not saying we know how gravity works, we say we can predict the effects of gravity which is different. We have fantastic proof that our gravity models work in our solar system when we can land a spacecraft on Mars with a high degree of accuracy.

We have even more proof that in the past we have noted gravitational effects for something we couldn't see, in our own solar system. So we started looking for what was causing these gravitational effects, and that's how Neptune was discovered. It turns out dark matter is much harder to detect than Neptune.

a reply to: Majic
With my physics education, I can usually understand what a lot of physicists are talking about if they aren't using too much specialized jargon in a specific field I'm not familiar with. But I was having a hard time following Poplawski and years ago I made a thread on Physicsforums asking about a paper he wrote. The feedback I got was politely stated something like if a more detailed analysis was done, then the flaws in Poplawski's paper would become more apparent, or something like that.

But Poplawski aside, this idea that something outside our universe might be affecting observations seems to be a popular thought by posters in this thread, but I think those folks need to try to read and understand the link I posted on page 1, and I'll re-post it here:

What Astronomers Wish Everyone Knew About Dark Matter And Dark Energy

As that article explains, there are multiple lines of evidence that all seem to fit together, using the dark energy and dark matter models. If you say "matter/energy continuously entering our universe from somewhere else", I don't know how that would even apply to dark matter. I'm not aware that any observations have suggested there is either more or less dark matter in the past.

In fact one thing that link talks about is why models other than dark matter don't seem to fit observations as well (like the MOND family) is because it presumes the early universe didn't have dark matter either. What happens if you accept MOND as explaining the rotation curves of galaxies is you have even more problems to solve like what causes gravitational lensing observed and you'd have to make a new model for the evolution of the universe which I don't know of anybody who has made one that fits and neither does the author of that article.

If you say dark matter is entering our universe that implies there was less in the past and again you have the same problem, we can't explain the evolution of the universe if the dark matter wasn't there in the past. Or if you had significantly more dark matter in the past and it was leaving the universe, again we also wouldn't have the universe evolving the way we see.

So I think the models we have for evolution of the universe put constraints on how much matter/energy content can be coming or going. You could try to come up with an entirely new model that could get around those constraints but until someone does that successfully, I don't see how dark matter coming or going is a viable hypothesis unless we are talking about amounts which are not very significant in which case it's not a viable solution to understanding dark matter observations, except as one more possible component.

The Dark Matter physicist who has posted on ATS, ErosA433 mentioned that there's no guarantee a hypothesized dark matter particle has to interact with ordinary matter. Neutrinos interact so weakly they almost all pass right through the entire Earth without interacting at all and if there's a dark matter particle that has essentially zero interaction with ordinary matter, it may not be possible to find it with any direct detection experiment, so we could be stuck with indirect detection, which isn't as gratifying but since we can make clearer maps than ever of where the dark matter is is as seen above, indirect detection does have some utility.

a reply to: Arbitrageur

Granted, the Wikipedia article probably isn't the best reference for Popławski, given its rather thick, jargon-laden paragraphic blob of a summary, but it's concise and not locked behind an institutional paywall.

That said, Doctor Popławski's work on the subject is by no means an unsupported proposition. Quite a bit more detail is available via links on his website on the Publications page.

His publications cover a wide range of subjects aside from the "big bounce", but one which seems to summarize it fairly well and offers plenty of supporting math and citations would be:

UNIVERSE IN A BLACK HOLE IN EINSTEIN–CARTAN GRAVITY (PDF)
The Astrophysical Journal, 832:96 (8pp), 2016 December 1

ABSTRACT

The conservation law for the angular momentum in curved spacetime, consistent with relativistic quantum mechanics, requires that the antisymmetric part of the affine connection (torsion tensor) is a variable in the principle of least action. The coupling between the spin of elementary particles and torsion in the Einstein–Cartan theory of gravity generates gravitational repulsion at extremely high densities in fermionic matter, approximated as a spin fluid, and thus avoids the formation of singularities in black holes. The collapsing matter in a black hole should therefore bounce at a finite density and then expand into a new region of space on the other side of the event horizon, which may be regarded as a nonsingular, closed universe. We show that quantum particle production caused by an extremely high curvature near a bounce can create enormous amounts of matter, produce entropy, and generate a finite period of exponential expansion (inflation) of this universe. This scenario can thus explain inflation without a scalar field and reheating. We show that, depending on the particle production rate, such a universe may undergo several nonsingular bounces until it has enough matter to reach a size at which the cosmological constant starts cosmic acceleration. The last bounce can be regarded as the big bang of this universe.

As I mentioned, Doctor Popławski's hypothesis could be utterly wrong, though I'm not convinced a polite but vague dismissal by unnamed members of a physics forum establishes that.

Also, it's certainly worth noting that my own interpretations of his proposals may be even more than utterly wrong, so I don't recommend accepting them as authoritative or representative of them in any way, just my own opinions subject, as always, to error.

As I understand it, the gist of the "big bounce" model is that if the Einstein–Cartan theory of gravity is correct, and Popławski is correct, then conservation of angular momentum prevents the formation of infinitely dense gravitational singularities within black holes.

Rather, at a certain point, collapsing matter "bounces" within the event horizon, and expands to form what could be considered a new universe with properties consistent with what we observe within our own. This process may repeat several times before attaining the kind of accelerating cosmological inflation currently attributed to "dark energy".

One possibility for where "dark matter" would potentially fit into all this is considered here:

Matter-antimatter asymmetry and dark matter from torsion
PHYSICAL REVIEW D 83, 084033 (2011)

We propose a simple scenario which explains the observed matter-antimatter imbalance and the origin of dark matter in the Universe. We use the Einstein-Cartan-Sciama-Kibble theory of gravity which naturally extends general relativity to include the intrinsic spin of matter. Spacetime torsion produced by spin generates, in the classical Dirac equation, the Hehl-Datta term which is cubic in spinor fields. We show that under a charge-conjugation transformation this term changes sign relative to the mass term. A classical Dirac spinor and its charge conjugate therefore satisfy different field equations. Fermions in the presence of torsion have higher energy levels than antifermions, which leads to their decay asymmetry.
Such a difference is significant only at extremely high densities that existed in the very early Universe. We propose that this difference caused a mechanism, according to which heavy fermions existing in such a Universe and carrying the baryon number decayed mostly to normal matter, whereas their antiparticles decayed mostly to hidden antimatter which forms dark matter. The conserved total baryon number of the Universe remained zero.

Interestingly, this "dark matter = antimatter" proposal does not depend on a "big bounce" as a precondition, but is compatible with that model.

There's a lot more to the question of relationships between a "big bounce" and "dark matter", of course, including but definitely not limited to considerations of how information/matter/energy from parent universes would affect child universes and how accelerating cosmological inflation may potentially, in and of itself, even explain gravity. Also, when I referred to "signs of matter/energy continuously entering our universe from somewhere else", I wasn't necessarily suggesting "dark matter" per se is being continually added to the universe, rather that the phenomena labeled "dark matter" and "dark energy" are possible signs of such a process, which is not the same thing, but I think I've already laid out enough to get started.

Ultimately, however, whether any of this is right or wrong, my intention is not to prove anything specific to a given theory. Rather, it is to point out that when you ask if anybody can think of a third option regarding possible explanations for the phenomenon of "dark matter", the answer is "yes".

originally posted by: Majic
One possibility for where "dark matter" would potentially fit into all this is considered here:

Matter-antimatter asymmetry and dark matter from torsion

Ultimately, however, whether any of this is right or wrong, my intention is not to prove anything specific to a given theory. Rather, it is to point out that when you ask if anybody can think of a third option regarding possible explanations for the phenomenon of "dark matter", the answer is "yes".

Yes, I asked if there was a third option because I would like to know if there is. I still don't.

If you're referring to that paper "Matter-antimatter asymmetry and dark matter from torsion" as a third option in the context of my question, I don't see it as a third option. It says dark matter = hidden antimatter which is a type of dark matter model, isn't it?

This chart of dark matter hypothesis has "hidden sector dark matter" as a candidate on it which seems to be what that paper is talking about:



I think I somewhat follow that paper by Poplawski better than the one I asked about years ago. That I follow it doesn't mean it's right, but it's suggesting a type of dark matter hypothesis.

Poplawski is not the only one with ideas of stuff we can't see in the hidden sector, it's also an idea in string theory, but apparently Poplawski hates string theory (I'm somewhat skeptical about it also as my signature suggests).

Dark matter may be hiding in a hidden sector

So the answer to my question about a third option could be yes, but I still haven't seen an example of a third option. Poplawski's idea is about stuff that's difficult to detect. That's dark matter, not a third option. As for what dark matter could be, obviously the graphic shows there are a lot of options for that.

a reply to: Arbitrageur

I suppose mentioning Popławski's "dark matter = antimatter" proposal -- and perhaps Popławski at all -- may have been a mistake, since, as you pointed out, that paper doesn't directly bear on the possibility that "dark matter" may be a consequence of the universe not being a closed system -- if it isn't, in fact, a closed system.

It may well be possible that the universe does indeed exist in complete isolation with no outside influences, and that "dark matter" can be explained entirely within that context. If so, that would likely be the simplest problem to solve for an observer within that universe, though not necessarily simple in itself.

But it may also be possible that "dark" phenomena are challenges to the assumption that the universe is a closed system -- an assumption that is just as unproven as the suggestion that it may not be closed. If that is the case, then theories which don't account for it would necessarily fail to explain these observations.

In all this, I'm not presuming to know one way or the other, just presenting it as a possibility that doesn't seem to fall within the domains of the two alternatives offered in the opening post.

I would also like to emphasize that while I find Popławski's work compelling in many ways, it doesn't explain everything, and is by no means the only possible mechanism by which matter and/or energy may be entering the universe. Indeed, the "big bounce" theory allows for accelerating cosmic inflation without the need for additional input after the threshold has been reached.

So please forgive my prior lack of focus on the specific question as I try to state what I consider to be a third option as simply as I can: That the phenomenon of "dark matter" may be a consequence of the universe not being a closed system.

You are, of course, free to reject it as a third option if you don't consider it possible, but what would be the basis for dismissing such a possibility?

a reply to: Majic
If you don't have a paper representing a third option to explain dark matter observations, can we say there is no third option that anybody has written a peer reviewed paper about yet?

originally posted by: Arbitrageur
a reply to: Majic
If you don't have a paper representing a third option to explain dark matter observations, can we say there is no third option that anybody has written a peer reviewed paper about yet?

We could say that, but that wouldn't mean anybody hasn't, and that wasn't the question you asked, nor that I was responding to. It also seems like an appeal to authority regarding a question for which authorities don't claim to have an answer.

More broadly, we could say that an unwillingness to consider alternatives when existing hypotheses fail to bear fruit would itself adequately explain most, if not all, impasses in research. Perhaps we could consider that a third option.

To think outside the box, it is necessary to realize there is a box.

a reply to: Majic
Of course I didn't want to have our thinking stuck inside a box when we're not having much luck finding dark matter.
So that's why when I heard "two options" I immediately wondered if there were more, and I didn't specifically say "credible" options but it should be implied.

I'm not saying open universe is not credible, but what I am saying is I don't understand how it would explain dark matter observations, so that's how I ended up asking for a paper where someone might have written an explanation I could understand, because I'm really not following your third option. I understand the open universe part as a general concept, I just don't see how that explains dark matter observations.

a reply to: Arbitrageur

Sorry if I came off as snarky on the peer review question, particularly since I'm well aware of how valuable it can be in verifying the methodology, cogency, consistency and validity of any kind of study, particularly observational studies. Papers presenting measurements of any kind should always satisfy rigorous peer review, period.

It can also be indispensable in shaking the fairy dust out of hypotheses, and any proposal that survives a diligent peer review process is far more likely to be confirmed than one that doesn't.

But in a speculative context, using peer review as a filter can exclude otherwise viable possibilities from consideration, and can confound progress when investigating unexplained phenomena. Peer review status is also, unfortunately, often erroneously conflated with infallibility, which it most definitely is not, and when confronting the unknown, orthodoxy is a liability.

Particularly in a case like dark matter, until a possibility can be logically dismissed based on sound evidence, it remains a possibility.

Regarding dark matter, I wanted to respond in more detail now, but am somewhat pressed for time (in the words of Elbert Hubbard, life is just one damn thing after another) and am hesitant to go into this further without sufficient context, and that means a lot more writing. It's not that the concepts themselves are particularly esoteric, but rather that it's essential to clarify where they fit in the grand scheme of things.

Ironically, while trying to write up a summary that was intended not to be a magnum opus, I found myself writing one anyway. It's already been necessary to break it into two parts and it might require more. I'm sure I could trim a lot of fat, but to paraphrase Pascal, it's longer because I haven't had time to make it shorter.

Brevity is the soul of wit (which means I have no soul), and I subscribe to the notion that the best explanations are the simplest, but without adequate background, a simple explanation on its own may not explain enough.

So if the prospect of an under-edited multi-post blast of stilted, conjectural prose isn't too off-putting, I'm willing to flesh it out and post it probably in the next few days or so, time permitting. A more concise summary would probably take longer to pare down without begging an excessive number of questions and possibly being even less helpful.

Thus I figure I should ask if you're okay with the long-winded version before continuing.

I came across this article on the cms.cern website. Unfortunately, there's no date or references. Have any experiments detected the "neutralino" yet?

Question for you and Eros if he's around about the collider - the article states: "So how do you detect an “invisible” particle? CMS will be able to find the neutralino indirectly – by identifying when the energy used to make it goes missing." In general, when a collision experiment is performed, does the energy in always equal the energy out? If there is an energy deficit, and assuming it is measurable, how would they know whether it was the result of one, two or more particles being produced by the collision?
Would appreciate a link to a publication if there is one. Thanks.

cms.cern...

Evidence from the depths of the Universe has ruled out a number of models for what the mysterious dark matter might be, but one candidate that fits so far is the lightest supersymmetric particle (LSP) otherwise known as the “neutralino”, the lightest of a whole range of new particles suggested by a theory called supersymmetry.

If the neutralino exists it will likely be stable, heavy, neutral, and will not interact electromagnetically. This makes it a perfect candidate for a substance that pervades the universe without being spotted. If supersymmetric particles exist, they are very likely to be produced in collisions in the LHC. The heavy particles will decay into combinations of leptons (like electrons and muons) and quarks (which will cause sprays of particles called jets) as well as into neutralinos that will not decay any further. Therefore many neutralinos will pass through the CMS detector, without depositing any energy or leaving a trail.

So how do you detect an “invisible” particle? CMS will be able to find the neutralino indirectly – by identifying when the energy used to make it goes missing.

Momentum in equals momentum out…
One of the most fundamental laws of physics is that ‘momentum is conserved’. In other words, the total momentum before a collision is equal to the total momentum after. If the total momentum of the observed particles that emerge from a proton-proton collision does not equal the momentum of the two protons, we can deduce there must be an invisible particle somewhere that carried away that missing momentum.

As we collect all the particles we can also add up their momenta and energies (in the “transverse” direction, i.e. at right angles to the beam line) and reconstruct the entire collision; like building a giant jigsaw puzzle. When a neutralino is formed and we can’t detect it we see an imbalance in the collision, with particles flying out one side but not the other and the energy not adding up. This shows up as a hole in the jigsaw puzzle: a missing particle seen through its missing energy or momentum.

The CMS is “hermetic” when it comes to finding missing particles. This means that, to the extent possible, it catches every detectable particle emerging from a collision. Large detectors have “channels for escape”, regions where particles cannot be detected because of cables or other mechanical support. These regions must be minimised to ensure that standard particles can't slip by undetected. This way, if the energy or momentum is "missing", it really is due to an invisible particle.

To search for this missing energy, it is important that the CMS has a good hadron calorimeter as well as detectors at every angle around the beam line. To ensure that particles flying from all directions will be detected, this includes the very shallow angles known as the “forward region”. Tags / keywords: Dark Matter

originally posted by: Majic
So if the prospect of an under-edited multi-post blast of stilted, conjectural prose isn't too off-putting, I'm willing to flesh it out and post it probably in the next few days or so, time permitting. A more concise summary would probably take longer to pare down without begging an excessive number of questions and possibly being even less helpful.

Thus I figure I should ask if you're okay with the long-winded version before continuing.

I can be too long-winded myself sometimes.

As Einstein said in 1933: “It can scarcely be denied that the supreme goal of all theory is to make the irreducible basic elements as simple and as few as possible without having to surrender the adequate representation of a single datum of experience.”

But I know what you mean, that's easier said than done. How many multi-posts are we talking about? The limit per post used to be something like 10,000 characters I think, might be lower now like 8000, not sure, but two posts at the upper limit would be fine, I would definitely read them. Ten posts at the upper limit, I probably wouldn't read them all unless I really got hooked on the first two posts.

originally posted by: Phantom423
I came across this article on the cms.cern website. Unfortunately, there's no date or references. Have any experiments detected the "neutralino" yet?

Question for you and Eros if he's around about the collider - the article states: "So how do you detect an “invisible” particle? CMS will be able to find the neutralino indirectly – by identifying when the energy used to make it goes missing." In general, when a collision experiment is performed, does the energy in always equal the energy out?

We observe that energy always seems to be conserved in physics, but measuring the exact energy in and exact energy out can be a challenge. For example if the LHC proton-proton collision is exactly head on it's the highest possible energy but what if it's not perfectly head on?

Regardless of whether the protons strike head on or not, we do know something about their momentum, which is that it's all in the directions of the opposing beams. There is no momentum perpendicular to the beams before the collision, so if the detector measures the momentum of all particles after the collisions, the net sum of all those momenta should still be zero in a direction perpendicular to the beam (transverse), if every particle could be detected.

If course, not every particle is detected, such as neutrinos, which mostly pass through the entire earth without interacting so there's little chance one will be detected in the CMS detector. If neutralinos exist and are similarly difficult to detect, the measured transverse momentum after the collision would not be zero.

If there is an energy deficit, and assuming it is measurable, how would they know whether it was the result of one, two or more particles being produced by the collision?
Would appreciate a link to a publication if there is one. Thanks.

That's a very complicated topic. This paper about the Missing transverse energy performance gets into more detail. It might be a bit dated as the LHC has since been upgraded but the conservation concepts are still the same at higher energies.

Missing transverse energy performance of the CMS detector

Neutral weakly interacting particles, such as neutrinos, escape from typical collider detectors without producing any direct response in the detector elements. The presence of such particles must be inferred from the imbalance of total momentum. The vector momentum imbalance in the plane perpendicular to the beam direction is particularly useful in pp and p¯ p colliders, and is known as missing transverse momentum...Its magnitude is called missing transverse energy...

originally posted by: Arbitrageur
How many multi-posts are we talking about?

My current estimate is greater than one, and less than the number of particles in the universe.

Kidding aside, it's looking more like 2-3 posts. My primary concern is getting them to explain the idea without being too convoluted and hopefully making them worth reading. The more concise the text, the greater my success on that front.

I'm rediscovering the difference between tossing out a general possibility and developing it enough to agree with current observations. I think I can do that, but if I fail to do so, I'll withdraw the proposal.

The process is also leading me to reexamine how dark phenomena might be explained within an isolated universe, since if that's possible, there's no need to postulate external influences.

One thing that keeps springing to mind is the nature of gravity and how that might relate to all this, which has me revisiting old notes from a fresh perspective.

a reply to: Majic
I'm sure I can handle reading a third post, especially if you're going to put that much thought into it!

a reply to: Arbitrageur

Oh great! No pressure.

At the moment, the biggest problem I'm running into is one of scope.

It's not hard to, say, invoke the first law of thermodynamics and point out that if energy is being added to what is assumed to be a closed system, observations will not agree with that assumption. That's essentially what I had in mind when suggesting it as a third option.

On the other hand, going into how that additional energy would manifest within our universe in a manner consistent with observation is a decidedly more ambitious task, whether specifically in the case of dark matter or more generally to include dark energy, with the two phenomena possibly interrelated.

Admittedly, I'm probably biting off more than I can chew with that, but it's proving to be an irresistible challenge, with the incidental benefit of learning (and relearning) a whole bunch of cool things about dark phenomena, physics and cosmology in the process.

Along the way, I'm thinking less in terms of a "magnum opus" and thinking more in terms of testing some postulates and seeing which ones are left standing. I'm also looking for published work that might shed some light on all this, whether through theory or observation.

As an example, if energy were added to the universe in a nonuniform manner, it would invalidate the cosmological principle and open the door to all sorts of funny business. Such energy could potentially distort spacetime in a manner that would be unexpected in a homogeneous, isotropic universe, but maybe ours isn't. Or maybe it is. Maybe someone has already looked at this.

I don't want to waste anyone's bandwidth (any more than I already have, anyway), so I want whatever I present here to have some legs. At the same time, if I have something interesting to share, I don't want to sequester it within some sort of grandiose ode to sophistry that never sees the light of day, so in those cases, I'll try to just post it and see where it goes.

Frankly, this is turning into more of a research project than a grand conjecture, but I find myself unable to be disappointed by that, and hope you won't be either.

a reply to: Arbitrageur

Thanks for this OP it is really interesting. I do not claim to fully understand it but I get the gist. I love info like this because I write sci-fi fantasy books and this will tye right into a story.

a reply to: Arbitrageur

Can anybody think of a third option?

I have an option in my proposal.

Unfortunately you don't understand my proposal.

I will try to explain my finding. And, hopefully. Someone might understand it.

I go on about implied zero's (although they're clearly written in my theory). They are a Lagrangian Point.

My proposal is on this page

Diagram 3 shows 9 connections of 2 charges (-) and (+). 3 sets for each axis' A, B and C. (-) to (+), (-) to (-) and (+) to (+).

There is no 0 in this table of connections. Signifying a Lagrangian Point. And therefore. A particle.

There has to be a Lagrangian point in the (-) to (+) connections in the A, B and C axis'. Even though my theory doesn't state it.

To test this. I have used two colours (green (-) and red (+)).

(-) to (-) green connection remains green.

(+) to (+) red connection remains red.

(-) to (+) green/red connections would have a Lagrangian Point where the two colours meet.

And. Therefore. A particle.



I thought it was an interesting observation. Although. I'm not sure if it would be DM or Graviton or another matter.