Flat earth theory?

originally posted by: InfiniteTrinity
a reply to: neutronflux

Whats the matter? Can you point out what you disagree with?

You ever state a clear and concise argument why you think geostationary /geosynchronous satellites do not have angular momentum when using the earth’s axis as a point of origin?

a reply to: Phage

Fine. Tell that to the other car when it hits the car in front of it.

Random example that has nothing to with this discussion. Please.

Toward Earth's center, but that acceleration is counteracted by the satellite's momentum, the result is an orbit.

I see. How does it maintain its speed to maintain its trajectory through space if its in fact being pulled down by gravity, Phage?

You see Phage, the other satellites have to fall around curvature to maintain a curved trajectory through space.

originally posted by: InfiniteTrinity
a reply to: neutronflux

So straight down then?

How does it maintain its speed to maintain the curved trajectory through space then?

Go ahead.

From the launch vehicle that placed it in orbit like any other satellite. Except for correction thrust from a limited supply of fuel?

Let’s check a cited source...

science.howstuffworks.com...

Orbital Velocity and Altitude
PREVNEXT
A rocket must accelerate to at least 25,039 mph (40,320 kph) to completely escape Earth's gravity and fly off into space (for more on escape velocity, visit this article at NASA).

Earth's escape velocity is much greater than what's required to place an Earth satellite in orbit. With satellites, the object is not to escape Earth's gravity, but to balance it. Orbital velocity is the velocity needed to achieve balance between gravity's pull on the satellite and the inertia of the satellite's motion -- the satellite's tendency to keep going. This is approximately 17,000 mph (27,359 kph) at an altitude of 150 miles (242 kilometers). Without gravity, the satellite's inertia would carry it off into space. Even with gravity, if the intended satellite goes too fast, it will eventually fly away. On the other hand, if the satellite goes too slowly, gravity will pull it back to Earth. At the correct orbital velocity, gravity exactly balances the satellite's inertia, pulling down toward Earth's center just enough to keep the path of the satellite curving like Earth's curved surface, rather than flying off in a straight line.

The orbital velocity of the satellite depends on its altitude above Earth. The nearer to Earth, the faster the required orbital velocity. At an altitude of 124 miles (200 kilometers), the required orbital velocity is a little more than 17,000 mph (about 27,400 kph). To maintain an orbit that is 22,223 miles (35,786 kilometers) above Earth, the satellite must orbit at a speed of about 7,000 mph (11,300 kph). That orbital speed and distance permit the satellite to make one revolution in 24 hours. Since Earth also rotates once in 24 hours, a satellite at 22,223 miles altitude stays in a fixed position relative to a point on Earth's surface. Because the satellite stays right over the same spot all the time, this kind of orbit is called "geostationary." Geostationary orbits are ideal for weather satellites and communications satellites.

a reply to: neutronflux

You ever state a clear and concise argument why you think geostationary /geosynchronous satellites do not have angular momentum when using the earth’s axis as a point of origin?

I didnt make that claim.

Also discussion still about stationary sats. You still dont know the difference?

a reply to: neutronflux

From the launch vehicle that placed it orbit like any other satellite. Except for correction thrust from a limited supply of fuel?

Didnt ask where it got the speed from.

I asked how it maintains that speed while being accelerated down to Earth by gravity.

Go ahead.

a reply to: InfiniteTrinity

Random example that has nothing to with this discussion.

Actually it does, since the discussion involves momentum.

How does it maintain it speed to maintain its trajectory through space if its in fact being pulled down by gravity,

I've explained that.

You see Phage, the other satellites have to fall around curvature to maintain a curved trajectory through space.

Satellites don't give a damn about Earth's rotation.

a reply to: Phage

Actually it does, since the discussion involves momentum.

I see so any random example involving momentum applies. Thank you Phage.

I've explained that.

Can you explain it again cause I cant find it.

Satellites don't give a damn about Earth's rotation.

I didnt make an argument about Earth rotation.

You see Phage, the other satellites have to fall around curvature to maintain a curved trajectory through space.

What part is about Earth rotation?

Can you answer the question Phage?

And this one,

How does it maintain it speed to maintain its trajectory through space if its in fact being pulled down by gravity.

originally posted by: InfiniteTrinity
a reply to: neutronflux

You ever state a clear and concise argument why you think geostationary /geosynchronous satellites do not have angular momentum when using the earth’s axis as a point of origin?

I didnt make that claim.

Also discussion still about stationary sats. You still dont know the difference?

Except geostationary/ geosynchronous satellites are only stationary to a fixed point on earth.

Back to Phage’s example...

If you are in a car moving at 20 mph and a car next to you is moving at 20 mph, does that mean the car next to you has no momentum?

That is exactly what is going on with a fixed point on earth in relationship to a geostationary/geosynchronous satellite.

But using the earth’s axis as a fixed point of reference shows the satellites do have angular momentum. Sorry.

a reply to: neutronflux

I didnt claim they that have no angular momentum.

When are you going to post something relevant?

How does it maintain its speed to maintain its trajectory through space if its in fact being pulled down by gravity.

Do you know what the other satellites need to do to solve that problem? They fall around the Earth.

a reply to: InfiniteTrinity

You

How does it maintain it speed to maintain its trajectory through space if its in fact being pulled down by gravity.

Do some of your own research....

How Satellites Stay in Orbit

www.universetoday.com...

So really, a satellites ability to maintain its orbit comes down to a balance between two factors: its velocity (or the speed at which it would travel in a straight line), and the gravitational pull between the satellite and the planet it orbits. The higher the orbit, the less velocity is required. The nearer the orbit, the faster it must move to ensure that it does not fall back to Earth.

And satellites don’t always win the battle between forces....

After fighting a losing battle against gravity, the European Space Agency's GOCE satellite has made splashdown somewhere in the south Atlantic Ocean, says the ESA.

www.csmonitor.com...!-Falling-GOCE-satellite-lands-in-the-Atlantic

a reply to: InfiniteTrinity

I see so any random example involving momentum applies.

You seemed to think that because a geostationary satellite does not move relative to Earth's surface it has no momentum. This is incorrect. I used an example (not random) to illustrate this.

Can you explain it again cause I cant find it.

Its speed is maintained because it is determined by the resultant vector of gravity and the satellite's momentum. The forces balance, the speed doesn't change.

I didnt make an argument about Earth rotation.

Your whole argument is based on the fact that an object in geostationary orbit has an angular velocity which matches Earth's rotation.

a reply to: neutronflux

So really, a satellites ability to maintain its orbit comes down to a balance between two factors: its velocity (or the speed at which it would travel in a straight line), and the gravitational pull between the satellite and the planet it orbits.

Yes Neutronflux, indeed. At the right velocity it keeps falling around the Earth and so it orbits.

Can you now explain how a geostationary sat does it? Since it doesnt fall around the Earth, which would maintain its velocity?

And satellites don’t always win the battle between forces....

Sigh, completely besides the point.

a reply to: InfiniteTrinity

I didnt claim they that have no angular momentum

You could have fooled me.....

The only correct frame of reference is the object Earth. In this frame of reference there is no angular momentum for the geostationary sat.

www.abovetopsecret.com...

How does it maintain its curved trajectory through space. An object has to fall around curvature to do that.

www.abovetopsecret.com...

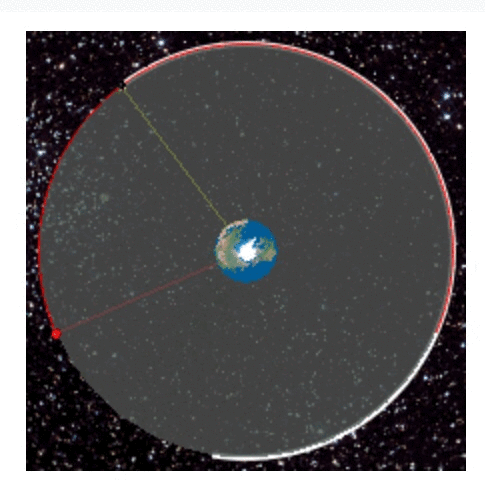
Because you cannot explain how it maintains a curved trajectory through space. You can keep posting those numbers like they mean anything but you have to explain HOW IT IS POSSIBLE that it keeps flying in this curved trajectory through space with a speed of 1.91 mi/s, 22,236 mi above a point on the equator.

Geostationary orbits debunked.

www.abovetopsecret.com...

Again. This would be much easier if you stated in a clear and concise by geostationary/ geosynchronous satellites are not in orbit.

Vs just being intellectually dishonest in general, and keep moving the goalposts.

a reply to: InfiniteTrinity

Since it doesnt fall around the Earth, which would maintain its velocity?

You should actually read what you quoted.

a reply to: InfiniteTrinity

You

Can you now explain how a geostationary sat does it? Since it doesnt fall around the Earth, which would maintain its velocity?

Again...

From the earth’s surface. A geostationary satellite appears to be “fixed”. But that same satellite is traveling the “curve” of earth’s gravity well. Orbiting around the center of earth’s gravity well. If you use the earth’s axis as a fixed point of origin, you can solve for the angular momentum of a geostationary / geosynchronous satellite.

You

You guys are hilarious. You keep posting the same dumb contradiction I debunked about 40 times now.

The only thing dumb is that you cannot realize the earth’s rotation has nothing to do with earth’s gravity well. And it’s ”dumb” that you think a satellite cannot be in orbit because it is at a height and speed that matches the earth’s rotation.


First off, the earth’s rotation has nothing to do with the ability of a satellite to orbit. In reality, a satellite does not orbit the center of the earth. A satellite orbits the center of Earth’s gravity well. An orbiting satellite could careless if the earth was rotating, and at what speed.

What is gravity?

spaceplace.nasa.gov...

Second: Why can you not get through your head a geostationary/ geosynchronous still obits the earth. It’s just at a speed that keeps it above a specific point and n earth. But a geostationary / geosynchronous satellite is still traveling around earth’s gravity well.

Geostationary orbit

en.m.wikipedia.org...

A geostationary orbit, often referred to as a geosynchronous equatorial orbit[1] (GEO), is a circular geosynchronous orbit 35,786 km (22,236 mi) above Earth's equator and following the direction of Earth's rotation. An object in such an orbit appears motionless, at a fixed position in the sky, to ground observers. Communications satellites and weather satellites are often placed in geostationary orbits, so that the satellite antennas (located on Earth) that communicate with them do not have to rotate to track them, but can be pointed permanently at the position in the sky where the satellites are located. Using this characteristic, ocean-color monitoring satellites with visible and near-infrared light sensors (e.g. GOCI) can also be operated in geostationary orbit in order to monitor sensitive changes of ocean environments.

a reply to: neutronflux

I said,

In this frame of reference

Try to read.

a reply to: InfiniteTrinity

And you were wrong. Again.
The satellite has momentum. Frame of reference is irrelevant to the discussion.

originally posted by: InfiniteTrinity
a reply to: neutronflux

I said,

In this frame of reference

Try to read.

No, your moving the goalposts.

The actual frame of reference in all reality is the center of earth’s gravity well which a geostationary/ geosynchronous circles. Again, a satellite could care less at what speed the earth rotates. The satellite cares about the center of the gravity well.

a reply to: Phage

You seemed to think that because a geostationary satellite does not move relative to Earth's surface it has no momentum.

No Phage you seem to think that. I didnt say that.

Its speed is maintained because it is determined by the resultant vector of gravity and the satellite's momentum. The forces balance, the speed doesn't change.

In order to balance the forces it has to keep falling around curvature Phage, just like the other sats.

Your whole argument is based on the fact that an object in geostationary orbit has an angular velocity which matches Earth's rotation.

You responded to this,

You see Phage, the other satellites have to fall around curvature to maintain a curved trajectory through space.

Respond to it.

a reply to: neutronflux

Again, a satellite could care less at what speed the earth rotates.

Didnt make such a claim. Can you say something relevant.