A New Relativistic Quantum Mechanics

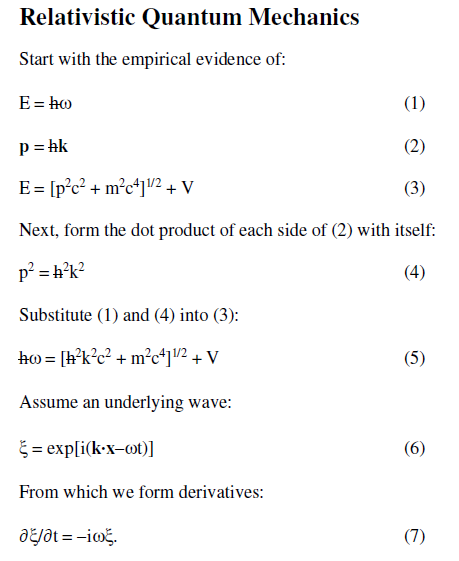
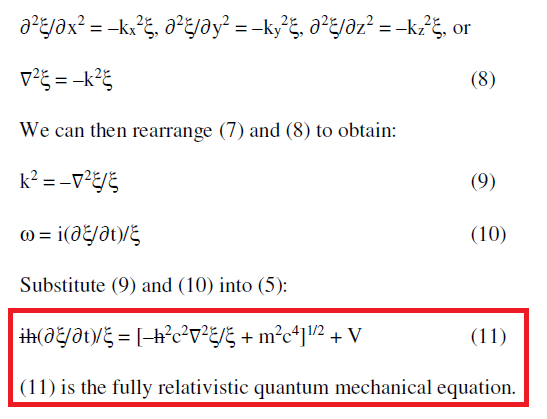
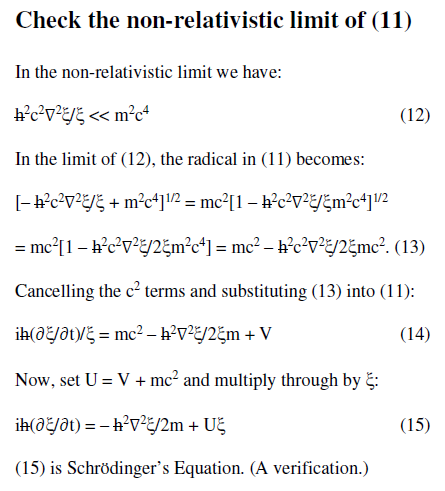
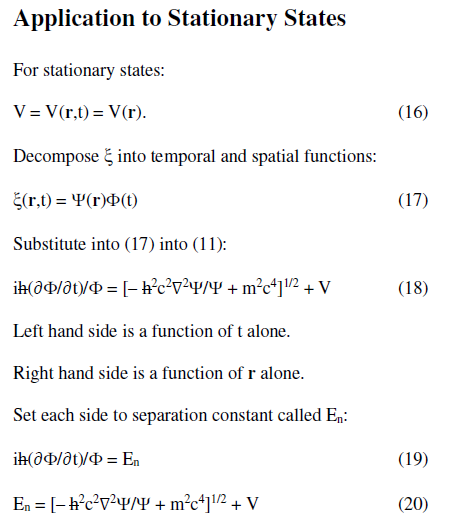
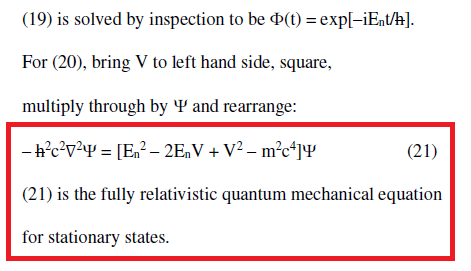
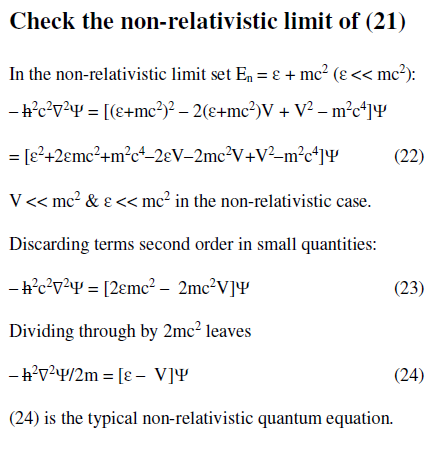
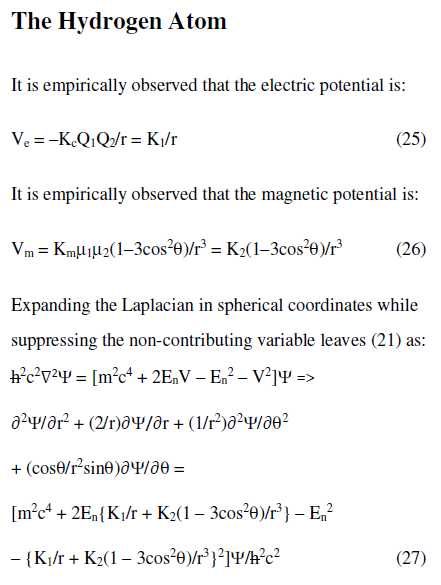
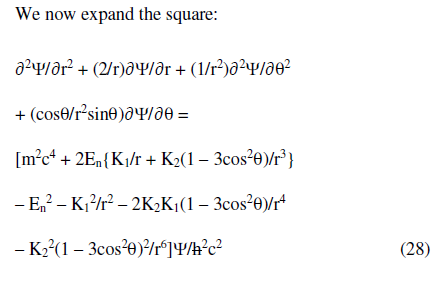
For roughly the past two years I've been trying to come up with a relativistic quantum mechanical solution to the two body problem. Above I extract equations from a paper I wish to publish, and I augment the equations with some sparse notes.

My approach is similar to Klein-Gordon, but certainly not the same. It is quite simple, in that a few empirical observations are stated and then a very simple assumption of an underlying wave is proposed. From there the math is quite straightforward. As shown above, the approach reduces to the standard non-relativistic forms in that limit.

The theory above is non-local, and so is more at home with Lorentz than Einstein. It is my guess that this may be why I have never seen this approach done before. In graduate school there was a mention of something similar to Equation (11), with the comment made that the Laplacian inside the radical was one reason to set this approach aside, and that the non-local nature was another reason to not pursue this path.

This approach does have advantages though. As seen above, it allows for an equation for stationary states.

I have not put in the Dirac delta function portion of the spin-spin magnetic potential, as I am unclear whether it is needed in this approach. (If elementary particles have a hard core, that may not matter.)

My goal was to find masses for leptons via my ABC Preon Model. However after a year of struggling with Equation (28) above, I was unable to find a solution. Equation (28) can likely be treated with numeric methods, but I am not expert on such, and would therefore appreciate any pointers to references that might help.

Yep...no...I mean of course...hold on a minute...What?

My level of knowledge just took body blows, and I don't even know WHY!!!

You mis-stated a variable. I'll tell you which one for ten bucks.

a reply to: Jonjonj

No. No.

You were a bit amiss in your post.

It should have been . . .

Saaaaaaaaaaaaaayyyyyyyyyyyyyyyyyyyy WHHHHHHHHHHUUUUUUUUTTTTTTTTTT???????????!!!!!!!!!!!

I think that MIGHT have been a bit closer to the reality of the post you were referring to.

[LOL]

I don't believe anything actually has a "hard core".

a reply to: delbertlarson

As a mathematics with a limited background in physics, this post was quite interesting, and I wish you the best of luck.
Though I am sure you are aware, Equation (28) is a linear 2-degree partial differential equation whose input is a very complicated sinusoidal(wave) function.

Here is a Link to a pdf on how to solve partial differential equations It is from Cornell university, I think and; hopefully, it might give you an idea on how to treat it though plotting it on something like Mathematica or Maple then finding numerical solutions.

Last, if I remember correctly, and I might not, there should be 16 solutions to the equation (28), each being functions that define the motion and spin along certain axis as it reacts to the curvature of spacetime, but half of those solutions are just the inverse curve, so I think you are free to ignore them.

Hope I was in anyway helpful, and good luck with you research.

originally posted by: delbertlarson
Equation (28) can likely be treated with numeric methods, but I am not expert on such, and would therefore appreciate any pointers to references that might help.

Your equation is too complicated.

This means you can't get any useful "insights" into the nature of the underlying phenomena.

You can solve all these complicated equations using numerical methods, if you have "boundary conditions" for some region of space specified. You can even use "FEA" Finite Element Analysis, otherwise called "FEM" Finite Element Methods, to get a picture of the region of space governed by your complicated equation. Again, you have to specify boundary conditions and/or initial conditions.

But, at the end of the day, Physics is about trying to find the "simple" explanations for phenomena, because, otherwise, we get lost in the complexity of the mathematics, and nothing useful can be obtained from the effort.

a reply to: AMPTAH

While I agree with the idea that initial conditions are necessary to solve such mathematical equations, the equation (28) is no more complicated than the standard Einstein-Field Equations which govern realistic physics.

Here is a detailed pdf on Einstein-Field Equations.

Our simple observations are limited by our abilities to make measurements and the fact that we are always a part of the system we study, yet Penrose has suggested that we can make observations on the topological nature of spacetime, which could prove that spacetime is "twisted" space. This twisted space theory could use complex manifolds to show that quantum gravity might be best seen as a correcting factor that explains away the measurement paradox.

To prove this one would have to make a mathematical correction to the original Field Equations so that they could provide information on the atomic scale that scientists can make predictions about events that would occur during tests at the particle accelerator labs.

a reply to: delbertlarson
Quite interesting post. But how this imroves the taste of my coffee?

Joke aside, I'm always happy to see brainy ATSrs around.

a reply to: delbertlarson

Well for what it is worth, setting the magnetic potential to zero will give you a second-order ode.

Mathematica even produces a solution, although not a pretty one.

Link:
www.wolframalpha.com/input/?i=DSolve%5By%27%27%5Br%5D+%2B+2+%2F+r+*+y%27%5Br%5D+%3D%3D+-((a+-+b+%2F+r)%5E2+-+c)+*+y%5Br%5D,+y%5Br%5D,+r%5D

originally posted by: moebius
a reply to: delbertlarson

Well for what it is worth, setting the magnetic potential to zero will give you a second-order ode.

Mathematica even produces a solution, although not a pretty one.

Link:
www.wolframalpha.com/input/?i=DSolve%5By%27%27%5Br%5D+%2B+2+%2F+r+*+y%27%5Br%5D+%3D%3D+-((a+-+b+%2F+r)%5E2+-+c)+*+y%5Br%5D,+y%5Br%5D,+r%5D

The standard procedure, if you have a closed solution for part of the problem, is to use "perturbation" theory to calculate the desired parameters for the full problem. So, put a small scaling parameter in front of the magnetic potential and treat it as a small perturbation to the system, to calculate what you want. Maybe you'll get somewhere.

SHHHH....thats it. The perfect formula for obtaining the winning lottery ticket next Wednesday. I am not sharing with anyone.

The mathematics is totally wrong. One cannot assume the relativistic wave function is a plane wave (equ. 6), work out what equation it satisfies and then claim that the equation is the general relativistic wave equation of a spinless particle! The general solution of a scalar wave equation with Lorentz invariance that describes a spinless particle moving in a potential field V is NOT a plane wave. That's the form of a FREE relativistic particle moving in the ABSENCE of an interaction potential V. It is mathematically wrong to work back from a particular solution in which V = 0 to an equation that is supposed to hold in the general case where V ≠ 0. The mathematical howlers in the analysis would not get past any physical journal editor. What has been misinderstood is that you can use equ. 1 with E^2 = p^2c^2 + (mc^2)^2 and equ. 6 only when the particle is free (V = 0). The relativistic wave equation in a potential V is well-known and can be found here:
en.wikipedia.org...

a reply to: Jonjonj

a reply to: bbarkow

a reply to: BO XIAN

a reply to: GmAndre

a reply to: Gothmog

Thanks so much for the light-hearted comments. Most often I simply get told I am wrong. What you write is like a breath of fresh air.

originally posted by: Rosinitiate
I don't believe anything actually has a "hard core".

I have always believed that a point-like nature for entities was not likely, as that leads to infinities. So I believe it will either be a hard core, or a soft one that can be penetrated, but ultimately I believe things will have some finite extent.

originally posted by: hubrisinxs
a reply to: delbertlarson

Here is a Link to a pdf on how to solve partial differential equations It is from Cornell university, I think and; hopefully, it might give you an idea on how to treat it though plotting it on something like Mathematica or Maple then finding numerical solutions.

Hope I was in anyway helpful, and good luck with you research.

Thanks for the link, and the good wishes. I plan to look into the link you sent over the next week. Perhaps it will help!

originally posted by: AMPTAH

originally posted by: delbertlarson
Equation (28) can likely be treated with numeric methods, but I am not expert on such, and would therefore appreciate any pointers to references that might help.

Your equation is too complicated.

This means you can't get any useful "insights" into the nature of the underlying phenomena.

You can solve all these complicated equations using numerical methods, if you have "boundary conditions" for some region of space specified. You can even use "FEA" Finite Element Analysis, otherwise called "FEM" Finite Element Methods, to get a picture of the region of space governed by your complicated equation. Again, you have to specify boundary conditions and/or initial conditions.

But, at the end of the day, Physics is about trying to find the "simple" explanations for phenomena, because, otherwise, we get lost in the complexity of the mathematics, and nothing useful can be obtained from the effort.

I have always believed that the important simplicity to seek was in the underlying modeling, and not in the equations themselves. Of course in modern physics the underlying modeling has been largely simplified by ignoring it altogether, but I think that is a mistaken path. I would say though, that my equations really aren't too complicated at all. It is the equations with the red boxes around them that are the fundamental ones, and they are just slightly more complicated than the non-relativistic versions. It is only when you put in the rather complicated potentials and expand the Laplacian that things get beastly - but if that is how nature is, than that is what we must solve.

As for the boundary conditions - yes you make a good point. The boundary condition on the polar angle will come from the fact that it must be repetitive, and one boundary condition on r is that it go to zero as it gets large. The boundary conditions at small r, for both the magnitude and the magnitude of its first derivative, will lead to different solutions I expect, and it is those different solutions that will be what we are after.

originally posted by: moebius
a reply to: delbertlarson

Well for what it is worth, setting the magnetic potential to zero will give you a second-order ode.

Mathematica even produces a solution, although not a pretty one.

Link:
www.wolframalpha.com/input/?i=DSolve%5By%27%27%5Br%5D+%2B+2+%2F+r+*+y%27%5Br%5D+%3D%3D+-((a+-+b+%2F+r)%5E2+-+c)+*+y%5Br%5D,+y%5Br%5D,+r%5D

Thanks. I will look into this next week. For Hydrogen, we need the magnetic potential, but it is possible that preons will not.

originally posted by: AMPTAH

originally posted by: moebius
a reply to: delbertlarson

Well for what it is worth, setting the magnetic potential to zero will give you a second-order ode.

Mathematica even produces a solution, although not a pretty one.

Link:
www.wolframalpha.com/input/?i=DSolve%5By%27%27%5Br%5D+%2B+2+%2F+r+*+y%27%5Br%5D+%3D%3D+-((a+-+b+%2F+r)%5E2+-+c)+*+y%5Br%5D,+y%5Br%5D,+r%5D

The standard procedure, if you have a closed solution for part of the problem, is to use "perturbation" theory to calculate the desired parameters for the full problem. So, put a small scaling parameter in front of the magnetic potential and treat it as a small perturbation to the system, to calculate what you want. Maybe you'll get somewhere.

Thanks. However my goal here is to find a non-perturbative approach, since the relativistic "correction" is actually dominant. Hence I was thinking of numerical methods to solve this first.

originally posted by: micpsi
The mathematics is totally wrong. One cannot assume the relativistic wave function is a plane wave (equ. 6), work out what equation it satisfies and then claim that the equation is the general relativistic wave equation of a spinless particle! The general solution of a scalar wave equation with Lorentz invariance that describes a spinless particle moving in a potential field V is NOT a plane wave. That's the form of a FREE relativistic particle moving in the ABSENCE of an interaction potential V. It is mathematically wrong to work back from a particular solution in which V = 0 to an equation that is supposed to hold in the general case where V ≠ 0. The mathematical howlers in the analysis would not get past any physical journal editor. What has been misinderstood is that you can use equ. 1 with E^2 = p^2c^2 + (mc^2)^2 and equ. 6 only when the particle is free (V = 0). The relativistic wave equation in a potential V is well-known and can be found here:
en.wikipedia.org...

I don't believe my mathematics is wrong at all, and if you do see a point where I have made an actual math error, please let me know.

However, your discussion about what you feel is wrong appears not to be mathematical, but rather interpretive. And I do agree with you that perhaps the rules that govern a free particle (when it is a plane wave) may no longer hold up once it is under the influence of a potential. My guess that the same relations do pertain is just that - a guess. But please note it is the same guess made in the original (non-relativistic) Schrödinger Equation. I do not know if that guess will be valid in nature relativistically, and the only way to really know is to see if the resultant analysis leads to predictions that are in agreement with experiment. And to do that, Eq. (28) needs to be solved. If after solving it one gets the Lamb shift, then my guess is probably sound. ( I do know there is a solution for the Lamb shift already that uses perturbation theory - my effort is to obtain a non-perturbative solution that can be used on other problems.)

As for your link, if you follow it yourself and take a look at the "Derivation" section you will see something very similar to my equation 11 (without the V) . It goes on to explain that my approach was set aside due to non-locality concerns as well as the cumbersomeness of having the Laplacian inside of the radical. (Note that I have mentioned these issues, as well as Klein-Gordon, in the OP.)

You are of course correct that my work will likely face hurdles at editorial desks. This issue was a hot topic 80 to 90 years ago, but many consider it a closed topic now. Nonetheless, I see no reason why my math is inferior to anyone else's math, as long as both are mathematically correct. Interpretations of course are something else entirely, but they have much less weight scientifically. What has weight scientifically is whether the math is right (the addition, subtraction, taking derivatives, etc. must be right) and whether the resultant equations agree with experiment. Again, if you see any actual math error, please let me know.

I do wish to thank you for your comments, as they do point out areas where I can likely improve the presentation of the eventual paper in order to make these issues more clear there as well.