It looks like you're using an Ad Blocker.
Please white-list or disable AboveTopSecret.com in your ad-blocking tool.
Thank you.
Some features of ATS will be disabled while you continue to use an ad-blocker.
share:
The Singular Primordial Preon Theory
Introduction
First of all: what are preons? In layman's terms, preons are tiny particles which are speculated to exist inside what we normally call "elementary particles". Just as objects are made of atoms, and atoms are made of particles, particles would be made of preons. We think that perhaps, just pehaps, all particles (up quark, down quark, charm quark, strange quark, top quark, bottom quark, up antiquark, down antiquark, charm antiquark, strange antiquark, top antiquark, bottom antiquark, electron, positron, muon, antimuon, tauon, antitauon, electron neutrino, muon neutrino, tauon neutrino, and gauge bosons) are made of less than four types of preons (in most models, usually two or three). And since "elementary" particles were observed to decay, then we think that these particles would actually be made of truly elemental preons, which would simply be exchanged during decay, causing the particle they compose to change properties. Those of us who propose the existence of preons are currently in minority in the scientific community. But this did not stop many scientists to submit preon models (in fact three major ones are around right now: the Rishon model, the ABC model, and Fredriksson's model), and even more scientists to consider the possibility.
The SPP Theory is my own preon model. I believe it is superior to all of those before mine in the sense that not only does it have far-reaching predictive powers, but above all it speculates the existence of only one preon (which can take two forms, that is, "a" or "b"). May it be the soil of Earth or the light of the farthest star, the depths of the seas or the nebulae floating in space, your atoms or that of the oldest dinosaur - everything sharing the same and singular master particle. Here I will present its third chapter, which I call Pillar Three. But before I dive in deeper, let us refresh our memories about the two first chapters.
******
The First Pillar of the theory
After I have gathered observational data related to all forms of decay of all known particles, I proposed some years ago that all elementary particles were in fact composite. Proceding with deduction, and basing myself solely upon observed decay data, I ventured the conclusion that all modes of decay could be explained by the simple exchange of preons. Furthermore, I noted that evidences most likely pointed to the existence of only two kinds of preons, which I named "a" and "b"; and that all "elementary" particles were most likely composed of six of such preons.
aaaaaa = positron
aaaaab = up quark
aaaabb = down antiquark
aaabbb = neutrino, bosons
aabbbb = down quark
abbbbb = up antiquark
bbbbbb = electron
With this model it can be easily shown that decaying elementary particles are neither destroyed nor created - they are merely exchanging preons. This process explains anything, such as the mechanism behind annihilation...
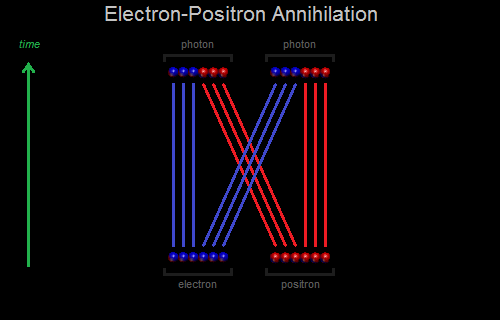
...the neutron's decay, pion decay, etc.
This sums up the nature of the Theory's First Pillar (which has been covered in more details here: www.abovetopsecret.com...). The First Pillar focuses mainly on modeling the electric charge of all particles and their interactions during decay.
But particles have way more properties than just electric charges. So this is where Pillar Two comes in. Devised only two months after Pillar One, Pillar Two is a bit more speculative.
Pillar Two
For starters, Pillar Two speculates that the six preons in an elementary particle are placed in the fashion of an octahedron:
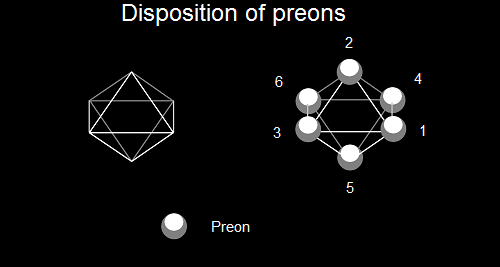
Also, preons are speculated to have two faces, as such:
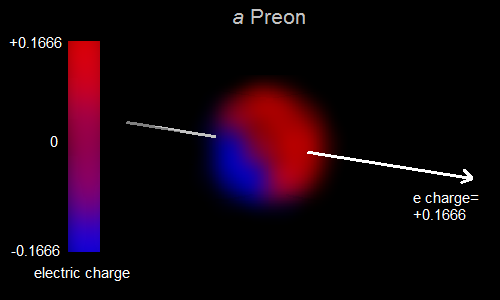
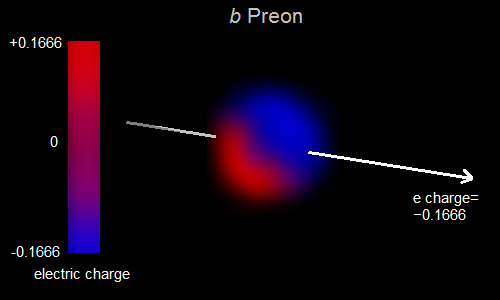
Such speculations enable the theory to propose an explanation for most other properties of particles. This includes:
-The three generations of fermions:
Elementary particles are tiny octahedrons. If the particle's momentum direction is aligned with one of the octahedron's vertex, this makes the particle a Generation 1; if it is aligned with an edge, this makes the particle a Generation 2; and if it is aligned with a face, this makes the particle a Generation 3.
-The spin of all matter and energy particles:
If the particle, composed of six preons, has identical top-most or bottom-most preons, the particle is a 1/2 spin particle (its lateral value changes after 1/2 of a spin); and if the particle's top-most preon is different than the bottom-most, the particle is a 1 spin particle (its lateral value changes not during a spin).
-The antiparticles of all matter particles and CT-symmetry:
Since preons have a face of opposed charge facing the opposite direction than the preon's momentum, then a composite particle going back in time would be indistinguishable from its antiparticle.
-the kaon oscillation mystery:
A beam of kaon is observed to periodically become its own antimatter equivalent. My theory solves the mystery by modeling the kaon as a rotating system - as it travels, the kaon reverses itself, and the preons inside show their opposite face - making the kaon's quarks show up as antiquarks.
-the anomalous magnetic moment of the muon:
Yet another mystery solved by the theory. Since muons are octahedrons whose edge is aligned with their momentum direction, then their preon's charge are offset relative to electrons (which have the same preonic composition but whose preons are actually aligned with the particle's momentum direction).
-The neutrino's oscillation into another flavour:
Since the mass of a particle can be shown to correlate the alignment of its octahedron's axis, then the oscillation of a neutrino is caused by a precession movement of the octahedron's axis during the particle's travel.
But yet a few other properties of particles were still left unexplained by the model (more details about Pillar Two here: www.abovetopsecret.com...). And this is why I took two years and came up with Pillar Three - the actual topic of this thread).
Introduction
First of all: what are preons? In layman's terms, preons are tiny particles which are speculated to exist inside what we normally call "elementary particles". Just as objects are made of atoms, and atoms are made of particles, particles would be made of preons. We think that perhaps, just pehaps, all particles (up quark, down quark, charm quark, strange quark, top quark, bottom quark, up antiquark, down antiquark, charm antiquark, strange antiquark, top antiquark, bottom antiquark, electron, positron, muon, antimuon, tauon, antitauon, electron neutrino, muon neutrino, tauon neutrino, and gauge bosons) are made of less than four types of preons (in most models, usually two or three). And since "elementary" particles were observed to decay, then we think that these particles would actually be made of truly elemental preons, which would simply be exchanged during decay, causing the particle they compose to change properties. Those of us who propose the existence of preons are currently in minority in the scientific community. But this did not stop many scientists to submit preon models (in fact three major ones are around right now: the Rishon model, the ABC model, and Fredriksson's model), and even more scientists to consider the possibility.
The SPP Theory is my own preon model. I believe it is superior to all of those before mine in the sense that not only does it have far-reaching predictive powers, but above all it speculates the existence of only one preon (which can take two forms, that is, "a" or "b"). May it be the soil of Earth or the light of the farthest star, the depths of the seas or the nebulae floating in space, your atoms or that of the oldest dinosaur - everything sharing the same and singular master particle. Here I will present its third chapter, which I call Pillar Three. But before I dive in deeper, let us refresh our memories about the two first chapters.
******
The First Pillar of the theory
After I have gathered observational data related to all forms of decay of all known particles, I proposed some years ago that all elementary particles were in fact composite. Proceding with deduction, and basing myself solely upon observed decay data, I ventured the conclusion that all modes of decay could be explained by the simple exchange of preons. Furthermore, I noted that evidences most likely pointed to the existence of only two kinds of preons, which I named "a" and "b"; and that all "elementary" particles were most likely composed of six of such preons.
aaaaaa = positron
aaaaab = up quark
aaaabb = down antiquark
aaabbb = neutrino, bosons
aabbbb = down quark
abbbbb = up antiquark
bbbbbb = electron
With this model it can be easily shown that decaying elementary particles are neither destroyed nor created - they are merely exchanging preons. This process explains anything, such as the mechanism behind annihilation...

...the neutron's decay, pion decay, etc.
This sums up the nature of the Theory's First Pillar (which has been covered in more details here: www.abovetopsecret.com...). The First Pillar focuses mainly on modeling the electric charge of all particles and their interactions during decay.
But particles have way more properties than just electric charges. So this is where Pillar Two comes in. Devised only two months after Pillar One, Pillar Two is a bit more speculative.
Pillar Two
For starters, Pillar Two speculates that the six preons in an elementary particle are placed in the fashion of an octahedron:

Also, preons are speculated to have two faces, as such:


Such speculations enable the theory to propose an explanation for most other properties of particles. This includes:
-The three generations of fermions:
Elementary particles are tiny octahedrons. If the particle's momentum direction is aligned with one of the octahedron's vertex, this makes the particle a Generation 1; if it is aligned with an edge, this makes the particle a Generation 2; and if it is aligned with a face, this makes the particle a Generation 3.
-The spin of all matter and energy particles:
If the particle, composed of six preons, has identical top-most or bottom-most preons, the particle is a 1/2 spin particle (its lateral value changes after 1/2 of a spin); and if the particle's top-most preon is different than the bottom-most, the particle is a 1 spin particle (its lateral value changes not during a spin).
-The antiparticles of all matter particles and CT-symmetry:
Since preons have a face of opposed charge facing the opposite direction than the preon's momentum, then a composite particle going back in time would be indistinguishable from its antiparticle.
-the kaon oscillation mystery:
A beam of kaon is observed to periodically become its own antimatter equivalent. My theory solves the mystery by modeling the kaon as a rotating system - as it travels, the kaon reverses itself, and the preons inside show their opposite face - making the kaon's quarks show up as antiquarks.
-the anomalous magnetic moment of the muon:
Yet another mystery solved by the theory. Since muons are octahedrons whose edge is aligned with their momentum direction, then their preon's charge are offset relative to electrons (which have the same preonic composition but whose preons are actually aligned with the particle's momentum direction).
-The neutrino's oscillation into another flavour:
Since the mass of a particle can be shown to correlate the alignment of its octahedron's axis, then the oscillation of a neutrino is caused by a precession movement of the octahedron's axis during the particle's travel.
But yet a few other properties of particles were still left unexplained by the model (more details about Pillar Two here: www.abovetopsecret.com...). And this is why I took two years and came up with Pillar Three - the actual topic of this thread).
edit on 29-10-2015 by swanne because: (no reason given)
******
Pillar Three (the actual topic of this thread)
Pillar Three is highly speculative in nature. But it makes very powerful propositions. And these are,
-composite particles inclination correlation with generation mass;
-composite particles correlation with charge radius;
-why are W/Z bosons virtual, and why are they so massive;
-preons as a cause for observed colour charges;
-offset in decay caused by composite particle offset;
-neutrino as trigger for decay;
-preons as dark matter candidates;
-only one "master" preon.
Let us explore each of these propositions in depth.
Composite particles inclination correlation with generation mass
The electron has a mass of one electron (obviously). The muon is 206.768284 times the electron, and the tauon is 3477.15015 times the electron.
Now. 206.768284^0.5 gives 14.3794396. Strangely enough, 3477.15015^0.333 gives 15.1498319. It seems that distribution of mass across the three generations are actually about equivalent, but a factor is squaring the value in practice. I believe this could be smoking gun evidences that the direction of octahedrons alignment is directly responsible for the resulting mass.
To take the example of the electron/muon/tauon: since the electron is of Generation 1, then it is an octahedron perfectly aligned with its momentum.
But the muon is from Generation 2, and it travels edge-first - thus they are misaligned on a lateral direction, providing an entire new dimension to the mass value. Analogy: a 2 inches line with one more dimension becomes a 4 square inches square.
And since the tauon is from Generation 3, it travels face-first, and its octahedron's direction is offset on two directions laterally (both horizontally and vertically), providing even more dimensions to the inital mass value. Analogy: a 2 inches line with two more dimensions becomes a 8 cube inches cube.
Composite particles correlation with charge radius
Since the model predicts that particles are octahedrons whose alignements determine generation, then as a side effect it also predicts that particles can have different electroweak cross-section size depending on the generation. To take the neutrino as an example: In the model the electron neutrino is travelling vertex-first, which causes its cross-section to be significantly larger than that of the tauon neutrino.
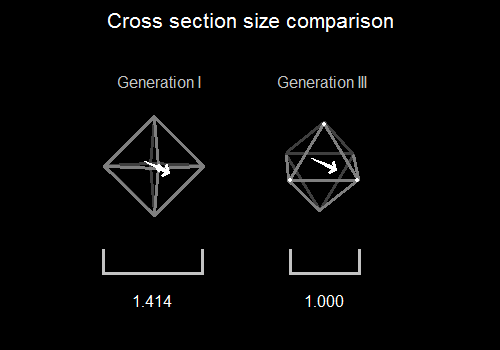
My model predicts the electron neutrino's electroweak cross-section radius to be 1.41 that of the tauon neutrino. Which is quite close to observations, as they recently measured the electron neutrino's radius to be about 1.78 that of the tauon neutrino (square root of "3.2 x 1 nanobar").
Pillar Three (the actual topic of this thread)
Pillar Three is highly speculative in nature. But it makes very powerful propositions. And these are,
-composite particles inclination correlation with generation mass;
-composite particles correlation with charge radius;
-why are W/Z bosons virtual, and why are they so massive;
-preons as a cause for observed colour charges;
-offset in decay caused by composite particle offset;
-neutrino as trigger for decay;
-preons as dark matter candidates;
-only one "master" preon.
Let us explore each of these propositions in depth.
Composite particles inclination correlation with generation mass
The electron has a mass of one electron (obviously). The muon is 206.768284 times the electron, and the tauon is 3477.15015 times the electron.
Now. 206.768284^0.5 gives 14.3794396. Strangely enough, 3477.15015^0.333 gives 15.1498319. It seems that distribution of mass across the three generations are actually about equivalent, but a factor is squaring the value in practice. I believe this could be smoking gun evidences that the direction of octahedrons alignment is directly responsible for the resulting mass.
To take the example of the electron/muon/tauon: since the electron is of Generation 1, then it is an octahedron perfectly aligned with its momentum.
But the muon is from Generation 2, and it travels edge-first - thus they are misaligned on a lateral direction, providing an entire new dimension to the mass value. Analogy: a 2 inches line with one more dimension becomes a 4 square inches square.
And since the tauon is from Generation 3, it travels face-first, and its octahedron's direction is offset on two directions laterally (both horizontally and vertically), providing even more dimensions to the inital mass value. Analogy: a 2 inches line with two more dimensions becomes a 8 cube inches cube.
Composite particles correlation with charge radius
Since the model predicts that particles are octahedrons whose alignements determine generation, then as a side effect it also predicts that particles can have different electroweak cross-section size depending on the generation. To take the neutrino as an example: In the model the electron neutrino is travelling vertex-first, which causes its cross-section to be significantly larger than that of the tauon neutrino.

My model predicts the electron neutrino's electroweak cross-section radius to be 1.41 that of the tauon neutrino. Which is quite close to observations, as they recently measured the electron neutrino's radius to be about 1.78 that of the tauon neutrino (square root of "3.2 x 1 nanobar").
Why are W/Z bosons virtual, and why are they so massive
In most preon models, the notion of a weak force boson is often dismissed. This is because most preon models are not capable of accountig for the existence of the W/Z boson. But my model not only acknowledges the existence of W/Z bosons, it goes on to explains why W/Z bosons exist only for a very short time, and, additionally, it explains why they are so massive compared to other bosons.
We already know that preons have (individually) more mass than the particle they compose. Now to understand why W/Z bosons have such a high mass, one needs only to observe the process of, say, neutron decay (which emits a W- boson) more closely. Here is an animation of the phenomenon:
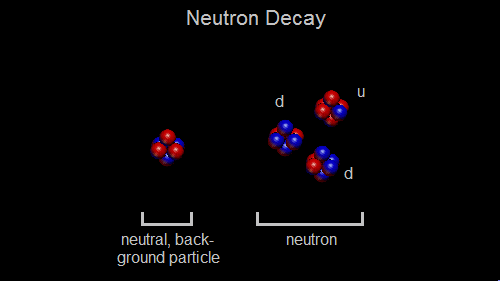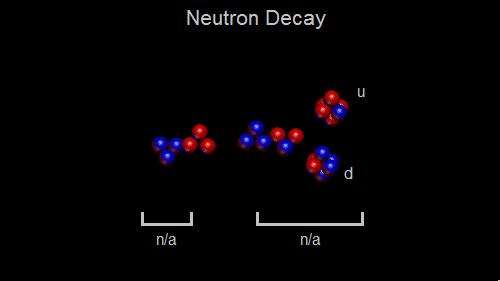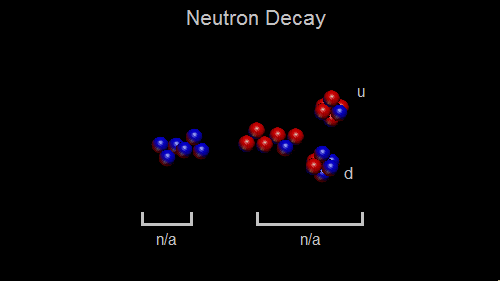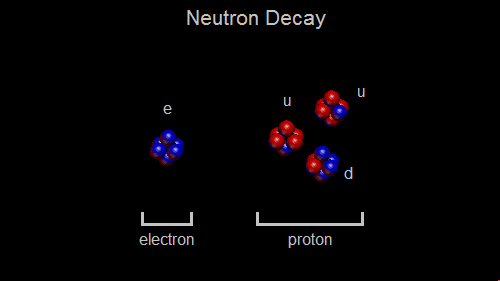
Notice that at one point during the exchange of preons, the three a preons from the neutral particle (I will explain its identity later) briefly meet the three b preons from the down quark. This meeting point exist only inside a very small window of time, after which the two groups of preons continue on their way to their target particles. This meeting point, this moment where the two groups of preon are right next to each other, constitutes a particle in itself - a particle which is dissolved as soon as it is created. A virtual particle - the W- boson. Now why is it so massive? Well, let us see - it is not composed of a group of six preons as any other bosons, but actually of two groups of three preons. Since normal, six-preons bosons are so relatively light, and since isolated preons are so insanely massive, then the existence of four groups of three preons (do not forget that the parent particles too have been reduced to three preons each) easily explains why the event is measured to have such a high mass.
Preons as a cause for observed colour charges
Why do quarks only bind with quarks and antiquarks, but leptons and bosons can neither bind with one another nor with quarks? The answer to this very question lies within the preon sequence inside the particles. Quite a bit like DNA, the two kind of preons can form sequences inside particles. For instance, the down quark must be composed of four "a" preons and two "b" preons, but many arrangements of these can come up, such as aabbbb, abbbba, bbbbaa, ababbb, abbabb, etc. These sequences actually seem to have an important function inside particle - that is, the attribution of colour charges (such as red, green, antiblue, etc.).
Three simple laws are governing the sequencing of preons and attribution of colours:
1. Group-particles whose appearance is a rotation, on the spin axis, of the original structure are of the same colour.
2. If there are more than 1 preon in minority, they must touch each other inside the particle (for it is by groups that they are exchanged).
3. In the event of preon equality (three a + three b), the three preons must both touch each other and one of them be present on the side of the particle at at least one point during the structure's rotation (for it is by groups and by the side that they are exchanged).
So let us now check all possible sequences.
Electrons have only one sequence possible:
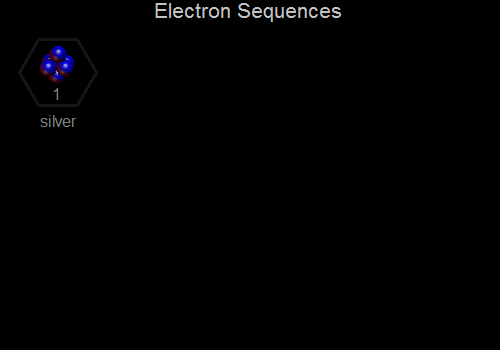
Thus it has only one possible colour, a colour which is incompatible with all others - I like to call it the silver colour (antisilver for the positron, which is bbbbbb). Since it has zero interaction with other colours, it can be said that in practice, the electron has zero colour.
Up quarks have quite a more diverse sequences range:
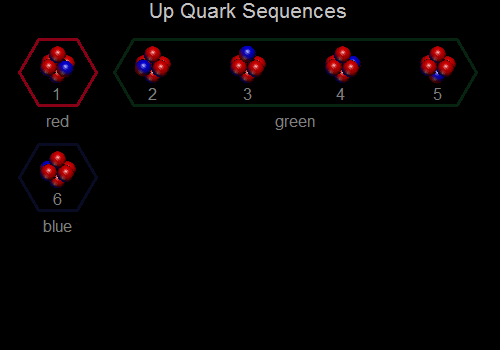
Out of the six permutations, Law 1 states that Sequence 2 to 5 are equivalent, and constitutes one colour - green. Sequence 1 constitues a second colour - red. Finally, Sequence 6 constitutes a third colour - blue.
Down quarks sequencing confirms the fact that quarks have no more, no less than three colours:

Once again here Sequence 13 to 15 are violating Law 2 and thus disqualify, for they form an exotic matter. From the twelve remaining permutations, Sequence 1 to 4 are equivalent by Law 1, Sequence 5 to 8 are also equivalent by Law 1, and Sequence 9 to 12 are also equivalent by Law 1.
Once again: red, green and blue.
Since quarks can form three colours, then the same applies for antiquarks, and thus we can add to that three other colours - which we would call antired, antigreen and antiblue.
Neutrinos sequencing is distinguishable from bosons sequencing in that neutrinos must have have similar top-most and bottom-most preons at least 1/2 of the time. This gives neutrinos these permutations:
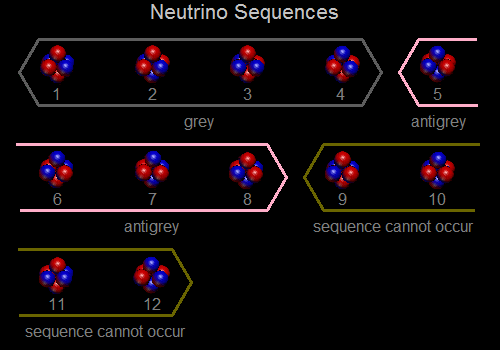
Law 3 rules out Sequence 9 to 12. Law 1 indicates that Sequence 1 to 4 are equivalent, forming one colour which I like to call grey. Surprisingly, Law 1 also states that there is a second set, namely Sequence 5 to 8, seemingly forming a corresponding anticolour. I believe that this is an indication that the model is predicting an antiparticle for the neutrino.
Boson sequencing froms eight permutations:
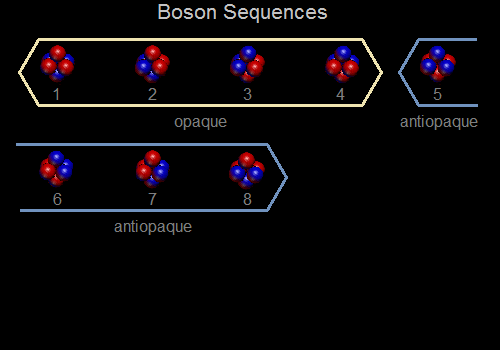
Once again, Sequence 1 to 4 are equivalent by Law 1, forming a colour I like to call opaque, and once again Law 1 also states that there is a set of a corresponding anticolour, that is, Sequence 5 to 8 - antiopaque. I believe this is a confirmation that some bosons, such as the W boson, indeed have an antiparticle.
Since opaque cannot bind with red nor green nor blue nor silver nor grey, then it can be said that particles with opaque colour charge have zero colour charge. Additionally, since silver cannot bind with red nor green nor blue nor opaque nor grey, then it can be said that particles with silver colour charge have zero colour charge. And since grey cannot bind with red nor green nor blue nor silver nor opaque, then it can be said that particles with grey colour charge have zero colour charge. And the same stands for all corresponding anticolours.
Offset in decay caused by composite particle offset
Because particles of other generations are inclined on different angles (dependent on said generation), then my model implies that some processes, such that of hadronic decay, will show a slight variation from the Standard Model. Particles with varying degrees of inclination will induce a proportionally varying degree of decay efficiency. This prediction is about to be confirmed by experimental evidences - recent experimentations at the LHC have uncovered puzzling evidences that B-mesons decay are slightly deviating from the SM's prediction:
www.nature.com...
In most preon models, the notion of a weak force boson is often dismissed. This is because most preon models are not capable of accountig for the existence of the W/Z boson. But my model not only acknowledges the existence of W/Z bosons, it goes on to explains why W/Z bosons exist only for a very short time, and, additionally, it explains why they are so massive compared to other bosons.
We already know that preons have (individually) more mass than the particle they compose. Now to understand why W/Z bosons have such a high mass, one needs only to observe the process of, say, neutron decay (which emits a W- boson) more closely. Here is an animation of the phenomenon:

Notice that at one point during the exchange of preons, the three a preons from the neutral particle (I will explain its identity later) briefly meet the three b preons from the down quark. This meeting point exist only inside a very small window of time, after which the two groups of preons continue on their way to their target particles. This meeting point, this moment where the two groups of preon are right next to each other, constitutes a particle in itself - a particle which is dissolved as soon as it is created. A virtual particle - the W- boson. Now why is it so massive? Well, let us see - it is not composed of a group of six preons as any other bosons, but actually of two groups of three preons. Since normal, six-preons bosons are so relatively light, and since isolated preons are so insanely massive, then the existence of four groups of three preons (do not forget that the parent particles too have been reduced to three preons each) easily explains why the event is measured to have such a high mass.
Preons as a cause for observed colour charges
Why do quarks only bind with quarks and antiquarks, but leptons and bosons can neither bind with one another nor with quarks? The answer to this very question lies within the preon sequence inside the particles. Quite a bit like DNA, the two kind of preons can form sequences inside particles. For instance, the down quark must be composed of four "a" preons and two "b" preons, but many arrangements of these can come up, such as aabbbb, abbbba, bbbbaa, ababbb, abbabb, etc. These sequences actually seem to have an important function inside particle - that is, the attribution of colour charges (such as red, green, antiblue, etc.).
Three simple laws are governing the sequencing of preons and attribution of colours:
1. Group-particles whose appearance is a rotation, on the spin axis, of the original structure are of the same colour.
2. If there are more than 1 preon in minority, they must touch each other inside the particle (for it is by groups that they are exchanged).
3. In the event of preon equality (three a + three b), the three preons must both touch each other and one of them be present on the side of the particle at at least one point during the structure's rotation (for it is by groups and by the side that they are exchanged).
So let us now check all possible sequences.
Electrons have only one sequence possible:

Thus it has only one possible colour, a colour which is incompatible with all others - I like to call it the silver colour (antisilver for the positron, which is bbbbbb). Since it has zero interaction with other colours, it can be said that in practice, the electron has zero colour.
Up quarks have quite a more diverse sequences range:

Out of the six permutations, Law 1 states that Sequence 2 to 5 are equivalent, and constitutes one colour - green. Sequence 1 constitues a second colour - red. Finally, Sequence 6 constitutes a third colour - blue.
Down quarks sequencing confirms the fact that quarks have no more, no less than three colours:

Once again here Sequence 13 to 15 are violating Law 2 and thus disqualify, for they form an exotic matter. From the twelve remaining permutations, Sequence 1 to 4 are equivalent by Law 1, Sequence 5 to 8 are also equivalent by Law 1, and Sequence 9 to 12 are also equivalent by Law 1.
Once again: red, green and blue.
Since quarks can form three colours, then the same applies for antiquarks, and thus we can add to that three other colours - which we would call antired, antigreen and antiblue.
Neutrinos sequencing is distinguishable from bosons sequencing in that neutrinos must have have similar top-most and bottom-most preons at least 1/2 of the time. This gives neutrinos these permutations:

Law 3 rules out Sequence 9 to 12. Law 1 indicates that Sequence 1 to 4 are equivalent, forming one colour which I like to call grey. Surprisingly, Law 1 also states that there is a second set, namely Sequence 5 to 8, seemingly forming a corresponding anticolour. I believe that this is an indication that the model is predicting an antiparticle for the neutrino.
Boson sequencing froms eight permutations:

Once again, Sequence 1 to 4 are equivalent by Law 1, forming a colour I like to call opaque, and once again Law 1 also states that there is a set of a corresponding anticolour, that is, Sequence 5 to 8 - antiopaque. I believe this is a confirmation that some bosons, such as the W boson, indeed have an antiparticle.
Since opaque cannot bind with red nor green nor blue nor silver nor grey, then it can be said that particles with opaque colour charge have zero colour charge. Additionally, since silver cannot bind with red nor green nor blue nor opaque nor grey, then it can be said that particles with silver colour charge have zero colour charge. And since grey cannot bind with red nor green nor blue nor silver nor opaque, then it can be said that particles with grey colour charge have zero colour charge. And the same stands for all corresponding anticolours.
Offset in decay caused by composite particle offset
Because particles of other generations are inclined on different angles (dependent on said generation), then my model implies that some processes, such that of hadronic decay, will show a slight variation from the Standard Model. Particles with varying degrees of inclination will induce a proportionally varying degree of decay efficiency. This prediction is about to be confirmed by experimental evidences - recent experimentations at the LHC have uncovered puzzling evidences that B-mesons decay are slightly deviating from the SM's prediction:
www.nature.com...
edit on 29-10-2015 by swanne because: (no reason given)
Neutrino as trigger for decay
In Pillar One I have ventured that the mechanism behind neutron decay was as follow: "neutron + unknown neutral background particle + unknown neutral background particle" would turn into "proton + electron + antineutrino". The exact identity of the neutral background particles which were interacting with the neutron (and triggering its decay) was unknown, but what was certain was that they were very light, neutral, and could interact via weak force.
But now Pillar Three proposes that this background particle is, in fact, the neutrino itself. Not only does this solves the identity problem, but it also eliminates the need for the second "neutral background particle".
Notice that neutrons are usually shown, for instance in Feynman diagrams, to decay into a proton plus a W- boson which in turn decays into an electron and an antineutrino (neutrino going back in time); the point being that wether the antineutrino is located in the past or future relative to the event is left to interpretation. Whereas my model contains no neutrino-like particles in the future side, it clearly shows the presence of a neutrino-like particle in the past side. My model could in fact be interpreting the process in a rather novel approach. Instead of the down quark giving birth to the W- which in turns gives birth to the antineutrino and the electron, my model could be describing the same event but with a different outcome hierarchy: the quark would couple with the antineutrino via the W- boson, causing the down quark to turn into an up quark and the antineutrino to turn into an electron.
Example - consider the process of neutron decay, as proposed by Pillar Three:
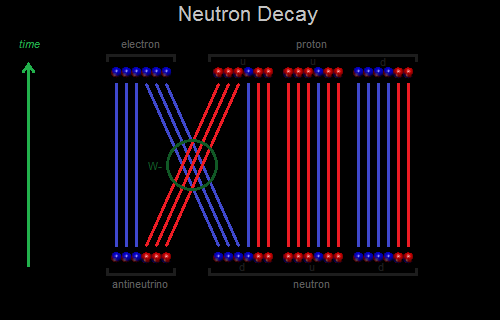
Note that by the Feynman-Stueckelberg interpretation, the two descriptions are equivalent:
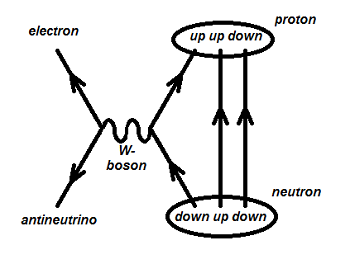
That is, in both descriptions there is a down quark turning to an up quark on one side, an electron & antineutrino on the other side, and a W- boson bridging it all together.
Additionally, there are evidences which already suggest that the magnitude of solar neutrino stream have impact on the rate of radioactive decay of particles:
phys.org...
Preons as dark matter candidates
Although my two preceding pillars assume that the existence of composite particles with less or more than six preons is unlikely, in this pillar we will examine the possibility that such events are nevertheless probable.
If there exist composite particles with less than six preons, then this open a wide, new field of candidates for dark matter. For instance, consider an anomalous composite particle made of five preons instead of six. We already know (because of the mechanism behind w/z bosons) that not only such composite particles would be much more massive than those of normal matter; but we can also know (because of the mechanism behind colour charges) that such composite particles would also form incompatible colour charges, and thus fail to strongly (by "strongly" I of course mean Strong Force) interact with composite particles of normal matter - albeit they would indeed interact gravitationally, filling the universe with mass. The principle would not only stand for anomalous particles made of five preons, but also for those made of four, three, two, one. Additionally, since packets of preons are responsible for the Weak Interaction itself and decay of particles, then these groups of preons would only interact weakly in addition of gravitationally - making them match exactly the profile of WIMPs (Weakly Interacting Massive Particles).
Only one "master" preon
We already know that preons have two sides, as described in Pillar Two. We also know, from the same Pillar, that preons can physically reverse themselves - a process which gives rise to the oscillation of kaon beams. Now, since a reversed preon is exactly identical to its counterpart (the reversed "a" preon is exactly identical to the "b" preon, and the reversed "b" preon is exactly identical to the "a" preon), then I make a final proposition - and it has the most shocking implication.
I propose that in essence, there is only one master preon - wether it takes the form of an "a" preon or "b" preon is only dependent on this primordial preon's alignment in space and time, just like the two faces of a coin.
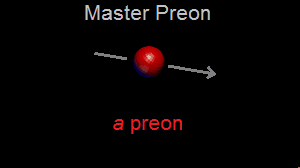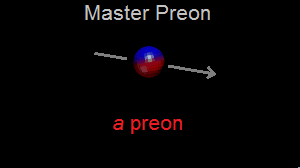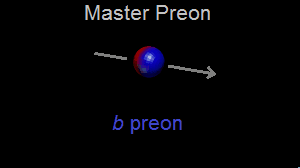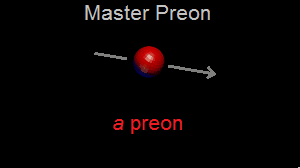
The most prominent implication of this is one which justifies my model as the most likely preon model out there. Simply put, it means that the entire universe, all of matter and all of energy, everything which exist, is made of a single master preon - one primordial particle which composes everything.
This represents a major advancement in the spirit of reductionism, and thus makes the model the most likely survivor in the face of Occam's Razor - for it is the only one in existence which has the power to model everything with only one particle.
In Pillar One I have ventured that the mechanism behind neutron decay was as follow: "neutron + unknown neutral background particle + unknown neutral background particle" would turn into "proton + electron + antineutrino". The exact identity of the neutral background particles which were interacting with the neutron (and triggering its decay) was unknown, but what was certain was that they were very light, neutral, and could interact via weak force.
But now Pillar Three proposes that this background particle is, in fact, the neutrino itself. Not only does this solves the identity problem, but it also eliminates the need for the second "neutral background particle".
Notice that neutrons are usually shown, for instance in Feynman diagrams, to decay into a proton plus a W- boson which in turn decays into an electron and an antineutrino (neutrino going back in time); the point being that wether the antineutrino is located in the past or future relative to the event is left to interpretation. Whereas my model contains no neutrino-like particles in the future side, it clearly shows the presence of a neutrino-like particle in the past side. My model could in fact be interpreting the process in a rather novel approach. Instead of the down quark giving birth to the W- which in turns gives birth to the antineutrino and the electron, my model could be describing the same event but with a different outcome hierarchy: the quark would couple with the antineutrino via the W- boson, causing the down quark to turn into an up quark and the antineutrino to turn into an electron.
Example - consider the process of neutron decay, as proposed by Pillar Three:

Note that by the Feynman-Stueckelberg interpretation, the two descriptions are equivalent:

That is, in both descriptions there is a down quark turning to an up quark on one side, an electron & antineutrino on the other side, and a W- boson bridging it all together.
Additionally, there are evidences which already suggest that the magnitude of solar neutrino stream have impact on the rate of radioactive decay of particles:
phys.org...
Preons as dark matter candidates
Although my two preceding pillars assume that the existence of composite particles with less or more than six preons is unlikely, in this pillar we will examine the possibility that such events are nevertheless probable.
If there exist composite particles with less than six preons, then this open a wide, new field of candidates for dark matter. For instance, consider an anomalous composite particle made of five preons instead of six. We already know (because of the mechanism behind w/z bosons) that not only such composite particles would be much more massive than those of normal matter; but we can also know (because of the mechanism behind colour charges) that such composite particles would also form incompatible colour charges, and thus fail to strongly (by "strongly" I of course mean Strong Force) interact with composite particles of normal matter - albeit they would indeed interact gravitationally, filling the universe with mass. The principle would not only stand for anomalous particles made of five preons, but also for those made of four, three, two, one. Additionally, since packets of preons are responsible for the Weak Interaction itself and decay of particles, then these groups of preons would only interact weakly in addition of gravitationally - making them match exactly the profile of WIMPs (Weakly Interacting Massive Particles).
Only one "master" preon
We already know that preons have two sides, as described in Pillar Two. We also know, from the same Pillar, that preons can physically reverse themselves - a process which gives rise to the oscillation of kaon beams. Now, since a reversed preon is exactly identical to its counterpart (the reversed "a" preon is exactly identical to the "b" preon, and the reversed "b" preon is exactly identical to the "a" preon), then I make a final proposition - and it has the most shocking implication.
I propose that in essence, there is only one master preon - wether it takes the form of an "a" preon or "b" preon is only dependent on this primordial preon's alignment in space and time, just like the two faces of a coin.

The most prominent implication of this is one which justifies my model as the most likely preon model out there. Simply put, it means that the entire universe, all of matter and all of energy, everything which exist, is made of a single master preon - one primordial particle which composes everything.
This represents a major advancement in the spirit of reductionism, and thus makes the model the most likely survivor in the face of Occam's Razor - for it is the only one in existence which has the power to model everything with only one particle.
******
Conclusion
I am not claiming that this model is the final truth on the matter of preons - the true scientific method calls for a skeptic attitude towards new propositions, and these include my hypothesis.
Additionally, I am already aware that I did not provide complex mathematical formulas - please note that nor is it my intention to do so here. My model is complex enough as it is now, my main priority here is clarity. Also: I am aware of the claim, by some, that preons "cannot exist" because of the so-called "mass paradox". The "paradox" can be summed up as the fact that although the preon is smaller than the particle they compose (obviously), their individual mass is expected to be greater than the particle they compose. This is sometimes used as "proof" that preons cannot exist. But as many physicists have already pointed out, "proof" is false, as the "paradox" is actually solved by postulating the existence of a cancelling binding force. Also, it is precisely this superior mass which holds the potential to solve the mystery behind dark matter itself.
And, finally, please know that it is not my intention to challenge any mainstream models such as the Sandard Model, the Quantum Model or String Theory - my only wish is to propose a hypothesis regarding the sub-structure of the observed particles (namely that of the 6 leptons, the 6 quarks, their antiparticles, and the bosons). All laws of the Standard Models, on scales larger than preons, still stand; for my hypothesis only concerns the inner composition of the particles, with the goal of matching their observed properties.
Time will tell if the SPP Theory is right, for although our current instruments cannot probe the inner structures of quarks yet, this excludes not the likeliness that compositeness will be discovered in the future, perhaps by using another approach than high energy collisions. To quote physicist Sverker Fredriksson:
"A first conclusion is therefore that the many quarks and leptons reflect a preon substructure"
cds.cern.ch...
I sincerely believe that I have just produced the most powerful preon model to date, given the fact that it reduces all elementary particles of the universe to only one master, primordial preon.
This concludes my original post, which is the culmination of two years of work.
At Time's End,
John Skieswanne
Conclusion
I am not claiming that this model is the final truth on the matter of preons - the true scientific method calls for a skeptic attitude towards new propositions, and these include my hypothesis.
Additionally, I am already aware that I did not provide complex mathematical formulas - please note that nor is it my intention to do so here. My model is complex enough as it is now, my main priority here is clarity. Also: I am aware of the claim, by some, that preons "cannot exist" because of the so-called "mass paradox". The "paradox" can be summed up as the fact that although the preon is smaller than the particle they compose (obviously), their individual mass is expected to be greater than the particle they compose. This is sometimes used as "proof" that preons cannot exist. But as many physicists have already pointed out, "proof" is false, as the "paradox" is actually solved by postulating the existence of a cancelling binding force. Also, it is precisely this superior mass which holds the potential to solve the mystery behind dark matter itself.
And, finally, please know that it is not my intention to challenge any mainstream models such as the Sandard Model, the Quantum Model or String Theory - my only wish is to propose a hypothesis regarding the sub-structure of the observed particles (namely that of the 6 leptons, the 6 quarks, their antiparticles, and the bosons). All laws of the Standard Models, on scales larger than preons, still stand; for my hypothesis only concerns the inner composition of the particles, with the goal of matching their observed properties.
Time will tell if the SPP Theory is right, for although our current instruments cannot probe the inner structures of quarks yet, this excludes not the likeliness that compositeness will be discovered in the future, perhaps by using another approach than high energy collisions. To quote physicist Sverker Fredriksson:
"A first conclusion is therefore that the many quarks and leptons reflect a preon substructure"
cds.cern.ch...
I sincerely believe that I have just produced the most powerful preon model to date, given the fact that it reduces all elementary particles of the universe to only one master, primordial preon.
This concludes my original post, which is the culmination of two years of work.
At Time's End,
John Skieswanne
I thought a Prion is a car! *__-
Behind the prions, you'll still find 'quantum information waves', Mindstuff, behind it all.
If your theories add to our Knowledge base (and they do), congrats!
Original critical thought is rare indeed!
"...scientists are condemned by their unexamined assumptions to study the nature of mirrors only by cataloging and investigating everything that mirrors can reflect. It is an endless process that never makes progress, that never reaches closure, that generates endless debate between those who have seen different reflected images, and whose enduring product is voluminous descriptions of particular phenomena." - The Adapted Mind
Behind the prions, you'll still find 'quantum information waves', Mindstuff, behind it all.
If your theories add to our Knowledge base (and they do), congrats!
Original critical thought is rare indeed!
"...scientists are condemned by their unexamined assumptions to study the nature of mirrors only by cataloging and investigating everything that mirrors can reflect. It is an endless process that never makes progress, that never reaches closure, that generates endless debate between those who have seen different reflected images, and whose enduring product is voluminous descriptions of particular phenomena." - The Adapted Mind
If there are different kinds of quarks then they, logically, would have to be composed of still smaller particles until there is only one that is
finally seen as ''the'' building block of all-that-is-and-all-that-will-be-give-or-take-a-nudge.
So the world is like a computer program made up of binary-code, except, instead of 1 and 0, representing ON or OFF, it's A or B represented by a
single preon.
Where would virtual particles or the point between energy and particle come into play? What converts energy into particles, why and how?
And where does Consciousness come into play?
Where would virtual particles or the point between energy and particle come into play? What converts energy into particles, why and how?
And where does Consciousness come into play?
originally posted by: arpgme
Where would virtual particles or the point between energy and particle come into play?
As already proposed in the OP, virtual particles such as the W boson would be created in the middle point of exchanges of preons.
What converts energy into particles, why and how?
Once again, exchange of preons. Rearrangement of the preons inside photons. If you can gather enough energy, you can cause nature to run a process opposed to annihilation - namely, particle pairs production. Instead of particles annihilating into photons, you have photons "deannihilating" into particles.
Using lasers to create particles
And where does Consciousness come into play?
I am not much into the New Age Movement.
edit on 29-10-2015 by swanne because: (no reason given)
I don't think conscious comes into play at this level at all. Unless one believes in a universal consciousness of some kind. But what a theory. I
think you lost me at some stage but it makes sense in some way. But I have always wondered about the mechanism that changes energy into matter.
originally posted by: grumpy64
But I have always wondered about the mechanism that changes energy into matter.
Simple: just read that graph upside down:

The two photons (energy, aaabbb + aaabbb) exchange 3 preons and become one electron and one positron (matter, aaaaaa + bbbbbb).
edit on 29-10-2015 by swanne because: (no reason given)
originally posted by: arpgme
So the world is like a computer program made up of binary-code, except, instead of 1 and 0, representing ON or OFF, it's A or B represented by a single preon.
I got the same impression from the decay diagrams, it looks like binary arrays undergoing bitwise operations or something. It makes sense when you think about it though because QM tells us all energy must be quantized and therefore anything can be represented using a finite number of bits, meaning there should be a way to describe reality at the binary level, and the appears the OP is getting close.
edit on 29/10/2015 by
ChaoticOrder because: (no reason given)
a reply to: namelesss
I know, geez… like setting of a firecracker and trying to count all the sparks.
You cant do that forever… building ever better detectors to finally know how many sparks are in a firecracker.
"...scientists are condemned by their unexamined assumptions to study the nature of mirrors only by cataloging and investigating everything that mirrors can reflect. It is an endless process that never makes progress, that never reaches closure, that generates endless debate between those who have seen different reflected images, and whose enduring product is voluminous descriptions of particular phenomena." - The Adapted Mind
I know, geez… like setting of a firecracker and trying to count all the sparks.
You cant do that forever… building ever better detectors to finally know how many sparks are in a firecracker.
I have no wtf clue what this is, dumb it down insanely to a few sentences please?
a reply to: swanne
Here's a question I have about photons: if they are made out of 6 preons like other elementary particles then why do they have no rest mass whereas all other types of elementary particles do have a rest mass and therefore get inhibited by the so called Higgs field? It seems to me photons are fundamentally different from all other types of particles and I'm not sure your theory accounts for that, or maybe I missed it. I'm guessing your answer will be that the symmetry of the preons causes the mass to be equalized? Why then is the neutrino classified as an aaabbb composite if it's supposed to have rest mass? Or is that the reason you developed your own explanation for the neutrino's oscillation into other flavours?
Also I have to say your ideas about preon composites as dark matter aren't nearly as terrible as I was expecting them to be. The way you can increase the mass while lowering strong interactions and maintaining gravitational interaction at the same time is impressive and seems to arise from your logic in a very organic manner, which is what I like to see. However if you are correct about dark matter being preons then it would invalidate my theory about negative energy, space, and time. Your statement about particles moving backwards in time being equivalent to anti-particles caught my attention. So in some sense when we create anti-matter, you're saying it's like creating particles which travel backwards through time?
Simple: just read that graph upside down:
Here's a question I have about photons: if they are made out of 6 preons like other elementary particles then why do they have no rest mass whereas all other types of elementary particles do have a rest mass and therefore get inhibited by the so called Higgs field? It seems to me photons are fundamentally different from all other types of particles and I'm not sure your theory accounts for that, or maybe I missed it. I'm guessing your answer will be that the symmetry of the preons causes the mass to be equalized? Why then is the neutrino classified as an aaabbb composite if it's supposed to have rest mass? Or is that the reason you developed your own explanation for the neutrino's oscillation into other flavours?
Also I have to say your ideas about preon composites as dark matter aren't nearly as terrible as I was expecting them to be. The way you can increase the mass while lowering strong interactions and maintaining gravitational interaction at the same time is impressive and seems to arise from your logic in a very organic manner, which is what I like to see. However if you are correct about dark matter being preons then it would invalidate my theory about negative energy, space, and time. Your statement about particles moving backwards in time being equivalent to anti-particles caught my attention. So in some sense when we create anti-matter, you're saying it's like creating particles which travel backwards through time?
edit on
29/10/2015 by ChaoticOrder because: (no reason given)
new topics
-
Traveling the world with no passport
Social Issues and Civil Unrest: 2 hours ago -
Happy Thanksgiving to ATS
General Chit Chat: 5 hours ago -
Simple Thanksgiving
Food and Cooking: 11 hours ago
top topics
-
Mind Blowing Cave under someones land
Fragile Earth: 16 hours ago, 17 flags -
The Party of Peace - Trump Cabinet Picks Targeted with Death Threats
US Political Madness: 17 hours ago, 15 flags -
Simple Thanksgiving
Food and Cooking: 11 hours ago, 15 flags -
Trump Presidential Transition Team will not use GSA or Government entities to come to DC
US Political Madness: 15 hours ago, 14 flags -
Trump could make a peaceful American Revolution
US Political Madness: 15 hours ago, 14 flags -
Happy Thanksgiving to ATS
General Chit Chat: 5 hours ago, 6 flags -
Traveling the world with no passport
Social Issues and Civil Unrest: 2 hours ago, 5 flags
active topics
-
Simple Thanksgiving
Food and Cooking • 28 • : yeahright -
Petition Calling for General Election at 564,016 and rising Fast
Political Issues • 107 • : gortex -
Traveling the world with no passport
Social Issues and Civil Unrest • 2 • : AdultMaleHumanUK -
I thought Trump was the existential threat?
World War Three • 116 • : andy06shake -
Well, here we go red lines crossed Biden gives the go ahead to use long range missiles
World War Three • 402 • : andy06shake -
Mind Blowing Cave under someones land
Fragile Earth • 18 • : Flyingclaydisk -
D.B. Cooper mystery may be solved
General Conspiracies • 22 • : andy06shake -
Happy Thanksgiving to ATS
General Chit Chat • 4 • : VariedcodeSole -
Post A Funny (T&C Friendly) Pic Part IV: The LOL awakens!
General Chit Chat • 7840 • : GENERAL EYES -
Trump Presidential Transition Team will not use GSA or Government entities to come to DC
US Political Madness • 14 • : WeMustCare
