The intrinsic necessity of quantum mechanics

a reply to: TKDRL

Can you give some examples?

There are a couple ways in which science could be "way off":
1. Our reality is projected in front of us to be a lie (along the lines of the Allegory of the Cave, or The Matrix)
2. Our senses are very limited (sight, sound, etc). There are many more senses beyond what we experience, so we don't know how to search for things that we've never experienced. In this case, we wouldn't know how to detect what we don't know about. I use the example: Imagine the universe as a water balloon. Everything you experience is made from the water in the balloon, but what if the walls of the balloon are made of a completely different material (rubber). We might truly have no way to know or experience the walls of the balloon because it is literally beyond everything else we experience inside the balloon. But that doesn't mean the walls aren't there. We just don't have a way to experience them. OK, it's probably not the best analogy.
3. Parallel dimensions exist and for some reason we just don't experience them right now. One day soon they may open up "again" and angels, demons, ghosts, etc will come pouring out and it will change our science forever.

But for the most part, science is based on repeatable observations. Superstring theory is developed from calculations born of observation, but hasn't been proven to be true, so there is unproven science that might unsettle you.

There are well known failures in the history of science, but those often lead to great science, like Phlogiston theory--the theory that fire/flame was a fundamental element.

Gathering more observations helps dispute bad theory and create more sound science. This applies to quantum mechanics and astronomy.

a reply to: ChaoticOrder

-part 2-

What is "quantum spacetime theory"? If you're talking about theories of quantized space-time such as loop quantum gravity...

Yes, it is quantized space-time. There is a video of TEDxBoulder in my previous post that talks about the specific theory.

The violations of Bell's inequalities, due to quantum entanglement, provide near definitive demonstrations of something that was already strongly suspected: that quantum physics cannot be represented by any version of the classical picture of physics. - Roger Penrose

I'm not claiming a "better classical model" will describe QM. I'm claiming a completely new model might describe QM's random (or seemingly random) properties. The quantized space-time theory works at the Planck scale to introduce movement across 9 spatial dimensions and 2 time dimensions. It also relates to ideas proposed for Vacuum energy.

[Leonard Susskind] has contributed a lot to science... True, string theory is not easy to verify and may never be, and I personally don't really buy into it, but it has still helped advanced many different aspects of science and there are many strong theoretical reasons to believe it could be true, or at least part of the solution

I suppose my argument is more in regards to the hundreds of physicists who went on to get Ph.D.s in a field that might turn out to be false. Was following Susskind a good idea for the future of science? Will these physicists be seen as a lost generation, following pseudo-science? I watched your video on Superstrings. Its origins do make sense. But tens of thousands of high-IQ man-hours have been thrown at the problem. That's a huge expenditure of rare resources. It's quite a gamble, in my mind. Simultaneously, bridging QM and Relativity is a big job. In this battle, there may be losses.

Separately, I thought I'd include this video for the thread, as it describes vacuum fluctuations:

a reply to: Protector
I got frustrated and had to stop. I am planning on restarting from the beginning tomorrow, see if I can get through the whole thing. I usually listen to audiobooks while I paint, the book was getting me annoyed and interrupting my flow lol. Gotta try and catch some sleep for now, hope OP don't mind me stepping into the thread.

a reply to: ChaoticOrder

You just answered your own question. If an empty vacuum is equivalent to nothingness then it can easily explain why our universe appears to be infinite and flat according to all our observations and inferences. Nothingness has no start or end, it has no boundaries, it has no origin. I understand the fact it's hard to equate the vacuum to nothing when it has properties we can ascribe to it and mass can effect the curvature of the space-time fabric, it's why many scientists prefer to describe complete nothingness as something with no space or time. I'm just not convinced that logic is entirely accurate, an empty vacuum could very well be the most fundamental type of nothingness.

I follow your assumption. But it's worth stating that the nothingness/vacuum might not be infinite. And my purpose for wanting an "origin story" for vacuum energy is that good science attempts to describe where "the unknown" comes from. Fluctuations in the vacuum then become magical sparkles and fairy dust that pervade the universe. While you could argue that it might be true, I think it's better to consider that vacuum energy also has a source that isn't the vacuum.

I would argue just this universe has a virtually inexhaustible supply of everything, but that doesn't mean those resources are easy to acquire, they're spread out across vast distances.

That is a plausible, but unsatisfying explanation... not that truth has to be satisfying. I still hate the idea of using infinity to describe anything. Particles aren't infinitely small, nor superstrings. The universe most likely isn't infinitely big, just incredibly big. Once you get rid of infinities, you do have boundaries that need good explanations. To me, that's good science. Remember, the story use to be, "The heavens are the realm of God(s) and that's all the explanation you need." Or, if you don't know something, add an infinity and say it's too big to know, or doesn't need further explanation. I hate it.

This logic is completely ridiculous as well because again you're assuming that some interaction in one universe will affect all others, and also you're assuming it's possible to destroy a universe with a bomb, which is a completely ridiculous premise to begin with.

Are you saying that you're a physicist who doesn't entertain "the ridiculous"? Quantum Mechanics was once consider ridiculous. I'm actually using a common mathematical approach of presenting a single instance that violates the whole. A "bomb" is just an stand-in for anything that could destroy spacetime... a great collapsing event... some lethal event that destroys a universe, including space and time. It's not that ridiculous. There is no reason to believe that universes must go on forever. Also, the Many Worlds theory IS RIDICULOUS. You can't expect someone to use a normal argument against something as asinine as infinitely branching universes. AND, in a branching multiverse, they would almost certainly be connected at the point where they branch off... which is a reasonable expectation of a branching multiverse.

Since you linked to the explanation of an infinitely expanding universe, from all points simultaneously, what your take on that? It sounded like you took issue with the explanation. What's a better explanation?

originally posted by: Protector
a reply to: ChaoticOrder

I told him what you said about Bell's theorem. He wanted me to pass along his statement above and find out where his logic is incorrect.

Well I've already given you my opinion on the matter and why I think that way, the randomness is intrinsically necessary. It's a complex topic because superdeterminism is still a possibility, although I think if that were true we'd see clear signs of it. Perhaps it would be more useful to show charts taken from two different surveys of physicists, mathematicians, etc:

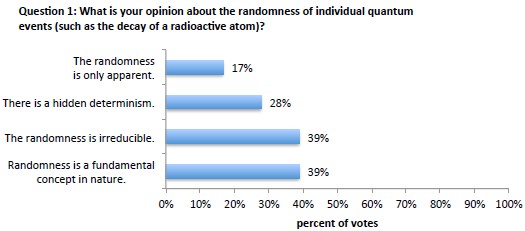
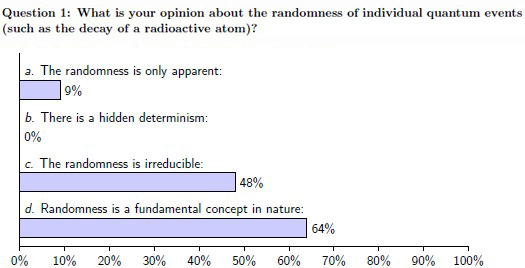
a reply to: Protector

Yes, it is quantized space-time. There is a video of TEDxBoulder in my previous post that talks about the specific theory.

Yes I'm quite aware of the theory because I tend to prefer my space loopy rather than stringy. But like I said before that still doesn't make reality deterministic.

I suppose my argument is more in regards to the hundreds of physicists who went on to get Ph.D.s in a field that might turn out to be false. Was following Susskind a good idea for the future of science?

Yes I agree you have a point to some extent but there's still a lot of useful insight coming from studying the subject even if it leads no where, which is why I have a bit more sympathy for the string theory camp then I do for say the dark matter WIMP camp, who've spent many years and many billions of dollars to build high precision DM detectors and have nothing to show for it, but wont even entertain the idea they're probably on the wrong track.

Separately, I thought I'd include this video for the thread, as it describes vacuum fluctuations:

Yes I've seen it before... I have some what of an addiction to watching science videos on YouTube lol.

a reply to: ChaoticOrder


I would concur with the position that chaos theory makes what to us look random is ordered upon some scale.


Problematic to a flat universe is that in the late 90's the universe as we understand it was about 40 billion light years wide and while today I have seen research to that of 156 billion light years wide. The problem I consider is that while from our point of view the universe does seem flat?

At issue would be if in fact it is curved beyond our comprehension.

In physics, the parallel axis theorem, also known as Huygens–Steiner theorem, or just as Steiner's theorem,[1] after Christiaan Huygens and Jakob Steiner, can be used to determine the mass moment of inertia or the second moment of area of a rigid body about any axis, given the body's moment of inertia about a parallel axis through the object's center of gravity and the perpendicular distance between the axis.

en.wikipedia.org...


Could the Universe be curved beyond our comprehension?

a reply to: Kashai

At issue would be if in fact it is curved beyond our comprehension.

It could very well be curved but there are multiple reasons I tend to believe it's "flat". All our analysis of the CMB indicates there's no curvature and as Krauss says it's the most mathematically elegant solution and a flat universe is essentially the only type of universe which can be a zero energy universe because a universe with a positive or negative energy density has curvature. However there are some special geometries that allow space to be flat yet still finite, however Occam's razor would indicate such special cases are unlikely, I prefer the simplest and most elegant solutions.

The exact shape is still a matter of debate in physical cosmology, but experimental data from various, independent sources (WMAP, BOOMERanG and Planck for example) confirm that the observable universe is flat with only a 0.4% margin of error.[3][4][5] Theorists have been trying to construct a formal mathematical model of the shape of the universe. In formal terms, this is a 3-manifold model corresponding to the spatial section (in comoving coordinates) of the 4-dimensional space-time of the universe. The model most theorists currently use is the Friedmann–Lemaître–Robertson–Walker (FLRW) model. Arguments have been put forward that the observational data best fit with the conclusion that the shape of the global universe is infinite and flat,[6] but the data are also consistent with other possible shapes, such as the so-called Poincaré dodecahedral space[7][8] and the Picard horn.[9]

Shape of the universe

a reply to: ChaoticOrder

Cosmologists normally work with a given space-like slice of spacetime called the comoving coordinates, the existence of a preferred set of which is possible and widely accepted in present-day physical cosmology.

The section of space-time that can be observed is the backward light cone (all points within the cosmic light horizon, given time to reach a given observer), while the related term Hubble volume can be used to describe either the past light cone or commoving space up to the surface of last scattering. To speak of "the shape of the universe (at a point in time)" is ontologically naïve from the point of view of special relativity alone: due to the relativity of simultaneity we cannot speak of different points in space as being "at the same point in time" nor, therefore, of "the shape of the universe at a point in time".

Same link.


Your position seems to argue that reality does not have some kind of Bias?

a reply to: Kashai

I wouldn't conclude we cannot say anything about the shape of the universe just because of general relativity. Also just because it's difficult to speak of singular moments in time doesn't mean we cannot generalize to long spans of time and speak meaningfully about the shape, and just because different frames of reference will report different observations, doesn't mean there is not an absolute frame of reference which observes how things really happen, reality can only unfold one way despite conflicting observations. Also I don't subscribe to the full implications of general relativity because it hasn't yet been merged with quantum mechanics.

The problem with surgery is that there in reality is no map.

You can ask but its really.....

Are photons perfect?

You will have to excuse me but I am also really into relating to these issues.

Hypothetically there are at least 5 ways......

Much of the early work on five dimensional space was in an attempt to develop a theory that unifies the four fundamental forces in nature: strong and weak nuclear forces, gravity and electromagnetism. German mathematician Theodor Kaluza and Swedish physicist Oskar Klein independently developed the Kaluza–Klein theory in 1921, which used the fifth dimension to unify gravity with electromagnetic force. Although their approaches were later found to be at least partially inaccurate, the concept provided a basis for further research over the past century.[1]

To explain why this dimension would not be directly observable, Klein suggested that the fifth dimension would be rolled up into a tiny, compact loop on the order of 10-33 centimeters.[1] Under his reasoning, he envisioned light as a disturbance caused by rippling in the higher dimension just beyond human perception, similar to how fish in a pond can only see shadows of ripples across the surface of the water caused by raindrops.[2] While not detectable, it would indirectly imply a connection between seemingly unrelated forces. Kaluza-Klein theory experienced a revival in the 1970s due to the emergence of superstring theory and supergravity: the concept that reality is composed of vibrating strands of energy, a postulate only mathematically viable in ten dimensions or more. Superstring theory then evolved into a more generalized approach known as M-theory. M-theory suggested a potentially observable extra dimension in addition to the ten essential dimensions which would allow for the existence of superstrings. The other 10 dimensions are compacted, or "rolled up", to a size below the subatomic level.[1][2] Kaluza–Klein theory today is seen as essentially a gauge theory, with the gauge being the circle group.[citation needed

en.wikipedia.org...

What about the idea that we are the result of randomness offering order in relation to Chaos theory?

Have you ever considered meditation with your eye's open?

Although the importance of Bohr's correspondence principle is largely undisputed, there is far less agreement concerning how the correspondence principle should be defined. It is important to distinguish between Bohr's own understanding of this principle and what it came to mean for the larger physics community. Even if one restricts oneself to Bohr's writings, however, there is still a disagreement among Bohr scholars regarding precisely which of the several relations between classical and quantum mechanics that Bohr discovered should be designated as the correspondence principle. There are three primary candidate-definitions in the literature. First, there is the frequency interpretation, according to which the correspondence principle is a statistical asymptotic agreement between one component in the Fourier decomposition of the classical frequency and the quantum frequency in the limit of large quantum numbers. Second, there is the intensity interpretation according to which it is a statistical agreement in the limit of large quantum numbers between the quantum intensity, understood in terms of the probability of a quantum transition, and the classical intensity, understood as the square of the amplitude of one component of the classical motion. Finally, there is the selection rule interpretation, according to which the correspondence principle is the statement that each allowed quantum transition between stationary states corresponds to one harmonic component of the classical motion.

plato.stanford.edu...