It looks like you're using an Ad Blocker.
Please white-list or disable AboveTopSecret.com in your ad-blocking tool.
Thank you.
Some features of ATS will be disabled while you continue to use an ad-blocker.
share:
This thread combines into a single thread all of the previous threads concerning the ABC Preon Model. This way the entire presentation can appear in
one place. Each thread took some time to write; the full effort took several weeks to get through. However, now that the we have reached the
conclusion of what exists to date, everything can be put into a single place.
In addition to including the earlier stand-alone threads, there is a discussion about the requirements of a good theory as well as some concluding remarks at the end of this presentation. There is of course little reason to separate out those final words into a thread of their own at this point, now that the full effort is done.
Combining things into a single thread makes it far easier to find other relevant postings if a need or desire for that arises.
Lastly, note that this is a thread on where the ABC Preon Model stands as of April, 2017. It is hoped that further progress will be made on the ABC Preon Model well into the future.
With those preliminaries out of the way, it is now time to go on to the presentation of the ABC Preon Model, which will be done in a series of comments following this initial post, due to the size limitations of what is allowed to be uploaded at any one time.
In addition to including the earlier stand-alone threads, there is a discussion about the requirements of a good theory as well as some concluding remarks at the end of this presentation. There is of course little reason to separate out those final words into a thread of their own at this point, now that the full effort is done.
Combining things into a single thread makes it far easier to find other relevant postings if a need or desire for that arises.
Lastly, note that this is a thread on where the ABC Preon Model stands as of April, 2017. It is hoped that further progress will be made on the ABC Preon Model well into the future.
With those preliminaries out of the way, it is now time to go on to the presentation of the ABC Preon Model, which will be done in a series of comments following this initial post, due to the size limitations of what is allowed to be uploaded at any one time.
1.a. Background: the Standard Model of Elementary Particle Physics
To get started, I'll first present a review of how the science of elementary particle physics got to where it is today.
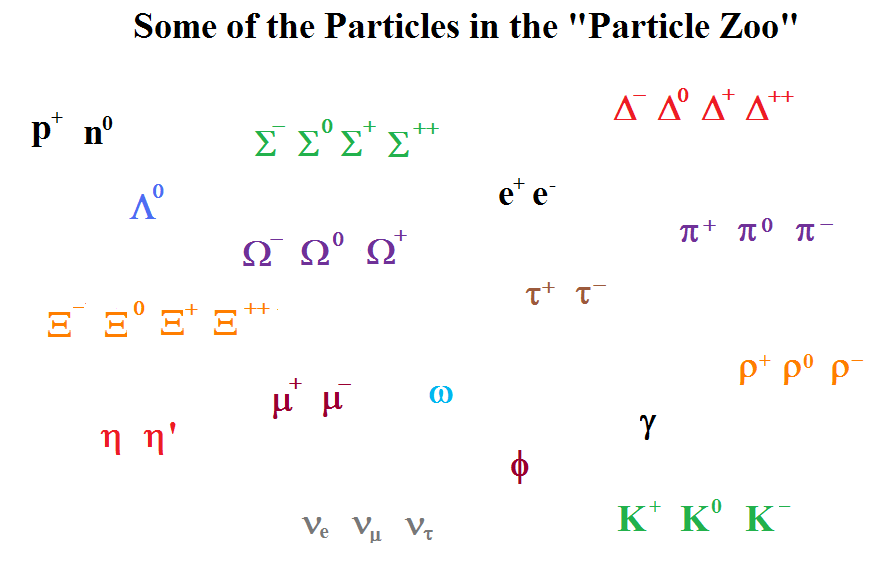
The figure above shows a portion of the particles that were discovered using particle accelerators. The number of such particles became so large that it was termed "a particle zoo". It was clear by the early 1960's that the number of particles discovered was getting so large that there was likely some underlying pattern that could simplify our view of elementary particle physics.
A major step forward in simplifying mankind's view of nature occurred in 1964 when Murry Gell-Mann and George Zweig proposed an underlying model. Gell-Mann had used the term quark for the elementary particles, while Zweig had used the term ace. Eventually, the term "quark" was accepted by the community. In the quark model, Hadronic matter is proposed to be built from underlying quarks. Baryons are states that have three bound quarks, while mesons are a bound quark-antiquark pair. Leptons were identified as a separate type of matter. As a result, in 1964, simplicity was reestablished. Nature consisted of three quarks, named up, down, and strange, and four leptons, which were the electron, the muon, and their two associated neutrinos.
The initial simplicity of the quark model began to fade into complexity almost immediately. In 1965 Glashow and Bjorken proposed a fourth quark, the charm quark, which was discovered by Richter and Ting in 1974. In 1970 Kobayashi and Maskawa theorized that CP violation in experimental results could be explained by adding two more quarks, and indeed these quarks were discovered by Ferimab researchers. The bottom quark was discovered in 1977 and the top in 1995. Also, over the period between 1974 and 1977, a new lepton, the tau, was discovered at SLAC by a team of collaborators.
In addition to the quarks and leptons, force carriers are a central part of today's standard model. In 1979, Glashow, Weinberg and Salam proposed the electro-weak theory of particle interactions to unify the weak and electromagnetic forces in a single theoretical framework. This work predicted the existence of three more particles, which were called the intermediate vector bosons. The weak bosons, called the W and Z, were discovered by a team at CERN led by Carlo Rubia in 1983. Simon van der Meer enabled the discovery by leading the development of stochastic cooling of particle beams. Note that the W boson comes in two types, one with a positive electric charge and the other negatively charged, while the Z particle has zero electric charge.
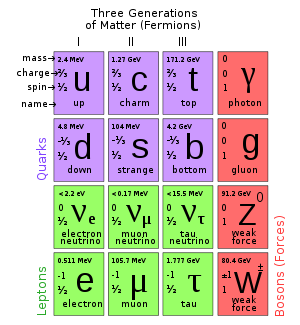
In the figure above we see a depiction of the standard model for elementary particles as advertised by its proponents. The depiction shows a rather simple set of 16 particles, which includes six quarks, six leptons and four force carriers.
Despite the advertised simplicity of the standard model, the model has several problems that leave it rather unsatisfactory from a philosophical point of view. The first additional complication is that the rules used to form particles involve a color charge. It is of course perfectly acceptable that nature may employ otherwise identical particles that have one of three color charges, but the downside is that this means that there are actually three quarks for each one listed in the figure above. The theory also specifies that there are eight different gluons, not just the one shown above. Secondly, each quark and lepton shown in the figure above has an antimatter counterpart. This too is OK, even necessary, but it means that there are twice as many particles than the number advertised above. And beyond the counting slight of hand, there are additional problems.
Fundamental to present theory is the result that no quark can be isolated. As quarks become separated from their partners, the theory stipulates that the force pulling them back in gets ever larger. Before a quark can be freed, separating it involves a force so large that the energy associated with it is capable of generating a quark antiquark pair, and each member of the pair then associates with the fragments of what was being pulled apart, so no quark can ever be isolated. In light of this, as philosophers we should ask: How can something be proven to exist if it can never be isolated? I would submit that such existence can never be proven - only inferred.
Another problem is that the weak force has no direction. Typical forces such as the electric, magnetic and gravitational forces have both magnitude and direction. They are vector quantities. But the weak force is really a particle exchange phenomena that has no direction associated with it. A last known problem is that there is no satisfactory calculational framework for the standard model. There are many good approximation schemes, but the mathematics is not anywhere close to the elegance and accuracy of quantum electrodynamics. This makes it hard to compare results against theory to test the model. (For instance, a pion is presumed to be a two body state. The two body problem is well known, yet there is no standard model prediction for pion masses.) For all of these reasons, despite its success, it took quite a while for the quark model to gain full acceptance in the physics community. I recall back in the early days speakers starting their comments by saying, "in what is now the standard way of doing things" and eventually "in what is becoming the standard model of our field". The standard model was indeed the model that became the standard way of looking at things, but early on everyone was under the belief that something better would soon come along.
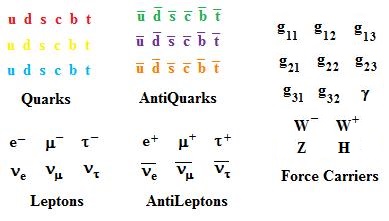
Above we see a figure that is more honest in its presentation of the existing standard model. Shown above are each of the three colors of each of the six quarks as well as their antimatter partners. Also shown are all six leptons along with their antimatter partners. Lastly, all the force carriers are shown. With this full accounting of particles it is seen that the standard model involves 61 elementary particles, since there are 18 quarks, 18 anti-quarks, 6 leptons and 13 force carriers. While some standard model proponents may argue that a red up quark is the same as a blue up quark, the rebuttal is that we certainly don't believe that a positron is the same particle as an electron. Even though a positron is identical to an electron in every aspect except for its electric charge and lepton number we still recognize that any such difference means that the particles are different particles. Similarly, an up quark with a red charge should be recognized as a different particle than an up quark with a blue charge if we are going to have an honest appraisal of our elementary particles. With this honest appraisal it is clear from the diagram presented above that the standard model has reached the point in its development where a simpler underpinning is desirable.
To get started, I'll first present a review of how the science of elementary particle physics got to where it is today.

The figure above shows a portion of the particles that were discovered using particle accelerators. The number of such particles became so large that it was termed "a particle zoo". It was clear by the early 1960's that the number of particles discovered was getting so large that there was likely some underlying pattern that could simplify our view of elementary particle physics.
A major step forward in simplifying mankind's view of nature occurred in 1964 when Murry Gell-Mann and George Zweig proposed an underlying model. Gell-Mann had used the term quark for the elementary particles, while Zweig had used the term ace. Eventually, the term "quark" was accepted by the community. In the quark model, Hadronic matter is proposed to be built from underlying quarks. Baryons are states that have three bound quarks, while mesons are a bound quark-antiquark pair. Leptons were identified as a separate type of matter. As a result, in 1964, simplicity was reestablished. Nature consisted of three quarks, named up, down, and strange, and four leptons, which were the electron, the muon, and their two associated neutrinos.

The initial simplicity of the quark model began to fade into complexity almost immediately. In 1965 Glashow and Bjorken proposed a fourth quark, the charm quark, which was discovered by Richter and Ting in 1974. In 1970 Kobayashi and Maskawa theorized that CP violation in experimental results could be explained by adding two more quarks, and indeed these quarks were discovered by Ferimab researchers. The bottom quark was discovered in 1977 and the top in 1995. Also, over the period between 1974 and 1977, a new lepton, the tau, was discovered at SLAC by a team of collaborators.
In addition to the quarks and leptons, force carriers are a central part of today's standard model. In 1979, Glashow, Weinberg and Salam proposed the electro-weak theory of particle interactions to unify the weak and electromagnetic forces in a single theoretical framework. This work predicted the existence of three more particles, which were called the intermediate vector bosons. The weak bosons, called the W and Z, were discovered by a team at CERN led by Carlo Rubia in 1983. Simon van der Meer enabled the discovery by leading the development of stochastic cooling of particle beams. Note that the W boson comes in two types, one with a positive electric charge and the other negatively charged, while the Z particle has zero electric charge.

In the figure above we see a depiction of the standard model for elementary particles as advertised by its proponents. The depiction shows a rather simple set of 16 particles, which includes six quarks, six leptons and four force carriers.
Despite the advertised simplicity of the standard model, the model has several problems that leave it rather unsatisfactory from a philosophical point of view. The first additional complication is that the rules used to form particles involve a color charge. It is of course perfectly acceptable that nature may employ otherwise identical particles that have one of three color charges, but the downside is that this means that there are actually three quarks for each one listed in the figure above. The theory also specifies that there are eight different gluons, not just the one shown above. Secondly, each quark and lepton shown in the figure above has an antimatter counterpart. This too is OK, even necessary, but it means that there are twice as many particles than the number advertised above. And beyond the counting slight of hand, there are additional problems.
Fundamental to present theory is the result that no quark can be isolated. As quarks become separated from their partners, the theory stipulates that the force pulling them back in gets ever larger. Before a quark can be freed, separating it involves a force so large that the energy associated with it is capable of generating a quark antiquark pair, and each member of the pair then associates with the fragments of what was being pulled apart, so no quark can ever be isolated. In light of this, as philosophers we should ask: How can something be proven to exist if it can never be isolated? I would submit that such existence can never be proven - only inferred.
Another problem is that the weak force has no direction. Typical forces such as the electric, magnetic and gravitational forces have both magnitude and direction. They are vector quantities. But the weak force is really a particle exchange phenomena that has no direction associated with it. A last known problem is that there is no satisfactory calculational framework for the standard model. There are many good approximation schemes, but the mathematics is not anywhere close to the elegance and accuracy of quantum electrodynamics. This makes it hard to compare results against theory to test the model. (For instance, a pion is presumed to be a two body state. The two body problem is well known, yet there is no standard model prediction for pion masses.) For all of these reasons, despite its success, it took quite a while for the quark model to gain full acceptance in the physics community. I recall back in the early days speakers starting their comments by saying, "in what is now the standard way of doing things" and eventually "in what is becoming the standard model of our field". The standard model was indeed the model that became the standard way of looking at things, but early on everyone was under the belief that something better would soon come along.

Above we see a figure that is more honest in its presentation of the existing standard model. Shown above are each of the three colors of each of the six quarks as well as their antimatter partners. Also shown are all six leptons along with their antimatter partners. Lastly, all the force carriers are shown. With this full accounting of particles it is seen that the standard model involves 61 elementary particles, since there are 18 quarks, 18 anti-quarks, 6 leptons and 13 force carriers. While some standard model proponents may argue that a red up quark is the same as a blue up quark, the rebuttal is that we certainly don't believe that a positron is the same particle as an electron. Even though a positron is identical to an electron in every aspect except for its electric charge and lepton number we still recognize that any such difference means that the particles are different particles. Similarly, an up quark with a red charge should be recognized as a different particle than an up quark with a blue charge if we are going to have an honest appraisal of our elementary particles. With this honest appraisal it is clear from the diagram presented above that the standard model has reached the point in its development where a simpler underpinning is desirable.
1.b. Background: the Standard Model of Elementary Particle Physics
The treatment I have presented here so far has focused solely on a mental picture of what particles and forces make up our world. But modern physics in general, and the standard model specifically, is considerably more than just a physical model. Indeed, there are many who take the position that a physical model of nature is not something we as mere mortals are even capable of understanding. That latter philosophy dates back to relativity and the early quantum theories, theories that originally seemed quite odd, but theories that survived every important test. And therefore, with the underlying physical modeling so difficult to mentally grasp, modern physics turned toward mathematics and underlying principles instead of physical mental pictures. Between them, the principle of relativity and the principle of least action have been used very successfully to blend into a Lagrangian approach that has produced a mathematical understanding of our world. The pictures above are rather gross simplifications of the true theory, and while those simplifications are useful to describe things to the public at large, the truer picture of the standard model comes from the Lagrangian, which is far more complex that even the rather complicated picture of 61 "elementary" particles.
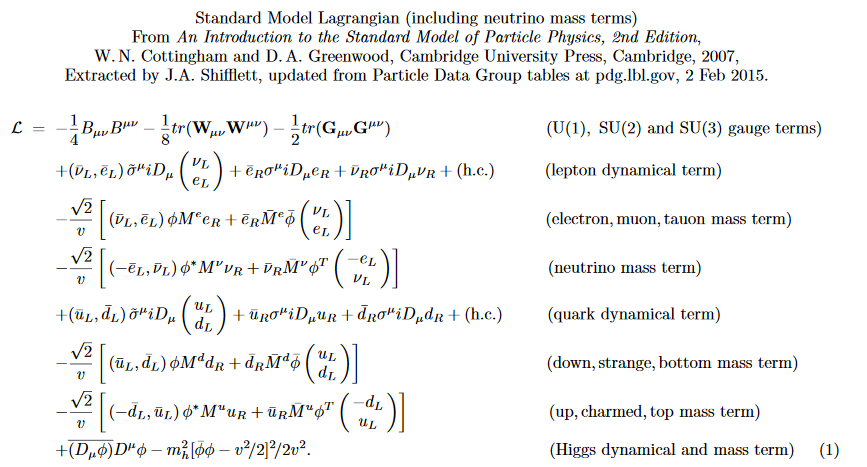
Above we see the first of 30 equations from a reference available here that serves as one reference for the Lagrangian of the present Standard Model. The terms in the Lagrangian got a good start based on the work of Dirac, who successfully arrived at a covariant formalism (meaning it is manifestly consistent with relativity) for electrons and positrons. From there, the work of many others has been successfully incorporated into a theory of mammoth proportions. We have come a long way from the simple expressions used by Newton, Maxwell, Lorentz and Einstein. So now, with such vast complexity, I believe we should ask "Is nature really that complex? Or might there be a simpler understanding?"
Of course, there are many good things about the standard model. First, it gets everything right. No known experiment is in violation of the standard model. And whenever new experiments indicate that something might not quite fit, the standard model has exhibited the room for growth needed to accommodate any new experimental results. Mixing angles and renormalization, as well as additional quarks and leptons have been added to the model over time. The analysis techniques are extremely complex, and it takes a decade or more to master them. A full Ph.D. in physics, as well as post doctoral training, are usually needed to fully grasp the intricacies of the model, and even then, practitioners may only be truly expert in a small portion of the overall model. Furthermore, development of the standard model has involved man-centuries of effort by some of the best, brightest and most trained members of the globe. As a result, the standard model is a monument to the creativity of man, and one that results in a complete modeling of all known particles and forces.
But at this moment, it is also important to note that there were many good things about the Music of the Spheres model for celestial mechanics as well. First, it got everything right. No known observation of stellar or planetary motions were in violation of its tenets. And whenever new experiments indicated that something might not quite fit, the celestial mechanics model exhibited the room for growth needed to accommodate any new experimental results. Additional spheres, cycles and epi-cycles were added to the model over time as new observations became verified. The analysis techniques were extremely complex, and it took practitioners of the time a decade or more to fully grasp the intricacies of the model. Furthermore, development of the classical celestial model involved man-centuries of effort by some of the best, brightest and most trained members of the globe. As a result, the classical celestial model was a monument to the creativity of man that resulted in a complete modeling of all known stellar and planetary motions.
Please be advised that I am not attempting to mock the standard model by comparing it to the medieval and now discredited celestial model. I truly believe that the medieval celestial model was indeed a monumental achievement, and I feel it deserves much more credit than it presently gets. The credit should come because of its attention to detail, its coherent fundamentals, and its mathematically correct and exact derivations that led to explanations of all experimental data. It was indeed an impressive effort. However, Kepler and Copernicus showed us that a much simpler model was possible. And it is my belief that nature is simpler than the standard model as well, the details of which we will get into on my next thread in this series.
The treatment I have presented here so far has focused solely on a mental picture of what particles and forces make up our world. But modern physics in general, and the standard model specifically, is considerably more than just a physical model. Indeed, there are many who take the position that a physical model of nature is not something we as mere mortals are even capable of understanding. That latter philosophy dates back to relativity and the early quantum theories, theories that originally seemed quite odd, but theories that survived every important test. And therefore, with the underlying physical modeling so difficult to mentally grasp, modern physics turned toward mathematics and underlying principles instead of physical mental pictures. Between them, the principle of relativity and the principle of least action have been used very successfully to blend into a Lagrangian approach that has produced a mathematical understanding of our world. The pictures above are rather gross simplifications of the true theory, and while those simplifications are useful to describe things to the public at large, the truer picture of the standard model comes from the Lagrangian, which is far more complex that even the rather complicated picture of 61 "elementary" particles.

Above we see the first of 30 equations from a reference available here that serves as one reference for the Lagrangian of the present Standard Model. The terms in the Lagrangian got a good start based on the work of Dirac, who successfully arrived at a covariant formalism (meaning it is manifestly consistent with relativity) for electrons and positrons. From there, the work of many others has been successfully incorporated into a theory of mammoth proportions. We have come a long way from the simple expressions used by Newton, Maxwell, Lorentz and Einstein. So now, with such vast complexity, I believe we should ask "Is nature really that complex? Or might there be a simpler understanding?"
Of course, there are many good things about the standard model. First, it gets everything right. No known experiment is in violation of the standard model. And whenever new experiments indicate that something might not quite fit, the standard model has exhibited the room for growth needed to accommodate any new experimental results. Mixing angles and renormalization, as well as additional quarks and leptons have been added to the model over time. The analysis techniques are extremely complex, and it takes a decade or more to master them. A full Ph.D. in physics, as well as post doctoral training, are usually needed to fully grasp the intricacies of the model, and even then, practitioners may only be truly expert in a small portion of the overall model. Furthermore, development of the standard model has involved man-centuries of effort by some of the best, brightest and most trained members of the globe. As a result, the standard model is a monument to the creativity of man, and one that results in a complete modeling of all known particles and forces.
But at this moment, it is also important to note that there were many good things about the Music of the Spheres model for celestial mechanics as well. First, it got everything right. No known observation of stellar or planetary motions were in violation of its tenets. And whenever new experiments indicated that something might not quite fit, the celestial mechanics model exhibited the room for growth needed to accommodate any new experimental results. Additional spheres, cycles and epi-cycles were added to the model over time as new observations became verified. The analysis techniques were extremely complex, and it took practitioners of the time a decade or more to fully grasp the intricacies of the model. Furthermore, development of the classical celestial model involved man-centuries of effort by some of the best, brightest and most trained members of the globe. As a result, the classical celestial model was a monument to the creativity of man that resulted in a complete modeling of all known stellar and planetary motions.
Please be advised that I am not attempting to mock the standard model by comparing it to the medieval and now discredited celestial model. I truly believe that the medieval celestial model was indeed a monumental achievement, and I feel it deserves much more credit than it presently gets. The credit should come because of its attention to detail, its coherent fundamentals, and its mathematically correct and exact derivations that led to explanations of all experimental data. It was indeed an impressive effort. However, Kepler and Copernicus showed us that a much simpler model was possible. And it is my belief that nature is simpler than the standard model as well, the details of which we will get into on my next thread in this series.
2. Modeling the Massive Leptons.
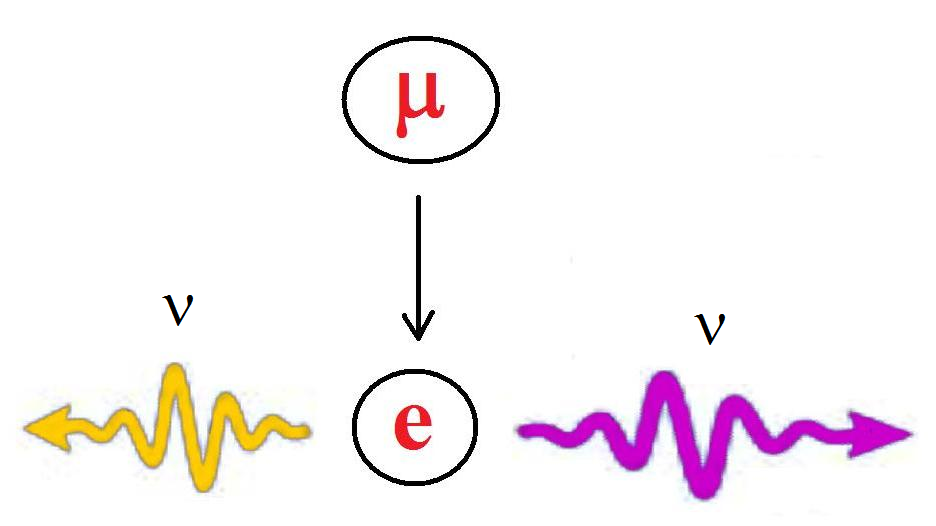
The first observation relevant to the ABC Preon Model occurred to me when I was a graduate student in the very early 1980's. I noticed that the decay of the muon was extremely similar to the decay of a hydrogen atom from an excited state into its ground state. Below we see drawings of the two events. In the first drawing, we can see a muon decay. The muon decays into an electron, and two neutrinos. Neutrinos were believed to be either massless or nearly so.
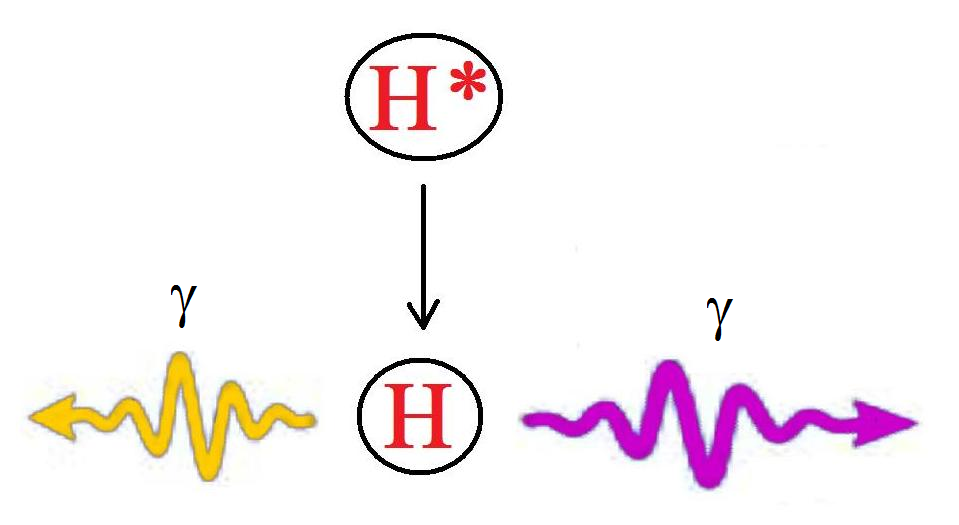
In the second drawing, we see hydrogen excited into its 2s state decaying into its ground state by emitting two photons. The photons are believed to be massless.
Notice that muon decay appears in many ways to be similar to the decay of hydrogen from its 2s state. A muon decays into an electron by emitting two neutrinos, while a hydrogen atom in its 2s state decays into a hydrogen atom in its 1s state by emitting two photons. Here we introduce the standard notation for neutrinos and photons by denoting a neutrino by the Greek letter nu, and a photon by the Greek letter gamma. It is known that the hydrogen atom is very effectively modeled as a proton and an electron being bound by a photon, and therefore the starting point for the ABC Preon Model is to propose that the massive leptons consist of two new particles, called preons, bound by a neutrino. The word preon is meant to confer a precursor particle to the ones presently assumed to be elementary, and that is why I refer to this new model as a preon model.
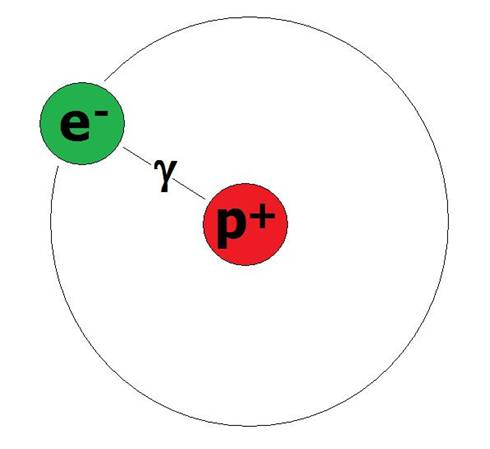
Below we see pictures of the internal structure of the hydrogen atom and our proposed preon model of the massive leptons. In the Hydrogen atom, an electron orbits a proton, and the force is carried by a photon:
In our newly proposed massive lepton model, we will propose an analogous substructure with one particle orbiting another. From experiment, we observe that hydrogen decays into its ground state by emitting photons, and a photon is the carrier of the force that binds it. Hence, since muons decay into electrons by emitting neutrinos, it follows from our analogy that the force that binds the preons together to form massive leptons is carried by the neutrino. Therefore a neutrino is shown as the binding quanta in the picture. At this point in the development we will simply name the preons "A" and "B" and we will investigate their properties later on, in future threads:

I want to emphasize how simple the onset of this new elementary particle model is. We simply look at the decay processes of Hydrogen and muons and propose that the internal structure of the muon is composed analogously to the internal structure of hydrogen. Since the radiated particle is a neutrino instead of a photon, we replace the photon by the neutrino. It is all just a simple observation at this point.
We've just seen how our analogy with the hydrogen atom has led to a proposal that the muon is the second quantum state of a composite system, and that the electron is the first quantum state. Of course, there is a third massive lepton, the tauon, that also has properties nearly identical to the muon and the electron, but with an even heavier mass. In the model proposed here, it is easy to identify the tauon as being the next excited state of the same composite system. And while the force binding the preon particles together is quite strong, neutrinos can still flow freely through matter as long as the cross section for the interaction is low. This is similar to the fact that some photons flow relatively freely through glass.
The first observation relevant to the ABC Preon Model occurred to me when I was a graduate student in the very early 1980's. I noticed that the decay of the muon was extremely similar to the decay of a hydrogen atom from an excited state into its ground state. Below we see drawings of the two events. In the first drawing, we can see a muon decay. The muon decays into an electron, and two neutrinos. Neutrinos were believed to be either massless or nearly so.

In the second drawing, we see hydrogen excited into its 2s state decaying into its ground state by emitting two photons. The photons are believed to be massless.

Notice that muon decay appears in many ways to be similar to the decay of hydrogen from its 2s state. A muon decays into an electron by emitting two neutrinos, while a hydrogen atom in its 2s state decays into a hydrogen atom in its 1s state by emitting two photons. Here we introduce the standard notation for neutrinos and photons by denoting a neutrino by the Greek letter nu, and a photon by the Greek letter gamma. It is known that the hydrogen atom is very effectively modeled as a proton and an electron being bound by a photon, and therefore the starting point for the ABC Preon Model is to propose that the massive leptons consist of two new particles, called preons, bound by a neutrino. The word preon is meant to confer a precursor particle to the ones presently assumed to be elementary, and that is why I refer to this new model as a preon model.
Below we see pictures of the internal structure of the hydrogen atom and our proposed preon model of the massive leptons. In the Hydrogen atom, an electron orbits a proton, and the force is carried by a photon:

In our newly proposed massive lepton model, we will propose an analogous substructure with one particle orbiting another. From experiment, we observe that hydrogen decays into its ground state by emitting photons, and a photon is the carrier of the force that binds it. Hence, since muons decay into electrons by emitting neutrinos, it follows from our analogy that the force that binds the preons together to form massive leptons is carried by the neutrino. Therefore a neutrino is shown as the binding quanta in the picture. At this point in the development we will simply name the preons "A" and "B" and we will investigate their properties later on, in future threads:

I want to emphasize how simple the onset of this new elementary particle model is. We simply look at the decay processes of Hydrogen and muons and propose that the internal structure of the muon is composed analogously to the internal structure of hydrogen. Since the radiated particle is a neutrino instead of a photon, we replace the photon by the neutrino. It is all just a simple observation at this point.
We've just seen how our analogy with the hydrogen atom has led to a proposal that the muon is the second quantum state of a composite system, and that the electron is the first quantum state. Of course, there is a third massive lepton, the tauon, that also has properties nearly identical to the muon and the electron, but with an even heavier mass. In the model proposed here, it is easy to identify the tauon as being the next excited state of the same composite system. And while the force binding the preon particles together is quite strong, neutrinos can still flow freely through matter as long as the cross section for the interaction is low. This is similar to the fact that some photons flow relatively freely through glass.
3. Assigning Some Quantum Numbers.
In the previous thread, we introduced the preonic modeling for the massive leptons, repeated here in the picture below:

At this point in our development, we can now move on to assign some quantum numbers to our preons. A first point of analysis is to note that to date, no experiment has shown the existence of free electric charge in fractional amounts. For that reason, we will begin by arbitrarily assigning our new A preon to have zero electric charge and our new B preon to have a charge of minus one. Since the neutrino has zero electric charge, this will leave our leptons as having a charge of minus one, as they must. Note that the antimatter leptons will have a charge of plus one, but we will deal with that topic later. Next, it is known that the neutrino has a half integer spin. In this model I am assuming that one quanta of the binding particle is contained within the composite particle, and hence, the A and B particles can either be both fermions or both bosons. Recall that Fermions are particles with half integer spin, while bosons are particles with integer spin.
By adding two fermions one will get an integer value, and then adding the half integer of the neutrino results in an overall half integer spin. Similarly, adding the spin of two bosons results in an integer spin, and then adding the half integer of the neutrino results in an overall half integer spin. Recall that in all of these additions, spin is a vector quantity. So if we add a half integer spin of the A to a half integer of the B we will get either one or zero. When we then add the half integer of the neutrino we will either get one half or one and a half. We will get one and a half if all three spins are aligned. Since leptons have a spin of one half, this means that all three such spins cannot be aligned. A similar analysis can be done if the spin of the A and the B are bosons with integer values of spin, and that case will have similar constraints on the needed alignments.
Here we will also propose a new charge law for the preons. Since the force carrier has been proposed to be the neutrino, we will call this new charge the neutrinic charge. Following our analogy with the hydrogen atom, where an electrically negative particle orbits a positive nucleus, here we will have a particle with a negative neutrinic charge orbiting a particle that has a positive neutrinic charge. We can arbitrarily assign a negative neutrinic charge to the B particle we proposed earlier, and a positive neutrinic charge to the A particle we proposed earlier. Since the neutrinic charge is arbitrary, we are free to attach the electric charge to either of the particles, and we have already chosen to assign the B particle a negative electric charge, while leaving the A particle with zero electric charge. Here we see a picture of the massive leptons with their quantum numbers assigned:
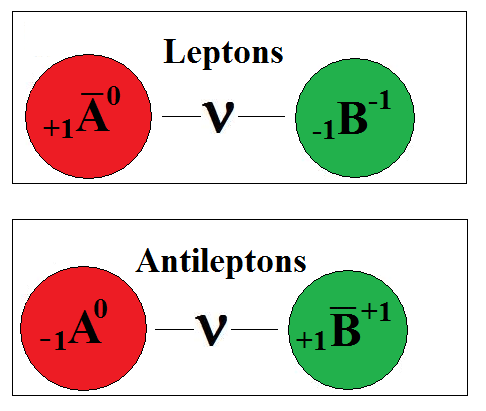
The nomenclature introduced above is to have a trailing superscript indicating the electric charge on the preon and a preceding subscript indicating the neutrinic charge on the preon. With the total electric charge being equal to minus one, we see that our preon model for leptons gives the correct electric charge. With each substituent having the opposite neutrinic charge, we see that our constructs have overall zero neutrinic charge. The result that stable particles have zero total neutrinic charge is the analogy of the fact that atoms also have zero total electrical charge. Lastly, by having the A and B particles be either both fermions or both bosons, the total spin of the leptons can be arranged to be half integer, since the bound neutrino is itself a half integer spin particle. Hence, all quantum numbers of the leptons are obtained in a model that readily allows for three generations of leptons. (At this point in the development, it is not known whether the spins of the preons are bosons or fermions, only that they are both fermions or both bosons, and the spins are constrained so that the total spin of the massive leptons is one half.)
Also introduced in the picture above are the anti-matter counterparts to the massive leptons, as well as anti-preons. A line (also called a bar) above the letter identifying the preon indicates it is an anti-preon. It will turn out in future analysis that the massive leptons are actually made up of a B and an anti-A, rather than a B and an A, so that improvement to the model is introduced above as well.
With massive leptons now modeled and their quantum numbers defined, we'll see how hadrons get modeled in the next post.
In the previous thread, we introduced the preonic modeling for the massive leptons, repeated here in the picture below:

At this point in our development, we can now move on to assign some quantum numbers to our preons. A first point of analysis is to note that to date, no experiment has shown the existence of free electric charge in fractional amounts. For that reason, we will begin by arbitrarily assigning our new A preon to have zero electric charge and our new B preon to have a charge of minus one. Since the neutrino has zero electric charge, this will leave our leptons as having a charge of minus one, as they must. Note that the antimatter leptons will have a charge of plus one, but we will deal with that topic later. Next, it is known that the neutrino has a half integer spin. In this model I am assuming that one quanta of the binding particle is contained within the composite particle, and hence, the A and B particles can either be both fermions or both bosons. Recall that Fermions are particles with half integer spin, while bosons are particles with integer spin.
By adding two fermions one will get an integer value, and then adding the half integer of the neutrino results in an overall half integer spin. Similarly, adding the spin of two bosons results in an integer spin, and then adding the half integer of the neutrino results in an overall half integer spin. Recall that in all of these additions, spin is a vector quantity. So if we add a half integer spin of the A to a half integer of the B we will get either one or zero. When we then add the half integer of the neutrino we will either get one half or one and a half. We will get one and a half if all three spins are aligned. Since leptons have a spin of one half, this means that all three such spins cannot be aligned. A similar analysis can be done if the spin of the A and the B are bosons with integer values of spin, and that case will have similar constraints on the needed alignments.
Here we will also propose a new charge law for the preons. Since the force carrier has been proposed to be the neutrino, we will call this new charge the neutrinic charge. Following our analogy with the hydrogen atom, where an electrically negative particle orbits a positive nucleus, here we will have a particle with a negative neutrinic charge orbiting a particle that has a positive neutrinic charge. We can arbitrarily assign a negative neutrinic charge to the B particle we proposed earlier, and a positive neutrinic charge to the A particle we proposed earlier. Since the neutrinic charge is arbitrary, we are free to attach the electric charge to either of the particles, and we have already chosen to assign the B particle a negative electric charge, while leaving the A particle with zero electric charge. Here we see a picture of the massive leptons with their quantum numbers assigned:

The nomenclature introduced above is to have a trailing superscript indicating the electric charge on the preon and a preceding subscript indicating the neutrinic charge on the preon. With the total electric charge being equal to minus one, we see that our preon model for leptons gives the correct electric charge. With each substituent having the opposite neutrinic charge, we see that our constructs have overall zero neutrinic charge. The result that stable particles have zero total neutrinic charge is the analogy of the fact that atoms also have zero total electrical charge. Lastly, by having the A and B particles be either both fermions or both bosons, the total spin of the leptons can be arranged to be half integer, since the bound neutrino is itself a half integer spin particle. Hence, all quantum numbers of the leptons are obtained in a model that readily allows for three generations of leptons. (At this point in the development, it is not known whether the spins of the preons are bosons or fermions, only that they are both fermions or both bosons, and the spins are constrained so that the total spin of the massive leptons is one half.)
Also introduced in the picture above are the anti-matter counterparts to the massive leptons, as well as anti-preons. A line (also called a bar) above the letter identifying the preon indicates it is an anti-preon. It will turn out in future analysis that the massive leptons are actually made up of a B and an anti-A, rather than a B and an A, so that improvement to the model is introduced above as well.
With massive leptons now modeled and their quantum numbers defined, we'll see how hadrons get modeled in the next post.
4. Modeling the Hadrons.
A simple new proposal for lepton substructure was presented in my previous thread, The ABC Preon Model. Modeling the Massive Leptons, which showed the following picture:

So it is now time to take a look at the hadrons. In all of the high energy physics experiments done over the last 70 years, it has been observed that almost all elementary particles that have been found can be classified as either a lepton or a hadron, and it has been observed that the hadrons come in two types. There are baryons, which are proposed to be made of three quarks, and there are mesons, which are proposed to be made of a quark and an antiquark.
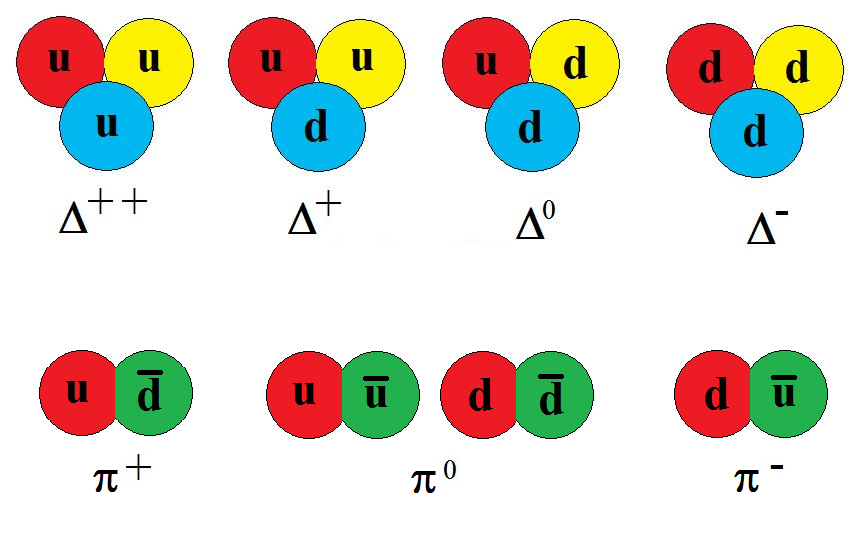
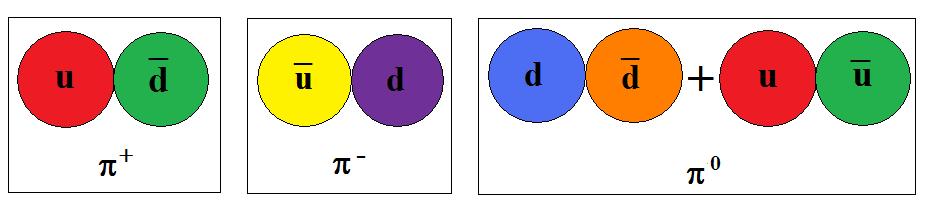
In the picture above, the delta family is shown as a representative baryon family. As can be seen, there are four possible ways to make a delta particle out of up and down quarks, and it is observed in nature that only these four particles are found. Shown below the delta family is the pi-meson (pion) family, which is a representative meson family. As can be seen, there are four ways to make pions out of up and down quark-antiquark pairs, and three pions have been found in nature. There is some evidence that the neutral pion is actually a superposition of two different types of quark substructure, as is show in the picture.
Note that the over-arching rule for making hadronic matter is that the total color of all particles must be white, and that one can obtain white particles in one of two ways. One can combine three primary colors, as in the case of the deltas, or one can combine a color with its anti-color, as in the case of the pions. Here we see why baryons are formed of three quarks, since that is how the three color combination can be achieved. And we can see why mesons must consist of quark-antiquark pairs, since that is how a color/anti-color combination can be obtained. In addition to the delta and pion families shown here, there are many, many more similar families of particles found in nature. The quark model allows for a replacement of a down quark by a heavier strange quark, or by an even heavier bottom quark, and it also allows for the up quark to be replaced by a heavier charm quark or an even heavier top quark. It is easy to see that making all permutations of such replacements would lead to an enormous number of particles. Of all particles found to date, there are none that fit outside of the quark, lepton and force carrier model, and hence there is quite good agreement between experiment and the present quark and lepton theory.
So it is clear how all known hadrons can be made from a model using quarks and antiquarks. However, there is another way that these particles can be made, as seen in the picture below:
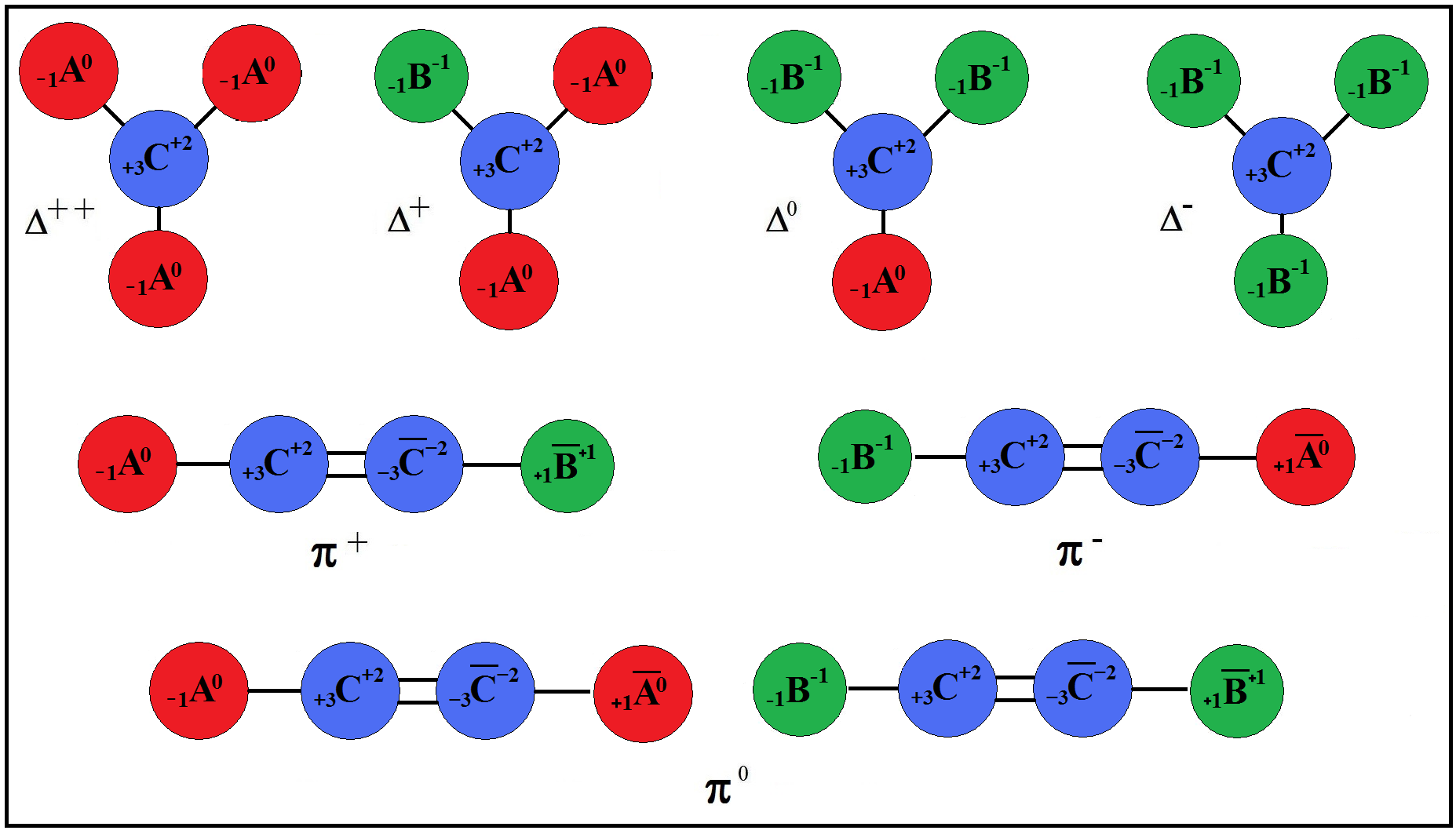
Above we see that if we propose a new preon, called C, and let it be bound to three A or B preons (where the A and B preons have been proposed in earlier threads of this series) that we again can have only four possible ways to make a delta particle. By assigning the electric charge of the C preon as plus two, the delta particle will have possible charge states of plus two, plus one, zero, and minus one just as is found in nature. We can also see that the mesons can be made if we allow a C preon to bind with its anti-preon, and further allow the C preon to bind to one additional A or B, and lastly allow the C anti-preon to bind to an anti-A or anti-B.
While at first it may seem that the arrangements shown in the picture above are arbitrary groupings, it can be shown that the groupings easily follow if we assign a neutrinic charge of plus three to the C preon. (Indeed, such neutrinic charges are shown in the diagram.) As in the case where the leptons were analogous to Hydrogen atoms, we can now see that the Baryons are analogous to Lithium atoms. In the Lithium atom a nucleus with an electric charge of plus three is orbited by three electrically negative particles. In baryons, a C preon with neutrinic charge of plus three is orbited by three neutrinically negative particles.

In the picture above I also show the binding neutrino for each binding. In this instance, I show a particle with electric charge of plus one, since the C particle has an electric charge of plus two, the B particle has an electric charge of minus one, and the A particles have zero electric charge. Of course, as shown in the previous picture, we could have three B's, three A's, or two B's and an A orbiting around the C preon, and in those cases the electric charges are different from what is shown above. But the important point is that assigning a neutrinic charge of plus three to the C preon leads to a situation where all of the known Delta particles can be formed, and no additional Delta particles are allowed. Hence, this new model is every bit as good as the quark model in predicting all of the known Delta particles. The same perfect match to nature is found with the Pi mesons.
Of course, there is a close relationship between the ABC Preons and quarks. The relationship of the ABC Preon Model to quarks will be discussed in my next thread.
A simple new proposal for lepton substructure was presented in my previous thread, The ABC Preon Model. Modeling the Massive Leptons, which showed the following picture:

So it is now time to take a look at the hadrons. In all of the high energy physics experiments done over the last 70 years, it has been observed that almost all elementary particles that have been found can be classified as either a lepton or a hadron, and it has been observed that the hadrons come in two types. There are baryons, which are proposed to be made of three quarks, and there are mesons, which are proposed to be made of a quark and an antiquark.

In the picture above, the delta family is shown as a representative baryon family. As can be seen, there are four possible ways to make a delta particle out of up and down quarks, and it is observed in nature that only these four particles are found. Shown below the delta family is the pi-meson (pion) family, which is a representative meson family. As can be seen, there are four ways to make pions out of up and down quark-antiquark pairs, and three pions have been found in nature. There is some evidence that the neutral pion is actually a superposition of two different types of quark substructure, as is show in the picture.
Note that the over-arching rule for making hadronic matter is that the total color of all particles must be white, and that one can obtain white particles in one of two ways. One can combine three primary colors, as in the case of the deltas, or one can combine a color with its anti-color, as in the case of the pions. Here we see why baryons are formed of three quarks, since that is how the three color combination can be achieved. And we can see why mesons must consist of quark-antiquark pairs, since that is how a color/anti-color combination can be obtained. In addition to the delta and pion families shown here, there are many, many more similar families of particles found in nature. The quark model allows for a replacement of a down quark by a heavier strange quark, or by an even heavier bottom quark, and it also allows for the up quark to be replaced by a heavier charm quark or an even heavier top quark. It is easy to see that making all permutations of such replacements would lead to an enormous number of particles. Of all particles found to date, there are none that fit outside of the quark, lepton and force carrier model, and hence there is quite good agreement between experiment and the present quark and lepton theory.
So it is clear how all known hadrons can be made from a model using quarks and antiquarks. However, there is another way that these particles can be made, as seen in the picture below:

Above we see that if we propose a new preon, called C, and let it be bound to three A or B preons (where the A and B preons have been proposed in earlier threads of this series) that we again can have only four possible ways to make a delta particle. By assigning the electric charge of the C preon as plus two, the delta particle will have possible charge states of plus two, plus one, zero, and minus one just as is found in nature. We can also see that the mesons can be made if we allow a C preon to bind with its anti-preon, and further allow the C preon to bind to one additional A or B, and lastly allow the C anti-preon to bind to an anti-A or anti-B.
While at first it may seem that the arrangements shown in the picture above are arbitrary groupings, it can be shown that the groupings easily follow if we assign a neutrinic charge of plus three to the C preon. (Indeed, such neutrinic charges are shown in the diagram.) As in the case where the leptons were analogous to Hydrogen atoms, we can now see that the Baryons are analogous to Lithium atoms. In the Lithium atom a nucleus with an electric charge of plus three is orbited by three electrically negative particles. In baryons, a C preon with neutrinic charge of plus three is orbited by three neutrinically negative particles.

In the picture above I also show the binding neutrino for each binding. In this instance, I show a particle with electric charge of plus one, since the C particle has an electric charge of plus two, the B particle has an electric charge of minus one, and the A particles have zero electric charge. Of course, as shown in the previous picture, we could have three B's, three A's, or two B's and an A orbiting around the C preon, and in those cases the electric charges are different from what is shown above. But the important point is that assigning a neutrinic charge of plus three to the C preon leads to a situation where all of the known Delta particles can be formed, and no additional Delta particles are allowed. Hence, this new model is every bit as good as the quark model in predicting all of the known Delta particles. The same perfect match to nature is found with the Pi mesons.
Of course, there is a close relationship between the ABC Preons and quarks. The relationship of the ABC Preon Model to quarks will be discussed in my next thread.
5. Quarks.
At this point in our development we can now make an identification between the ABC preons and quarks. In the figure below, we again see a depiction of the delta plus particle as understood by the ABC Preon Model, but I have placed rectangles around some of the components.
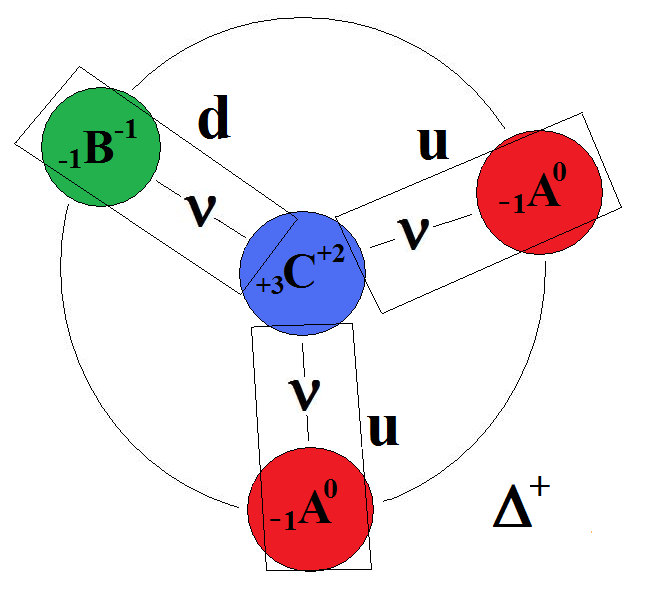
The rectangle drawn around the B preon, a binding neutrino, and a portion of the C preon is identified as a down quark. Rectangles drawn around an A preon, a binding neutrino, and a portion of the C preon are identified as up quarks. In the ABC Preon Model, quarks don't actually exist as particles. Instead, quarks are identified as quantum states involving the orbiting A and B preons and the central C preon. As with leptons, this realization allows us to understand how generations of quarks come about in nature. The u and d quarks are the ground states of their respective systems, while the strange quark is the first excited state of the orbiting B preon, and the bottom quark is the second excited state of the orbiting B preon. Similarly, the charm quark is the first excited state of the orbiting A preon, and the top quark (should it exist, we'll discuss that in a later post) would be the second excited state of the orbiting A preon.
With mesons known to be composed of quark antiquark pairs, we can now construct mesons in the ABC preon model by taking the relevant preons and anti-preons and combining them appropriately. First, we see below how the pi mesons are modeled in the standard model as quarks bound to anti-quarks.
In the ABC preon model, the u quark is seen two pictures up to be the state composed from an A preon bound to a C preon, and that structure appears in some of the pi mesons has well. Also a d quark was seen to be composed of a B preon bound to a C preon. Moving to anti-quarks, the anti-down quark is be proposed to be a B anti-preon bound to a C anti-preon, while the anti-up quark is an A anti-preon bound to a C anti-preon. The figure below shows mesons as understood by the ABC Preon Model with boxes drawn around what are now known as quarks and anti-quarks.

In the above picture, I have introduced a notation where a line joining two preons or anti-preons is used to represent a binding neutrino. Since the C preon has a neutrinic charge of plus 3, and its antimatter partner has a neutrinic charge of -3, a double bond appears between the C preon and its anti-preon. Hence, a total of three bonds appear in the diagram above for both the C preon and the C anti-preon. Note that I have also used the standard notation for anti-particles in the diagram, where a bar appearing above the letter indicates an anti-particle. The double bond between the C preon and the C anti-preon is analogous to double bonds in chemistry, where atoms share electrons in chemical bonding. Here, the binding neutrinos play the role that the binding electrons play in chemistry. Each C preon and anti-preon have three neutrino sites available for binding, while the A and B preons and the A and B anti-preons each have a single neutrino site for binding.
Note that in the model proposed here, quarks are identified as simply being a notation for an energy level and type of binding between a C preon and either an A or a B preon. And while it can be seen how massive leptons can be isolated as an A anti-preon bound to a B preon, it is clear that a quark can never be isolated by itself since it is in reality the manifestation of a portion of a C preon bound to an A or a B preon. This explains why free quarks have never been observed. Finally, note that in all of the modeling we have done so far, the rule has been that particles found in nature are those that have zero total neutrinic charge. This fact was true for the modeling of massive leptons, and it is also true for baryons and mesons. Hence, already in our development we have answered three questions about nature: 1) Why do generations of quarks and leptons exist? 2) Why can quarks not be isolated? and 3) Why do only certain types of leptonic and hadronic matter form? As a subset of question 1, we can answer I.I. Rabi's question about the muon (which was who ordered that?). The ABC Preon Model has clear answers to all of these questions, while also greatly reducing the number of elementary particles required by nature.
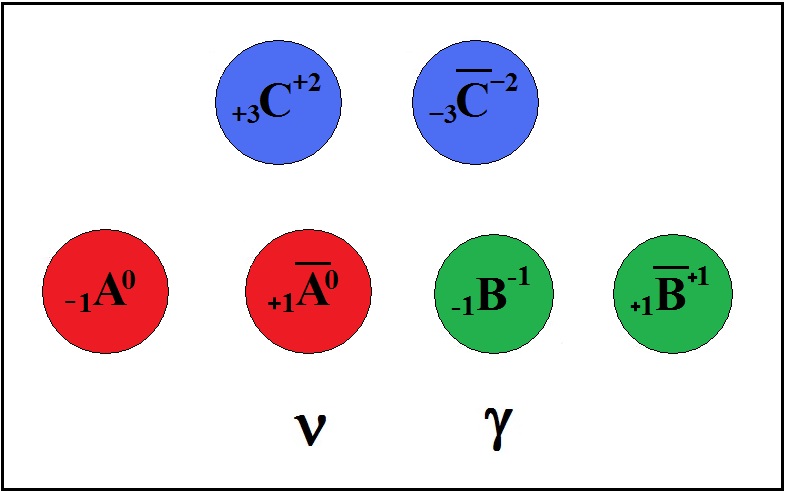
So at this point in the development we can now formalize much about the constituents of the ABC Preon Model. The model consists of three preons. The A preon has zero electric charge and a neutrinic charge of minus one. The B preon has an electric charge of minus one and a neutrinic charge of minus one. And the C preon has an electric charge of plus two and a neutrinic charge of plus three. The anti-preons have the opposite charges of the preons. Two force carriers have been proposed. The photon, which carries the electromagnetic force, and the neutrino, which carries the neutrinic force.
The particles of nature as described by the ABC Preon Model are shown in the drawing below.
At this point in our development we can now make an identification between the ABC preons and quarks. In the figure below, we again see a depiction of the delta plus particle as understood by the ABC Preon Model, but I have placed rectangles around some of the components.

The rectangle drawn around the B preon, a binding neutrino, and a portion of the C preon is identified as a down quark. Rectangles drawn around an A preon, a binding neutrino, and a portion of the C preon are identified as up quarks. In the ABC Preon Model, quarks don't actually exist as particles. Instead, quarks are identified as quantum states involving the orbiting A and B preons and the central C preon. As with leptons, this realization allows us to understand how generations of quarks come about in nature. The u and d quarks are the ground states of their respective systems, while the strange quark is the first excited state of the orbiting B preon, and the bottom quark is the second excited state of the orbiting B preon. Similarly, the charm quark is the first excited state of the orbiting A preon, and the top quark (should it exist, we'll discuss that in a later post) would be the second excited state of the orbiting A preon.
With mesons known to be composed of quark antiquark pairs, we can now construct mesons in the ABC preon model by taking the relevant preons and anti-preons and combining them appropriately. First, we see below how the pi mesons are modeled in the standard model as quarks bound to anti-quarks.

In the ABC preon model, the u quark is seen two pictures up to be the state composed from an A preon bound to a C preon, and that structure appears in some of the pi mesons has well. Also a d quark was seen to be composed of a B preon bound to a C preon. Moving to anti-quarks, the anti-down quark is be proposed to be a B anti-preon bound to a C anti-preon, while the anti-up quark is an A anti-preon bound to a C anti-preon. The figure below shows mesons as understood by the ABC Preon Model with boxes drawn around what are now known as quarks and anti-quarks.

In the above picture, I have introduced a notation where a line joining two preons or anti-preons is used to represent a binding neutrino. Since the C preon has a neutrinic charge of plus 3, and its antimatter partner has a neutrinic charge of -3, a double bond appears between the C preon and its anti-preon. Hence, a total of three bonds appear in the diagram above for both the C preon and the C anti-preon. Note that I have also used the standard notation for anti-particles in the diagram, where a bar appearing above the letter indicates an anti-particle. The double bond between the C preon and the C anti-preon is analogous to double bonds in chemistry, where atoms share electrons in chemical bonding. Here, the binding neutrinos play the role that the binding electrons play in chemistry. Each C preon and anti-preon have three neutrino sites available for binding, while the A and B preons and the A and B anti-preons each have a single neutrino site for binding.
Note that in the model proposed here, quarks are identified as simply being a notation for an energy level and type of binding between a C preon and either an A or a B preon. And while it can be seen how massive leptons can be isolated as an A anti-preon bound to a B preon, it is clear that a quark can never be isolated by itself since it is in reality the manifestation of a portion of a C preon bound to an A or a B preon. This explains why free quarks have never been observed. Finally, note that in all of the modeling we have done so far, the rule has been that particles found in nature are those that have zero total neutrinic charge. This fact was true for the modeling of massive leptons, and it is also true for baryons and mesons. Hence, already in our development we have answered three questions about nature: 1) Why do generations of quarks and leptons exist? 2) Why can quarks not be isolated? and 3) Why do only certain types of leptonic and hadronic matter form? As a subset of question 1, we can answer I.I. Rabi's question about the muon (which was who ordered that?). The ABC Preon Model has clear answers to all of these questions, while also greatly reducing the number of elementary particles required by nature.
So at this point in the development we can now formalize much about the constituents of the ABC Preon Model. The model consists of three preons. The A preon has zero electric charge and a neutrinic charge of minus one. The B preon has an electric charge of minus one and a neutrinic charge of minus one. And the C preon has an electric charge of plus two and a neutrinic charge of plus three. The anti-preons have the opposite charges of the preons. Two force carriers have been proposed. The photon, which carries the electromagnetic force, and the neutrino, which carries the neutrinic force.
The particles of nature as described by the ABC Preon Model are shown in the drawing below.

6. Relationship to the Standard Model.
As a review before we delve deeper into the model, it is now useful to recap what we've covered so far and see how the ABC Preon Model relates to the Standard Model. Recall that the massive leptons are identifed within the ABC Preon Model as the various states of an anti-A preon bound to a B preon while encompassing a single neutrino, and that the massive antileptons are simply the antimatter counterparts of the massive leptons, as shown below.

The electron is understood to be the ground state of the massive lepton depicted above, the muon the first excited state and the tauon the second excited state. The positron is understood to be the ground state of the massive anti-lepton depicted above, the anti-muon the first excited state and the anti-tauon the second excited state. Along with the neutrino (which we will discuss more fully in the next thread) this shows that all of the leptons employed in the standard model are also available in the ABC Preon Model.
The quarks are identified as a bound state of an A or B preon to a portion of a C preon, while the anti-quarks are identified as a bound state of an anti-A or anti-B preon with a portion of an anti-C preon. Below we see an example baryon and an example meson family, using the delta plus as the example baryon and the pion family as an example meson family.


The up quark is understood to be the ground state of an A preon bound to a portion of a C preon with a neutrino as depicted above, the charm quark is the first excited state of that system and the top quark will get its own thread later in this series. The bottom quark is understood to be the ground state of a B preon bound to a portion of a C preon with a neutrino as depicted above, the strange quark is the first excited state of that system and the bottom quark is the second exited state. The anti-quarks are constructed by replacing each preon with its anti-preon. This shows that all of the quarks (except for the top, as will be discussed later) that are employed in the standard model are also available in the ABC Preon Model.
Since all of the quarks and massive leptons employed in the Standard Model are also available in the ABC Preon Model, all known hadronic and massive leptonic particles consistent with the Standard Model are also consistent with the ABC Preon Model. This is a very important point, since it is well known that the Standard Model is capable of modeling all known particles. Hence (setting aside issues regarding neutrinos and the top quark that will be addressed in future threads) the ABC Preon Model is also capable of modeling all known particles.
The fact that the ABC Preon Model dovetails into the Standard Model is a result of the fact that the ABC Preon Model is a preon model. Preons are assumed to be lower level building blocks for what have heretofore been assumed to be nature's elementary particles. Since the Standard Model so successfully predicts the vast array of experimental data, any preon model will need to be consistent with the Standard Model within certain limits, and we now see clearly how the ABC Preon Model achieves that needed consistency.
Yet in order for any scientific theory to really make a difference, it must have a difference. It is not sufficient to just proclaim a new model that in the end does everything the same as the one we aim to replace. And while a simpler set of elementary particles is appealing, it isn't really enough to separate the ABC Preon Model from the Standard Model. What is needed are different predictions that can be tested. And those different predictions will come into play when one considers high energy physics phenomena beyond what quarks and leptons allow. Those important issues will indeed be looked at in future threads.
Since all of the quarks and heavy leptons employed in the Standard Model are also available in the ABC Preon Model, all known hadronic and heavy leptonic particles consistent with the Standard Model are also consistent with the ABC Preon Model. This is a very important point, since it is well known that the Standard Model is capable of modeling all known particles. Hence (setting aside issues regarding neutrinos and the top quark that will be addressed in future threads) the ABC Preon Model is also capable of modeling all known particles.
The fact that the ABC Preon Model dovetails into the Standard Model is a result of the fact that the ABC Preon Model is a preon model. Preons are assumed to be lower level building blocks for what have heretofore been assumed to be nature's elementary particles. Since the Standard Model so successfully predicts the vast array of experimental data, any preon model will need to be consistent with the Standard Model within certain limits, and we now see clearly how the ABC Preon Model achieves that needed consistency.
Yet in order for any scientific theory to really make a difference, it must have a difference. It is not sufficient to just proclaim a new model that in the end does everything the same as the one we aim to replace. And while a simpler set of elementary particles is appealing, it isn't really enough to separate the ABC Preon Model from the Standard Model. What is needed are different predictions that can be tested. And those different predictions will come into play when one considers high energy physics phenomena beyond what the quarks and leptons allow. Those important issues will indeed be looked at in future threads.
As a review before we delve deeper into the model, it is now useful to recap what we've covered so far and see how the ABC Preon Model relates to the Standard Model. Recall that the massive leptons are identifed within the ABC Preon Model as the various states of an anti-A preon bound to a B preon while encompassing a single neutrino, and that the massive antileptons are simply the antimatter counterparts of the massive leptons, as shown below.

The electron is understood to be the ground state of the massive lepton depicted above, the muon the first excited state and the tauon the second excited state. The positron is understood to be the ground state of the massive anti-lepton depicted above, the anti-muon the first excited state and the anti-tauon the second excited state. Along with the neutrino (which we will discuss more fully in the next thread) this shows that all of the leptons employed in the standard model are also available in the ABC Preon Model.
The quarks are identified as a bound state of an A or B preon to a portion of a C preon, while the anti-quarks are identified as a bound state of an anti-A or anti-B preon with a portion of an anti-C preon. Below we see an example baryon and an example meson family, using the delta plus as the example baryon and the pion family as an example meson family.


The up quark is understood to be the ground state of an A preon bound to a portion of a C preon with a neutrino as depicted above, the charm quark is the first excited state of that system and the top quark will get its own thread later in this series. The bottom quark is understood to be the ground state of a B preon bound to a portion of a C preon with a neutrino as depicted above, the strange quark is the first excited state of that system and the bottom quark is the second exited state. The anti-quarks are constructed by replacing each preon with its anti-preon. This shows that all of the quarks (except for the top, as will be discussed later) that are employed in the standard model are also available in the ABC Preon Model.
Since all of the quarks and massive leptons employed in the Standard Model are also available in the ABC Preon Model, all known hadronic and massive leptonic particles consistent with the Standard Model are also consistent with the ABC Preon Model. This is a very important point, since it is well known that the Standard Model is capable of modeling all known particles. Hence (setting aside issues regarding neutrinos and the top quark that will be addressed in future threads) the ABC Preon Model is also capable of modeling all known particles.
The fact that the ABC Preon Model dovetails into the Standard Model is a result of the fact that the ABC Preon Model is a preon model. Preons are assumed to be lower level building blocks for what have heretofore been assumed to be nature's elementary particles. Since the Standard Model so successfully predicts the vast array of experimental data, any preon model will need to be consistent with the Standard Model within certain limits, and we now see clearly how the ABC Preon Model achieves that needed consistency.
Yet in order for any scientific theory to really make a difference, it must have a difference. It is not sufficient to just proclaim a new model that in the end does everything the same as the one we aim to replace. And while a simpler set of elementary particles is appealing, it isn't really enough to separate the ABC Preon Model from the Standard Model. What is needed are different predictions that can be tested. And those different predictions will come into play when one considers high energy physics phenomena beyond what quarks and leptons allow. Those important issues will indeed be looked at in future threads.
Since all of the quarks and heavy leptons employed in the Standard Model are also available in the ABC Preon Model, all known hadronic and heavy leptonic particles consistent with the Standard Model are also consistent with the ABC Preon Model. This is a very important point, since it is well known that the Standard Model is capable of modeling all known particles. Hence (setting aside issues regarding neutrinos and the top quark that will be addressed in future threads) the ABC Preon Model is also capable of modeling all known particles.
The fact that the ABC Preon Model dovetails into the Standard Model is a result of the fact that the ABC Preon Model is a preon model. Preons are assumed to be lower level building blocks for what have heretofore been assumed to be nature's elementary particles. Since the Standard Model so successfully predicts the vast array of experimental data, any preon model will need to be consistent with the Standard Model within certain limits, and we now see clearly how the ABC Preon Model achieves that needed consistency.
Yet in order for any scientific theory to really make a difference, it must have a difference. It is not sufficient to just proclaim a new model that in the end does everything the same as the one we aim to replace. And while a simpler set of elementary particles is appealing, it isn't really enough to separate the ABC Preon Model from the Standard Model. What is needed are different predictions that can be tested. And those different predictions will come into play when one considers high energy physics phenomena beyond what the quarks and leptons allow. Those important issues will indeed be looked at in future threads.
7. Neutrinos.
At this point it is important to consider the role of the neutrino in the ABC preon model.
There is only one neutrino in the ABC Preon Model. There is no labeling of neutrinos as being an electron neutrino, a muon neutrino or a tauon neutrino. Nor are anti-neutrinos tagged as being different from regular neutrinos in the ABC Preon Model. Like photons, it is assumed that neutrinos can be pair produced in vacuum. But just as the photons that are produced from pair-production are regular photons, so the neutrinos that are produced from pair-production are just regular neutrinos. The neutrino, like the photon, is its own anti-particle as understood from the ABC Preon Model.
The original rationale for neutrinos not coming in different flavors and types was just a straight-forward analogy with photons since the ABC Preon Model proposes that the neutrino, like the photon, is a force carrier. But another reason for proposing that all neutrinos are the same comes from the modeling of mesons within the ABC Preon Model. Below we repeat the drawing from a previous thread:

In the above picture recall that a notation is employed wherein a line joining preons represents a binding neutrino. From the picture it is easy to see how an anti-neutrino could bind the anti-A to the anti-C, and how an anti-neutrino could bind the anti-B to the anti-C, as then anti-quarks would involve anti-neutrinos along with anti-preon constituents. But what about the binding between the C and the anti-C? It is there that the sameness of anti-neutrinos and neutrinos becomes apparent.
A Prediction Realized. The original publication (about 20 years ago) predicted that neutrino oscillations should exist at some level. This prediction was based on a pure simplicity argument founded upon an analogy with photons as mentioned above. It was admitted that it might indeed prove necessary to include a labeling and separation of the various types of neutrino if experimental data demanded it, but that would leave the ABC preon model no better nor worse than the standard model as far as neutrinos are concerned. The argument as to why neutrino oscillations had not been seen at the time of publication was that it may be that the cross section for interaction is just so small that oscillations hadn't been observed yet.
Years after the prediction for neutrino oscillations was made, neutrino oscillations were indeed found. Rather recent experimentation has determined that the oscillations are consistent with a theory of neutrinos involving a small neutrino mass. And so the central prediction of the original ABC Preon Model - that all neutrinos should be the same - has been proven by observations. What starts out as one "flavor" of neutrino will eventually evolve to the other flavor types. This of course indicates that all neutrinos are indeed the same, and that their flavor is just a matter of some flavor-phase that they are in at the time and place of observation.
Neutrino interaction cross sections are known to be very small. Yet in the ABC Preon Model neutrinos are responsible for what will be shown in upcoming posts to be very large binding energies. Help on understanding how this can be so is found by considering what happens with the scattering of photons off of matter.
First, consider what happens as photon energies get large. Photons in the visible spectrum already travel through glass with very small attenuation. But when one gets to x-rays and gamma-rays the photons travel through even strongly absorbing materials more and more easily as their energy increases. (For a reference, click here.) Hence, we see that the cross section for interaction decreases to smaller and smaller values as the photon energy is increased into the MeV and then to the GeV range.
Secondly, consider what happens when photons of small energy interact with atoms. For photons in the infrared, the energy of the photon is not enough to excite the atomicly bound electrons into a new quantum state. Hence, low energy photons are incapable of changing the electron's energy level within the atom.
With the above two facts to guide us, as well as with an assumption that preonic bindings will involve energies of a GeV or more, it should not be surprising that the cross sections are small for observed neutrino/matter events. The GeV binding energies mean that there is a very strong bond between the two preons, so having a neutrino affect that bond will require a neutrino energy high enough to change the internal energy state. And having such a high energy neutrino will lead to a very small cross section for those events to occur.
As for low energy neutrino scatterings, that may indeed occur. But how could we measure it? In order to detect the neutrino in the first place we generally must have the neutrinos interact with matter to produce something that we can then subsequently detect. And in order to get that which we can detect, the neutrinos must change the internal energy state of the bound preons in order to form something new. Which, as described in the paragraph above, will require a very high energy neutrino. Hence, low energy neutrino scattering will likely be impossible to see.
So low energy neutrino scattering will likely be impossible to see, and high energy neutrino interactions will have an extremely low cross section. Therefore we won't experimentally detect much interaction between neutrinos and matter at all, even though neutrinos are proposed to be responsible for what is an extremely strong force that binds the preons together.
Neutrinos have a half-integer spin and so they will also have a helicity. And this leads to the conclusion that there will be at least two helicity states of the neutrinos. One could identify these states separately. But we typically don't do that for photons or electrons or any other particle with spin, so I see no need to do that for the neutrinos of the ABC Preon Model either. And of course, neutrinos may have different energies as well, but that is usually not grounds for considering something to be a different entity. So for the purposes of the ABC Preon Model we will continue to use a simple single label for all neutrinos discussed and consider them to be identical particles, although they may of course have different momenta, energy, spin and flavor phase at any given place and time.
At this point it is important to consider the role of the neutrino in the ABC preon model.
There is only one neutrino in the ABC Preon Model. There is no labeling of neutrinos as being an electron neutrino, a muon neutrino or a tauon neutrino. Nor are anti-neutrinos tagged as being different from regular neutrinos in the ABC Preon Model. Like photons, it is assumed that neutrinos can be pair produced in vacuum. But just as the photons that are produced from pair-production are regular photons, so the neutrinos that are produced from pair-production are just regular neutrinos. The neutrino, like the photon, is its own anti-particle as understood from the ABC Preon Model.
The original rationale for neutrinos not coming in different flavors and types was just a straight-forward analogy with photons since the ABC Preon Model proposes that the neutrino, like the photon, is a force carrier. But another reason for proposing that all neutrinos are the same comes from the modeling of mesons within the ABC Preon Model. Below we repeat the drawing from a previous thread:

In the above picture recall that a notation is employed wherein a line joining preons represents a binding neutrino. From the picture it is easy to see how an anti-neutrino could bind the anti-A to the anti-C, and how an anti-neutrino could bind the anti-B to the anti-C, as then anti-quarks would involve anti-neutrinos along with anti-preon constituents. But what about the binding between the C and the anti-C? It is there that the sameness of anti-neutrinos and neutrinos becomes apparent.
A Prediction Realized. The original publication (about 20 years ago) predicted that neutrino oscillations should exist at some level. This prediction was based on a pure simplicity argument founded upon an analogy with photons as mentioned above. It was admitted that it might indeed prove necessary to include a labeling and separation of the various types of neutrino if experimental data demanded it, but that would leave the ABC preon model no better nor worse than the standard model as far as neutrinos are concerned. The argument as to why neutrino oscillations had not been seen at the time of publication was that it may be that the cross section for interaction is just so small that oscillations hadn't been observed yet.
Years after the prediction for neutrino oscillations was made, neutrino oscillations were indeed found. Rather recent experimentation has determined that the oscillations are consistent with a theory of neutrinos involving a small neutrino mass. And so the central prediction of the original ABC Preon Model - that all neutrinos should be the same - has been proven by observations. What starts out as one "flavor" of neutrino will eventually evolve to the other flavor types. This of course indicates that all neutrinos are indeed the same, and that their flavor is just a matter of some flavor-phase that they are in at the time and place of observation.
Neutrino interaction cross sections are known to be very small. Yet in the ABC Preon Model neutrinos are responsible for what will be shown in upcoming posts to be very large binding energies. Help on understanding how this can be so is found by considering what happens with the scattering of photons off of matter.
First, consider what happens as photon energies get large. Photons in the visible spectrum already travel through glass with very small attenuation. But when one gets to x-rays and gamma-rays the photons travel through even strongly absorbing materials more and more easily as their energy increases. (For a reference, click here.) Hence, we see that the cross section for interaction decreases to smaller and smaller values as the photon energy is increased into the MeV and then to the GeV range.
Secondly, consider what happens when photons of small energy interact with atoms. For photons in the infrared, the energy of the photon is not enough to excite the atomicly bound electrons into a new quantum state. Hence, low energy photons are incapable of changing the electron's energy level within the atom.
With the above two facts to guide us, as well as with an assumption that preonic bindings will involve energies of a GeV or more, it should not be surprising that the cross sections are small for observed neutrino/matter events. The GeV binding energies mean that there is a very strong bond between the two preons, so having a neutrino affect that bond will require a neutrino energy high enough to change the internal energy state. And having such a high energy neutrino will lead to a very small cross section for those events to occur.
As for low energy neutrino scatterings, that may indeed occur. But how could we measure it? In order to detect the neutrino in the first place we generally must have the neutrinos interact with matter to produce something that we can then subsequently detect. And in order to get that which we can detect, the neutrinos must change the internal energy state of the bound preons in order to form something new. Which, as described in the paragraph above, will require a very high energy neutrino. Hence, low energy neutrino scattering will likely be impossible to see.
So low energy neutrino scattering will likely be impossible to see, and high energy neutrino interactions will have an extremely low cross section. Therefore we won't experimentally detect much interaction between neutrinos and matter at all, even though neutrinos are proposed to be responsible for what is an extremely strong force that binds the preons together.
Neutrinos have a half-integer spin and so they will also have a helicity. And this leads to the conclusion that there will be at least two helicity states of the neutrinos. One could identify these states separately. But we typically don't do that for photons or electrons or any other particle with spin, so I see no need to do that for the neutrinos of the ABC Preon Model either. And of course, neutrinos may have different energies as well, but that is usually not grounds for considering something to be a different entity. So for the purposes of the ABC Preon Model we will continue to use a simple single label for all neutrinos discussed and consider them to be identical particles, although they may of course have different momenta, energy, spin and flavor phase at any given place and time.
8. Weak Decays.
With a preon model for all known particles now established, the next things to investigate are the many interactions that particles have with one another. A very important class of interactions are the weak interactions. The weak interactions cause quarks to change from one kind to another, and also allow for lepton creation during beta decay. The best known of the weak interactions takes place when a neutron decays into a proton, emitting an electron and a neutrino in the process. Note that while an antineutrino is typically specified, as said in my previous thread neutrinos are their own anti-particle in the ABC Preon Model.

The decay of a neutron is shown in the top portion of the picture above as described by the standard model. The top portion of the picture shows the neutron decaying to a proton, electron and neutrino. The bottom portion of the picture shows the process schematically. In the standard model, one of the down quarks emits a virtual weak vector boson, called a W minus. Since the W minus has a negative electric charge, this changes the charge state of one of the quarks from minus one third to plus two thirds, converting a down quark to an up quark. The virtual W particle then decays into an electron and a neutrino. And when the down quark is converted to an up quark, the neutron is transformed into a proton during this process.
Now, let's look at beta decay from the point of view of the ABC Preon Model:
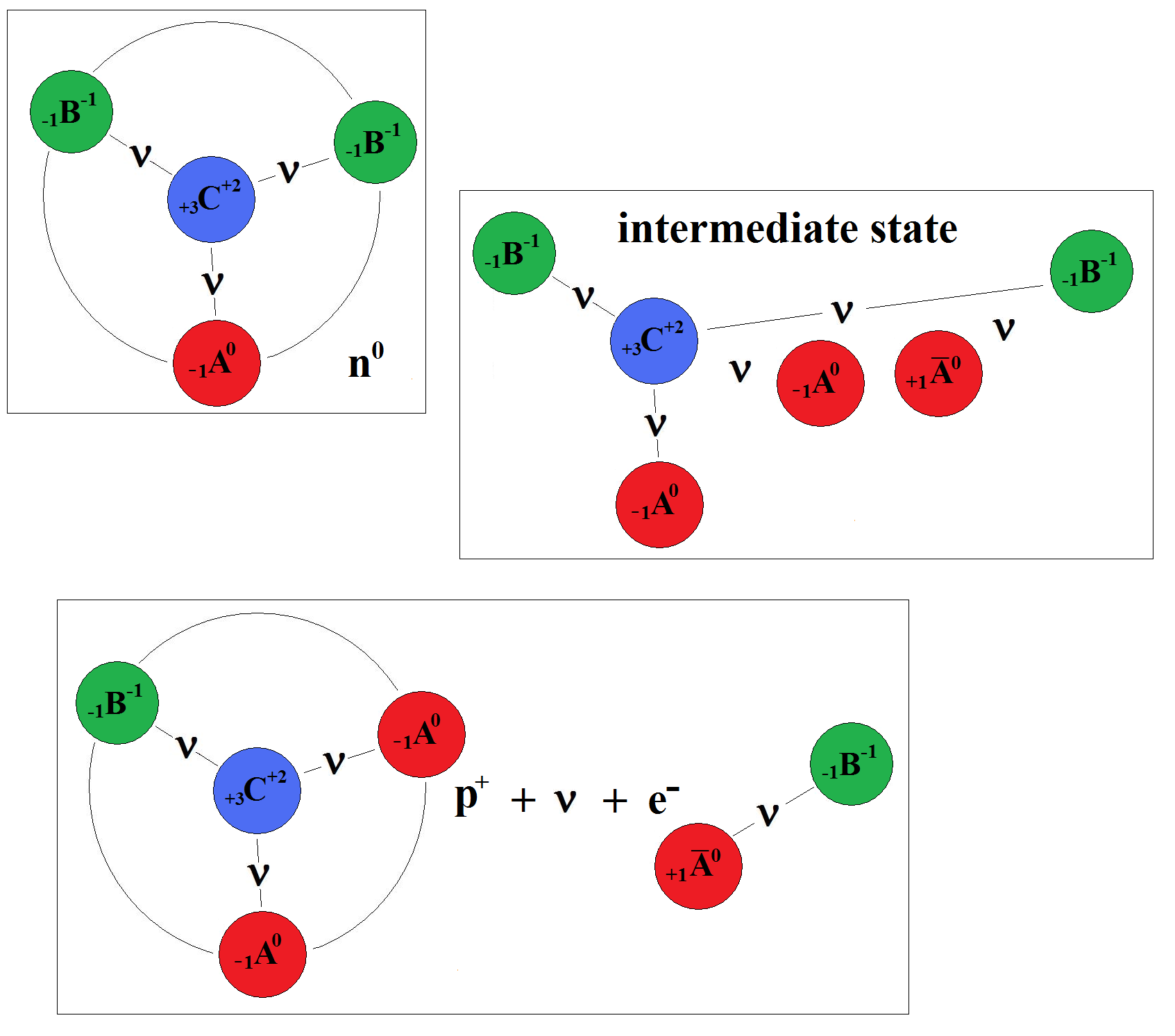
In the picture above we see the process of neutron decay, also known as beta decay, as modeled by the ABC preon model. We start with our model of the neutron, which consists of a C particle, two orbiting B particles, an orbiting A particle and the associated binding neutrinos. The decay process is most easily visualized by taking one of the B particles and having it undergo quantum tunneling out of its binding relationship. Once the B particle has separated away from the C particle, creation of an A/anti-A preon pair, as well as creation of a pair of neutrinos, can be formed out of the vacuum. Please note that vacuum formation of particle/anti-particle pairs is very common in physics, so this portion of the decay is not at all unusual. From this intermediate state, the anti-A preon and one of the neutrinos combine with the liberated B preon to form an electron. The remaining A preon takes the place that the original B preon had, and this leaves what we recognize to be a proton. Lastly we have one neutrino left over. Hence, the ABC preon model exactly models what happens in beta decay. (The neutron, proton and electron pictured above were introduced in earlier threads of this series.)
There are some important points to make regarding the process of beta decay that was just discussed. First, it is important to note that in the ABC preon model beta decay is modelled to be analogous to alpha decay from a Uranium nucleus. In alpha decay, it is known that the process occurs via quantum tunneling. Alpha particles from within the Uranium nucleus are trapped by the nuclear binding forces within the nucleus. However, there is a small portion of the alpha particle's quantum mechanical wave function that extends far enough away from the nucleus so that once the alpha particle momentarily materializes at that distant point the alpha particle can be freed. Since the wave function density is so small at that distant point, the probability for decay is small, and therefore decay of the Uranium nucleus takes a long time.
In the ABC Preon Model we see that beta decay is a similar process to Uranium alpha decay, although it also involves pair creation from vacuum. The wave function for the B preon will have a small value at a point far enough away from the C preon that allows formation of a free electron and a free neutrino and conversion of the neutron to a proton. It will be seen later that the mass of the intermediate state involved in beta decay will sum to about the mass of what is now called the W boson, and hence this process is related to something that has a mass approximately equal to that of what is now called the W boson. Finally, note that all weak decays can be handled similarly, since a B preon can tunnel out of any of the down, strange or bottom quarks, and an A can tunnel out of any of the up, charm or top quarks.

Above we see our earlier picture of the delta-plus particle as being made up of quarks. Recall that a down, strange or bottom quark has been identified as a binding between a C particle and a B particle. Charged weak decays involve the B particle tunneling through the potential barrier, with A/anti-A and neutrino pairs forming between the B and the C. The anti-A combines with the B to form a massive lepton, and the A combines with the C to change the quark from one type to another. For the up, charm or top quarks, charged weak decays involve an A particle tunneling through the potential barrier and formation of B/anti-B and neutrino pairs, again leading to the emission of a massive lepton and a change in the type of quark. Each process will involve the formation of a free neutrino as well.
Hence, in the ABC Preon model the weak decays are identified as radioactive tunneling decays, and there is no weak force.
When I was first introduced to the weak force one of the oddest things was that I was told that the weak force had no direction! But all of nature's other forces have a direction, since gravity is attractive, electric forces are either attractive or repulsive, and the strong force is attractive. We can now see why the weak force was different, and that is because it isn't really a force at all. So an additional benefit of the ABC preon model is that in our efforts to simplify the number of elementary particles we have also simplified the number of forces that exist in nature.
With a preon model for all known particles now established, the next things to investigate are the many interactions that particles have with one another. A very important class of interactions are the weak interactions. The weak interactions cause quarks to change from one kind to another, and also allow for lepton creation during beta decay. The best known of the weak interactions takes place when a neutron decays into a proton, emitting an electron and a neutrino in the process. Note that while an antineutrino is typically specified, as said in my previous thread neutrinos are their own anti-particle in the ABC Preon Model.

The decay of a neutron is shown in the top portion of the picture above as described by the standard model. The top portion of the picture shows the neutron decaying to a proton, electron and neutrino. The bottom portion of the picture shows the process schematically. In the standard model, one of the down quarks emits a virtual weak vector boson, called a W minus. Since the W minus has a negative electric charge, this changes the charge state of one of the quarks from minus one third to plus two thirds, converting a down quark to an up quark. The virtual W particle then decays into an electron and a neutrino. And when the down quark is converted to an up quark, the neutron is transformed into a proton during this process.
Now, let's look at beta decay from the point of view of the ABC Preon Model:

In the picture above we see the process of neutron decay, also known as beta decay, as modeled by the ABC preon model. We start with our model of the neutron, which consists of a C particle, two orbiting B particles, an orbiting A particle and the associated binding neutrinos. The decay process is most easily visualized by taking one of the B particles and having it undergo quantum tunneling out of its binding relationship. Once the B particle has separated away from the C particle, creation of an A/anti-A preon pair, as well as creation of a pair of neutrinos, can be formed out of the vacuum. Please note that vacuum formation of particle/anti-particle pairs is very common in physics, so this portion of the decay is not at all unusual. From this intermediate state, the anti-A preon and one of the neutrinos combine with the liberated B preon to form an electron. The remaining A preon takes the place that the original B preon had, and this leaves what we recognize to be a proton. Lastly we have one neutrino left over. Hence, the ABC preon model exactly models what happens in beta decay. (The neutron, proton and electron pictured above were introduced in earlier threads of this series.)
There are some important points to make regarding the process of beta decay that was just discussed. First, it is important to note that in the ABC preon model beta decay is modelled to be analogous to alpha decay from a Uranium nucleus. In alpha decay, it is known that the process occurs via quantum tunneling. Alpha particles from within the Uranium nucleus are trapped by the nuclear binding forces within the nucleus. However, there is a small portion of the alpha particle's quantum mechanical wave function that extends far enough away from the nucleus so that once the alpha particle momentarily materializes at that distant point the alpha particle can be freed. Since the wave function density is so small at that distant point, the probability for decay is small, and therefore decay of the Uranium nucleus takes a long time.
In the ABC Preon Model we see that beta decay is a similar process to Uranium alpha decay, although it also involves pair creation from vacuum. The wave function for the B preon will have a small value at a point far enough away from the C preon that allows formation of a free electron and a free neutrino and conversion of the neutron to a proton. It will be seen later that the mass of the intermediate state involved in beta decay will sum to about the mass of what is now called the W boson, and hence this process is related to something that has a mass approximately equal to that of what is now called the W boson. Finally, note that all weak decays can be handled similarly, since a B preon can tunnel out of any of the down, strange or bottom quarks, and an A can tunnel out of any of the up, charm or top quarks.

Above we see our earlier picture of the delta-plus particle as being made up of quarks. Recall that a down, strange or bottom quark has been identified as a binding between a C particle and a B particle. Charged weak decays involve the B particle tunneling through the potential barrier, with A/anti-A and neutrino pairs forming between the B and the C. The anti-A combines with the B to form a massive lepton, and the A combines with the C to change the quark from one type to another. For the up, charm or top quarks, charged weak decays involve an A particle tunneling through the potential barrier and formation of B/anti-B and neutrino pairs, again leading to the emission of a massive lepton and a change in the type of quark. Each process will involve the formation of a free neutrino as well.
Hence, in the ABC Preon model the weak decays are identified as radioactive tunneling decays, and there is no weak force.
When I was first introduced to the weak force one of the oddest things was that I was told that the weak force had no direction! But all of nature's other forces have a direction, since gravity is attractive, electric forces are either attractive or repulsive, and the strong force is attractive. We can now see why the weak force was different, and that is because it isn't really a force at all. So an additional benefit of the ABC preon model is that in our efforts to simplify the number of elementary particles we have also simplified the number of forces that exist in nature.
9. The W and Z. A and B masses. First Free Preons.
With weak decays now understood (see my previous thread), it is time to turn to the experimental record given by high energy physics experiments. The first class of high energy physics experiments to discuss are proton antiproton collision experiments. Such experiments were done in the European laboratory CERN in 1983 and they led to the discovery of the W and Z particles of the standard model. Carlo Rubbia and Simon van der Meer received Nobel prizes for their important contributions to these discoveries. However, was it really a W and Z that were found? Or can these monumental discoveries have a different explanation?
In a proton antiproton collider, a beam of protons is accelerated to nearly the speed of light, and a beam of antiprotons is also accelerated to nearly the speed of light. The beams are sent in opposing directions and made to collide. Particles formed by the collision are then studied in large detectors.
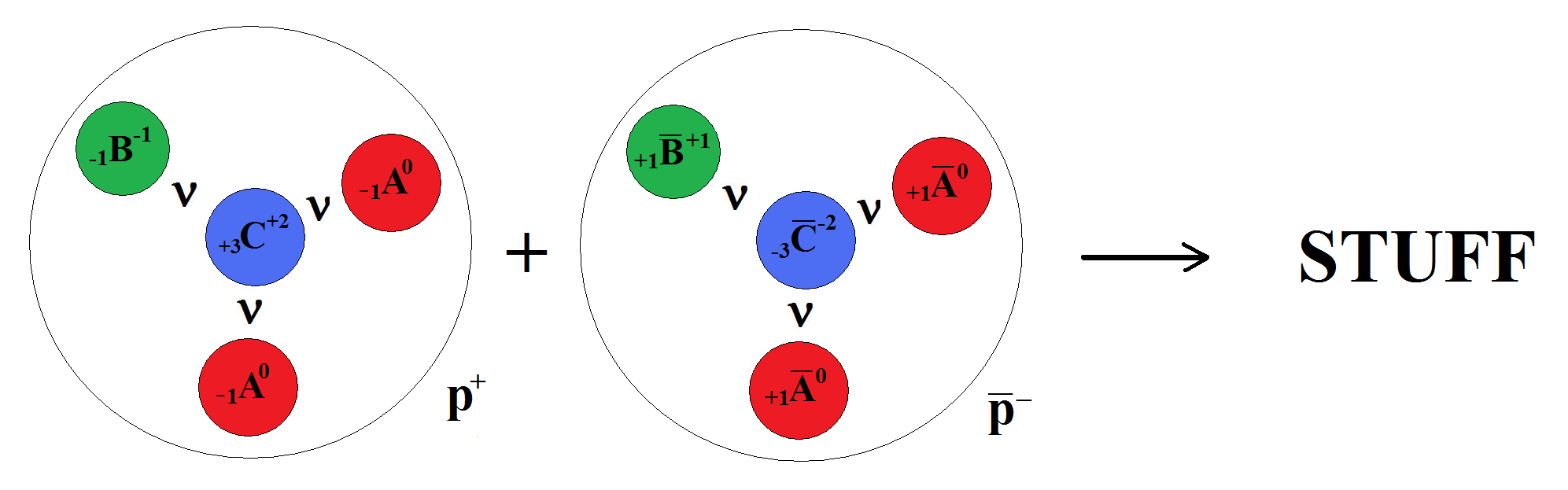
The picture above shows a proton antiproton collision as understood from the ABC Preon Model. A proton is shown on the left, and an antiproton is shown in the center. From our earlier post on Modeling the Hadrons we recall that a proton is made of a central C particle, two A's and a B, while the antiproton is the antimatter counterpart of the proton. After colliding, these particles can create many different combinations of particles.
As a first possible outcome, let's look at what is known as a W Event:
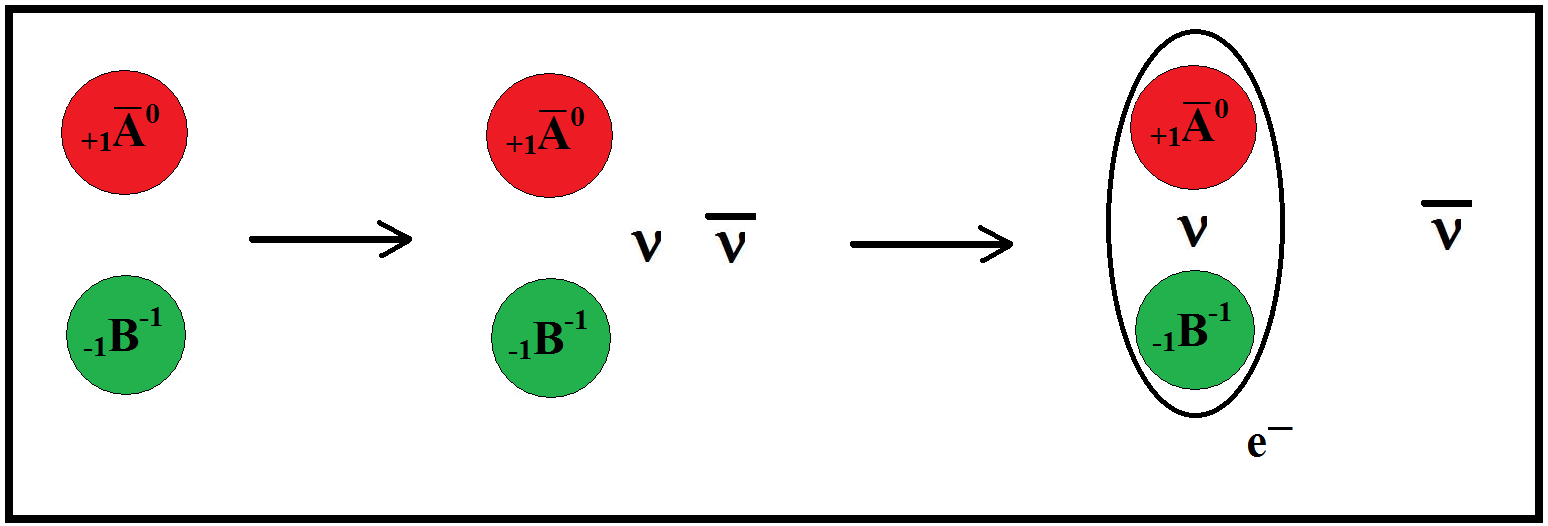
One clear possibility for a proton antiproton collision is for it to result in what is shown above, where a B preon gets knocked off of a proton and an anti-A preon gets knocked off of an antiproton. Next, a pair of neutrinos can form out of the vacuum, with one going to bind the B and anti-A into a massive lepton, and the other neutrino being free. (Massive leptons are a B bound to an anti-A by a neutrino, see The ABC Preon Model. Modeling the Massive Leptons. for more details.)
Note that vacuum creation of particle anti-particle pairs is a very frequent occurance in high energy physics experiments. It happens all the time. And so the important point here is that we can readily see how proton antiproton collisions can result in a situation where we have a massive lepton and a neutrino formed. This, along with the remaining shower of particles caused by the other proton and antiproton fragments that are left over, is what has been discovered as evidence of the W particle in proton antiproton colliders.
The W particle mass times the speed of light squared has been measured to be about 80.4 GeV. Since in the ABC Preon Model this phenomena is caused by the freeing of an A and a B preon, this allows us to arrive at the relation that the mass of the A particle plus the mass of the B particle is 80.4 GeV/c^2.
Next, let's look at what is known as a Z Event:
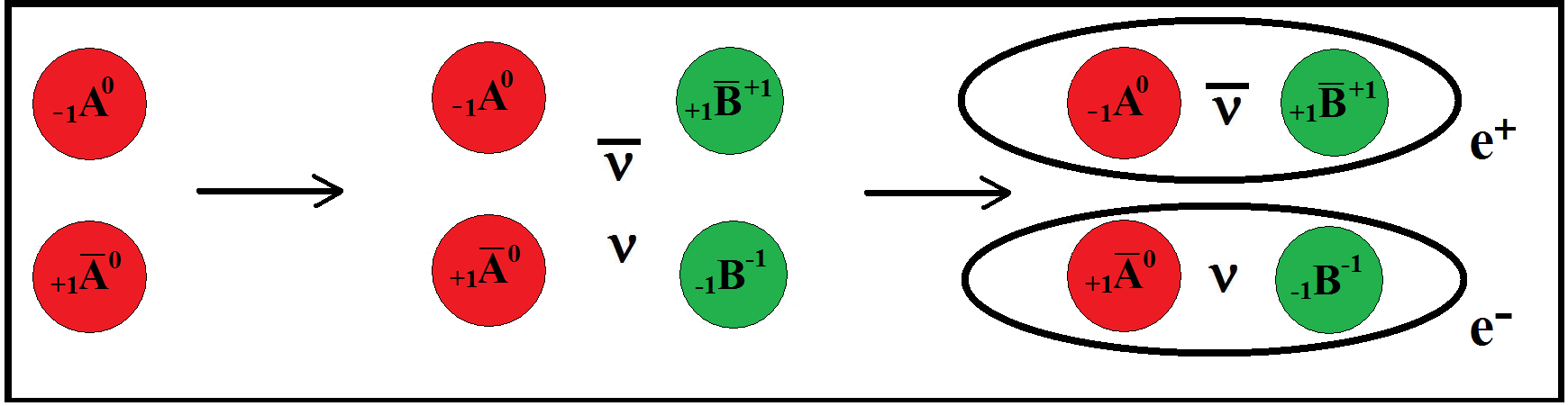
A second possible outcome of a proton antiproton collision is shown above. In this case, we note that it is possible for an A particle to be knocked off of a proton and an anti-A to be knocked off of an antiproton. In this case, a B anti-B pair and a pair of neutrinos can be formed from the vacuum. The B and one neutrino will bind with the anti-A to form a massive lepton, while the anti-B and the other neutrino will bind with the A particle to form a massive anti-lepton. This is exactly one of the signatures found for the Z particle in high energy physics experiments. (The Z can also form quark anti-quark pairs via formation of C anti-C preon pairs and additional neutrinos.)
The Z particle mass times the speed of light squared has been measured to be about 91.2 GeV. Since in the ABC Preon Model this phenomena is caused by the freeing of an A and an anti-A preon, this allows us to arrive at the relation that twice the mass of the A preon is 91.2 GeV/c^2. (The anti-preons masses are assumed to be the same as the mass of their corresponding preon.) Hence, the mass of the A preon is determined to be 45.6 GeV/c^2, and from our relationship derived above concerning "W Events" we can determine that the mass of the B preon is 34.8 GeV/c^2.
And now we see that what has been thought of as the W and Z discoveries are something quite different as understood from the ABC Preon Model. From the understanding of the ABC Preon Model, what were known as W events and Z events were actually the first experimental discovery of free preons!
With weak decays now understood (see my previous thread), it is time to turn to the experimental record given by high energy physics experiments. The first class of high energy physics experiments to discuss are proton antiproton collision experiments. Such experiments were done in the European laboratory CERN in 1983 and they led to the discovery of the W and Z particles of the standard model. Carlo Rubbia and Simon van der Meer received Nobel prizes for their important contributions to these discoveries. However, was it really a W and Z that were found? Or can these monumental discoveries have a different explanation?
In a proton antiproton collider, a beam of protons is accelerated to nearly the speed of light, and a beam of antiprotons is also accelerated to nearly the speed of light. The beams are sent in opposing directions and made to collide. Particles formed by the collision are then studied in large detectors.

The picture above shows a proton antiproton collision as understood from the ABC Preon Model. A proton is shown on the left, and an antiproton is shown in the center. From our earlier post on Modeling the Hadrons we recall that a proton is made of a central C particle, two A's and a B, while the antiproton is the antimatter counterpart of the proton. After colliding, these particles can create many different combinations of particles.
As a first possible outcome, let's look at what is known as a W Event:

One clear possibility for a proton antiproton collision is for it to result in what is shown above, where a B preon gets knocked off of a proton and an anti-A preon gets knocked off of an antiproton. Next, a pair of neutrinos can form out of the vacuum, with one going to bind the B and anti-A into a massive lepton, and the other neutrino being free. (Massive leptons are a B bound to an anti-A by a neutrino, see The ABC Preon Model. Modeling the Massive Leptons. for more details.)
Note that vacuum creation of particle anti-particle pairs is a very frequent occurance in high energy physics experiments. It happens all the time. And so the important point here is that we can readily see how proton antiproton collisions can result in a situation where we have a massive lepton and a neutrino formed. This, along with the remaining shower of particles caused by the other proton and antiproton fragments that are left over, is what has been discovered as evidence of the W particle in proton antiproton colliders.
The W particle mass times the speed of light squared has been measured to be about 80.4 GeV. Since in the ABC Preon Model this phenomena is caused by the freeing of an A and a B preon, this allows us to arrive at the relation that the mass of the A particle plus the mass of the B particle is 80.4 GeV/c^2.
Next, let's look at what is known as a Z Event:

A second possible outcome of a proton antiproton collision is shown above. In this case, we note that it is possible for an A particle to be knocked off of a proton and an anti-A to be knocked off of an antiproton. In this case, a B anti-B pair and a pair of neutrinos can be formed from the vacuum. The B and one neutrino will bind with the anti-A to form a massive lepton, while the anti-B and the other neutrino will bind with the A particle to form a massive anti-lepton. This is exactly one of the signatures found for the Z particle in high energy physics experiments. (The Z can also form quark anti-quark pairs via formation of C anti-C preon pairs and additional neutrinos.)
The Z particle mass times the speed of light squared has been measured to be about 91.2 GeV. Since in the ABC Preon Model this phenomena is caused by the freeing of an A and an anti-A preon, this allows us to arrive at the relation that twice the mass of the A preon is 91.2 GeV/c^2. (The anti-preons masses are assumed to be the same as the mass of their corresponding preon.) Hence, the mass of the A preon is determined to be 45.6 GeV/c^2, and from our relationship derived above concerning "W Events" we can determine that the mass of the B preon is 34.8 GeV/c^2.
And now we see that what has been thought of as the W and Z discoveries are something quite different as understood from the ABC Preon Model. From the understanding of the ABC Preon Model, what were known as W events and Z events were actually the first experimental discovery of free preons!
10. Physics in Electron Positron Colliders. A Prediction.
In the previous thread we took an initial look at the results from proton antiproton colliders. But another branch of high energy physics experimentation involves experiments done in electron positron colliders.
Above we see the process of an electron positron collision as modeled by the ABC preon model. An electron, composed of an A anti-preon and a B preon, collides with a positron, which is an A preon and an anti-B preon. We've seen in the prior thread how the production of a free A anti-A pair leads to what is known as the Z Signature in proton antiproton colliders, and here we can see that the same production will take place in electron positron colliders.
The Z signature will result when the mass of the collision is such that the B and anti-B annihilate, leaving a free A anti-A pair. Once that happens, a B anti-B pair and a neutrino pair can be produced from vacuum, leading to two leptons which is one signature for the Z particle. But in addition to forming a lepton pair, it is also possible that C anti-C pairs can form from vacuum as well, and that will lead to various quark anti-quark combinations. Hence, it can be noted that the ABC preon model nicely dovetails with the Standard Model. All of the various lepton and quark production channels known to exist in the standard model can also be seen to occur through the ABC Preon Model for what are known as Z events. Of course this is the idea behind all preon models. The idea is that a simpler precursor model underlying the quarks and leptons will reproduce known results by leading to an understanding of the composition of what are now known as quarks and leptons.
So it is clear that A anti-A pairs can be easily seen to result from electron positron colliders, and we can see how that production is consistent with known experiments. But note that it should also be possible to produce B anti-B pairs as well, through annihilation of the A anti-A portions of the electron and positron. In that case, massive leptons will be produced through A anti-A and neutrino pair creation, and quark anti-quark pairs will also be produced through C anti-C and neutrino pair production. This leads to a prediction for new physics from the ABC Preon Model. A signature that has the same decay channels as the Z signature should also exist at 69.6 GeV in electron positron colliders. Note that it may not be as strong a signal as the one at 91.2 GeV, but it should exist.
As a reminder of where the energies 69.6 GeV and 91.2 GeV come from, recall that the prior thread showed us that the mass of the B is 34.8 GeV/c^2 and that the mass of the A is 45.6 GeV/c^2. A B anti-B formation will occur at twice the mass of the B times c^2, or 69.6 GeV. An A anti-A formation will occur at twice the mass of the A times c^2, or 91.2 GeV.
And now let's return to a look at proton antiproton collisions. We saw in the prior thread how the ABC preon model leads to an understanding of what are presently described as W events through the formation of an anti-A preon and a B preon. We also saw how the model led to an understanding of what are presently described as Z events through the formation of an A anti-A preon pair. But as was the case for the electron positron collisions, it should also be possible to form a B anti-B preon pair in proton antiproton colliders. Hence, the same decay channels seen at the 91.2 GeV Z peak should also be seen at 69.6 GeV in proton antiproton colliders. Since there are only half as many B particles as there are A particles in the proton, and half again as many anti-B particles as anti-A particles in the antiproton, this will lead to only a quarter of the amount of production at the lower energy than there is at the high energy. Additionally, the cross section for production may be smaller, as evidenced by the more tightly bound B particles in the down family of quarks. But the signal should exist at some level.
In the previous thread we took an initial look at the results from proton antiproton colliders. But another branch of high energy physics experimentation involves experiments done in electron positron colliders.

Above we see the process of an electron positron collision as modeled by the ABC preon model. An electron, composed of an A anti-preon and a B preon, collides with a positron, which is an A preon and an anti-B preon. We've seen in the prior thread how the production of a free A anti-A pair leads to what is known as the Z Signature in proton antiproton colliders, and here we can see that the same production will take place in electron positron colliders.
The Z signature will result when the mass of the collision is such that the B and anti-B annihilate, leaving a free A anti-A pair. Once that happens, a B anti-B pair and a neutrino pair can be produced from vacuum, leading to two leptons which is one signature for the Z particle. But in addition to forming a lepton pair, it is also possible that C anti-C pairs can form from vacuum as well, and that will lead to various quark anti-quark combinations. Hence, it can be noted that the ABC preon model nicely dovetails with the Standard Model. All of the various lepton and quark production channels known to exist in the standard model can also be seen to occur through the ABC Preon Model for what are known as Z events. Of course this is the idea behind all preon models. The idea is that a simpler precursor model underlying the quarks and leptons will reproduce known results by leading to an understanding of the composition of what are now known as quarks and leptons.
So it is clear that A anti-A pairs can be easily seen to result from electron positron colliders, and we can see how that production is consistent with known experiments. But note that it should also be possible to produce B anti-B pairs as well, through annihilation of the A anti-A portions of the electron and positron. In that case, massive leptons will be produced through A anti-A and neutrino pair creation, and quark anti-quark pairs will also be produced through C anti-C and neutrino pair production. This leads to a prediction for new physics from the ABC Preon Model. A signature that has the same decay channels as the Z signature should also exist at 69.6 GeV in electron positron colliders. Note that it may not be as strong a signal as the one at 91.2 GeV, but it should exist.
As a reminder of where the energies 69.6 GeV and 91.2 GeV come from, recall that the prior thread showed us that the mass of the B is 34.8 GeV/c^2 and that the mass of the A is 45.6 GeV/c^2. A B anti-B formation will occur at twice the mass of the B times c^2, or 69.6 GeV. An A anti-A formation will occur at twice the mass of the A times c^2, or 91.2 GeV.
And now let's return to a look at proton antiproton collisions. We saw in the prior thread how the ABC preon model leads to an understanding of what are presently described as W events through the formation of an anti-A preon and a B preon. We also saw how the model led to an understanding of what are presently described as Z events through the formation of an A anti-A preon pair. But as was the case for the electron positron collisions, it should also be possible to form a B anti-B preon pair in proton antiproton colliders. Hence, the same decay channels seen at the 91.2 GeV Z peak should also be seen at 69.6 GeV in proton antiproton colliders. Since there are only half as many B particles as there are A particles in the proton, and half again as many anti-B particles as anti-A particles in the antiproton, this will lead to only a quarter of the amount of production at the lower energy than there is at the high energy. Additionally, the cross section for production may be smaller, as evidenced by the more tightly bound B particles in the down family of quarks. But the signal should exist at some level.
11. Deep Inelastic Scattering. The C Mass.
In an earlier thread we investigated experiments that allow a determination of the masses of the A and B preons. In order to determine the mass of the C preon we turn to a look at deep inelastic scattering experiments. Deep inelastic scattering experiments are done by having a high energy electron beam collide with hadronic matter. For the case of the proton, these experiments resulted in observations consistent with 35% of the proton momentum being carried by particles with positive charge, about 18% by particles with negative charge, and 47% by particles of neutral charge. The standard model agrees with these values by assigning 35% of the proton momentum to be carried by positively charged up quarks, 18% of the proton momentum to be carried by negatively charged down quarks, and the remaining 47% to be carried by charge neutral gluons.
For the ABC Preon Model the situation will of course be a bit different. The proton as understood by the ABC Preon Model is shown below:

In the ABC Preon Model, it is readily observed that if we set the mass of the C particle to be 67.9 GeV/c^2, we have a situation where 35% of the mass of the proton consists of the positively charged C particle, 18% by the mass of the negatively charged B particle (34.8 GeV/c^2), and 47% by the mass of the two uncharged A particles (45.6 GeV/c^2 each, for a total of 91.2 GeV/c^2). Hence, by fitting a single parameter (the C mass) we obtain a good fit to all three data points. When developing this model, I was struck by this fact. There were not enough free parameters to fit the data, and therefore I took this to be a rather strong confirmation that the model is indeed representative of nature. The ABC Preon Model did not need an additional proposal to explain the result, such as the gluon proposal needed for the standard model interpretation. Also, like the quark model, the ABC Preon Model involves small particles within the proton that can serve as scattering centers for the hard scattering events discovered in the deep inelastic scattering experiments. It appeared to be an excellent fit between the ABC Preon Model and experiment.
The original publication of the ABC Preon Model appeared in the reviewed journal Physics Essays in 1997. At that time, the issue of deep inelastic scattering was treated no further than what is mentioned above. Yet while the ABC Preon Model readily explains the right division of momentum within the proton, deep inelastic scattering analysis involves more than just that simple top-level result. The Standard Model analysis of deep inelastic scattering goes much further and is quite detailed and complicated. (Ref. 1)).
There are a couple issues of concern when we dig further into deep inelastic scattering as it pertains to the ABC Preon Model. The first issue of concern is that the ABC Preon Model specifies that the small objects within the proton have masses on the scales of 10's of GeV, and that is much larger than the quark masses of the Standard Model. However note that early theorizing regarding deep inelastic scattering involved the possibility of heavy proton constituents. Quoting from one of the Nobel prize lectures (Ref. 2): "there was a serious problem in making the 'free' behavior of the constituents during photon absorption compatible with the required strong final state interaction. One of the ways to get out of this difficulty was to assign quarks very large masses" (Ref. 2, page 9 of the 24 page PDF, which is labeled as page 723.) The second issue of concern with the ABC Preon Model is that the "isospin symmetry" argument used in Ref 1 is now rather dubious, since in the ABC Preon Model the neutron will have a different number of charged scattering centers than does the proton. Although as far as neutrons are concerned, we should note that there are problems with understanding deep inelastic scattering still to this day. (See Ref. 1, bottom of page 11 of the 15 page PDF, which is labeled as page 197.)
Despite the above issues, there is reason to believe that the ABC Preon Model is consistent with the deep inelastic scattering experiments due similarities between it and the quark model. In the quark model of the proton, the ratio of the charge on the positively charged up quarks to the charge on the negatively charged down quarks is -2. (2/3rds to -1/3rd.) In the ABC Preon Model of the proton, the ratio of the charge on the positively charged C particle to the negatively charged B particle is also -2. (2 to -1). Also, the ratio of the mass of the positive charges within the proton to the mass of the negative charges within the proton is the same in both models. As a result of these facts, it should be possible to obtain a match to the experimental data by adjusting the assumed probabilities that underlie the calculation. Note that while Ref. 1 does not mention the assumed probabilities behind the deep inelastic scattering analysis, the Nobel prize lecture, Ref. 2, does. (See Ref. 2, P(N) on page 8 of the 24 page PDF, which is labeled as page 722.) Therefore the ABC Preon Model will continue to use the high level deep inelastic scattering results to set the C mass at 67.9 GeV/c^2, noting that this also results in the positive, negative and neutral constituents of the proton having the same contribution to proton momentum as they do in the present quark model.
With the masses for the A, B, and C preons now estimated, it is a good time to step back and take notice of an important aspect of the theory. Recall that we have postulated than the massive leptons are made up of an A antiparticle bound to a B particle with a force mediated by the neutrino. And yet we have seen that the mass of the A is 45.6 GeV/c^2 and the mass of the B is 34.8 GeV/c^2. But the mass of the lightest massive lepton, the electron, is only 511 keV/c^2. Therefore the ABC Preon Model is proposing that the constituents of the electron weigh about 100,000 times more than the electron itself! Of course such a situation is indeed entirely possible. Perhaps the best known equation in all of physics, incorrectly attributed to Einstein by the way, is the formula E = mc^2.
It is well known that the atomic nuclei that bind protons with neutrons have lower masses than the sum of the masses of the protons and neutrons making them up. The lighter mass is due to the effect of the binding energy between the protons and neutrons. Similarly, in the ABC Preon Model, the light mass of the electron shows that there is a considerable amount of binding energy between the A and the B. In fact, the binding energy is so large that the mass of the electron is only a small fraction of the mass of its constituents. This condition also holds for the hadrons and mesons, as they are much lighter than their constituents as well.
In an earlier thread we investigated experiments that allow a determination of the masses of the A and B preons. In order to determine the mass of the C preon we turn to a look at deep inelastic scattering experiments. Deep inelastic scattering experiments are done by having a high energy electron beam collide with hadronic matter. For the case of the proton, these experiments resulted in observations consistent with 35% of the proton momentum being carried by particles with positive charge, about 18% by particles with negative charge, and 47% by particles of neutral charge. The standard model agrees with these values by assigning 35% of the proton momentum to be carried by positively charged up quarks, 18% of the proton momentum to be carried by negatively charged down quarks, and the remaining 47% to be carried by charge neutral gluons.
For the ABC Preon Model the situation will of course be a bit different. The proton as understood by the ABC Preon Model is shown below:

In the ABC Preon Model, it is readily observed that if we set the mass of the C particle to be 67.9 GeV/c^2, we have a situation where 35% of the mass of the proton consists of the positively charged C particle, 18% by the mass of the negatively charged B particle (34.8 GeV/c^2), and 47% by the mass of the two uncharged A particles (45.6 GeV/c^2 each, for a total of 91.2 GeV/c^2). Hence, by fitting a single parameter (the C mass) we obtain a good fit to all three data points. When developing this model, I was struck by this fact. There were not enough free parameters to fit the data, and therefore I took this to be a rather strong confirmation that the model is indeed representative of nature. The ABC Preon Model did not need an additional proposal to explain the result, such as the gluon proposal needed for the standard model interpretation. Also, like the quark model, the ABC Preon Model involves small particles within the proton that can serve as scattering centers for the hard scattering events discovered in the deep inelastic scattering experiments. It appeared to be an excellent fit between the ABC Preon Model and experiment.
The original publication of the ABC Preon Model appeared in the reviewed journal Physics Essays in 1997. At that time, the issue of deep inelastic scattering was treated no further than what is mentioned above. Yet while the ABC Preon Model readily explains the right division of momentum within the proton, deep inelastic scattering analysis involves more than just that simple top-level result. The Standard Model analysis of deep inelastic scattering goes much further and is quite detailed and complicated. (Ref. 1)).
There are a couple issues of concern when we dig further into deep inelastic scattering as it pertains to the ABC Preon Model. The first issue of concern is that the ABC Preon Model specifies that the small objects within the proton have masses on the scales of 10's of GeV, and that is much larger than the quark masses of the Standard Model. However note that early theorizing regarding deep inelastic scattering involved the possibility of heavy proton constituents. Quoting from one of the Nobel prize lectures (Ref. 2): "there was a serious problem in making the 'free' behavior of the constituents during photon absorption compatible with the required strong final state interaction. One of the ways to get out of this difficulty was to assign quarks very large masses" (Ref. 2, page 9 of the 24 page PDF, which is labeled as page 723.) The second issue of concern with the ABC Preon Model is that the "isospin symmetry" argument used in Ref 1 is now rather dubious, since in the ABC Preon Model the neutron will have a different number of charged scattering centers than does the proton. Although as far as neutrons are concerned, we should note that there are problems with understanding deep inelastic scattering still to this day. (See Ref. 1, bottom of page 11 of the 15 page PDF, which is labeled as page 197.)
Despite the above issues, there is reason to believe that the ABC Preon Model is consistent with the deep inelastic scattering experiments due similarities between it and the quark model. In the quark model of the proton, the ratio of the charge on the positively charged up quarks to the charge on the negatively charged down quarks is -2. (2/3rds to -1/3rd.) In the ABC Preon Model of the proton, the ratio of the charge on the positively charged C particle to the negatively charged B particle is also -2. (2 to -1). Also, the ratio of the mass of the positive charges within the proton to the mass of the negative charges within the proton is the same in both models. As a result of these facts, it should be possible to obtain a match to the experimental data by adjusting the assumed probabilities that underlie the calculation. Note that while Ref. 1 does not mention the assumed probabilities behind the deep inelastic scattering analysis, the Nobel prize lecture, Ref. 2, does. (See Ref. 2, P(N) on page 8 of the 24 page PDF, which is labeled as page 722.) Therefore the ABC Preon Model will continue to use the high level deep inelastic scattering results to set the C mass at 67.9 GeV/c^2, noting that this also results in the positive, negative and neutral constituents of the proton having the same contribution to proton momentum as they do in the present quark model.
With the masses for the A, B, and C preons now estimated, it is a good time to step back and take notice of an important aspect of the theory. Recall that we have postulated than the massive leptons are made up of an A antiparticle bound to a B particle with a force mediated by the neutrino. And yet we have seen that the mass of the A is 45.6 GeV/c^2 and the mass of the B is 34.8 GeV/c^2. But the mass of the lightest massive lepton, the electron, is only 511 keV/c^2. Therefore the ABC Preon Model is proposing that the constituents of the electron weigh about 100,000 times more than the electron itself! Of course such a situation is indeed entirely possible. Perhaps the best known equation in all of physics, incorrectly attributed to Einstein by the way, is the formula E = mc^2.
It is well known that the atomic nuclei that bind protons with neutrons have lower masses than the sum of the masses of the protons and neutrons making them up. The lighter mass is due to the effect of the binding energy between the protons and neutrons. Similarly, in the ABC Preon Model, the light mass of the electron shows that there is a considerable amount of binding energy between the A and the B. In fact, the binding energy is so large that the mass of the electron is only a small fraction of the mass of its constituents. This condition also holds for the hadrons and mesons, as they are much lighter than their constituents as well.
12. Not the Top Quark. Preons.
There is one experimental result where it may at first seem that the ABC Preon Model fails, and that involves the experimental evidence for the top quark. In fact, the abstract of the original 1997 publication ended with the final sentence "The model does not require the existence of the top quark." In this thread we will explore the meaning behind that final sentence, as well as present powerful additional evidence for the existence of preons.

Above we recall our earlier depiction of the delta-plus particle, wherein the up quark is identified as the binding of an A particle to a C particle. Also recall that the ABC Preon Model defines the charm quark as the first excited state of the A and C binding, and that the top quark would be the second excited state, should it exist.
But note that there should be no binding at all if the A can be freed from its bond at an energy less than that of a possible second exited state. As an approximate upper limit, it should not be possible to have a quark form at a mass much above twice the mass of the A, since putting that much energy into the bond should be enough to free the A from the C particle.
To see how this is so, observe that a free A will have a mass of m_A. Less obvious is that the remnant that the A escapes from will also have a mass estimated to be about m_A. This is because the energy of the bond reduces the total mass of the system containing both entities that participate in the bond, and here I propose an estimate that when freed each entity has a mass that is about equal to the other entity participating in the bond. Hence, if we put into the bond an amount of energy equal to about twice the mass of the A times c squared, the A will be freed. It may require somewhat more or less energy to free the A, but twice the mass of the A is a reasonable upper estimate for how heavy the top quark could be.
In an earlier thread we found that twice the mass of the A is 91.2 GeV/c^2, and yet the top quark has been reported to exist with a mass of 172.4 GeV/c^2. Since 172.4 GeV/c^2 is much more than 91.2 GeV/c^2, this would at first appear to be counter-indicative of the ABC Preon Model. In fact, for some years after the announced discovery of the top quark, I had thought that the likelihood of the ABC Preon Model being a correct model of nature had been considerably damaged experimentally by the proclaimed top quark discovery.
But then I noticed an important point, which is that the top quark is theorized to decay very quickly. The experimental evidence does not come from the top quark itself, but rather from its decay products, which in turn come from the decays of a bottom quark and a W boson. And it is clearly possible to create a bottom quark in conjunction with a W boson as understood from the ABC Preon Model.
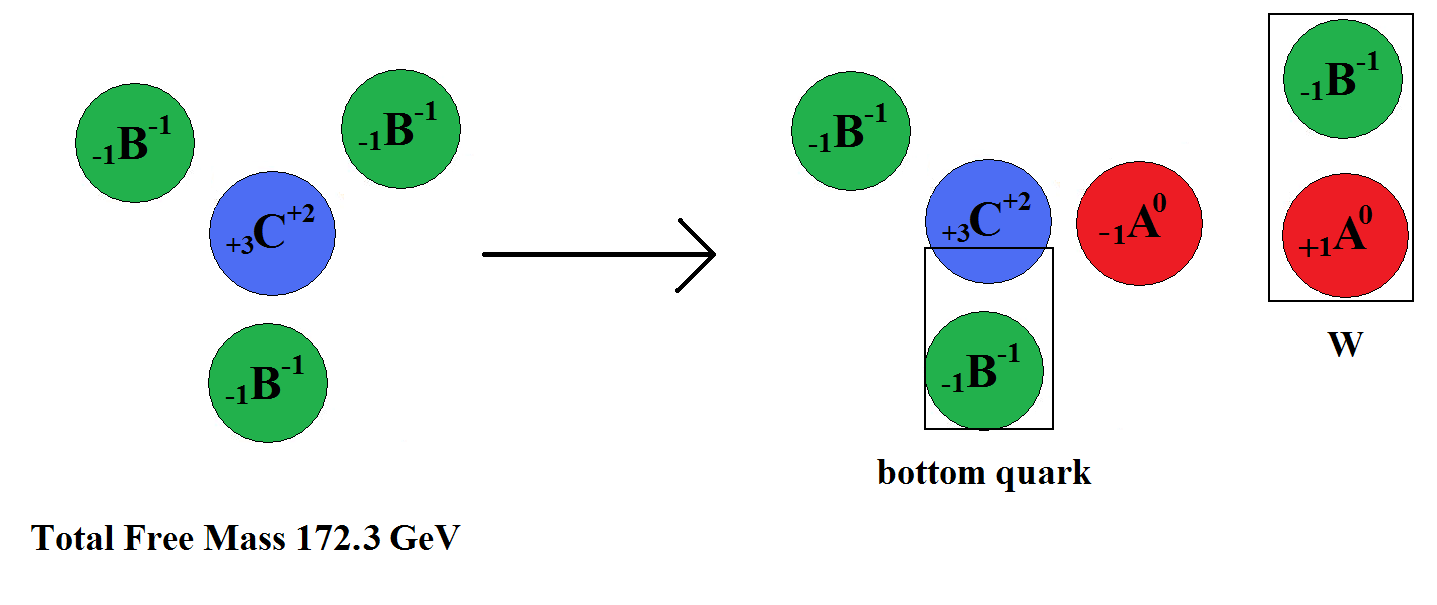
Above we see the case where a C particle and three B particles are produced in a high energy collision. Recall from our previous threads that the C preon has a mass of 67.9 GeV/c^2 while a B preon has a mass of 34.8 GeV/c^2. Hence, the combination of a C and three B's as shown above has a total mass of 67.9 GeV/c^2 + (3 x 34.8) GeV/c^2 = 172.3 GeV/c^2, which is an excellent fit to the observed mass associated with what is known as the top quark. (What is known as the top quark is observed at a mass of 172.4 GeV/c^2.)
In addition to getting the mass correct, it also is important what the decay products are. If an A anti-A pair forms out of vacuum, the anti-A B combination can be recognized as leading to the W signature (as shown in this earlier thread), and one of the C B bindings can readily be seen to be able to form a bottom quark. After formation of a W and a bottom quark, the remaining B and A preons as well as background production of other preon and neutrino pairs will hadronize the bottom quark, resulting in the signature identified as the top quark signature.
So the ABC Preon Model does indeed predict the top quark signature as found in high energy physics experiments, both qualitatively (it gets the correct decay channels) and quantitatively (the center of mass of the decay products is predicted). The explanation for the observed results are different from those of the Standard Model, since instead of a top quark forming, the ABC Preon Model recognizes the results as showing the formation of free preons.
The nearly exact match between the predictions of the ABC Preon Model and the experimental results presently known as the top quark signature provides significant additional support for the ABC Preon Model. In our previous thread we saw how the ABC Preon Model predicted all three deep inelastic scattering ratios by fitting a single parameter, the C mass, to the data. In this thread, we see that the ABC Preon Model predicts the top quark mass with no additional free parameter required.
As can be seen, the ABC Preon Model makes more predictions than what can be arrived at by setting the few free parameters that go into the model - which is leading to increasing evidence that the ABC Preon Model may indeed be the correct model for what makes up our world.
There is one experimental result where it may at first seem that the ABC Preon Model fails, and that involves the experimental evidence for the top quark. In fact, the abstract of the original 1997 publication ended with the final sentence "The model does not require the existence of the top quark." In this thread we will explore the meaning behind that final sentence, as well as present powerful additional evidence for the existence of preons.

Above we recall our earlier depiction of the delta-plus particle, wherein the up quark is identified as the binding of an A particle to a C particle. Also recall that the ABC Preon Model defines the charm quark as the first excited state of the A and C binding, and that the top quark would be the second excited state, should it exist.
But note that there should be no binding at all if the A can be freed from its bond at an energy less than that of a possible second exited state. As an approximate upper limit, it should not be possible to have a quark form at a mass much above twice the mass of the A, since putting that much energy into the bond should be enough to free the A from the C particle.
To see how this is so, observe that a free A will have a mass of m_A. Less obvious is that the remnant that the A escapes from will also have a mass estimated to be about m_A. This is because the energy of the bond reduces the total mass of the system containing both entities that participate in the bond, and here I propose an estimate that when freed each entity has a mass that is about equal to the other entity participating in the bond. Hence, if we put into the bond an amount of energy equal to about twice the mass of the A times c squared, the A will be freed. It may require somewhat more or less energy to free the A, but twice the mass of the A is a reasonable upper estimate for how heavy the top quark could be.
In an earlier thread we found that twice the mass of the A is 91.2 GeV/c^2, and yet the top quark has been reported to exist with a mass of 172.4 GeV/c^2. Since 172.4 GeV/c^2 is much more than 91.2 GeV/c^2, this would at first appear to be counter-indicative of the ABC Preon Model. In fact, for some years after the announced discovery of the top quark, I had thought that the likelihood of the ABC Preon Model being a correct model of nature had been considerably damaged experimentally by the proclaimed top quark discovery.
But then I noticed an important point, which is that the top quark is theorized to decay very quickly. The experimental evidence does not come from the top quark itself, but rather from its decay products, which in turn come from the decays of a bottom quark and a W boson. And it is clearly possible to create a bottom quark in conjunction with a W boson as understood from the ABC Preon Model.

Above we see the case where a C particle and three B particles are produced in a high energy collision. Recall from our previous threads that the C preon has a mass of 67.9 GeV/c^2 while a B preon has a mass of 34.8 GeV/c^2. Hence, the combination of a C and three B's as shown above has a total mass of 67.9 GeV/c^2 + (3 x 34.8) GeV/c^2 = 172.3 GeV/c^2, which is an excellent fit to the observed mass associated with what is known as the top quark. (What is known as the top quark is observed at a mass of 172.4 GeV/c^2.)
In addition to getting the mass correct, it also is important what the decay products are. If an A anti-A pair forms out of vacuum, the anti-A B combination can be recognized as leading to the W signature (as shown in this earlier thread), and one of the C B bindings can readily be seen to be able to form a bottom quark. After formation of a W and a bottom quark, the remaining B and A preons as well as background production of other preon and neutrino pairs will hadronize the bottom quark, resulting in the signature identified as the top quark signature.
So the ABC Preon Model does indeed predict the top quark signature as found in high energy physics experiments, both qualitatively (it gets the correct decay channels) and quantitatively (the center of mass of the decay products is predicted). The explanation for the observed results are different from those of the Standard Model, since instead of a top quark forming, the ABC Preon Model recognizes the results as showing the formation of free preons.
The nearly exact match between the predictions of the ABC Preon Model and the experimental results presently known as the top quark signature provides significant additional support for the ABC Preon Model. In our previous thread we saw how the ABC Preon Model predicted all three deep inelastic scattering ratios by fitting a single parameter, the C mass, to the data. In this thread, we see that the ABC Preon Model predicts the top quark mass with no additional free parameter required.
As can be seen, the ABC Preon Model makes more predictions than what can be arrived at by setting the few free parameters that go into the model - which is leading to increasing evidence that the ABC Preon Model may indeed be the correct model for what makes up our world.
13. Higgs Boson (Actually Free Preon) Decay Channels.
The discovery of the Higgs Boson was announced in 2012. But, similar to the top quark discovery which was discussed in the previous thread of this series, the discovery of the Higgs actually involves finding decay products, not the Higgs itself, since the Higgs decays too quickly to appear within the detector apparatus.
And once again, just as with the top quark, the ABC Preon Model can explain what is known as the Higgs signature by recognizing the formation of free preons; this time involving an A, a B and an anti-A. The combined mass of these three preons is twice the mass of the A (2 times 45.6 GeV/c2 = 91.2 GeV/c2) plus the mass of the B (34.8 eV/c2) which leads to a total of 126.0 GeV/c2 – consistent with the mass measured for what is known as the Higgs. But while the mass is very important to predict, it is equally important that the decay channels predicted are also those that are observed.
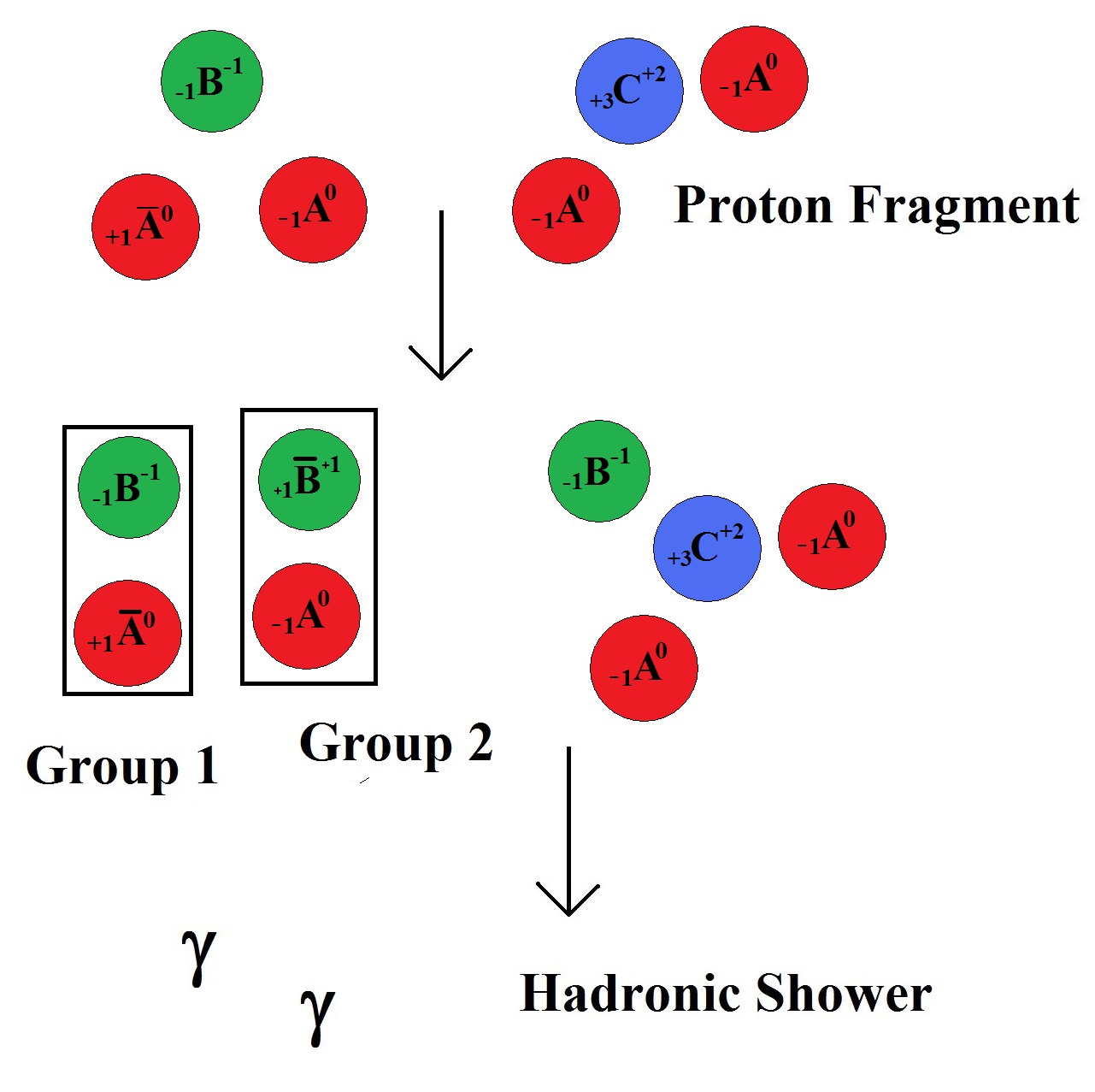
One of the observed decay channels is shown above. The A, B, and anti-A preons are formed from a high energy collision, with the B being knocked out of a proton and the A and anti-A being formed from the energy of the collision. What is left of the proton, now without its B preon, is shown as well. Then, a B / anti-B pair (virtual, or off-shell) forms out of vacuum. The B combines with what was left of the proton to create a hadronic shower. (High energy physics events often result in hadronic showers since the violence of the collision rips apart the colliding particles. While clean decay products may result from a portion of what is ripped apart, the remainder must combine into hadrons, and this frequently leads to a shower of particles.)
What is left after we use up the particles that create the hadronic shower are an A, a B, an anti-A and an anti-B. The figure above shows those four particles grouped in such a way so as to result in what is understood to be the preonic constituents of the massive leptons.(See thread 2 in this series for how massive leptons are modeled.) Lepton anti-lepton annihilation to two photons is commonly known - and so the above groupings show us how a two photon decay channel results from the original free A, B, and anti-A.
The above figure can also be used to explain W pair production. If a neutrino/neutrino pair forms in association with Group 1 and another neutrino/neutrino pair forms in association with Group 2, then W pair production results, since that combination of particles in each group lead to W signatures as was explained in thread 9 of this series.
The above figure can also be used to explain lepton/anti-lepton pair production. In that case only a single neutrino/neutrino pair forms, with one neutrino combining with the Group 1 preons to form a lepton and the other neutrino combining with the Group 2 preons to form an anti-lepton. (Again refer to thread 2 in this series for a description of the modeling of the massive leptons).
The above figure can also be used to explain quark/anti-quark production, which will occur if the B and anti-B annihilate into a C and anti-C followed by neutrino/neutrino production out of vacuum, with the C, neutrino and remaining A forming a quark and the anti-C, neutrino and remaining anti-A forming an anti-quark. (See thread 5 in this series for a description of the modeling of quarks). Note that what has just been described will result in quark/anti-quark pairs of the up family. If instead the A annihilates with the anti-A into a C and anti-C, the remaining B and anti-B will combine with the C and anti-C to form the down family of quark/anti-quark pairs instead.
There is also another possible decay channel for the free A, B, and anti-A preons as shown below:
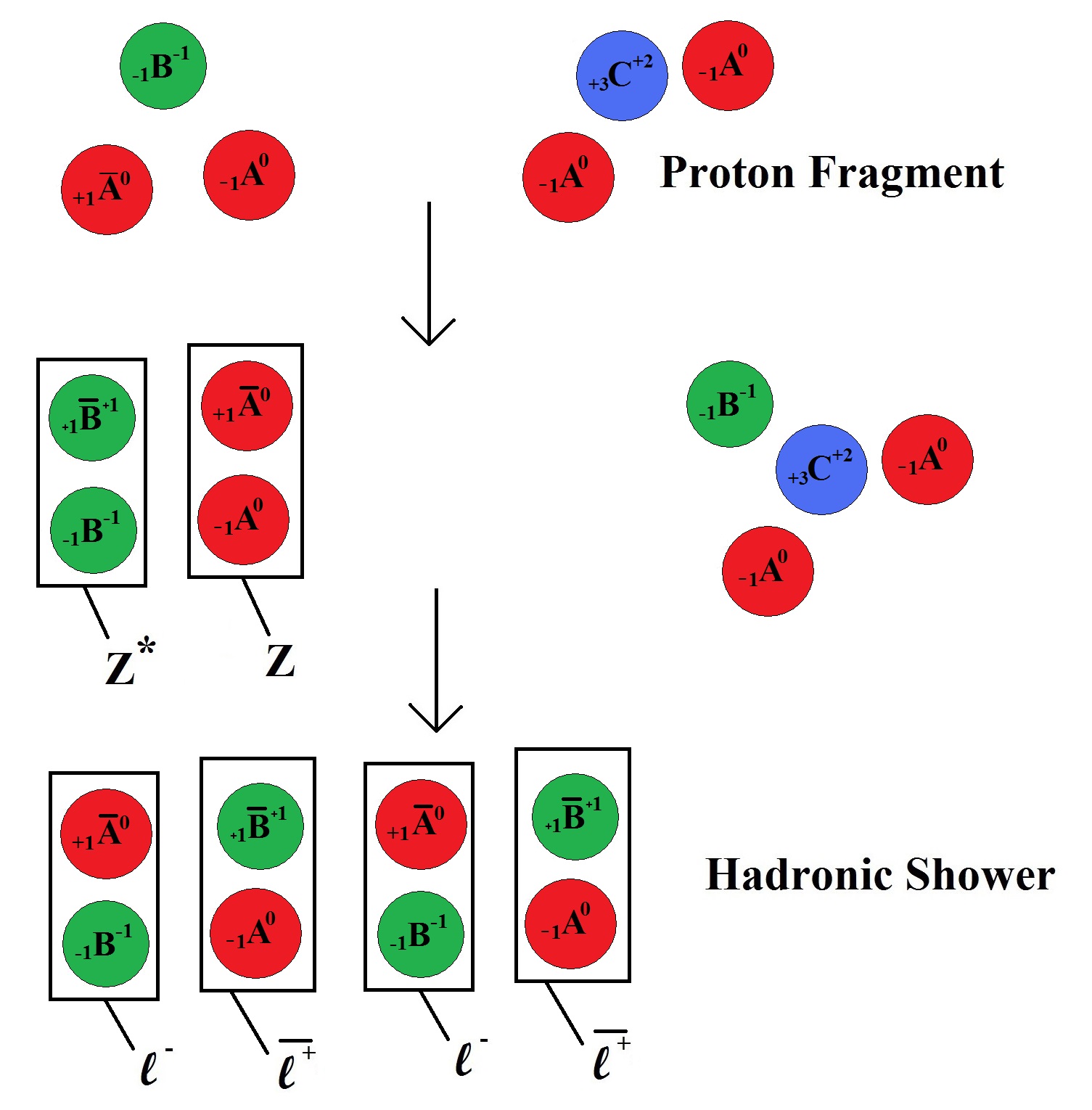
The above figure shows how four high energy leptons will be produced from a free A, B, and anti-A as understood by the ABC Preon Model. Vacuum creation of a B / anti-B pair enables one B to combine with a portion of the proton fragments, leading to a hadronic shower just as in the earlier figure. This time however, we group the A with the anti-A and the B with the anti-B. The A/anti-A pair is understood within the ABC Preon Model to be what leads to the various Z signatures (See thread 9 of this series which discussed Z formation). One of the predictions of the 1997 ABC Preon Model publication is the B/anti-B pair, labeled Z* in the figure above. The Z* has not yet been seen in isolation, and it will lead to signatures similar to those produced by an A/anti-A pair, only at somewhat lower energy. (See thread 10 of this series for more on the prediction of the Z*).
In one of the free A/anti-A pair decay modes, a B/anti-B pair and a neutrino/neutrino pair will form out of vacuum resulting in the components needed to make a lepton/antilepton pair. In one of the B/anti-B pair decay modes an A/anti-A pair and a neutrino/neutrino pair will form out of vacuum and join with the B/anti-B pair resulting in the components needed to make a second lepton/antilepton pair. Other decay modes are possible, such as the Z and Z* forming quark / anti-quark pairs via vacuum formation of C / anti-C preon pairs and additional neutrinos.
Once again, just as was the case of the top quark signature discussed in the previous thread, the ABC Preon Model predicts all of the decay modes seen in nature, this time for what is known as the Higgs signature.
The discovery of the Higgs Boson was announced in 2012. But, similar to the top quark discovery which was discussed in the previous thread of this series, the discovery of the Higgs actually involves finding decay products, not the Higgs itself, since the Higgs decays too quickly to appear within the detector apparatus.
And once again, just as with the top quark, the ABC Preon Model can explain what is known as the Higgs signature by recognizing the formation of free preons; this time involving an A, a B and an anti-A. The combined mass of these three preons is twice the mass of the A (2 times 45.6 GeV/c2 = 91.2 GeV/c2) plus the mass of the B (34.8 eV/c2) which leads to a total of 126.0 GeV/c2 – consistent with the mass measured for what is known as the Higgs. But while the mass is very important to predict, it is equally important that the decay channels predicted are also those that are observed.

One of the observed decay channels is shown above. The A, B, and anti-A preons are formed from a high energy collision, with the B being knocked out of a proton and the A and anti-A being formed from the energy of the collision. What is left of the proton, now without its B preon, is shown as well. Then, a B / anti-B pair (virtual, or off-shell) forms out of vacuum. The B combines with what was left of the proton to create a hadronic shower. (High energy physics events often result in hadronic showers since the violence of the collision rips apart the colliding particles. While clean decay products may result from a portion of what is ripped apart, the remainder must combine into hadrons, and this frequently leads to a shower of particles.)
What is left after we use up the particles that create the hadronic shower are an A, a B, an anti-A and an anti-B. The figure above shows those four particles grouped in such a way so as to result in what is understood to be the preonic constituents of the massive leptons.(See thread 2 in this series for how massive leptons are modeled.) Lepton anti-lepton annihilation to two photons is commonly known - and so the above groupings show us how a two photon decay channel results from the original free A, B, and anti-A.
The above figure can also be used to explain W pair production. If a neutrino/neutrino pair forms in association with Group 1 and another neutrino/neutrino pair forms in association with Group 2, then W pair production results, since that combination of particles in each group lead to W signatures as was explained in thread 9 of this series.
The above figure can also be used to explain lepton/anti-lepton pair production. In that case only a single neutrino/neutrino pair forms, with one neutrino combining with the Group 1 preons to form a lepton and the other neutrino combining with the Group 2 preons to form an anti-lepton. (Again refer to thread 2 in this series for a description of the modeling of the massive leptons).
The above figure can also be used to explain quark/anti-quark production, which will occur if the B and anti-B annihilate into a C and anti-C followed by neutrino/neutrino production out of vacuum, with the C, neutrino and remaining A forming a quark and the anti-C, neutrino and remaining anti-A forming an anti-quark. (See thread 5 in this series for a description of the modeling of quarks). Note that what has just been described will result in quark/anti-quark pairs of the up family. If instead the A annihilates with the anti-A into a C and anti-C, the remaining B and anti-B will combine with the C and anti-C to form the down family of quark/anti-quark pairs instead.
There is also another possible decay channel for the free A, B, and anti-A preons as shown below:

The above figure shows how four high energy leptons will be produced from a free A, B, and anti-A as understood by the ABC Preon Model. Vacuum creation of a B / anti-B pair enables one B to combine with a portion of the proton fragments, leading to a hadronic shower just as in the earlier figure. This time however, we group the A with the anti-A and the B with the anti-B. The A/anti-A pair is understood within the ABC Preon Model to be what leads to the various Z signatures (See thread 9 of this series which discussed Z formation). One of the predictions of the 1997 ABC Preon Model publication is the B/anti-B pair, labeled Z* in the figure above. The Z* has not yet been seen in isolation, and it will lead to signatures similar to those produced by an A/anti-A pair, only at somewhat lower energy. (See thread 10 of this series for more on the prediction of the Z*).
In one of the free A/anti-A pair decay modes, a B/anti-B pair and a neutrino/neutrino pair will form out of vacuum resulting in the components needed to make a lepton/antilepton pair. In one of the B/anti-B pair decay modes an A/anti-A pair and a neutrino/neutrino pair will form out of vacuum and join with the B/anti-B pair resulting in the components needed to make a second lepton/antilepton pair. Other decay modes are possible, such as the Z and Z* forming quark / anti-quark pairs via vacuum formation of C / anti-C preon pairs and additional neutrinos.
Once again, just as was the case of the top quark signature discussed in the previous thread, the ABC Preon Model predicts all of the decay modes seen in nature, this time for what is known as the Higgs signature.
14. Prediction for the Higgs Mass.
The discovery of the Higgs Boson was announced in 2012. But, similar to the top quark discovery which was discussed in thread 12 of this series, the discovery of the Higgs actually involves finding decay products, not the Higgs itself, since the Higgs decays too quickly to appear within the detector apparatus. In thread 13 we looked at the decay channels of what is presently known as the Higgs Boson, and we saw how all of those decay channels are predicted by the ABC Preon Model. In this thread we will look at the other important aspect of the discovery announced in 2012 - its mass.
In thread 13 it was shown that what is known as the Higgs signature instead arises from an A, an anti-A and a B preon when understood from the ABC Preon Model. In thread 9 it is shown that the mass of an anti-A plus the mass of a B equals m_W, where m_W is the mass of what is known as the W boson, and that the mass of the A is equal to m_Z/2, where m_Z is the mass of what is known as the Z boson. Hence, by the ABC Preon Model, the mass of what is known as the Higgs should be m_W + m_Z/2, or 126.0 GeV/c^2, or 125.98 +/- 0.02 GeV/c^2 if we expand things to another significant digit.
At the present time, the Higgs mass is claimed to be 125.09 +/- 0.24 GeV/c^2(Ref. 1). This result is between three and four standard deviations away from what the ABC Preon Model predicts for the mass. However, it is important now to take a deeper look at Ref. 1.
Above is a figure extracted from Ref. 1. As can be seen, the presently claimed Higgs mass comes from four separate experimental result sets, with two experiments from each of two collaborations. The ATLAS collaboration results of the two photon decay channel measured the Higgs mass to be 126.02+/-0.51 GeV/c^2. The CMS collaboration results of the two photon decay channel measured the Higgs mass to be 124.70+/-0.34 GeV/c^2. The ATLAS result for the four lepton decay channel measured the Higgs mass to be 124.51+/-0.52 GeV/c^2. And the CMS result for the four lepton decay channel measured the Higgs mass to be 125.59+/-0.45 GeV/c^2. These four results are then combined to arrive at the final result of 125.09 +/- 0.24 GeV/c^2.
On Arbitrageur's most excellent AMA thread Arbitrageur, ErosA433 and I had a discussion concerning the error estimate given in Ref. 1 for the combined result. I argued my position that the real error estimate should be significantly larger than the +/- 0.24 GeV/c^2 that is proclaimed. Click here if you wish to get into the details of that discussion.
As can be seen, the ABC Preon Model mass prediction of 126.0 GeV/c^2 matches the ATLAS two photon result nearly exactly, is about four standard deviations away from the CMS two photon result, about three standard deviations away from the ALTAS four lepton result and within one standard deviation of the CMS four lepton result. Given the results and their spread of values, I believe it is entirely possible that further experimentation will show a Higgs mass close to 126 GeV/c^2 because so many other experimental results are lining up to support the ABC Preon Model.
One of the reasons for my optimism that the eventual Higgs mass will be close to 126 GeV/c^2 comes from what happened with the W, Z, and top quark masses. Several years ago the derived masses of the A, B and C preons, (which are based on experimental values of the W mass, Z mass and deep inelastic scattering momentum partitions) led to a top quark prediction that was off by about 1 GeV/c^2. With the passage of time, further experimentation led to better estimates for the W, Z, and top quark masses. And with those better mass estimates the ABC Preon Model prediction is now in excellent agreement with measurements of what is known as the top quark mass. I believe a similar evolution will be seen in the mass of what is known as the Higgs, eventually bringing it into agreement with the prediction of the ABC Preon Model.
The discovery of the Higgs Boson was announced in 2012. But, similar to the top quark discovery which was discussed in thread 12 of this series, the discovery of the Higgs actually involves finding decay products, not the Higgs itself, since the Higgs decays too quickly to appear within the detector apparatus. In thread 13 we looked at the decay channels of what is presently known as the Higgs Boson, and we saw how all of those decay channels are predicted by the ABC Preon Model. In this thread we will look at the other important aspect of the discovery announced in 2012 - its mass.
In thread 13 it was shown that what is known as the Higgs signature instead arises from an A, an anti-A and a B preon when understood from the ABC Preon Model. In thread 9 it is shown that the mass of an anti-A plus the mass of a B equals m_W, where m_W is the mass of what is known as the W boson, and that the mass of the A is equal to m_Z/2, where m_Z is the mass of what is known as the Z boson. Hence, by the ABC Preon Model, the mass of what is known as the Higgs should be m_W + m_Z/2, or 126.0 GeV/c^2, or 125.98 +/- 0.02 GeV/c^2 if we expand things to another significant digit.
At the present time, the Higgs mass is claimed to be 125.09 +/- 0.24 GeV/c^2(Ref. 1). This result is between three and four standard deviations away from what the ABC Preon Model predicts for the mass. However, it is important now to take a deeper look at Ref. 1.

Above is a figure extracted from Ref. 1. As can be seen, the presently claimed Higgs mass comes from four separate experimental result sets, with two experiments from each of two collaborations. The ATLAS collaboration results of the two photon decay channel measured the Higgs mass to be 126.02+/-0.51 GeV/c^2. The CMS collaboration results of the two photon decay channel measured the Higgs mass to be 124.70+/-0.34 GeV/c^2. The ATLAS result for the four lepton decay channel measured the Higgs mass to be 124.51+/-0.52 GeV/c^2. And the CMS result for the four lepton decay channel measured the Higgs mass to be 125.59+/-0.45 GeV/c^2. These four results are then combined to arrive at the final result of 125.09 +/- 0.24 GeV/c^2.
On Arbitrageur's most excellent AMA thread Arbitrageur, ErosA433 and I had a discussion concerning the error estimate given in Ref. 1 for the combined result. I argued my position that the real error estimate should be significantly larger than the +/- 0.24 GeV/c^2 that is proclaimed. Click here if you wish to get into the details of that discussion.
As can be seen, the ABC Preon Model mass prediction of 126.0 GeV/c^2 matches the ATLAS two photon result nearly exactly, is about four standard deviations away from the CMS two photon result, about three standard deviations away from the ALTAS four lepton result and within one standard deviation of the CMS four lepton result. Given the results and their spread of values, I believe it is entirely possible that further experimentation will show a Higgs mass close to 126 GeV/c^2 because so many other experimental results are lining up to support the ABC Preon Model.
One of the reasons for my optimism that the eventual Higgs mass will be close to 126 GeV/c^2 comes from what happened with the W, Z, and top quark masses. Several years ago the derived masses of the A, B and C preons, (which are based on experimental values of the W mass, Z mass and deep inelastic scattering momentum partitions) led to a top quark prediction that was off by about 1 GeV/c^2. With the passage of time, further experimentation led to better estimates for the W, Z, and top quark masses. And with those better mass estimates the ABC Preon Model prediction is now in excellent agreement with measurements of what is known as the top quark mass. I believe a similar evolution will be seen in the mass of what is known as the Higgs, eventually bringing it into agreement with the prediction of the ABC Preon Model.
15. More Predictions.
The discovery of the Higgs Boson was announced in 2012. But, similar to the top quark discovery which was discussed in thread 12 of this series, the discovery of the Higgs actually involves finding decay products, not the Higgs itself, since the Higgs decays too quickly to appear within the detector apparatus. In thread 13 we looked at the decay channels of what is presently known as the Higgs Boson, and we saw how all of those decay channels are predicted by the ABC Preon Model. In this thread we will look at the other important aspect of the discovery announced in 2012 - its mass.
In thread 13 it was shown that what is known as the Higgs signature instead arises from an A, an anti-A and a B preon when understood from the ABC Preon Model. In thread 9 it is shown that the mass of an anti-A plus the mass of a B equals m_W, where m_W is the mass of what is known as the W boson, and that the mass of the A is equal to m_Z/2, where m_Z is the mass of what is known as the Z boson. Hence, by the ABC Preon Model, the mass of what is known as the Higgs should be m_W + m_Z/2, or 126.0 GeV/c^2, or 125.98 +/- 0.02 GeV/c^2 if we expand things to another significant digit.
At the present time, the Higgs mass is claimed to be 125.09 +/- 0.24 GeV/c^2(Ref. 1). This result is between three and four standard deviations away from what the ABC Preon Model predicts for the mass. However, it is important now to take a deeper look at Ref. 1.

Above is a figure extracted from Ref. 1. As can be seen, the presently claimed Higgs mass comes from four separate experimental result sets, with two experiments from each of two collaborations. The ATLAS collaboration results of the two photon decay channel measured the Higgs mass to be 126.02+/-0.51 GeV/c^2. The CMS collaboration results of the two photon decay channel measured the Higgs mass to be 124.70+/-0.34 GeV/c^2. The ATLAS result for the four lepton decay channel measured the Higgs mass to be 124.51+/-0.52 GeV/c^2. And the CMS result for the four lepton decay channel measured the Higgs mass to be 125.59+/-0.45 GeV/c^2. These four results are then combined to arrive at the final result of 125.09 +/- 0.24 GeV/c^2.
On Arbitrageur's most excellent AMA thread Arbitrageur, ErosA433 and I had a discussion concerning the error estimate given in Ref. 1 for the combined result. I argued my position that the real error estimate should be significantly larger than the +/- 0.24 GeV/c^2 that is proclaimed. Click here if you wish to get into the details of that discussion.
As can be seen, the ABC Preon Model mass prediction of 126.0 GeV/c^2 matches the ATLAS two photon result nearly exactly, is about four standard deviations away from the CMS two photon result, about three standard deviations away from the ALTAS four lepton result and within one standard deviation of the CMS four lepton result. Given the results and their spread of values, I believe it is entirely possible that further experimentation will show a Higgs mass close to 126 GeV/c^2 because so many other experimental results are lining up to support the ABC Preon Model.
One of the reasons for my optimism that the eventual Higgs mass will be close to 126 GeV/c^2 comes from what happened with the W, Z, and top quark masses. Several years ago the derived masses of the A, B and C preons, (which are based on experimental values of the W mass, Z mass and deep inelastic scattering momentum partitions) led to a top quark prediction that was off by about 1 GeV/c^2. With the passage of time, further experimentation led to better estimates for the W, Z, and top quark masses. And with those better mass estimates the ABC Preon Model prediction is now in excellent agreement with measurements of what is known as the top quark mass. I believe a similar evolution will be seen in the mass of what is known as the Higgs, eventually bringing it into agreement with the prediction of the ABC Preon Model.
The discovery of the Higgs Boson was announced in 2012. But, similar to the top quark discovery which was discussed in thread 12 of this series, the discovery of the Higgs actually involves finding decay products, not the Higgs itself, since the Higgs decays too quickly to appear within the detector apparatus. In thread 13 we looked at the decay channels of what is presently known as the Higgs Boson, and we saw how all of those decay channels are predicted by the ABC Preon Model. In this thread we will look at the other important aspect of the discovery announced in 2012 - its mass.
In thread 13 it was shown that what is known as the Higgs signature instead arises from an A, an anti-A and a B preon when understood from the ABC Preon Model. In thread 9 it is shown that the mass of an anti-A plus the mass of a B equals m_W, where m_W is the mass of what is known as the W boson, and that the mass of the A is equal to m_Z/2, where m_Z is the mass of what is known as the Z boson. Hence, by the ABC Preon Model, the mass of what is known as the Higgs should be m_W + m_Z/2, or 126.0 GeV/c^2, or 125.98 +/- 0.02 GeV/c^2 if we expand things to another significant digit.
At the present time, the Higgs mass is claimed to be 125.09 +/- 0.24 GeV/c^2(Ref. 1). This result is between three and four standard deviations away from what the ABC Preon Model predicts for the mass. However, it is important now to take a deeper look at Ref. 1.

Above is a figure extracted from Ref. 1. As can be seen, the presently claimed Higgs mass comes from four separate experimental result sets, with two experiments from each of two collaborations. The ATLAS collaboration results of the two photon decay channel measured the Higgs mass to be 126.02+/-0.51 GeV/c^2. The CMS collaboration results of the two photon decay channel measured the Higgs mass to be 124.70+/-0.34 GeV/c^2. The ATLAS result for the four lepton decay channel measured the Higgs mass to be 124.51+/-0.52 GeV/c^2. And the CMS result for the four lepton decay channel measured the Higgs mass to be 125.59+/-0.45 GeV/c^2. These four results are then combined to arrive at the final result of 125.09 +/- 0.24 GeV/c^2.
On Arbitrageur's most excellent AMA thread Arbitrageur, ErosA433 and I had a discussion concerning the error estimate given in Ref. 1 for the combined result. I argued my position that the real error estimate should be significantly larger than the +/- 0.24 GeV/c^2 that is proclaimed. Click here if you wish to get into the details of that discussion.
As can be seen, the ABC Preon Model mass prediction of 126.0 GeV/c^2 matches the ATLAS two photon result nearly exactly, is about four standard deviations away from the CMS two photon result, about three standard deviations away from the ALTAS four lepton result and within one standard deviation of the CMS four lepton result. Given the results and their spread of values, I believe it is entirely possible that further experimentation will show a Higgs mass close to 126 GeV/c^2 because so many other experimental results are lining up to support the ABC Preon Model.
One of the reasons for my optimism that the eventual Higgs mass will be close to 126 GeV/c^2 comes from what happened with the W, Z, and top quark masses. Several years ago the derived masses of the A, B and C preons, (which are based on experimental values of the W mass, Z mass and deep inelastic scattering momentum partitions) led to a top quark prediction that was off by about 1 GeV/c^2. With the passage of time, further experimentation led to better estimates for the W, Z, and top quark masses. And with those better mass estimates the ABC Preon Model prediction is now in excellent agreement with measurements of what is known as the top quark mass. I believe a similar evolution will be seen in the mass of what is known as the Higgs, eventually bringing it into agreement with the prediction of the ABC Preon Model.
16. A Needed Improvement.
In the threads so far it has been shown that the ABC Preon Model dovetails nicely into the power of the Standard Model, and that the ABC Preon Model is simpler in its underlying description of nature. The previous thread lists numerous predictions for new physics that are expected to be observed in the future, many of which are predictions beyond what the Standard Model anticipates. Therefore the ABC Preon Model is presently in the position of being a good start at a viable alternative to the Standard Model. Yet the ABC Preon Model certainly has opportunity for improvement.
The biggest area of needed improvement is that the ABC Preon Model has very limited calculational abilities. Ideally, there should be some underlying theory that can be used to derive the masses of the various composite particles that are known to exist. Note that the Standard Model also has difficulties in this regard, but that the Standard Model is further along with its theory of quantum chromodynamics.
At present, the ABC Preon Model is a model, not a theory. It does have some high level calculational ability that enables many predictions that indicate that it may be a correct model of nature, but there is no dynamics as of yet. The calculational ability that it does have comes about simply because once preons are freed, the cumulative mass of the preons so freed lead to predictions on that basis alone.
To go beyond a simple model we need to develop an underlying theory as well. Yet there is a major problem in arriving at an underlying theory, and that involves the strength of the binding, which precludes a typical perturbation analysis. The leptons are modeled as simple two body states, and yet finding a theoretical framework for deriving lepton masses has proven elusive.
In another thread I outlined the derivation of an equation for a non-perturbative, high-velocity, quantum mechanics that offers some hope for attacking the problem of a theoretical underpinning for the ABC Preon Model. The goal of that approach is to achieve something similar to what is achieved by the very successful quantum mechanical treatment of the hydrogen atom. I spent a couple of years looking for an analytic solution to the equations, but so far I have been unable to find one. I now believe that numerical techniques are likely required to solve the equation.
One problem with the development of the ABC Preon Model is that it has only been pursued by a single individual for about three years of cumulative effort, whereas the Standard Model has been pursued by a large community of physicists for decades. Hence, it of course stands to reason that the ABC Preon Model remains in its embryonic state, and opportunity exists for improvement. My request of the high energy physics community is that they not look for the first possible reason to reject the model and then consign it to the heap of nutty ideas, but rather that the high energy community point out reasons for rejection and then work to understand how to possibly overcome those problems. Keep in mind that the Standard Model grew in just that way.
The difference of course is that the Standard Model has been the leading model for decades, and so when problems arose it was viewed as a great achievement to overcome such problems. The problems were not used to condemn the entire Standard Model. As one example of how this approach can be applied to the ABC Preon Model, consider the top quark analysis presented in thread 12. At first I believed that since the ABC Preon Model does not allow a quark at such a high mass that this was a severe indictment against the model. But upon further reflection, I understood how the signature naturally came about. I suspect that a similar process may be required when new problems arise. Of course it may be that salvation of the model requires that some new particle be proposed at some point. And while that would be somewhat regrettable, it would not be surprising. Note that every theory of matter has suffered from just that problem in the past. But such a fate does not mean that we should abandon the theory completely, as it still can be very useful. For instance, one need look no further than the Standard Model itself.
In the threads so far it has been shown that the ABC Preon Model dovetails nicely into the power of the Standard Model, and that the ABC Preon Model is simpler in its underlying description of nature. The previous thread lists numerous predictions for new physics that are expected to be observed in the future, many of which are predictions beyond what the Standard Model anticipates. Therefore the ABC Preon Model is presently in the position of being a good start at a viable alternative to the Standard Model. Yet the ABC Preon Model certainly has opportunity for improvement.
The biggest area of needed improvement is that the ABC Preon Model has very limited calculational abilities. Ideally, there should be some underlying theory that can be used to derive the masses of the various composite particles that are known to exist. Note that the Standard Model also has difficulties in this regard, but that the Standard Model is further along with its theory of quantum chromodynamics.
At present, the ABC Preon Model is a model, not a theory. It does have some high level calculational ability that enables many predictions that indicate that it may be a correct model of nature, but there is no dynamics as of yet. The calculational ability that it does have comes about simply because once preons are freed, the cumulative mass of the preons so freed lead to predictions on that basis alone.
To go beyond a simple model we need to develop an underlying theory as well. Yet there is a major problem in arriving at an underlying theory, and that involves the strength of the binding, which precludes a typical perturbation analysis. The leptons are modeled as simple two body states, and yet finding a theoretical framework for deriving lepton masses has proven elusive.
In another thread I outlined the derivation of an equation for a non-perturbative, high-velocity, quantum mechanics that offers some hope for attacking the problem of a theoretical underpinning for the ABC Preon Model. The goal of that approach is to achieve something similar to what is achieved by the very successful quantum mechanical treatment of the hydrogen atom. I spent a couple of years looking for an analytic solution to the equations, but so far I have been unable to find one. I now believe that numerical techniques are likely required to solve the equation.
One problem with the development of the ABC Preon Model is that it has only been pursued by a single individual for about three years of cumulative effort, whereas the Standard Model has been pursued by a large community of physicists for decades. Hence, it of course stands to reason that the ABC Preon Model remains in its embryonic state, and opportunity exists for improvement. My request of the high energy physics community is that they not look for the first possible reason to reject the model and then consign it to the heap of nutty ideas, but rather that the high energy community point out reasons for rejection and then work to understand how to possibly overcome those problems. Keep in mind that the Standard Model grew in just that way.
The difference of course is that the Standard Model has been the leading model for decades, and so when problems arose it was viewed as a great achievement to overcome such problems. The problems were not used to condemn the entire Standard Model. As one example of how this approach can be applied to the ABC Preon Model, consider the top quark analysis presented in thread 12. At first I believed that since the ABC Preon Model does not allow a quark at such a high mass that this was a severe indictment against the model. But upon further reflection, I understood how the signature naturally came about. I suspect that a similar process may be required when new problems arise. Of course it may be that salvation of the model requires that some new particle be proposed at some point. And while that would be somewhat regrettable, it would not be surprising. Note that every theory of matter has suffered from just that problem in the past. But such a fate does not mean that we should abandon the theory completely, as it still can be very useful. For instance, one need look no further than the Standard Model itself.
17. Comparison to the Competition.
In this thread, we'll compare the ABC Preon Model to its competition.
Preon models have been around since at least 1974 which is the date of publication of a paper by Pati and Salam that proposed a substructure to quarks and leptons. A book on preon theories was published in 1992 by D'Souza and Kalman, and I would refer you to that book as a good place to read about preon history.
There are, of course, many, many theories of elementary particles that have been put forth by individuals over the past several decades. It is really quite a cottage industry. As a reviewer for Physics Essays, I saw many such efforts. The vast majority are dismissed as the works of cranks and crackpots, and for the vast majority that it is a fair judgement. Most of these works have a common theme. They generally start out with some ill defined concepts that the author feels are quite profound. The ill defined concepts are then infinitely malleable so that an exact calculation of the mass of every known particle can be obtained to the limit available on the author's calculational device. Almost without exception, these efforts to explain nature yield no new predictions for results not yet known, and the author will even claim to be able to explain anything new once it has occurred. It is this large number of crackpot efforts that makes it very difficult to get any new radical idea heard, since scientists generally (and often correctly) reject any such idea quickly once it sounds sufficiently different than the Standard Model.
And yet the primary competition to any preon model is the Standard Model itself, which in fact suffers to some degree from many of the same flaws as do the crackpot efforts. The Standard Model has a very large number of arbitrary inputs, and has been modified over time whenever a new result was discovered that was outside of its original bounds. But while the Standard Model does commit the same kind of sins as the crackpot efforts, there is a large difference in scope. The Standard Model has been modified relatively rarely, and does not need a modification for each and every new experimental result. And the Standard Model has predicted some phenomena before they were discovered. So while the Standard Model does have its issues, any true judgement must be that it is far superior to the vast majority of its competition.
This series of threads has presented an overview of the ABC Preon Model. While it is in its early stages of development, and while it has some opportunities for improvement, there are many philosophical advantages that the ABC Preon Model has that will now be emphasized.
The first advantage is that the ABC Preon Model dovetails nicely into the Standard Model as a means to explain the various particles and forces known to exist. The ABC Preon Model is, after all, a preon model. This means that it proposes the existence of a smaller number of new particles that can be used to construct the known, existing ones. Quarks are understood to be quantum states of a C preon bound to either an A or a B preon, while leptons are understood to be quantum states of an anti-A preon bound to a B preon. Hence, the vast majority of the Standard Model success can be transferred into the ABC Preon Model. Leptons still exist, and quarks can still be used as handy names for the categorization of more complicated hadrons.
Another advantage of the ABC Preon Model is simplicity, as the 36 quarks and 12 leptons of the standard model have been replaced by the 6 ABC preon particles. Historically, the finding of such simplicity generally leads to advancement in our knowledge about our universe.
The next significant philosophical advance of the ABC Preon Model is that it reduces the number of forces understood to exist in nature. Gravity and electromagnetism remain unchanged as one goes from the Standard Model to the ABC Preon model. A force proposed to be carried by the neutrino is postulated to be the force that binds preons into leptons and also binds preons into hadrons. But the weak force has been identified not as a force, but rather as another of nature's examples of quantum tunneling.
In addition to the advantage of reducing the number of forces, the elimination of the weak force is further advantageous in that it restores the attribute of direction to all known forces. In the Standard Model the weak force does not have a direction, while the other forces do. In the ABC Preon Model all forces have direction.
Low energy weak interactions are governed by a process mediated by the masses of the weak bosons in standard theory. In the ABC Preon Model those mass relations are again seen to come into play during the quantum tunneling of the preons, as the virtual mass of the tunneled states are similar to the masses of the theorized weak bosons.
Another advantage is that the ABC Preon Model allows for an entire quantum number, color, to be set aside. Of course the new "neutrinic charge" has been added to the discussion, but by eliminating color, one can also eliminate the eight gluons from the mix of elementary particles.
A further advantage of the ABC Preon Model is that hadronic matter and leptonic matter are each now seen to be made up from the same preonic material, rather than having a disjoint set of constituents as is the case for the Standard Model's description of how particles are constructed.
Importantly, the ABC Preon Model leads to a clear understanding as to why nature has several generations of particles that are otherwise identical. We have seen that the massive leptons are each various excited states of the same basic bound preon structure, while the various quark flavors are simply various excited states of basic bound preon structures as well.
Also, it is important to note that the elementary particles predicted by the ABC Preon Model can, in principle, be isolated, whereas the quarks of the Standard Model cannot be. In fact, when looked at through the ABC Preon Model we can see that the A, B, and C preons have already been isolated, as that is what happened when the W, Z and top particles were discovered.
Lastly, the ABC Preon Model has predictive power. In thread 15 it is shown how the ABC Preon Model predicts several new experimental results for future high energy physics experiments. This can be done, despite the lack of an underlying dynamical theory, from the simple realization that free preons of known masses are being formed in high energy collisions. Recall from thread 15 that there are a total of 18 predictions and of those, nine are already seen or expected. (Z pairs, W pairs, WZ events are seen or expected. The W, Z, top and Higgs events and two independent deep inelastic scattering ratios have been seen.) The ABC Preon Model fits 8 of those 9 events nearly perfectly, and the ninth - the Higgs Mass - is fit to within three and a half standard deviations of measurement. All these fits to the data are done with only three free parameters (the A, B and C masses). This quantitative evidence, coupled with the qualitative evidence discussed above, lends credence to the proposition that the ABC Preon Model is a correct model for what makes up our world.
In this thread, we'll compare the ABC Preon Model to its competition.
Preon models have been around since at least 1974 which is the date of publication of a paper by Pati and Salam that proposed a substructure to quarks and leptons. A book on preon theories was published in 1992 by D'Souza and Kalman, and I would refer you to that book as a good place to read about preon history.
There are, of course, many, many theories of elementary particles that have been put forth by individuals over the past several decades. It is really quite a cottage industry. As a reviewer for Physics Essays, I saw many such efforts. The vast majority are dismissed as the works of cranks and crackpots, and for the vast majority that it is a fair judgement. Most of these works have a common theme. They generally start out with some ill defined concepts that the author feels are quite profound. The ill defined concepts are then infinitely malleable so that an exact calculation of the mass of every known particle can be obtained to the limit available on the author's calculational device. Almost without exception, these efforts to explain nature yield no new predictions for results not yet known, and the author will even claim to be able to explain anything new once it has occurred. It is this large number of crackpot efforts that makes it very difficult to get any new radical idea heard, since scientists generally (and often correctly) reject any such idea quickly once it sounds sufficiently different than the Standard Model.
And yet the primary competition to any preon model is the Standard Model itself, which in fact suffers to some degree from many of the same flaws as do the crackpot efforts. The Standard Model has a very large number of arbitrary inputs, and has been modified over time whenever a new result was discovered that was outside of its original bounds. But while the Standard Model does commit the same kind of sins as the crackpot efforts, there is a large difference in scope. The Standard Model has been modified relatively rarely, and does not need a modification for each and every new experimental result. And the Standard Model has predicted some phenomena before they were discovered. So while the Standard Model does have its issues, any true judgement must be that it is far superior to the vast majority of its competition.
This series of threads has presented an overview of the ABC Preon Model. While it is in its early stages of development, and while it has some opportunities for improvement, there are many philosophical advantages that the ABC Preon Model has that will now be emphasized.
The first advantage is that the ABC Preon Model dovetails nicely into the Standard Model as a means to explain the various particles and forces known to exist. The ABC Preon Model is, after all, a preon model. This means that it proposes the existence of a smaller number of new particles that can be used to construct the known, existing ones. Quarks are understood to be quantum states of a C preon bound to either an A or a B preon, while leptons are understood to be quantum states of an anti-A preon bound to a B preon. Hence, the vast majority of the Standard Model success can be transferred into the ABC Preon Model. Leptons still exist, and quarks can still be used as handy names for the categorization of more complicated hadrons.
Another advantage of the ABC Preon Model is simplicity, as the 36 quarks and 12 leptons of the standard model have been replaced by the 6 ABC preon particles. Historically, the finding of such simplicity generally leads to advancement in our knowledge about our universe.
The next significant philosophical advance of the ABC Preon Model is that it reduces the number of forces understood to exist in nature. Gravity and electromagnetism remain unchanged as one goes from the Standard Model to the ABC Preon model. A force proposed to be carried by the neutrino is postulated to be the force that binds preons into leptons and also binds preons into hadrons. But the weak force has been identified not as a force, but rather as another of nature's examples of quantum tunneling.
In addition to the advantage of reducing the number of forces, the elimination of the weak force is further advantageous in that it restores the attribute of direction to all known forces. In the Standard Model the weak force does not have a direction, while the other forces do. In the ABC Preon Model all forces have direction.
Low energy weak interactions are governed by a process mediated by the masses of the weak bosons in standard theory. In the ABC Preon Model those mass relations are again seen to come into play during the quantum tunneling of the preons, as the virtual mass of the tunneled states are similar to the masses of the theorized weak bosons.
Another advantage is that the ABC Preon Model allows for an entire quantum number, color, to be set aside. Of course the new "neutrinic charge" has been added to the discussion, but by eliminating color, one can also eliminate the eight gluons from the mix of elementary particles.
A further advantage of the ABC Preon Model is that hadronic matter and leptonic matter are each now seen to be made up from the same preonic material, rather than having a disjoint set of constituents as is the case for the Standard Model's description of how particles are constructed.
Importantly, the ABC Preon Model leads to a clear understanding as to why nature has several generations of particles that are otherwise identical. We have seen that the massive leptons are each various excited states of the same basic bound preon structure, while the various quark flavors are simply various excited states of basic bound preon structures as well.
Also, it is important to note that the elementary particles predicted by the ABC Preon Model can, in principle, be isolated, whereas the quarks of the Standard Model cannot be. In fact, when looked at through the ABC Preon Model we can see that the A, B, and C preons have already been isolated, as that is what happened when the W, Z and top particles were discovered.
Lastly, the ABC Preon Model has predictive power. In thread 15 it is shown how the ABC Preon Model predicts several new experimental results for future high energy physics experiments. This can be done, despite the lack of an underlying dynamical theory, from the simple realization that free preons of known masses are being formed in high energy collisions. Recall from thread 15 that there are a total of 18 predictions and of those, nine are already seen or expected. (Z pairs, W pairs, WZ events are seen or expected. The W, Z, top and Higgs events and two independent deep inelastic scattering ratios have been seen.) The ABC Preon Model fits 8 of those 9 events nearly perfectly, and the ninth - the Higgs Mass - is fit to within three and a half standard deviations of measurement. All these fits to the data are done with only three free parameters (the A, B and C masses). This quantitative evidence, coupled with the qualitative evidence discussed above, lends credence to the proposition that the ABC Preon Model is a correct model for what makes up our world.
18. Requirements of a Good Theory. Concluding Remarks.
When looking back through this rather long thread, we can see that the philosophical advantages of the ABC Preon Model make it a very strong competitor in the arena of elementary particle theory. But to judge its place as a scientific theory, we should first list the criteria that any scientific theory must have, and then see how the ABC Preon Model meets those criteria. The criteria are:
1) It is important that any theory be mathematically and logically correct;
2) Any theory must be consistent with all known facts;
3) It is desirable that any theory lead to at least one testable difference between itself and its competitors; and
4) It is highly desirable that the theory be as simple as possible.
The first two criteria are absolutely necessary for any theory. (Although note that sometimes facts can change as more experimental evidence is added.) The last two are somewhat optional, but strongly desired criteria. Criteria 3 results from the fact that if no testable difference exists, either what is being presented is not really new, or it does not have sufficient rigor for what is a sound scientific theory.
Note that the world is full of crank theories that violate all four of the above criteria. A very frequent violation occurs in the third criteria, where authors claim to be able to always derive any mass or energy value from their theory, but never predict anything new. Such theories generally also have impossible-to-understand underpinnings, known only to their authors, and such theories should of course be rejected. But note also that the Standard Model suffers from weaknesses on both the third and fourth criteria itself. Over time the Standard Model has evolved whenever something was discovered outside of its tenets, and the Standard Model is now anything but simple. Of course nature may not be simple, and the Standard Model is a reasonably good map to what is known to exist, and for that reason the last two criteria are mentioned as highly desired, but they are not absolute requirements.
With the requirements for a good theory listed, we can now evaluate how well the ABC Preon Model has met those requirements. As can be seen in the preceding presentation, the ABC Preon Model is numerically and logically sound. The mathematical simplicity was introduced by the new concept of neutrinic charge, with the C particle having a plus three charge and the A and B each having minus one, and their antiparticles having the opposite charge. Once those simple charges were introduced, we saw how all massive leptons can be made by B particles binding to anti-A particles and how all quarks can be made as a binding of C particles to A or B particles. The second criteria is also met, since we have seen how the ABC Preon Model dovetails nicely into the Standard Model. Since the ABC Preon Model can be used to show how quarks and leptons are constructed, it can also construct those particles that are presently believed to be made up of those quarks and leptons. But the ABC Preon Model also meets the optional criteria as well. We have seen above that the ABC Preon Model predicts that a signature similar to that of the Z particle should occur around 69.6 GeV, and other predictions for new physics are described above as well. Additionally, neutrino oscillations were qualitatively predicted by the model. Finally, the ABC Preon Model clearly meets the desired criterion of simplicity, and in this regard it is time to put it in its historic place by reviewing the history of mankind's belief in what the world is made of.
The first model of what the world is made of was the Fire, Earth, Air and Water model of the ancient Greeks:
The model of the ancient Greeks required additional elements and modifications of the original elements and eventually Mendeleev produced a periodic table of the elements with 92 basic particles making up our world:
A great simplification of our view of what makes up our world was enabled by the observation that the elements of Mendeleev are themselves composed of protons, neutrons and electrons, with each element containing a different number of these particles internally. But even at this point in time a few additional particles were known to exist in addition to the proton, neutron and electron:
As scientists continued to experiment with high energy physics accelerators, many new particles were quickly found, leading to a particle zoo, a portion of which is shown here:
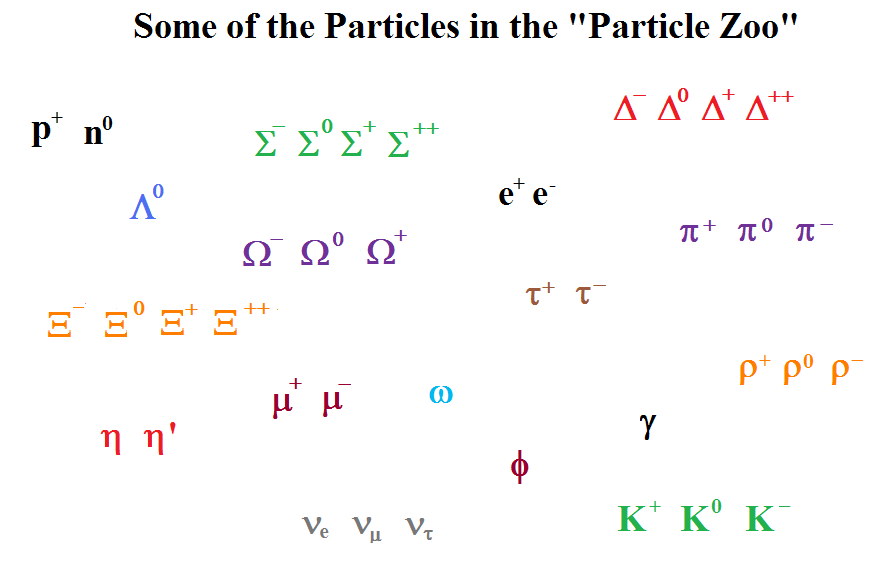
A great simplification was enabled by Murray Gell Mann and George Zweig's quark model, which explained the origin of some of the particles occupying the particle zoo by showing that they could be made up of precursor particles. At first there were three quarks, along with a few leptons, but as experimentation continued additional quarks and leptons were added. Here is the present Standard Model for particle physics as advertised by its proponents:
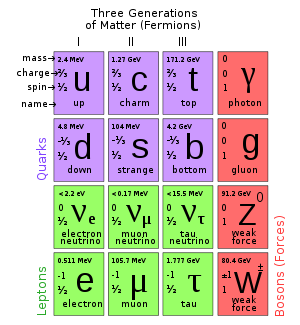
But a true picture of the Standard Model is more complex than what is advertised. Each quark comes in one of three colors, and each has an associated anti-quark that comes in one of three anti-colors. Each lepton comes with an anti-lepton partner, and the strong force is believed to be carried by eight gluons. This leaves a Standard Model that has over 60 elementary particles within it:
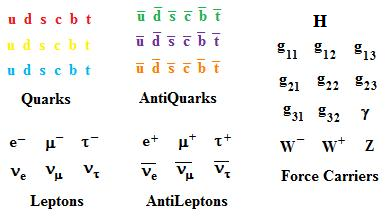
The next great simplification is the ABC Preon Model that has been presented here, which reduces the number of elementary particles from the 61 of the standard model to just eight. It can be seen that the ABC Preon Model is the next step in the pattern that has exhibited itself in mankind's searches for answers to the question of what the world is made of. Complexity gives way to simplicity as underlying patterns in the complexity are found. The simple model then gains complexity as additional experimentation occurs, until a new simple underlying pattern is found. Hence the ABC Preon Model is revealed as the latest example of a simplification of a previously complex model:
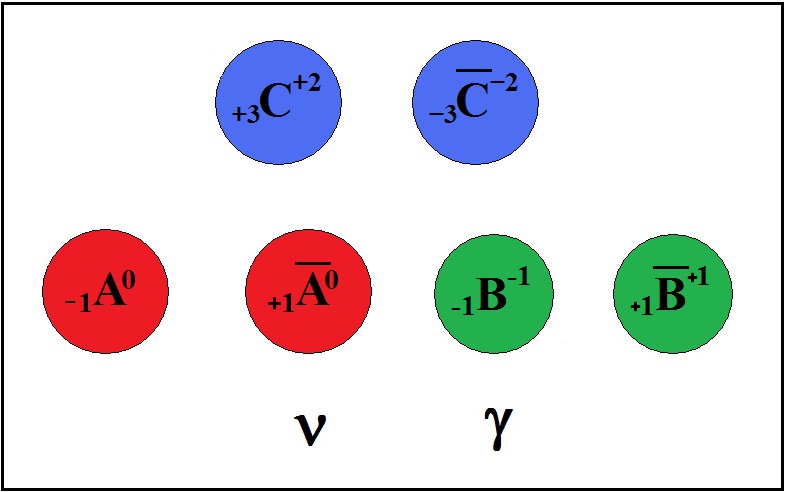
In conclusion, you have just seen how the ABC Preon Model is a scientifically correct, testable and simple model for elementary particle physics. The scientific correctness follows from the fact that it is shown how the preons can be used to construct the quarks and leptons of the Standard Model, which enables the ABC Preon Model to also share in the successes of the Standard Model. The testable nature of the ABC Preon Model exists in the fact that many predictions are made for events that are not predicted by the Standard Model. The simplicity is evident in the great reduction in the number of needed elementary particles, as well as the reduction in the number of forces at play in the universe. As with any new theory, opportunity exists for further research. The theory should be advanced with mathematical models, and one goal should be the accurate ability to derive masses for the massive leptons and hadrons. Experimentally, verification of the predicted preon signatures would be an important confirmation of the theory.
I hope you have enjoyed this presentation of the ABC Preon Model. Thank you for your time!
When looking back through this rather long thread, we can see that the philosophical advantages of the ABC Preon Model make it a very strong competitor in the arena of elementary particle theory. But to judge its place as a scientific theory, we should first list the criteria that any scientific theory must have, and then see how the ABC Preon Model meets those criteria. The criteria are:
1) It is important that any theory be mathematically and logically correct;
2) Any theory must be consistent with all known facts;
3) It is desirable that any theory lead to at least one testable difference between itself and its competitors; and
4) It is highly desirable that the theory be as simple as possible.
The first two criteria are absolutely necessary for any theory. (Although note that sometimes facts can change as more experimental evidence is added.) The last two are somewhat optional, but strongly desired criteria. Criteria 3 results from the fact that if no testable difference exists, either what is being presented is not really new, or it does not have sufficient rigor for what is a sound scientific theory.
Note that the world is full of crank theories that violate all four of the above criteria. A very frequent violation occurs in the third criteria, where authors claim to be able to always derive any mass or energy value from their theory, but never predict anything new. Such theories generally also have impossible-to-understand underpinnings, known only to their authors, and such theories should of course be rejected. But note also that the Standard Model suffers from weaknesses on both the third and fourth criteria itself. Over time the Standard Model has evolved whenever something was discovered outside of its tenets, and the Standard Model is now anything but simple. Of course nature may not be simple, and the Standard Model is a reasonably good map to what is known to exist, and for that reason the last two criteria are mentioned as highly desired, but they are not absolute requirements.
With the requirements for a good theory listed, we can now evaluate how well the ABC Preon Model has met those requirements. As can be seen in the preceding presentation, the ABC Preon Model is numerically and logically sound. The mathematical simplicity was introduced by the new concept of neutrinic charge, with the C particle having a plus three charge and the A and B each having minus one, and their antiparticles having the opposite charge. Once those simple charges were introduced, we saw how all massive leptons can be made by B particles binding to anti-A particles and how all quarks can be made as a binding of C particles to A or B particles. The second criteria is also met, since we have seen how the ABC Preon Model dovetails nicely into the Standard Model. Since the ABC Preon Model can be used to show how quarks and leptons are constructed, it can also construct those particles that are presently believed to be made up of those quarks and leptons. But the ABC Preon Model also meets the optional criteria as well. We have seen above that the ABC Preon Model predicts that a signature similar to that of the Z particle should occur around 69.6 GeV, and other predictions for new physics are described above as well. Additionally, neutrino oscillations were qualitatively predicted by the model. Finally, the ABC Preon Model clearly meets the desired criterion of simplicity, and in this regard it is time to put it in its historic place by reviewing the history of mankind's belief in what the world is made of.
The first model of what the world is made of was the Fire, Earth, Air and Water model of the ancient Greeks:

The model of the ancient Greeks required additional elements and modifications of the original elements and eventually Mendeleev produced a periodic table of the elements with 92 basic particles making up our world:

A great simplification of our view of what makes up our world was enabled by the observation that the elements of Mendeleev are themselves composed of protons, neutrons and electrons, with each element containing a different number of these particles internally. But even at this point in time a few additional particles were known to exist in addition to the proton, neutron and electron:

As scientists continued to experiment with high energy physics accelerators, many new particles were quickly found, leading to a particle zoo, a portion of which is shown here:

A great simplification was enabled by Murray Gell Mann and George Zweig's quark model, which explained the origin of some of the particles occupying the particle zoo by showing that they could be made up of precursor particles. At first there were three quarks, along with a few leptons, but as experimentation continued additional quarks and leptons were added. Here is the present Standard Model for particle physics as advertised by its proponents:

But a true picture of the Standard Model is more complex than what is advertised. Each quark comes in one of three colors, and each has an associated anti-quark that comes in one of three anti-colors. Each lepton comes with an anti-lepton partner, and the strong force is believed to be carried by eight gluons. This leaves a Standard Model that has over 60 elementary particles within it:

The next great simplification is the ABC Preon Model that has been presented here, which reduces the number of elementary particles from the 61 of the standard model to just eight. It can be seen that the ABC Preon Model is the next step in the pattern that has exhibited itself in mankind's searches for answers to the question of what the world is made of. Complexity gives way to simplicity as underlying patterns in the complexity are found. The simple model then gains complexity as additional experimentation occurs, until a new simple underlying pattern is found. Hence the ABC Preon Model is revealed as the latest example of a simplification of a previously complex model:

In conclusion, you have just seen how the ABC Preon Model is a scientifically correct, testable and simple model for elementary particle physics. The scientific correctness follows from the fact that it is shown how the preons can be used to construct the quarks and leptons of the Standard Model, which enables the ABC Preon Model to also share in the successes of the Standard Model. The testable nature of the ABC Preon Model exists in the fact that many predictions are made for events that are not predicted by the Standard Model. The simplicity is evident in the great reduction in the number of needed elementary particles, as well as the reduction in the number of forces at play in the universe. As with any new theory, opportunity exists for further research. The theory should be advanced with mathematical models, and one goal should be the accurate ability to derive masses for the massive leptons and hadrons. Experimentally, verification of the predicted preon signatures would be an important confirmation of the theory.
I hope you have enjoyed this presentation of the ABC Preon Model. Thank you for your time!
new topics
-
Meta Llama local AI system is scary good
Science & Technology: 3 hours ago -
This is why ALL illegals who live in the US must go
Social Issues and Civil Unrest: 5 hours ago -
UK Borders are NOT Secure!
Social Issues and Civil Unrest: 6 hours ago -
Former ‘GMA Producer’ Sues NPR-Legacy Media Exposed
Propaganda Mill: 8 hours ago -
New Footage - Randy Rhoads 1979 LIVE Guitar Solo Footage at the Whisky - Pro Shot
Music: 9 hours ago -
Happy Hanukkah…
General Chit Chat: 9 hours ago
top topics
-
This is why ALL illegals who live in the US must go
Social Issues and Civil Unrest: 5 hours ago, 13 flags -
Former ‘GMA Producer’ Sues NPR-Legacy Media Exposed
Propaganda Mill: 8 hours ago, 8 flags -
A Merry Christmas.
General Chit Chat: 16 hours ago, 7 flags -
Happy Hanukkah…
General Chit Chat: 9 hours ago, 7 flags -
UK Borders are NOT Secure!
Social Issues and Civil Unrest: 6 hours ago, 6 flags -
New Footage - Randy Rhoads 1979 LIVE Guitar Solo Footage at the Whisky - Pro Shot
Music: 9 hours ago, 5 flags -
Meta Llama local AI system is scary good
Science & Technology: 3 hours ago, 4 flags
active topics
-
This is why ALL illegals who live in the US must go
Social Issues and Civil Unrest • 13 • : WeMustCare -
Meta Llama local AI system is scary good
Science & Technology • 13 • : gort69 -
Merry Christmas !!
General Chit Chat • 19 • : argentus -
Smartest Man in the World Tells His Theory About What Happens At Death
Philosophy and Metaphysics • 54 • : TzarChasm -
Panamanian President-“every square meter” of the Panama Canal belongs to Panama.
New World Order • 46 • : fringeofthefringe -
Orange County Makes Shoplifting a Felony
Other Current Events • 31 • : GENERAL EYES -
Happy Hanukkah…
General Chit Chat • 20 • : JJproductions -
NYPD arrests migrant who allegedly set woman on fire on subway train, watched her burn to death
Breaking Alternative News • 55 • : xuenchen -
Plane Crash Today --Azerbaijanian E190 passenger jet
Mainstream News • 36 • : Oldcarpy2 -
FAA Investigates Christmas Drone Show Gone Wrong in Orlando, FL 12/2024
Other Current Events • 11 • : ScottKirkland
