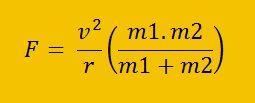It looks like you're using an Ad Blocker.
Please white-list or disable AboveTopSecret.com in your ad-blocking tool.
Thank you.
Some features of ATS will be disabled while you continue to use an ad-blocker.
11
share:
In this thread, I'll be deriving an alternative form of Newton's famous Law
of Universal Attraction and demonstrating that it's perfectly possible to achieve identical results with this alternative derivation. However,
there is one very important difference ... the need to invoke, or use, his Gravitational
Constant is completely eliminated. We therefore end up with an equation that calculates the force of attraction between an orbiting body and the
body being orbited exactly as does Newton's equation yet does so without having to rely on mysterious "gravitational constants" to make the
equation work.
Finally, I'll attempt to show the underlying basis of this Gravitational constant that appears not only in Newton's equation but in many others and how it can be derived quite simply as a result of nothing more sophisticated than one body orbiting another.
Mathematically, Newton's concept essentially states that every particle in the universe attracts every other particle in the universe with a force which is directly proportional to the product of their masses and inversely proportional to the square of the distance between them.
This concept, or Law of Universal Attraction is expressed by this equation:
[atsimg]http://files.abovetopsecret.com/images/member/9e0dff96e71e.jpg[/atsimg]
and here's an image to help get a more visual 'feel' for what this law is saying:
[atsimg]http://files.abovetopsecret.com/images/member/0c3850db6910.jpg[/atsimg]
The only term in the above equation that may cause some confusion is the G term that represents what's known as the Gravitational constant and basically was a scaling factor that had to be introduced to make the Law of Universal Gravitation work. Newton found it necessary to provide a "gravitational constant" so his results could be manipulated into the correct dimension of "force units".
Thus, if the gravitational constant (G) is removed from Newton's equation so it looks like this
[atsimg]http://files.abovetopsecret.com/images/member/646993035eb1.jpg[/atsimg]
the results from this equation are no longer in force dimensions.
So, even though Newton had no idea of where G comes from or what it represents, it's very apparent that the primary purpose for Newton needing to invent and include this gravitational constant (G) was to "manipulate or fudge" the otherwise incorrect results his equation would have given and convert them into the correct dimensions of force.
Ok, the above is a brief introduction and explanation regarding the Law of Universal Attraction and it's dependence on the gravitational constant G. In the following sections, I'll be demonstrating how it's possible to remove entirely the reference to G thereby producing an alternative form of Newton's equation that still produces the correct answers.
I'll start by making my 1st postulate that
In other words, I'm stating that there is a unique numeric constant associated with the sun and the planets orbiting it and there is another unique constant associated with each planet and the moons orbiting that planet.
This "orbiting" constant is the result of the product of the distance (the radius) between the orbiting body and the primary body being orbited, multiplied by the square of the orbiting body's velocity. If we represent this "orbiting" constant by the letter "k", we have the following equation:
[atsimg]http://files.abovetopsecret.com/images/member/9f8e3c2d5a94.jpg[/atsimg]
To show that such an "orbiting" constant actually does exist, I've generated a number of tables showing each primary body and every orbiting body associated with that primary. In other words, the sun and it's planets ... Earth and it's moon (plus The ISS and Hubble) ... Mars and it's two moons ... Jupiter and it's moons ... etc, etc
Note, in the following tables, the very minor discrepancies in "orbiting" constants within each system I believe are due solely to minor published inaccuracies in mean radii and mean velocities.
[atsimg]http://files.abovetopsecret.com/images/member/69c282b7569c.jpg[/atsimg]
As is clearly obvious, each primary body and it's associated orbiting body's has a unique and identifiable "orbiting" constant. The implication here is that any orbiting body that has a radius and velocity that produces the correct "orbiting" constant for the system that it's a part of, is therefore in a STABLE orbit. The corollary is that if the calculated "orbiting" constant value is not the correct one for the system, then that body's orbit is UNSTABLE.
Using the Earth as the primary body, we notice that both the ISS and Hubble have been placed in stable orbits around the Earth and their respective "orbiting" constants therefore match that of the moon's "orbiting" constant.
The above leads me to my 2nd postulate
Ok, moving on ...
Usually, when we need to calculate the velocity of a body that's orbiting another (usually much larger) body, the following equation might be used.
[atsimg]http://files.abovetopsecret.com/images/member/cb36d8a2cc7a.jpg[/atsimg]
It basically states that the velocity of an orbiting body is dependent on the combined masses (M) of the primary body and the orbiting body, the distance (r) separating the two bodies as well as the necessary inclusion of yet again, Newton's Gravitational constant (G).
Now this is a very interesting equation because it allows us to rearrange it's terms to arrive at another equation which has a surprising and unexpected, yet very direct link or relationship to the "orbiting constants" that I spent so much time discussing earlier on.
So starting with the original velocity equation, we rearrange terms
[atsimg]http://files.abovetopsecret.com/images/member/d819e5fea602.jpg[/atsimg]
The end result is an equation that gives the product of G and M in terms of a velocity (v) and a radius (r).
Continued next post ...
Finally, I'll attempt to show the underlying basis of this Gravitational constant that appears not only in Newton's equation but in many others and how it can be derived quite simply as a result of nothing more sophisticated than one body orbiting another.
Mathematically, Newton's concept essentially states that every particle in the universe attracts every other particle in the universe with a force which is directly proportional to the product of their masses and inversely proportional to the square of the distance between them.
This concept, or Law of Universal Attraction is expressed by this equation:
[atsimg]http://files.abovetopsecret.com/images/member/9e0dff96e71e.jpg[/atsimg]
and here's an image to help get a more visual 'feel' for what this law is saying:
[atsimg]http://files.abovetopsecret.com/images/member/0c3850db6910.jpg[/atsimg]
The only term in the above equation that may cause some confusion is the G term that represents what's known as the Gravitational constant and basically was a scaling factor that had to be introduced to make the Law of Universal Gravitation work. Newton found it necessary to provide a "gravitational constant" so his results could be manipulated into the correct dimension of "force units".
Thus, if the gravitational constant (G) is removed from Newton's equation so it looks like this
[atsimg]http://files.abovetopsecret.com/images/member/646993035eb1.jpg[/atsimg]
the results from this equation are no longer in force dimensions.
So, even though Newton had no idea of where G comes from or what it represents, it's very apparent that the primary purpose for Newton needing to invent and include this gravitational constant (G) was to "manipulate or fudge" the otherwise incorrect results his equation would have given and convert them into the correct dimensions of force.
Ok, the above is a brief introduction and explanation regarding the Law of Universal Attraction and it's dependence on the gravitational constant G. In the following sections, I'll be demonstrating how it's possible to remove entirely the reference to G thereby producing an alternative form of Newton's equation that still produces the correct answers.
I'll start by making my 1st postulate that
[Color=Yellow]for each and every primary body (e.g. the sun, earth, mars, jupiter, etc) that has a secondary body (or bodies) orbiting it, there exists a unique NUMERIC CONSTANT that is entirely specific to that primary body and it's associated orbiting bodies.
In other words, I'm stating that there is a unique numeric constant associated with the sun and the planets orbiting it and there is another unique constant associated with each planet and the moons orbiting that planet.
This "orbiting" constant is the result of the product of the distance (the radius) between the orbiting body and the primary body being orbited, multiplied by the square of the orbiting body's velocity. If we represent this "orbiting" constant by the letter "k", we have the following equation:
[atsimg]http://files.abovetopsecret.com/images/member/9f8e3c2d5a94.jpg[/atsimg]
To show that such an "orbiting" constant actually does exist, I've generated a number of tables showing each primary body and every orbiting body associated with that primary. In other words, the sun and it's planets ... Earth and it's moon (plus The ISS and Hubble) ... Mars and it's two moons ... Jupiter and it's moons ... etc, etc
Note, in the following tables, the very minor discrepancies in "orbiting" constants within each system I believe are due solely to minor published inaccuracies in mean radii and mean velocities.
[atsimg]http://files.abovetopsecret.com/images/member/69c282b7569c.jpg[/atsimg]
As is clearly obvious, each primary body and it's associated orbiting body's has a unique and identifiable "orbiting" constant. The implication here is that any orbiting body that has a radius and velocity that produces the correct "orbiting" constant for the system that it's a part of, is therefore in a STABLE orbit. The corollary is that if the calculated "orbiting" constant value is not the correct one for the system, then that body's orbit is UNSTABLE.
Using the Earth as the primary body, we notice that both the ISS and Hubble have been placed in stable orbits around the Earth and their respective "orbiting" constants therefore match that of the moon's "orbiting" constant.
The above leads me to my 2nd postulate
within every primary body/orbiting body system, stable orbits can ONLY exist when the individual "orbiting constants" match the "orbiting constant" specific to the system they inhabit. This is analogous to electrons in an atom being able to exist only in well defined and stable energy levels.
Ok, moving on ...
Usually, when we need to calculate the velocity of a body that's orbiting another (usually much larger) body, the following equation might be used.
[atsimg]http://files.abovetopsecret.com/images/member/cb36d8a2cc7a.jpg[/atsimg]
It basically states that the velocity of an orbiting body is dependent on the combined masses (M) of the primary body and the orbiting body, the distance (r) separating the two bodies as well as the necessary inclusion of yet again, Newton's Gravitational constant (G).
Now this is a very interesting equation because it allows us to rearrange it's terms to arrive at another equation which has a surprising and unexpected, yet very direct link or relationship to the "orbiting constants" that I spent so much time discussing earlier on.
So starting with the original velocity equation, we rearrange terms
[atsimg]http://files.abovetopsecret.com/images/member/d819e5fea602.jpg[/atsimg]
The end result is an equation that gives the product of G and M in terms of a velocity (v) and a radius (r).
Continued next post ...
Continued from previous post ...
Now if you've been paying attention, you'll recall that the "orbiting constant" equation I gave earlier
[atsimg]http://files.abovetopsecret.com/images/member/9f8e3c2d5a94.jpg[/atsimg]
also has the identical velocity and radius terms ... but in that equation, we saw that it's purpose was to produce a "unique constant" for every primary body/orbiting body system (as evidenced in the previous tables).
This means that both equations are equivalent as shown here
[atsimg]http://files.abovetopsecret.com/images/member/dfbcd3fe0846.jpg[/atsimg]
Rearranging the terms of that last equation
[atsimg]http://files.abovetopsecret.com/images/member/91975f473f00.jpg[/atsimg]
Ok, we're finally almost there !
We can use the above "modified" equation for G and substitute it back into Newton's original Law of Universal Attraction equation as follows
[atsimg]http://files.abovetopsecret.com/images/member/8b32bff52fbb.jpg[/atsimg]
Thats it, we've finally arrived at an alternative version of Newton's Law of Universal Attraction MINUS his famous Gravitational constant.
Instead, we have an equivalent equation that uses the velocity of the orbiting body, the primary and orbiting masses, and the distance between the two masses.
[atsimg]http://files.abovetopsecret.com/images/member/d1b81d325679.jpg[/atsimg]
You're probably saying "ok, that's all well and good but does it produce identical results to Newton's original equation ?"
Let's give it a try and calculate the force between the sun and the earth.
We'll use the following values:
[atsimg]http://files.abovetopsecret.com/images/member/60069a009904.jpg[/atsimg]
As the above shows, the modified form of Newton's original equation produces IDENTICAL results ... and without needing to know that such a thing as a Gravitational constant even exists !
So in conclusion, I'll be the 1st to admit that the above is nothing earth-shattering and won't be turning physics on it's head. But it is an interesting exercise showing that firstly, we can duplicate Newton's results without invoking a mysterious Gravitational constant to get the correct results. Secondly, that there is a little known constant that I've called the "orbiting constant" that shows that each and every orbital system has it's own "unique" signature. And thirdly, that orbiting bodies such as planets and moons apparently have, based on this "orbiting constant", levels of stable orbits similar in some ways to the stable energy levels occupied by electrons around the atom.
Now if you've been paying attention, you'll recall that the "orbiting constant" equation I gave earlier
[atsimg]http://files.abovetopsecret.com/images/member/9f8e3c2d5a94.jpg[/atsimg]
also has the identical velocity and radius terms ... but in that equation, we saw that it's purpose was to produce a "unique constant" for every primary body/orbiting body system (as evidenced in the previous tables).
This means that both equations are equivalent as shown here
[atsimg]http://files.abovetopsecret.com/images/member/dfbcd3fe0846.jpg[/atsimg]
Rearranging the terms of that last equation
further more implies that Newton's famous Gravitational constant (G) is NOT itself a fundamental constant but is actually derived from the above "orbiting constant" simply being divided by the combined mass of the primary body being orbited and the orbiting body itself.
[atsimg]http://files.abovetopsecret.com/images/member/91975f473f00.jpg[/atsimg]
Ok, we're finally almost there !
We can use the above "modified" equation for G and substitute it back into Newton's original Law of Universal Attraction equation as follows
[atsimg]http://files.abovetopsecret.com/images/member/8b32bff52fbb.jpg[/atsimg]
Thats it, we've finally arrived at an alternative version of Newton's Law of Universal Attraction MINUS his famous Gravitational constant.
Instead, we have an equivalent equation that uses the velocity of the orbiting body, the primary and orbiting masses, and the distance between the two masses.
[atsimg]http://files.abovetopsecret.com/images/member/d1b81d325679.jpg[/atsimg]
You're probably saying "ok, that's all well and good but does it produce identical results to Newton's original equation ?"
Let's give it a try and calculate the force between the sun and the earth.
We'll use the following values:
[atsimg]http://files.abovetopsecret.com/images/member/60069a009904.jpg[/atsimg]
As the above shows, the modified form of Newton's original equation produces IDENTICAL results ... and without needing to know that such a thing as a Gravitational constant even exists !
So in conclusion, I'll be the 1st to admit that the above is nothing earth-shattering and won't be turning physics on it's head. But it is an interesting exercise showing that firstly, we can duplicate Newton's results without invoking a mysterious Gravitational constant to get the correct results. Secondly, that there is a little known constant that I've called the "orbiting constant" that shows that each and every orbital system has it's own "unique" signature. And thirdly, that orbiting bodies such as planets and moons apparently have, based on this "orbiting constant", levels of stable orbits similar in some ways to the stable energy levels occupied by electrons around the atom.
reply to post by tauristercus
I love your mathematical threads tauristercus. Your prime number thread and the Norway Spiral analysis threads were great, but I think this is even better. You aren't giving yourself enough credit on this one imo. You've just fashioned a whole new equation which works without the need of a gravitational constant, I think that's remarkable. I remember learning this equation back at school, it's crazy to think I could learn something like this on ATS. It's beautiful.
I love your mathematical threads tauristercus. Your prime number thread and the Norway Spiral analysis threads were great, but I think this is even better. You aren't giving yourself enough credit on this one imo. You've just fashioned a whole new equation which works without the need of a gravitational constant, I think that's remarkable. I remember learning this equation back at school, it's crazy to think I could learn something like this on ATS. It's beautiful.
edit on 7-9-2011 by ChaoticOrder because: spelling
Originally posted by ChaoticOrder
reply to post by tauristercus
I love your mathematical threads tauristercus. Your prime number thread and the Norway Spiral analysis threads were great, but I think this is even better. You aren't giving yourself enough credit on this one imo. You've just fashioned a whole new equation which works without the need of a gravitational constant, I think that's remarkable. I remember learning this equation back at school, it's crazy to think I could learn something like this on ATS. It's beautiful.
edit on 7-9-2011 by ChaoticOrder because: spelling
Thanks very much for the thumbs up ... definitely appreciated
However, I'm just someone that likes to dabble occasionally in a bit of low level math and see what jumps out of the hat. I'm more than certain that someone will come along shortly and shoot it right down
I just worked out the force between the Earth and Moon using your equation! Lets take a look.
Here are all the variables we need. NOTE: I prefer to use "d" instead of "r" in these equations.
G = 6.67x10^-11
v = 0.1023x10^4
m1 = 5.97x10^24
m2 = 7.35x10^22
d = 3.84x10^8
If we were using the original equation of F = G x (m1 x m2 / d^2) our calculations would go something like this:
Now if we do it using your altered equation of F = (v^2 / d) x ((m1 x m2) / (m1 + m2)) it would go something like this:
As we can see the answers are very close but slightly different. I noticed the the orbital velocity of the moon can change quite a bit. I assume the force exerted will change as the velocity changes, so your equation is probably much more precise than the typical equation is it not?
Here are all the variables we need. NOTE: I prefer to use "d" instead of "r" in these equations.
G = 6.67x10^-11
v = 0.1023x10^4
m1 = 5.97x10^24
m2 = 7.35x10^22
d = 3.84x10^8
If we were using the original equation of F = G x (m1 x m2 / d^2) our calculations would go something like this:
F = (6.67x10^-11) x ((5.97x10^24) x (7.35x10^22)) / (3.84x10^8)^2
F = 1.984837952x10^20
Now if we do it using your altered equation of F = (v^2 / d) x ((m1 x m2) / (m1 + m2)) it would go something like this:
F = ((0.1023x10^4)^2 / 3.84x10^8) x ((5.97x10^24) x (7.35x10^22) / (5.97x10^24) + (7.35x10^22))
F = 1.978760292x10^20
As we can see the answers are very close but slightly different. I noticed the the orbital velocity of the moon can change quite a bit. I assume the force exerted will change as the velocity changes, so your equation is probably much more precise than the typical equation is it not?
edit on 7-9-2011 by ChaoticOrder because: (no reason given)
To the OP great work! It checks out very nicely...
To the poster above me- with calculations such as these you would need to take significant figures into account...
To the poster above me- with calculations such as these you would need to take significant figures into account...
reply to post by tauristercus
Congratulations, you have just derived the Standard Gravitational Parameter.
Also, the M in
[atsimg]http://files.abovetopsecret.com/images/member/cb36d8a2cc7a.jpg[/atsimg]
is not the combined masses... it's the mass of the central body (the one being orbited).
And this is, in fact, not even the equation. There is no such equality. This is only an approximation. Your equation appears to work because the masses of the central bodies you're using are much larger than the masses of the orbiting objects. If you were using that equation to calculate the force between two bodies of similar mass, it would fail (as ChaoticOrder found). To account for such a situation, you need to include the mass of the smaller body:
[atsimg]http://files.abovetopsecret.com/images/member/4afabddd8971.png[/atsimg]
I think you'll find this doesn't reduce quite so nicely.
So, not only have you derived a parameter already in existence (the only difference is, what you called k, astrophysics calls μ.), you've also done so using a very limited form of the orbital velocity equation.
Congratulations, you have just derived the Standard Gravitational Parameter.
Also, the M in
[atsimg]http://files.abovetopsecret.com/images/member/cb36d8a2cc7a.jpg[/atsimg]
is not the combined masses... it's the mass of the central body (the one being orbited).
And this is, in fact, not even the equation. There is no such equality. This is only an approximation. Your equation appears to work because the masses of the central bodies you're using are much larger than the masses of the orbiting objects. If you were using that equation to calculate the force between two bodies of similar mass, it would fail (as ChaoticOrder found). To account for such a situation, you need to include the mass of the smaller body:
[atsimg]http://files.abovetopsecret.com/images/member/4afabddd8971.png[/atsimg]
I think you'll find this doesn't reduce quite so nicely.
So, not only have you derived a parameter already in existence (the only difference is, what you called k, astrophysics calls μ.), you've also done so using a very limited form of the orbital velocity equation.
edit on 7-9-2011 by CLPrime because: (no reason given)
Originally posted by Phage
Didn't we go through this before? Yes, yes we did.
www.abovetopsecret.com...
Interesting. Seems I showed up a couple months too late.
Tauristercus, I also want you to consider the following equation:
[atsimg]http://files.abovetopsecret.com/images/member/9773f902bab0.png[/atsimg]
This is one of the Friedmann equations, describing the universal conditions existing within a given cosmological model. Try rewriting G in terms of v²r/M and see how much sense it makes. I guarantee you, neither v, r, nor M have any place in this equation.
So is it more fun the second time around?
Originally posted by Phage
Didn't we go through this before? Yes, yes we did.
www.abovetopsecret.com...
Why start a new thread, what was wrong with the old one?
Besides the point that CLprime made showing the math is incorrect,, let's presume for a minute that the approach had been able to produce accurate results (which obviously it doesn't). Even in that case, there are over a trillion objects in the universe (probably more than that in just the Milky Way alone). Are you seriously suggesting that replacing one universal constant with over a trillion different constants, is somehow "better"? It seems much worse to me even if the approach had produced valid calculations. Given a choice between working with one constant or trillions of them, if both were equally accurate, it seems much simpler to use the one, doesn't it?
And as out very successful program of launching probes to other planets has shown, the one constant works pretty darn well, the pioneer anomaly notwithstanding (which may have been solved).
First, if you really developed a new theory of gravity. Why would you post it on ATS?
Second, this is your second thread on the same exact thing.
Third, if you aren't doing this just for stars and flags, or s and giggles. I'd recommend going to the doctor. It would seem that you are suffering from a type of serious delusion.
Second, this is your second thread on the same exact thing.
Third, if you aren't doing this just for stars and flags, or s and giggles. I'd recommend going to the doctor. It would seem that you are suffering from a type of serious delusion.
Using velocity of the orbiting body, the primary and orbiting masses, and the distance between the two masses to derive Newton's Universal Law of
Attraction without knowing the pesky G value could be quite handy for unmeasured masses and space travel.
Granted I would be relying on my nav robot to carry out the quadratics however this is a very nice move to determine the attraction factor. (If I ever get a chance to fly a shuttle.) (Yes I am a pilot)
Thank you for sharing. Will you be posting this in any journals? I think that it is worth sharing this with as large an audience as possible.
I have never felt comfortable with the spooky G I attribute the attractive force to resonance effect more than an unknown force. I do appreciate you sharing this information.
Granted I would be relying on my nav robot to carry out the quadratics however this is a very nice move to determine the attraction factor. (If I ever get a chance to fly a shuttle.) (Yes I am a pilot)
Thank you for sharing. Will you be posting this in any journals? I think that it is worth sharing this with as large an audience as possible.
I have never felt comfortable with the spooky G I attribute the attractive force to resonance effect more than an unknown force. I do appreciate you sharing this information.
Nice try. But you've got an error in your derivation as others pointed out. Additionaly you are restricting yourself to orbiting bodies. Newtons law
of gravitation works independent of the relative motion. Yes, you can measure gravitational attraction between two masses at rest.
new topics
-
The Popular Vote does not matter
US Political Madness: 58 minutes ago -
Gaetz withdraws from attorney general consideration
US Political Madness: 3 hours ago -
Bridgewater Triangle
General Chit Chat: 3 hours ago -
Is Russia Using a New Type of Beam Weapon Against Ukraine?
Weaponry: 5 hours ago -
Here is why Western leaders in NATO have zero fear of nuclear warfare. At all. Zero.
World War Three: 6 hours ago -
International Criminal Court Issues Arrest Warrant For Netanyahu
Breaking Alternative News: 7 hours ago -
racist rant, but she made the arguement to get rid of DEI
US Political Madness: 8 hours ago -
Well we know Putins ICBMs won't fail in their silos
World War Three: 9 hours ago
top topics
-
Well we know Putins ICBMs won't fail in their silos
World War Three: 9 hours ago, 12 flags -
racist rant, but she made the arguement to get rid of DEI
US Political Madness: 8 hours ago, 9 flags -
International Criminal Court Issues Arrest Warrant For Netanyahu
Breaking Alternative News: 7 hours ago, 8 flags -
Is Russia Using a New Type of Beam Weapon Against Ukraine?
Weaponry: 5 hours ago, 8 flags -
Gaetz withdraws from attorney general consideration
US Political Madness: 3 hours ago, 7 flags -
Bridgewater Triangle
General Chit Chat: 3 hours ago, 5 flags -
Here is why Western leaders in NATO have zero fear of nuclear warfare. At all. Zero.
World War Three: 6 hours ago, 4 flags -
Why isn't Psychiatry involved?
Social Issues and Civil Unrest: 15 hours ago, 4 flags -
Help in song interpretation
Music: 17 hours ago, 3 flags -
The Popular Vote does not matter
US Political Madness: 58 minutes ago, 3 flags
active topics
-
Well we know Putins ICBMs won't fail in their silos
World War Three • 115 • : KrustyKrab -
Gaetz ethics investigation results "hacked".
US Political Madness • 37 • : Owlwatcher -
International Criminal Court Issues Arrest Warrant For Netanyahu
Breaking Alternative News • 35 • : JJproductions -
Help in song interpretation
Music • 10 • : tarantulabite1 -
The Popular Vote does not matter
US Political Madness • 3 • : Vermilion -
Gaetz withdraws from attorney general consideration
US Political Madness • 12 • : argentus -
Russia Ukraine Update Thread - part 3
World War Three • 6861 • : Oldcarpy2 -
Is Russia Using a New Type of Beam Weapon Against Ukraine?
Weaponry • 13 • : YouSir -
Mood Music Part VI
Music • 3711 • : SLAYER69 -
-@TH3WH17ERABB17- -Q- ---TIME TO SHOW THE WORLD--- -Part- --44--
Dissecting Disinformation • 3340 • : Thoughtful3
11