Antarctica Is Losing So Much Ice It's Throwing Off Earth's Gravity

originally posted by: Infinitis

Antarctica Is Losing So Much Ice It's Throwing Off Earth's Gravity

a reply to: cuckooold

lmao!!!!!!!!!!!!!!!!!!!!!!

The things people will believe....

And your qualifications for this are?

As I said in my OP, there will be those who will ridicule this without offering anything of their own to counter the theory. Now whether it is true or not, respect is to be given to those who will approach the hypothesis in a manner such as to offer something of substance as to whether this be be true or false.
Referring to believers in anthropogenic warming as 'those on the global warming gravy train' are equally unhelpful as they simply attack those who accept scientific consensus. I would like to think that this study has been done in a scientic manner, and the article itself says;

And this revelation is backed up by the findings of a number of other scientific satellites. The joint US-German Grace satellite has been detecting similar gravitational disturbances — albeit at a much coarser resolution — for more than a decade and the ESA’s own CryoSat satellite has found that West Antarctic Ice Sheet’s rate of loss has tripled every year since 2009 and has caused the entire continent to shrink by 125 cubic kilometres a year since 2011.

These are gravitational disturbances, and if those who disbelieve have a compelling scientific argument why this is false they are welcome to offer their own hypotheses. Statements like 'LMAO' , and 'Oh, noes! Quick, let's tax everyone and we can stack the cash on the pole and fix the fluctuating gravity!
/facepalm ' offer little except an indication of certain individuals bias and unwillingness to do anything other than act like children saying 'Nya Nya Nya'.

Hmm... not sure how they came up with that... theory. One has been reading that the Antarctic region has been setting records as to the ice encircling the southernmost continent. Speaking last week with a Lt. Colonel with the USAF that is stationed there confirms it. The jury is still out on this one, so to speak, since there are too many atmospheric professionals engaged in that discussion that assert the presumption is false. Many are seeing the potential for another mini ice age.

The position that humans are causing the so called global warming "crisis" is vague at best. If indeed humans are contributing to warming phenomena (if it actually is occurring), one would assert that the so-called "carbon footprint" of individual humans is not the problem. While one should remain cognizant of his/her actions to minimize the potential to harm the environment... do no harm, the collective impact pales in comparison to the pollution perpetrated by human slaves who devote their energies to Corporations in return for paper debt instruments (Federal Reserve Notes)... unwittingly contributing to the pollution, possible global warming, and/or any other environmental malady one might choose to consider. The legal system(s) presume that if one does not object, he/she consents. So, in summary, while one might adhere to devoting their energies to improving the environment in their personal time... the contribution they make in their work-for-debt job far outweighs any positive influence they might have. Simply not considering that in reality their actions are part of the problem. Cognitive dissonance is far more easily ignored than confronted. The problems won't go away until there's a change in collective conscious thought.

originally posted by: LeviWardrobe
a reply to: Klassified
Seems like it eh? Indistinguishable from normal fluctuations? And what exactly are your qualifications? What makes you say that? Oh, a gut feeling or something. Gotcha. Thanks.

No snideness necessary. I'm glad there are people as smart as you here, to help not so smart folks like me understand these things.

a reply to: cuckooold

So OP is this ice and snow melting into space..............Common sense on this on. So if it is not melting away into space than the earth mass would remain the same. Gotta love progressive logic.

well if it's inland ice it'll be full soon.
The 2014 Antarctic winter shows record ice.
Most ice accumulation since Satellites began imaging it.

I guess these rules below apply to ice in the same way.
When the water is in ice form it's concentrated to one area (Antarctica in this case) and when it melts, it's dispersed in the oceans thereby having a localized gravitational anomaly because of the missing ice.
Makes sense to me
CLICK ME

Local variations in topography (such as the presence of mountains) and geology (such as the density of rocks in the vicinity) cause fluctuations in the Earth's gravitational field, known as gravitational anomalies. Some of these anomalies can be very extensive, resulting in bulges in sea level, and throwing pendulum clocks out of synchronisation.

The study of these anomalies forms the basis of gravitational geophysics. The fluctuations are measured with highly sensitive gravimeters, the effect of topography and other known factors is subtracted, and from the resulting data conclusions are drawn. Such techniques are now used by prospectors to find oil and mineral deposits. Denser rocks (often containing mineral ores) cause higher than normal local gravitational fields on the Earth's surface. Less dense sedimentary rocks cause the opposite.

Antartica was once ice free...and no due to humans...

While it's interesting to observe and record...it tells us nothing.

The problem is the sensational title of the thread:

"Antarctica Is Losing So Much Ice It's Throwing Off Earth's Gravity"

(however it IS the original title of the news article linked by the OP, and we are suppose to do that).

Stating that the Earth's gravity is being "thrown off", is suggesting that Earth's gravity has one value and is a constant. That is, and always has been, untrue.

The Earth's gravity has never been "in balance" as a uniform force all over it's surface:

A perfect sphere of spherically uniform density (density varies solely with distance from centre) would produce a gravitational field of uniform magnitude at all points on its surface, always pointing directly towards the sphere's centre. However, the Earth deviates slightly from this ideal, and there are consequently slight deviations in both the magnitude and direction of gravity across its surface.

Apparent gravity on the earth's surface varies by around 0.7%, from 9.7639 m/s2 on the Nevado Huascarán mountain in Peru to 9.8337 m/s2 at the surface of the Arctic Ocean.[5] In large cities, it ranges from 9.766 in Kuala Lumpur, Mexico City, and Singapore to 9.825 in Oslo and Helsinki.

Source

The news article was interesting enough and did not need some "Doom Porn" title, especially when the title is technically a lie.

The Earth's gravity has never been a single, balanced constant that has now suddenly become "thrown off". It varies all over it's surface (but not by any amount that we can actually feel), and as topographical features change (plates moving, volcanoes erupting, water moving, ice receeding or advancing), the gravity map of the Earth's surface has also change, and will continue to change as long as the Earth has active geology and weather.

ETA:

The title of the article should have been:

""Antarctica Is Losing So Much Ice It Has Changed The Local Gravity"

Our climate is in constant flux over eons. As we move through our galaxy and the universe, a cyclical process of climate and geological effect take place on our planet and every other planet and celestial body.

Jupiters storm has changed saturn hexagnol poles have changed antarctica never use to be covered in ice as evidence of the flora remnants and finds come on.

Our poles shift our continents drift our climate changes an all on a scale obviously unfathomable to many.

The sooner people get off the ledge and focus on those things they can truly affect the better all of us will truly be.

originally posted by: Grimpachi

So would you say because on the opposite side of the globe from antarctica which would be the Arctic N pole which is seeing a heavy loss of Ice each year would that would be adding to the changes in gravity?

"Heavy loss of ice each year???"

a reply to: SlapMonkey

SEA ICE

originally posted by: Kali74
a reply to: SlapMonkey



SEA ICE

Okay, let's take this step by step, Kali74:

From the original article and quote in the OP:

Rising sea levels inundating coastal cities are the least of our global warming problems. According to a new report by the European Space Agency, the loss of snowpack along the antarctic ice shelf is throwing off Earth’s gravitational field.

So, even though I'm no scientist by trade, let's look at this quote--an ice shelf is a FLOATING chunk of ice attached to land, often where a glacier meets the sea. Sea ice is FLOATING ice, therefore, an ice shelf IS sea ice.

Now, if I were to, let's say, post a link to an article that cites indisputible data showing that sea ice in both the arctic AND antarctic is increasing, would it be logical to respond to someone claiming that there is a...hold on, let me get the quote...

originally posted by: Grimpachi
So would you say because on the opposite side of the globe from antarctica which would be the Arctic N pole which is seeing a heavy loss of Ice each year would that would be adding to the changes in gravity?

...with said link showing them that they're incorrect? (emphasis in quote is mine)

That's rhetorical--I know the answer is yes.

What I still can't figure out is the reason for your response, which says nothing of significance nor has any point in this discussion, other than to point out that I was correct in discussing sea ice.

a reply to: SlapMonkey

For you to understand it would need to be taken in context with who I was responding to and my question in reference to inverse square law.

My understanding is gravity on one side of the earth can be affected by the amount of mass on the opposite.

It is no wonder you would be confused if you didn't get the context of my reply to stormcell BTW I am still hoping stormcell will reply. Only time will tell.

originally posted by: Grimpachi
a reply to: SlapMonkey

For you to understand it would need to be taken in context with who I was responding to and my question in reference to inverse square law.

My understanding is gravity on one side of the earth can be affected by the amount of mass on the opposite.

It is no wonder you would be confused if you didn't get the context of my reply to stormcell BTW I am still hoping stormcell will reply. Only time will tell.

Actually, the statement of: " gravity on one side of the earth can be affected by the amount of mass on the opposite" is false.

That's not how gravity works with a spherical object.

First, the Shell theorem.

inside a solid sphere of constant density the gravitational force varies linearly with distance from the centre, becoming zero by symmetry at the centre of mass.

For working with gravity of the Earth, we have to consider how gravity works with depth:

An approximate depth dependence of density in the Earth can be obtained by assuming that the mass is spherically symmetric (it depends only on depth, not on latitude or longitude). In such a body, the gravitational acceleration is towards the center. The gravity at a radius r depends only on the mass inside the sphere of radius r; all the contributions from outside cancel out. This is a consequence of the inverse-square law of gravitation.

All the formulas for finding the gravitational acceleration uses "r" or Radius. Not Diameter. Opposite ends of the Earth would the diameter, not the radius. As we get closer to the core of the Earth, the gravitational acceleration drops to 0:



So changes in topography on one side of the Earth can and will change the gravitational acceleration in that local area based upon increase/decrease of altitude or depth to the Earth's core, but it will not change the local gravitational acceleration opposite on the Earth of where the change happened at.

Changes in density can also do this (Ice becoming water, Oil deposits being drained, voids being dug aka tunnels), but again, it will not affect the opposite side of the Earth from where that is.

The biggest change anyone experiences (but again, is so small you won't feel it) is the change in depth and altitude. A volcano erupting and pouring lava on top of a land mass is not really adding any mass, as the lava itself was right under where you were. So the mass was already there.

However: it can change the elevation of the land, making it higher, so that the distance to the core is now greater, and now has you further from the core of the Earth technically.

a reply to: Grimpachi

That still doesn't change the veracity of my response concerning your claim.

I get that irregularly-shaped objects--the earth being every-so-slightly irregularly shaped (but not enought to cause any noticeable or major concern...it has a central point of gravity)--have offset or even multiple centers of gravity. That's not in question, here. Your claim concerning the arctic sea ice is.

Context of a response doesn't matter if the response is factually wrong.

a reply to: eriktheawful

Nice post.

a reply to: SlapMonkey

You want to play semantics? Not interested, knock yourself out though.

a reply to: eriktheawful

inside a solid sphere of constant density the gravitational force varies linearly with distance from the centre, becoming zero by symmetry at the centre of mass.

Changes in density can also do this (Ice becoming water, Oil deposits being drained, voids being dug aka tunnels), but again, it will not affect the opposite side of the Earth from where that is.

Thanks that is what I wanted to know. I assume by your response that the same will hold true even though the Earth is an oblate spheroid instead of a sphere.

Side question: Would the gravity experienced at the equator be less than at the poles?

a reply to: SlapMonkey

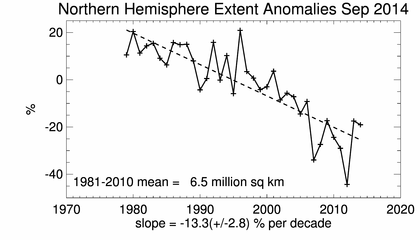
That's not in question, here. Your claim concerning the arctic sea ice is.

Every official report I have read says that the arctic is still losing ice not just the seasonal ice either but the ice that has built up over years.

On September 17, 2014, sea ice extent dropped to 5.02 million square kilometers (1.94 million square miles). This appears to have been the lowest extent of the year. In response to the setting sun and falling temperatures, ice extent will now climb through autumn and winter. However, a shift in wind patterns or a period of late season melt could still push the ice extent lower. The minimum extent was reached two days later than the 1981 to 2010 average minimum date of September 15.

nsidc.org...

originally posted by: Grimpachi
a reply to: eriktheawful

inside a solid sphere of constant density the gravitational force varies linearly with distance from the centre, becoming zero by symmetry at the centre of mass.

Changes in density can also do this (Ice becoming water, Oil deposits being drained, voids being dug aka tunnels), but again, it will not affect the opposite side of the Earth from where that is.

Thanks that is what I wanted to know. I assume by your response that the same will hold true even though the Earth is an oblate spheroid instead of a sphere.

Side question: Would the gravity experienced at the equator be less than at the poles?

Technically, yes:

Gravity at Latitude:

The surface of the Earth is rotating, so it is not an inertial frame of reference. At latitudes nearer the Equator, the outward centrifugal force produced by Earth's rotation is larger than at polar latitudes. This counteracts the Earth's gravity to a small degree – up to a maximum of 0.3% at the Equator – and reduces the apparent downward acceleration of falling objects.

The second major reason for the difference in gravity at different latitudes is that the Earth's equatorial bulge (itself also caused by inertia) causes objects at the Equator to be farther from the planet's centre than objects at the poles. Because the force due to gravitational attraction between two bodies (the Earth and the object being weighed) varies inversely with the square of the distance between them, an object at the Equator experiences a weaker gravitational pull than an object at the poles.

In combination, the equatorial bulge and the effects of the Earth's inertia mean that sea-level gravitational acceleration increases from about 9.780 m/s2 at the Equator to about 9.832 m/s2 at the poles, so an object will weigh about 0.5% more at the poles than at the Equator.

So things at the poles will weigh up to 0.005 times more. For example, a 150 pound person at the equator will weigh 150.75 pounds at the poles.

You're not really going to feel the change.

However, given deli prices of meat and cheese per pound, it would be cheaper at the equator.